Theoretical predictions and numerical simulations are used to determine the transition to bubble and conical vortex breakdown in low-Mach-number laminar axisymmetric variable-density swirling jets. A critical value of the swirl number 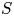 $S$ for the onset of the bubble (
$S$ for the onset of the bubble (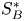 $S^*_B$) and the cone (
$S^*_B$) and the cone (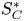 $S^*_C$) is determined as the jet-to-ambient density ratio
$S^*_C$) is determined as the jet-to-ambient density ratio  $\varLambda$ is varied, with the temperature dependence of the gas density and viscosity appropriate to that of air. The criterion of failure of the slender quasi-cylindrical approximation predicts
$\varLambda$ is varied, with the temperature dependence of the gas density and viscosity appropriate to that of air. The criterion of failure of the slender quasi-cylindrical approximation predicts 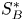 $S^*_B$ that decreases with increasing values of
$S^*_B$ that decreases with increasing values of  $\varLambda$ for a jet in solid-body rotation emerging sharply into a quiescent atmosphere. In addition, a new criterion for the onset of conical breakdown is derived from divergence of the initial value of the radial spreading rate of the jet occurring at
$\varLambda$ for a jet in solid-body rotation emerging sharply into a quiescent atmosphere. In addition, a new criterion for the onset of conical breakdown is derived from divergence of the initial value of the radial spreading rate of the jet occurring at  $S^*_C$, found to be independent of
$S^*_C$, found to be independent of 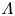 $\varLambda$, in an asymptotic analysis for small distances from the inlet plane. To maintain stable flow in the unsteady numerical simulations, an effective Reynolds number
$\varLambda$, in an asymptotic analysis for small distances from the inlet plane. To maintain stable flow in the unsteady numerical simulations, an effective Reynolds number 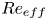 $Re_{eff}$, defined employing the geometric mean of the viscosity in the jet and ambient atmosphere, is fixed at
$Re_{eff}$, defined employing the geometric mean of the viscosity in the jet and ambient atmosphere, is fixed at  $Re_{eff}=200$ for all
$Re_{eff}=200$ for all  $\varLambda$. Similar to the theoretical predictions, numerical calculations of
$\varLambda$. Similar to the theoretical predictions, numerical calculations of  $S^*_B$ decrease monotonically as
$S^*_B$ decrease monotonically as 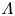 $\varLambda$ is increased. The critical swirl numbers for the cone,
$\varLambda$ is increased. The critical swirl numbers for the cone,  $S^*_C$, are found to depend strongly on viscous effects; for
$S^*_C$, are found to depend strongly on viscous effects; for 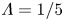 $\varLambda =1/5$, the low jet Reynolds number (51) at
$\varLambda =1/5$, the low jet Reynolds number (51) at 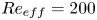 $Re_{eff}=200$ delays the transition to the cone, while for
$Re_{eff}=200$ delays the transition to the cone, while for  $\varLambda =5$ at
$\varLambda =5$ at  $Re_{eff}=200$, the large increase in kinematic viscosity in the external fluid produces a similar trend, significantly increasing
$Re_{eff}=200$, the large increase in kinematic viscosity in the external fluid produces a similar trend, significantly increasing 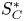 $S^*_C$.
$S^*_C$.
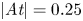 $\vert \textit {At} \vert = 0.25$
$\vert \textit {At} \vert = 0.25$