We show that for any set D of at least two digits in a given base b, almost all even integers taking digits only in D when written in base b satisfy the Goldbach conjecture. More formally, if $\mathcal {A}$ is the set of numbers whose digits base b are exclusively from D, almost all elements of $\mathcal {A}$ satisfy the Goldbach conjecture. Moreover, the number of even integers in $\mathcal {A}$ which are less than X and not representable as the sum of two primes is less than $|\mathcal {A}\cap \{1,\ldots ,X\}|^{1-\delta }$.

For $g \geqslant 2$, we show that the number of positive integers at most X which can be written as sum of two base g palindromes is at most ${X}/{\log^c X}$. This answers a question of Baxter, Cilleruelo and Luca.

For $\lambda \in (0,\,1/2]$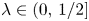 let $K_\lambda \subset \mathbb {R}$ be a self-similar set generated by the iterated function system $\{\lambda x,\, \lambda x+1-\lambda \}$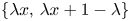. Given $x\in (0,\,1/2)$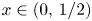, let $\Lambda (x)$ 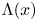be the set of $\lambda \in (0,\,1/2]$ 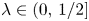such that $x\in K_\lambda$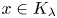. In this paper we show that $\Lambda (x)$ is a topological Cantor set having zero Lebesgue measure and full Hausdorff dimension. Furthermore, we show that for any $y_1,\,\ldots,\, y_p\in (0,\,1/2)$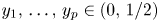 there exists a full Hausdorff dimensional set of $\lambda \in (0,\,1/2]$ 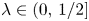such that $y_1,\,\ldots,\, y_p \in K_\lambda$.

The Thue–Morse sequence $\{t(n)\}_{n\geqslant 0}$ is the indicator function of the parity of the number of ones in the binary expansion of nonnegative integers n, where $t(n)=1$ (resp. $=0$) if the binary expansion of n has an odd (resp. even) number of ones. In this paper, we generalize a recent result of E. Miyanohara by showing that, for a fixed Pisot or Salem number $\beta>\sqrt {\varphi }=1.272019\ldots $, the set of the numbers

$$\begin{align*}1,\quad \sum_{n\geqslant1}\frac{t(n)}{\beta^{n}},\quad \sum_{n\geqslant1}\frac{t(n^2)}{\beta^{n}},\quad \dots, \quad \sum_{n\geqslant1}\frac{t(n^k)}{\beta^{n}},\quad \dots \end{align*}$$

$\mathbb {Q}(\beta )$

$\varphi :=(1+\sqrt {5})/2$

$k\geqslant 1$

$a_1,a_2,\ldots ,a_k\in \mathbb {Q}(\beta )$

$a_1t(n)+a_2t(n^2)+\cdots +a_kt(n^k)\}_{n\geqslant 1}$

We prove $\times a \times b$ measure rigidity for multiplicatively independent pairs when $a\in \mathbb {N}$ and $b>1$ is a ‘specified’ real number (the b-expansion of $1$ has a tail or bounded runs of $0$s) under a positive entropy condition. This is done by proving a mean decay of the Fourier series of the point masses average along $\times b$ orbits. We also prove a quantitative version of this decay under stronger conditions on the $\times a$ invariant measure. The quantitative version together with the $\times b$ invariance of the limit measure is a step toward a general Host-type pointwise equidistribution theorem in which the equidistribution is for Parry measure instead of Lebesgue. We show that finite memory length measures on the a-shift meet the mentioned conditions for mean convergence. Our main proof relies on techniques of Hochman.

For an integer $b\geq 2$, a positive integer is called a b-Niven number if it is a multiple of the sum of the digits in its base-b representation. In this article, we show that every arithmetic progression contains infinitely many b-Niven numbers.

We use bounds of character sums and some combinatorial arguments to show the abundance of very smooth numbers which also have very few nonzero binary digits.

We show, from a topological viewpoint, that most numbers are not normal in a strong sense. More precisely, the set of numbers $x \in (0,1]$ with the following property is comeager: for all integers $b\ge 2$ and $k\ge 1$ , the sequence of vectors made by the frequencies of all possibile strings of length k in the b-adic representation of x has a maximal subset of accumulation points, and each of them is the limit of a subsequence with an index set of nonzero asymptotic density. This extends and provides a streamlined proof of the main result given by Olsen (2004) in this Journal. We provide analogues in the context of analytic P-ideals and regular matrices.

We construct a geometrico-symbolic version of the natural extension of the random $\beta $-transformation introduced by Dajani and Kraaikamp [Random $\beta $-expansions. Ergod. Th. & Dynam. Sys. 23(2) (2003) 461–479]. This construction provides a new proof of the existence of a unique absolutely continuous invariant probability measure for the random $\beta $-transformation, and an expression for its density. We then prove that this natural extension is a Bernoulli automorphism, generalizing to the random case the result of Smorodinsky [$\beta $-automorphisms are Bernoulli shifts. Acta Math. Acad. Sci. Hungar. 24 (1973), 273–278] about the greedy transformation.

We investigate the leading digit distribution of the kth largest prime factor of n (for each fixed $k=1,2,3,\dots $ ) as well as the sum of all prime factors of n. In each case, we find that the leading digits are distributed according to Benford’s law. Moreover, Benford behavior emerges simultaneously with equidistribution in arithmetic progressions uniformly to small moduli.

When a page, represented by the interval $[0,1]$ , is folded right over left $n $ times, the right-hand fold contains a sequence of points. We specify these points using two different representation techniques, both involving binary signed-digit representations.

In this paper, we discuss a connection between geometric measure theory and number theory. This method brings a new point of view for some number-theoretic problems concerning digit expansions. Among other results, we show that for each integer k, there is a number $M>0$ such that if $b_{1},\ldots ,b_{k}$ are multiplicatively independent integers greater than M, there are infinitely many integers whose base $b_{1},b_{2},\ldots ,b_{k}$ expansions all do not have any zero digits.

The Thue–Morse sequence is a prototypical automatic sequence found in diverse areas of mathematics, and in computer science. We study occurrences of factors w within this sequence, or more precisely, the sequence of gaps between consecutive occurrences. This gap sequence is morphic; we prove that it is not automatic as soon as the length of w is at least $2$ , thereby answering a question by J. Shallit in the affirmative. We give an explicit method to compute the discrepancy of the number of occurrences of the block $\mathtt {01}$ in the Thue–Morse sequence. We prove that the sequence of discrepancies is the sequence of output sums of a certain base- $2$ transducer.

We generalize the greedy and lazy $\beta $ -transformations for a real base $\beta $ to the setting of alternate bases ${\boldsymbol {\beta }}=(\beta _0,\ldots ,\beta _{p-1})$ , which were recently introduced by the first and second authors as a particular case of Cantor bases. As in the real base case, these new transformations, denoted $T_{{\boldsymbol {\beta }}}$ and $L_{{\boldsymbol {\beta }}}$ respectively, can be iterated in order to generate the digits of the greedy and lazy ${\boldsymbol {\beta }}$ -expansions of real numbers. The aim of this paper is to describe the measure-theoretical dynamical behaviors of $T_{{\boldsymbol {\beta }}}$ and $L_{{\boldsymbol {\beta }}}$ . We first prove the existence of a unique absolutely continuous (with respect to an extended Lebesgue measure, called the p-Lebesgue measure) $T_{{\boldsymbol {\beta }}}$ -invariant measure. We then show that this unique measure is in fact equivalent to the p-Lebesgue measure and that the corresponding dynamical system is ergodic and has entropy $({1}/{p})\log (\beta _{p-1}\cdots \beta _0)$ . We give an explicit expression of the density function of this invariant measure and compute the frequencies of letters in the greedy ${\boldsymbol {\beta }}$ -expansions. The dynamical properties of $L_{{\boldsymbol {\beta }}}$ are obtained by showing that the lazy dynamical system is isomorphic to the greedy one. We also provide an isomorphism with a suitable extension of the $\beta $ -shift. Finally, we show that the ${\boldsymbol {\beta }}$ -expansions can be seen as $(\beta _{p-1}\cdots \beta _0)$ -representations over general digit sets and we compare both frameworks.

Let $g \geq 2$ be an integer. A natural number is said to be a base-g Niven number if it is divisible by the sum of its base-g digits. Assuming Hooley’s Riemann hypothesis, we prove that the set of base-g Niven numbers is an additive basis, that is, there exists a positive integer $C_g$ such that every natural number is the sum of at most $C_g$ base-g Niven numbers.

Let S be the sum-of-digits function in base 2, which returns the number of 1s in the base-2 expansion of a nonnegative integer. For a nonnegative integer t, define the asymptotic density

$${c_t} = \mathop {\lim }\limits_{N \to \infty } {1 \over N}|\{ 0 \le n < N:s(n + t) \ge s(n)\} |.$$

The level of distribution of a complex-valued sequence $b$ measures the quality of distribution of $b$ along sparse arithmetic progressions $nd+a$. We prove that the Thue–Morse sequence has level of distribution $1$, which is essentially best possible. More precisely, this sequence gives one of the first nontrivial examples of a sequence satisfying a Bombieri–Vinogradov-type theorem for each exponent $\theta <1$. This result improves on the level of distribution $2/3$ obtained by Müllner and the author. As an application of our method, we show that the subsequence of the Thue–Morse sequence indexed by $\lfloor n^c\rfloor$, where $1 < c < 2$, is simply normal. This result improves on the range $1 < c < 3/2$ obtained by Müllner and the author and closes the gap that appeared when Mauduit and Rivat proved (in particular) that the Thue–Morse sequence along the squares is simply normal.

Given a positive integer M and $q \in (1, M+1]$ we consider expansions in base q for real numbers $x \in [0, {M}/{q-1}]$ over the alphabet $\{0, \ldots , M\}$ . In particular, we study some dynamical properties of the natural occurring subshift $(\boldsymbol{{V}}_q, \sigma )$ related to unique expansions in such base q. We characterize the set of $q \in \mathcal {V} \subset (1,M+1]$ such that $(\boldsymbol{{V}}_q, \sigma )$ has the specification property and the set of $q \in \mathcal {V}$ such that $(\boldsymbol{{V}}_q, \sigma )$ is a synchronized subshift. Such properties are studied by analysing the combinatorial and dynamical properties of the quasi-greedy expansion of q. We also calculate the size of such classes as subsets of $\mathcal {V}$ giving similar results to those shown by Blanchard [ 10 ] and Schmeling in [ 36 ] in the context of $\beta $ -transformations.

Fix an alphabet $A=\{0,1,\ldots ,M\}$ with $M\in \mathbb{N}$. The univoque set $\mathscr{U}$ of bases $q\in (1,M+1)$ in which the number $1$ has a unique expansion over the alphabet $A$ has been well studied. It has Lebesgue measure zero but Hausdorff dimension one. This paper describes how the points in the set $\mathscr{U}$ are distributed over the interval $(1,M+1)$ by determining the limit

$$\begin{eqnarray}f(q):=\lim _{\unicode[STIX]{x1D6FF}\rightarrow 0}\dim _{\text{H}}(\mathscr{U}\cap (q-\unicode[STIX]{x1D6FF},q+\unicode[STIX]{x1D6FF}))\end{eqnarray}$$

$q\in (1,M+1)$

$f(q)>0$

$q\in \overline{\mathscr{U}}\backslash \mathscr{C}$

$\mathscr{C}$

$f$

$\mathscr{U}$

$\mathscr{U}$

We obtain a lower bound on the largest prime factor of the denominator of rational numbers in the Cantor set. This gives a stronger version of a recent result of Schleischitz [‘On intrinsic and extrinsic rational approximation to Cantor sets’, Ergodic Theory Dyn. Syst. to appear] obtained via a different argument.