Let ${{T}_{\Omega }}$ be the singular integral operator with kernel $\left( \Omega \left( x \right) \right)/{{\left| x \right|}^{n}}$, where $\Omega $ is homogeneous of degree zero, has mean value zero, and belongs to ${{L}^{q}}\left( {{S}^{n-1}} \right)$ for some $q\,\in \,\left( 1,\,\infty \right]$. In this paper, the authors establish the compactness on weighted ${{L}^{p}}$ spaces and the Morrey spaces, for the commutator generated by $\text{CMO}\left( {{\mathbb{R}}^{n}} \right)$ function and ${{T}_{\Omega }}$. The associated maximal operator and the discrete maximal operator are also considered.

Let $S$ be a surface of general type. In this article, when there exists a relatively minimal hyperelliptic fibration $f:\,S\,\to \,{{\mathbb{P}}^{1}}$ whose slope is less than or equal to four, we give a lower bound on the Euler–Poincaré characteristic of $S$. Furthermore, we prove that our bound is the best possible by giving required hyperelliptic fibrations.

We show that the assignment of the (left) completely bounded multiplier algebra $M_{cb}^{l}({{L}^{1}}(\mathbb{G}))$ to a locally compact quantum group $\mathbb{G}$, and the assignment of the intrinsic group, form functors between appropriate categories. Morphisms of locally compact quantum groups can be described by Hopf $*$-homomorphisms between universal ${{C}^{*}}$-algebras, by bicharacters, or by special sorts of coactions. We show that the whole theory of completely bounded multipliers can be lifted to the universal ${{C}^{*}}$-algebra level, and that the different pictures of both multipliers (reduced, universal, and as centralisers) and morphisms then interact in extremely natural ways. The intrinsic group of a quantum group can be realised as a class of multipliers, and so our techniques immediately apply. We also show how to think of the intrinsic group using the universal ${{C}^{*}}$-algebra picture, and then, again, how the differing views on the intrinsic group interact naturally with morphisms. We show that the intrinsic group is the “maximal classical” quantum subgroup of a locally compact quantum group, that it is even closed in the strong Vaes sense, and that the intrinsic group functor is an adjoint to the inclusion functor from locally compact groups to quantum groups.

We study in detail two row Springer fibres of even orthogonal type from an algebraic as well as a topological point of view. We show that the irreducible components and their pairwise intersections are iterated ${{\mathbb{P}}^{1}}$-bundles. Using results of Kumar and Procesi we compute the cohomology ring with its action of the Weyl group. The main tool is a type $\text{D}$ diagram calculus labelling the irreducible components in a convenient way that relates to a diagrammatical algebra describing the category of perverse sheaves on isotropic Grassmannians based on work of Braden. The diagram calculus generalizes Khovanov's arc algebra to the type $\text{D}$ setting and should be seen as setting the framework for generalizing well-known connections of these algebras in type $\text{A}$ to other types.

Let $\mathfrak{g}$ be a compact simple Lie algebra of dimension $d$. It is a classical result that the convolution of any $d$ non-trivial, $G$-invariant, orbital measures is absolutely continuous with respect to Lebesgue measure on $\mathfrak{g}$, and the sum of any $d$ non-trivial orbits has non-empty interior. The number $d$ was later reduced to the rank of the Lie algebra (or rank +1 in the case of type ${{A}_{n}}$). More recently, the minimal integer $k\,=\,k\left( X \right)$ such that the $k$-fold convolution of the orbital measure supported on the orbit generated by $X$ is an absolutely continuous measure was calculated for each $X\,\in \,\mathfrak{g}$.

In this paper $\mathfrak{g}$ is any of the classical, compact, simple Lie algebras. We characterize the tuples $\left( {{X}_{1}},\,.\,.\,.\,,\,{{X}_{L}} \right)$, with ${{X}_{i}}\,\in \,\mathfrak{g}$, which have the property that the convolution of the $L$-orbital measures supported on the orbits generated by the ${{X}_{i}}$ is absolutely continuous, and, equivalently, the sum of their orbits has non-empty interior. The characterization depends on the Lie type of $\mathfrak{g}$ and the structure of the annihilating roots of the ${{X}_{i}}$. Such a characterization was previously known only for type ${{A}_{n}}$.

Let $K/\mathbb{Q}$ be Galois and let 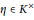$\eta \,\in \,{{K}^{\times }}$ be such that $\text{Re}{{\text{g}}_{\infty }}\left( \eta \right)\,\ne \,0$. We define the local 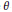$\theta $-regulator 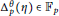$\Delta _{p}^{\theta }\left( \eta \right)\,\in \,{{\mathbb{F}}_{p}}$ for the ${{\mathbb{Q}}_{p}}$-irreducible characters 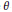$\theta $ of $G=\,\text{Gal}\left( K/\mathbb{Q} \right)$. Let 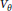${{V}_{\theta }}$ be the 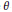$\theta $-irreducible representation. A linear representation ${{\mathfrak{L}}^{\theta }}\,\simeq \,\delta \,{{V}_{\theta }}$ is associated with $\Delta _{p}^{\theta }\left( \eta \right)$ whose nullity is equivalent to 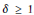$\delta \,\ge \,1$. Each $\Delta _{p}^{\theta }\left( \eta \right)$ yields $\text{R}eg_{p}^{\theta }\left( \eta \right)$ modulo $p$ in the factorization 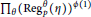${{\Pi }_{\theta }}{{\left( \text{Reg}_{p}^{\theta }\left( \eta \right) \right)}^{\phi \left( 1 \right)}}$ of 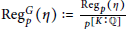$\text{Reg}_{p}^{G}\,\left( \eta \right)\,:=\frac{\text{Re}{{\text{g}}_{p}}\left( \eta \right)}{_{p}[K\,:\,\mathbb{Q}]}$ (normalized $p$-adic regulator). 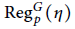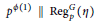From Prob $\left( \Delta _{p}^{\theta }\left( \eta \right)=0\,\text{and}\,{{\mathfrak{L}}^{\theta }}\simeq \delta {{V}_{\theta }} \right)\,\le {{p}^{-f{{\delta }^{2}}}}$ ($f\,\ge \,1$ is a residue degree) and the Borel-Cantelli heuristic, we conjecture that for $p$ large enough, $\text{Reg}_{p}^{G}\left( \eta \right)$ is a $p$-adic unit or ${{p}^{\phi \left( 1 \right)}}\,||\,\text{Reg}_{p}^{G}\left( \eta \right)$ (a single 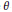$\theta $ with 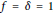$f\,=\,\delta \,=\,1$); this obstruction may be led assuming the existence of a binomial probability law confirmed through numerical studies (groups ${{C}_{3,}}\,{{C}_{5}},\,{{D}_{6}}$) is conjecture would imply that for all $p$ large enough, Fermat quotients, normalized $p$-adic regulators are $p$-adic units and that number fields are $p$-rational.We recall some deep cohomological results that may strengthen such conjectures.

Fontaine described the category of formal groups over the ring of Witt vectors over a finite field of characteristic $p$ with the aid of triples consisting of the module of logarithms, the Dieudonné module, and the morphism from the former to the latter. We propose an explicit construction for the kernels in this category in term of Fontaine's triples. The construction is applied to the formal norm homomorphism in the case of an unramified extension of ${{\mathbb{Q}}_{p}}$ and of a totally ramified extension of degree less or equal than $p$ . A similar consideration applied to a global extension allows us to establish the existence of a strict isomorphism between the formal norm torus and a formal group law coming from $L$ -series.

In this paper, we study the global regularity for regular Monge-Ampère type equations associated with semilinear Neumann boundary conditions. By establishing a priori estimates for second order derivatives, the classical solvability of the Neumann boundary value problem is proved under natural conditions. The techniques build upon the delicate and intricate treatment of the standard Monge-Ampère case by Lions, Trudinger, and Urbas in 1986 and the recent barrier constructions and second derivative bounds by Jiang, Trudinger, and Yang for the Dirichlet problem. We also consider more general oblique boundary value problems in the strictly regular case.

This article provides an account of the functorial correspondence between irreducible singular $G$-monopoles on ${{S}^{1}}\,\times \,\sum $ and $\vec{t}$-stable meromorphic pairs on $\sum $. A theorem of B.Charbonneau and J. Hurtubise is thus generalized here from unitary to arbitrary compact, connected gauge groups. The required distinctions and similarities for unitary versus arbitrary gauge are clearly outlined, and many parallels are drawn for easy transition. Once the correspondence theorem is complete, the spectral decomposition is addressed.

Let ${{C}^{M}}$ denote a Denjoy–Carleman class of ${{C}^{\infty }}$ functions (for a given logarithmically-convex sequence $M\,=\,\left( {{M}_{n}} \right))$. We construct: (1) a function in ${{C}^{M}}\left( \left( -1,\,1 \right) \right)$ that is nowhere in any smaller class; (2) a function on $\mathbb{R}$ that is formally ${{C}^{M}}$ at every point, but not in ${{C}^{M}}\left( \mathbb{R} \right)$; (3) (under the assumption of quasianalyticity) a smooth function on ${{\mathbb{R}}^{p}}\,\left( p\,\ge \,2 \right)$ that is ${{C}^{M}}$ on every ${{C}^{M}}$ curve, but not in ${{C}^{M}}\left( {{\mathbb{R}}^{p}} \right)$.

Assuming Lang's conjectured lower bound on the heights of non-torsion points on an elliptic curve, we show that there exists an absolute constant $C$ such that for any elliptic curve $E/\mathbb{Q}$ and non-torsion point $P\,\in \,E\left( \mathbb{Q} \right)$, there is at most one integral multiple $\left[ n \right]P$ such that $n\,>\,C$. The proof is a modification of a proof of Ingram giving an unconditional, but not uniform, bound. The new ingredient is a collection of explicit formulæ for the sequence $v\left( {{\Psi }_{n}} \right)$ of valuations of the division polynomials. For $P$ of non-singular reduction, such sequences are already well described in most cases, but for $P$ of singular reduction, we are led to define a new class of sequences called elliptic troublemaker sequences, which measure the failure of the Néron local height to be quadratic. As a corollary in the spirit of a conjecture of Lang and Hall, we obtain a uniform upper bound on $\widehat{h}\left( P \right)/h\left( E \right)$ for integer points having two large integral multiples.

Let $C$ denote the Fermat curve over $\mathbb{Q}$ of prime exponent $l$ . The Jacobian $\text{Jac(}C\text{)}$ of $C$ splits over $\mathbb{Q}$ as the product of Jacobians $\text{Jac(}{{C}_{k}})$ , $1\,\le \,k\,\le \,\ell \,-\text{2}$ , where ${{C}_{k}}$ are curves obtained as quotients of $C$ by certain subgroups of automorphisms of $C$ . It is well known that $\text{Jac(}{{C}_{k}}\text{)}$ is the power of an absolutely simple abelian variety ${{B}_{k}}$ with complex multiplication. We call degenerate those pairs $(l,\,k)$ for which ${{B}_{k}}$ has degenerate $\text{CM}$ type. For a non-degenerate pair $(l,\,k)$ , we compute the Sato–Tate group of $\text{Jac(}{{C}_{k}}\text{)}$ , prove the generalized Sato–Tate Conjecture for it, and give an explicit method to compute the moments and measures of the involved distributions. Regardless of whether $(l,\,k)$ is degenerate, we also obtain Frobenius equidistribution results for primes of certain residue degrees in the $l$ -th cyclotomic field. Key to our results is a detailed study of the rank of certain generalized Demjanenko matrices.

Let $J$ be a Jacobian variety with toric reduction over a local field $K$. Let $J\,\to \,E$ be an optimal quotient defined over $K$, where $E$ is an elliptic curve. We give examples in which the functorially induced map ${{\Phi }_{J}}\,\to \,{{\Phi }_{E}}$ on component groups of the Néron models is not surjective. This answers a question of Ribet and Takahashi. We also give various criteria under which ${{\Phi }_{J}}\,\to \,{{\Phi }_{E}}$ is surjective and discuss when these criteria hold for the Jacobians of modular curves.

The morphism $f\,:\,{{\mathbb{P}}^{N}}\,\to \,{{\mathbb{P}}^{N}}$ is called post-critically finite $\left( \text{PCF} \right)$ if the forward image of the critical locus, under iteration of $f$ , has algebraic support. In the case $N\,=\,1$ , a result of Thurston implies that there are no algebraic families of PCF morphisms, other than a well-understood exceptional class known as the flexible Lattés maps. A related arithmetic result states that the set of PCF morphisms corresponds to a set of bounded height in the moduli space of univariate rational functions. We prove corresponding results for a certain subclass of the regular polynomial endomorphisms of ${{\mathbb{P}}^{N}}$ for any $N$ .

In this paper, we prove that for $\ell \,=\,1$ or 2 the rate of best $\ell $ - monotone polynomial approximation in the ${{L}_{p}}$ norm $\left( 1\,\le \,p\,\le \,\infty \right)$ weighted by the Jacobi weight ${{w}_{\alpha ,\,\beta }}\left( x \right)\,:=\,{{\left( 1\,+\,x \right)}^{\alpha }}{{\left( 1\,-\,x \right)}^{\beta }}$ with $\alpha ,\,\beta \,>\,-1/p$ if $p\,<\,\infty $ , or $\alpha ,\,\beta \,\ge \,0$ if $p\,=\,\infty $ , is bounded by an appropriate $\left( \ell \,+\,1 \right)$ -st modulus of smoothness with the same weight, and that this rate cannot be bounded by the $\left( \ell \,+\,2 \right)$ -nd modulus. Related results on constrained weighted spline approximation and applications of our estimates are also given.

For a fixed $K\,\gg \,1$ and $n\,\in \,\mathbb{N}$, $n\,\gg \,1$ we study metric spaces which admit embeddings with distortion $\le \,K$ into each $n$-dimensional Banach space. Classical examples include spaces embeddable into log $n$-dimensional Euclidean spaces, and equilateral spaces.

We prove that good embeddability properties are preserved under the operation of metric composition of metric spaces. In particular, we prove that $n$-point ultrametrics can be embedded with uniformly bounded distortions into arbitrary Banach spaces of dimension $\log \,n$.

The main result of the paper is a new example of a family of finite metric spaces which are not metric compositions of classical examples and which do embed with uniformly bounded distortion into any Banach space of dimension $n$. This partially answers a question of G. Schechtman.

We study a natural discrete Bochner-type inequality on graphs, and explore its merit as a notion of “curvature” in discrete spaces. An appealing feature of this discrete version of the so-called ${{\Gamma }_{2}}$-calculus (of Bakry-Émery) seems to be that it is fairly straightforward to compute this notion of curvature parameter for several specific graphs of interest, particularly, abelian groups, slices of the hypercube, and the symmetric group under various sets of generators. We further develop this notion by deriving Buser-type inequalities (à la Ledoux), relating functional and isoperimetric constants associated with a graph. Our derivations provide a tight bound on the Cheeger constant (i.e., the edge-isoperimetric constant) in terms of the spectral gap, for graphs with nonnegative curvature, particularly, the class of abelian Cayley graphs, a result of independent interest.

We develop a derivative version of the relative trace formula on $\text{PGL}\left( 2 \right)$ studied in our previous work, and derive an asymptotic formula of an average of central values (derivatives) of automorphic $L$-functions for Hilbert cusp forms. As an application, we prove the existence of Hilbert cusp forms with non-vanishing central values (derivatives) such that the absolute degrees of their Hecke fields are arbitrarily large.

The representation theory of semisimple algebraic groups over the complex numbers (equivalently, semisimple complex Lie algebras or Lie groups, or real compact Lie groups) and the questions of whether a given complex representation is symplectic or orthogonal have been solved since at least the 1950s. Similar results for Weyl modules of split reductive groups over fields of characteristic different from 2 hold by using similar proofs. This paper considers analogues of these results for simple, induced, and tilting modules of split reductive groups over fields of prime characteristic as well as a complete answer for Weyl modules over fields of characteristic 2.

In this work type II Hermite-Padé approximants for a vector of Cauchy transforms of smooth Jacobi-type densities are considered. It is assumed that densities are supported on mutually disjoint intervals (an Angelesco system with complex weights). The formulae of strong asymptotics are derived for any ray sequence of multi-indices.