Hostname: page-component-745bb68f8f-v2bm5 Total loading time: 0 Render date: 2025-02-11T10:21:59.088Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal Quotients of Jacobians With ToricReduction and Component Groups

Published online by Cambridge University Press:  20 November 2018

Mihran Papikian  and
Joseph Rabinoff
Mihran Papikian
Affiliation:
Department of Mathematics, Pennsylvania State University, University Park, PA 16802, USA e-mail: papikian@psu.edu
Joseph Rabinoff
Affiliation:
School of Mathematics, Georgia Institute of Technology, 686 Cherry Street, Atlanta, GA 30332 e-mail: rabinoff@post.harvard.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $J$ be a Jacobian variety with toric reduction over a local field $K$. Let $J\,\to \,E$ be an optimal quotient defined over $K$, where $E$ is an elliptic curve. We give examples in which the functorially induced map 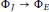${{\Phi }_{J}}\,\to \,{{\Phi }_{E}}$ on component groups of the Néron models is not surjective. This answers a question of Ribet and Takahashi. We also give various criteria under which 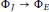${{\Phi }_{J}}\,\to \,{{\Phi }_{E}}$ is surjective and discuss when these criteria hold for the Jacobians of modular curves.

Keywords

11G1814G2214G20Jacobians with toric reductioncomponent groupsmodular curves
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 68 , Issue 6 , 01 December 2016 , pp. 1362 - 1381
Copyright
Copyright © Canadian Mathematical Society 2016

References

[1] Agashe, A., Ribet, K., and Stein, W., The modular degree, congruence primes, and multiplicity one. In: Number theory, analysis and geometry, Springer, New York, 2012, pp. 19–49.Google Scholar
[2] Bertolini, M. and Darmon, H., p-adic periods, p-adic L-functions, and the p-adic uniformization of Shimura curves. Duke Math. J. 98(1999), no. 2, 305–334. http://dx.doi.org/10.1215/S0012-7094-99-09809-5 Google Scholar
[3] Bosch, S. and Liitkebohmert, W., Degenerating abelian varieties. Topology 30(1991), 653–698. http://dx.doi.Org/10.1016/0040-9383(91)90045-6 Google Scholar
[4] Bosch, S., Liitkebohmert, W., and Raynaud, M., Néron models. Ergebnisse der Mathematik und ihrer Grenzgebiete (3), 21, Springer-Verlag, Berlin, 1990.Google Scholar
[5] J.-RBoutot, and Carayol, H., Uniformisation p-adique des courbes de Shimura: les théorèmes de Cerednik et de Drinfeld. Astérisque (1991), no. 196–197, 45-158 (1992), Courbes modulaires et courbes de Shimura (Orsay, 1987/1988).Google Scholar
[6] Coleman, R., The monodromy pairing. Asian J. Math. 4(2000), 315–330. http://dx.doi.Org/10.4310/AJM.2000.v4.n2.a2 Google Scholar
[7] Conrad, B. and Stein, W., Component groups of purely toric quotients. Math. Res. Let. 8(2001), 745–766.http://dx.doi.org/10.4310/MRL.2001.v8.n6.a5 Google Scholar
[8] Emerton, M., Optimal quotients of modular Jacobians. Math. Ann. 327(2003), no. 3, 429–458.http://dx.doi.Org/10.1007/s00208-003-0449-2 Google Scholar
[9] Fresnel, J. and van der Put, M., Rigid analytic geometry and its applications, Progress in Mathematics, 218, Birkhâuser Boston, Boston, MA, 2004.Google Scholar
[10] Gekeler, E.-U., Analytical construction of Weil curves over function fields. J. Théor. Nombres Bordeaux 7(1995), 27–49. http://dx.doi.org/10.5802/jtnb.129 Google Scholar
[11] Gekeler, E.-U. and Reversât, M., Jacobians of Drinfeld modular curves. J. Reine Angew. Math. 476(1996), 27–93.Google Scholar
[12] Gerritzen, L., Ûber Endomorphismen nichtarchimedischer holomorpher Tori. Invent. Math. 11(1970), 27–36.http://dx.doi.Org/10.1007/BF01389803 Google Scholar
[13] Gross, B., Heights and the special values of L-series. In: Number theory. CMS Conf. Proc. 7, Amer. Math. Soc, Providence, RI, 1987, pp. 115–187.Google Scholar
[14] Grothendieck, A., Modèles de Néron et monodromie, SGA 7, Exposé IX, 1972.Google Scholar
[15] Howe, E., Leprévost, F., and Poonen, B., Large torsion subgroups of split Jacobians of curves of genus two or three. Forum Math. 12(2000), no. 3, 315–364.Google Scholar
[16] Kani, E., The number of curves of genus two with elliptic differentials. J. Reine Angew. Math. 485(1997), 93–121.Google Scholar
[17] Katz, N. and Mazur, B., Arithmetic moduli of elliptic curves. Annals of Mathematics Studies 108, Princeton University Press, 1985.Google Scholar
[18] Mazur, B., Modular curves and the Eisenstein ideal. Inst. Hautes Études Sci. Publ. Math. 47(1977), 33–186.Google Scholar
[19] Mestre, J.-F. and Oesterlé, J., Courbes de Weil semi-stables de discriminant une puissance m-ième. J. Reine Angew. Math. 400(1989), 173–184.Google Scholar
[20] Papikian, M., On Jacquet-Langlands isogeny over function fields. J. Number Theory 131(2011), no. 7, 1149–1175. http://dx.doi.Org/10.1016/j.jnt.2O11.01.002 Google Scholar
[21] Ribet, K., Endomorphisms of semi-stable abelian varieties over number fields. Ann. Math. (2) 101(1975), 555–562.http://dx.doi.org/!0.2307/1970941 Google Scholar
[22] Ribet, K., Mod p Hecke operators and congruences between modular forms. Invent. Math. 71(1983), no. 1, 193–205. http://dx.doi.org/10.1007/BF01393341 Google Scholar
[23] Ribet, K., Letter to J.-F. Mestre. 1987. arxiv:math.AC/0105124 Google Scholar
[24] Ribet, K., On the modular representations of Gal(ℚ/ℚ) arising from modular forms. Invent. Math. 100(1990), 431–476. http://dx.doi.org/10.1007/BF01231195 Google Scholar
[25] Ribet, K., Torsion points on Jo(N) and Galois representations. In: Arithmetic theory of elliptic curves. Lecture Notes in Math. 1716, Springer, Berlin, 1999, pp. 145–166.Google Scholar
[26] Serre, J.-P., Rational points on curves over finite fields, Lectures given at Harvard University, Notes by F.Gouvêa, 1985. Google Scholar
[27] Takahashi, S., Degrees of parametrizations of elliptic curves by Shimura curves. J. Number Theory 90(2001), no. 1, 74–88. http://dx.doi.org/10.1006/jnth.2000.2614 Google Scholar
[28] Takahashi, S., p-adic periods of modular elliptic curves and the level-lowering theorem. Int. J. Number Theory 4 (2008), no. 1, 15–23. http://dx.doi.Org/10.1142/S1793042108001183 Google Scholar
[29] Takahashi, S., Maps on groups of connected components induced from parametrizations of elliptic curves by Shimura curves. JP J. Algebra Number Theory Appl. 13(2009), no. 1, 57–63.Google Scholar
[30] Teitelbaum, J., p-adic periods of genus two Mumford-Schottky curves, J. Reine Angew. Math. 385(1988), 117–151.Google Scholar
[31] Zagier, D., Modular parametrizations of elliptic curves. Canad. Math. Bull. 28(1985), no. 3, 372–384. http://dx.doi.org/10.4153/CMB-1985-044-8 Google Scholar