We prove a large deviation principle for the slow-fast rough differential equations (RDEs) under the controlled rough path (RP) framework. The driver RPs are lifted from the mixed fractional Brownian motion (FBM) with Hurst parameter $H\in (1/3,1/2)$. Our approach is based on the continuity of the solution mapping and the variational framework for mixed FBM. By utilizing the variational representation, our problem is transformed into a qualitative property of the controlled system. In particular, the fast RDE coincides with Itô stochastic differential equation (SDE) almost surely, which possesses a unique invariant probability measure with frozen slow component. We then demonstrate the weak convergence of the controlled slow component by averaging with respect to the invariant measure of the fast equation and exploiting the continuity of the solution mapping.

Recently it has been shown that the unique local perimeter minimizing partitioning of the plane into three regions, where one region has finite area and the other two have infinite measure, is given by the so-called standard lens partition. Here we prove a sharp stability inequality for the standard lens, hence strengthening the local minimality of the lens partition in a quantitative form. As an application of this stability result we consider a nonlocal perturbation of an isoperimetric problem.

The notion of effective topological complexity, introduced by Błaszczyk and Kaluba, deals with using group actions in the configuration space in order to reduce the complexity of the motion planning algorithm. In this article, we focus on studying several properties of this notion of topological complexity. We introduce a notion of effective LS category which mimics the behaviour the usual LS category has in the non-effective setting. We use it to investigate the relationship between these effective invariants and the orbit map with respect to the group action, and we give numerous examples. Additionally, we investigate non-vanishing criteria based on a cohomological dimension bound of the saturated diagonal.

We establish a weak local boundedness to Lane–Emden systems in two-dimensional domains involving general second-order elliptic operators in divergence form and arbitrary positive powers whose product equals 1. Our result is complete in the sense that it reduces to that of Trudinger for single equations. As a counterpart, we derive a new Harnack estimate for such systems and, as a by-product, for biharmonic equations.

We investigate the existence of 4-torsion in the integral cohomology of oriented Grassmannians. We establish bounds on the characteristic rank of oriented Grassmannians and prove some cases of our previous conjecture on the characteristic rank. We also discuss the relation between the characteristic rank and a result of Stong on the height of w1 in the cohomology of Grassmannians. The existence of 4-torsion classes follows from the results on the characteristic rank via Steenrod square considerations. We thus exhibit infinitely many examples of 4-torsion classes for oriented Grassmannians. We also prove bounds on torsion exponents of oriented flag manifolds. The article also discusses consequences of our results for a more general perspective on the relation between the torsion exponent and deficiency for homogeneous spaces.

We prove a synthetic Bonnet–Myers rigidity theorem for globally hyperbolic Lorentzian length spaces with global curvature bounded below by K < 0 and an open distance realizer of length $L=\frac{\pi}{\sqrt{|K|}}$: It states that the space necessarily is a warped product with warping function $\cos: (-\frac{\pi}{2},\frac{\pi}{2})\to\mathbb{R}_+$. From this, one also sees that a globally hyperbolic spacetime with curvature bounded above by K < 0 and an open distance realizer of length $L=\frac{\pi}{\sqrt{|K|}}$ is a warped product with warping function cos.

Let X be a complex Banach space and B be a closed linear operator with domain $\mathcal{D}(B) \subset X,\,\, a,b,c,d\in\mathbb{R},$ and $0 \lt \beta \lt \alpha.$ We prove that the problem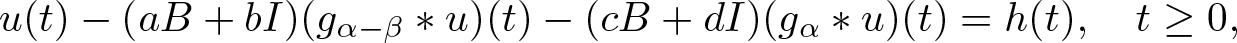

\begin{equation*}u(t) -(aB+bI)(g_{\alpha-\beta}\ast u)(t) -(cB+dI)(g_{\alpha}\ast u)(t) = h(t), \quad t\geq 0,\end{equation*}

where $g_{\alpha}(t)=t^{\alpha-1}/\Gamma(\alpha)$ and $h:\mathbb{R}_+\to X$ is given, has a unique solution for any initial condition on $\mathcal{D}(B)\times X$ as long as the operator B generates an ad-hoc Laplace transformable and strongly continuous solution family $\{R_{\alpha,\beta}(t)\}_{t\geq 0} \subset \mathcal{L}(X).$ It is shown that such a solution family exists whenever the pair 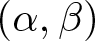$(\alpha,\beta)$ belongs to a subset of the set $(1,2]\times(0,1]$ and B is the generator of a cosine family or a C0-semigroup in $X.$ In any case, it also depends on certain compatibility conditions on the real parameters $a,b,c,d$ that must be satisfied.

The topological structure of ‘mean dichotomy spectrum’ is shown in this article, as an extension of Sacker–Sell spectrum and non-uniform dichotomy spectrum. With regard to mean hyperbolic systems, the coexistence of expansion and contraction behaviours can lead to non-hyperbolic phenomena during evolution process. To be precise, distinct from uniform and non-uniform hyperbolic cases, error hyperbolic degree $\varepsilon(t,\tau)$ is vital to depict the spectral manifolds. As application, the reducibility theorem for mean hyperbolic systems is provided to deduce block diagonalization.

We characterize the functions with ‘small’ bounded mean oscillation (BMO) norm by establishing the precise connection between the space BMO and class $A_\infty$ of Muckenhoupt weights. We prove that there exists a universal constant $c^*_2$ such that $\Vert f \Vert_{BMO} \lt c^*_2$ if and only if $\exp f \in A_2$, where $c^*_2$ is the sharp constant in the John and Nirenberg inequality. Similarly, in dimension one, we prove that $\Vert f \Vert_{BLO} \lt 1$ if and only if $\exp f \in A_1$. As application we introduce a structure of metric space in $A_\infty$ and prove that the closed unit ball of $A_\infty$ is a Banach space.

In this article, we study the two membranes problem for operators given in terms of a mean value formula on a regular tree. We show existence of solutions under adequate conditions on the boundary data and the involved source terms. We also show that, when the boundary data are strictly separated, the coincidence set is separated from the boundary and thus it contains only a finite number of nodes.

Works by O’Grady allow to associate with a two-dimensional Gushel–Mukai (GM) variety, which is a K3 surface, a double Eisenbud–Popescu–Walter (EPW) sextic. We characterize the $K3$ surfaces whose associated double EPW sextic is smooth. As a consequence, we are able to produce symplectic actions on some families of smooth double EPW sextics which are hyper-Kähler manifolds.

We also provide bounds for the automorphism group of GM varieties in dimension 2 and higher.

We introduce a relaxed version of the metric definition of quasiconformality that is natural also for mappings of low regularity, including $W_{\mathrm{loc}}^{1,1}({\mathbb R}^n;{\mathbb R}^n)$-mappings. Then we show on the plane that this relaxed definition can be used to prove Sobolev regularity, and that these ‘finely quasiconformal’ mappings are in fact quasiconformal.

Given a group G acting faithfully on a set S, we characterize precisely when the twisted Brin–Thompson group SVG is finitely presented. The answer is that SVG is finitely presented if and only if we have the following: G is finitely presented, the action of G on S has finitely many orbits of two-element subsets of S, and the stabilizer in G of any element of S is finitely generated. Since twisted Brin–Thompson groups are simple, a consequence is that any subgroup of a group admitting an action as above satisfies the Boone–Higman conjecture. In the course of proving this, we also establish a sufficient condition for a group acting cocompactly on a simply connected complex to be finitely presented, even if certain edge stabilizers are not finitely generated, which may be of independent interest.

The present article is concerned with the Lyapunov stability of stationary solutions to the Allen–Cahn equation with a strong irreversibility constraint, which was first intensively studied in [2] and can be reduced to an evolutionary variational inequality of obstacle type. As a feature of the obstacle problem, the set of stationary solutions always includes accumulation points, and hence, it is rather delicate to determine the stability of such non-isolated equilibria. Furthermore, the strongly irreversible Allen–Cahn equation can also be regarded as a (generalized) gradient flow; however, standard techniques for gradient flows such as linearization and Łojasiewicz–Simon gradient inequalities are not available for determining the stability of stationary solutions to the strongly irreversible Allen–Cahn equation due to the non-smooth nature of the obstacle problem.

Nilpotency concepts for skew braces are among the main tools with which we are nowadays classifying certain special solutions of the Yang–Baxter equation, a consistency equation that plays a relevant role in quantum statistical mechanics and in many areas of mathematics. In this context, two relevant questions have been raised in F. Cedó, A. Smoktunowicz and L. Vendramin (Skew left braces of nilpotent type. Proc. Lond. Math. Soc. (3) 118 (2019), 1367–1392) (see questions 2.34 and 2.35) concerning right- and central nilpotency. The aim of this short note is to give a negative answer to both questions: thus, we show that a finite strong-nil brace B need not be right-nilpotent. On a positive note, we show that there is one (and only one, by our examples) special case of the previous questions that actually holds. In fact, we show that if B is a skew brace of nilpotent type and $b\ \ast \ b=0$ for all $b\in B$, then B is centrally nilpotent.

We denote by $\mathcal{H}_{d,g,r}$ the Hilbert scheme of smooth curves, which is the union of components whose general point corresponds to a smooth, irreducible, and non-degenerate curve of degree d and genus g in $\mathbb{P}^r.$ In this article, we study $\mathcal{H}_{15,g,5}$ for every possible genus g and determine when it is irreducible. We also study the moduli map $\mathcal{H}_{15,g,5}\rightarrow\mathcal{M}_g$ and several key properties such as gonality of a general element as well as characterizing smooth elements of each component.

We introduce two new notions called the Daugavet constant and Δ-constant of a point, which measure quantitatively how far the point is from being Daugavet point and Δ-point and allow us to study Daugavet and Δ-points in Banach spaces from a quantitative viewpoint. We show that these notions can be viewed as a localized version of certain global estimations of Daugavet and diametral local diameter two properties such as Daugavet indices of thickness. As an intriguing example, we present the existence of a Banach space X in which all points on the unit sphere have positive Daugavet constants despite the Daugavet indices of thickness of X being zero. Moreover, using the Daugavet and Δ-constants of points in the unit sphere, we describe the existence of almost Daugavet and Δ-points, as well as the set of denting points of the unit ball. We also present exact values of the Daugavet and Δ-constant on several classical Banach spaces, as well as Lipschitz-free spaces. In particular, it is shown that there is a Lipschitz-free space with a Δ-point, which is the furthest away from being a Daugavet point. Finally, we provide some related stability results concerning the Daugavet and Δ-constant.

This paper is concerned with a singular limit of the Kobayashi–Warren–Carter system, a phase field system modelling the evolutions of structures of grains. Under a suitable scaling, the limit system is formally derived when the interface thickness parameter tends to zero. Different from many other problems, it turns out that the limit system is a system involving fractional time derivatives, although the original system is a simple gradient flow. A rigorous derivation is given when the problem is reduced to a gradient flow of a single-well Modica–Mortola functional in a one-dimensional setting.

In this article, we calculate the Birkhoff spectrum in terms of the Hausdorff dimension of level sets for Birkhoff averages of continuous potentials for a certain family of diagonally affine iterated function systems. Also, we study Besicovitch–Eggleston sets for finite generalized Lüroth series number systems with redundancy. The redundancy refers to the fact that each number $x \in [0,1]$ has uncountably many expansions in the system. We determine the Hausdorff dimension of digit frequency sets for such expansions along fibres.

In this article, we study the following Schrödinger equation

\begin{align*}\begin{cases}-\Delta u -\frac{\mu}{|x|^2} u+\lambda u =f(u), &\text{in}~ \mathbb{R}^N\backslash\{0\},\\\int_{\mathbb{R}^{N}}|u|^{2}\mathrm{d} x=a, & u\in H^1(\mathbb{R}^{N}),\end{cases}\end{align*}

where $N\geq 3$, a > 0, and $\mu \lt \frac{(N-2)^2}{4}$. Here $\frac{1}{|x|^2} $ represents the Hardy potential (or ‘inverse-square potential’), λ is a Lagrange multiplier, and the nonlinearity function f satisfies the general Sobolev critical growth condition. Our main goal is to demonstrate the existence of normalized ground state solutions for this equation when $0 \lt \mu \lt \frac{(N-2)^2}{4}$. We also analyse the behaviour of solutions as $\mu\to0^+$ and derive the existence of normalized ground state solutions for the limiting case where µ = 0. Finally, we investigate the existence of normalized solutions when µ < 0 and analyse the asymptotic behaviour of solutions as $\mu\to 0^-$.