We consider the relationship between the Mahler measure $M(f)$ of a polynomial f and its separation $\operatorname {sep}(f)$. Mahler [‘An inequality for the discriminant of a polynomial’, Michigan Math. J. 11 (1964), 257–262] proved that if $f(x) \in \mathbb {Z}[x]$ is separable of degree n, then $\operatorname {sep}(f) \gg _n M(f)^{-(n-1)}$. This spurred further investigations into the implicit constant involved in that relationship and led to questions about the optimal exponent on $M(f)$. However, there has been relatively little study concerning upper bounds on $\operatorname {sep}(f)$ in terms of $M(f)$. We prove that if $f(x) \in \mathbb {C}[x]$ has degree n, then $\operatorname {sep}(f) \ll n^{-1/2}M(f)^{1/(n-1)}$. Moreover, this bound is sharp up to the implied constant factor. We further investigate the constant factor under various additional assumptions on $f(x)$; for example, if it has only real roots.

Our goal of the paper is to investigate the Waring problem for upper triangular matrix algebras, which gives a complete solution of a conjecture proposed by Panja and Prasad in 2023.

We obtain an effective analytic formula, with explicit constants, for the number of distinct irreducible factors of a polynomial $f \in \mathbb {Z}[x]$ . We use an explicit version of Mertens’ theorem for number fields to estimate a related sum over rational primes. For a given $f \in \mathbb {Z}[x]$ , our result yields a finite list of primes that certifies the number of distinct irreducible factors of f.

The classical Gauss–Lucas theorem states that the critical points of a polynomial with complex coefficients are in the convex hull of its zeros. This fundamental theorem follows from the fact that if all the zeros of a polynomial are in a half plane, then the same is true for its critical points. The main result of this work replaces the half plane with a sector as follows.

We show that if the coefficients of a monic polynomial $p(z)$ are in the sector {tei𝜓 : 𝜓∈ [0, 𝜙], t⩾0}, for some $\unicode[STIX]{x1D719}\in [0,\unicode[STIX]{x1D70B})$, and the zeros are not in its interior, then the critical points of $p(z)$ are also not in the interior of that sector.

In addition, we give a necessary condition for a polynomial to satisfy the premise of the main result.

We present the geometry behind counting twin prime polynomials in $\mathbb{F}_{q}[T]$ in general. We compute cohomology and explicitly count points by means of a twisted Lefschetz trace formula applied to these parametrizing varieties for cubic twin prime polynomials. The elliptic curve $X^{3}=Y(Y-1)$ occurs in the geometry, and thus counting cubic twin prime polynomials involves the associated modular form. In theory, this approach can be extended to higher degree twin primes, but the computations become harder.

The formula we get in degree 3 is compatible with the Hardy–Littlewood heuristic on average, agrees with the prediction for $q\equiv 2$ (mod 3), but shows anomalies for $q\equiv 1$ (mod 3).

We prove Lawton's conjecture about the upper bound on themeasure of the set on the unit circle on which a complex polynomial with a bounded number of coefficients takes small values. Namely, we prove that Lawton's bound holds for polynomials that are not necessarily monic. We also provide an analogous bound for polynomials in several variables. Finally, we investigate the dependence of the bound on the multiplicity of zeros for polynomials in one variable.

We extend the characterization of extremal valued fields given in [2] to the missing case of valued fields of mixed characteristic with perfect residue field. This leads to a complete characterization of the tame valued fields that are extremal. The key to the proof is a model theoretic result about tame valued fields in mixed characteristic. Further, we prove that in an extremal valued field of finite p-degree, the images of all additive polynomials have the optimal approximation property. This fact can be used to improve the axiom system that is suggested in [8] for the elementary theory of Laurent series fields over finite fields. Finally we give examples that demonstrate the problems we are facing when we try to characterize the extremal valued fields with imperfect residue fields. To this end, we describe several ways of constructing extremal valued fields; in particular, we show that in every ℵ1 saturated valued field the valuation is a composition of extremal valuations of rank 1.

In this paper, we give a general explicit form of Cassels’ $p$-adic embedding theorem for number fields. We also give its refined form in the case of cyclotomic fields. As a byproduct, given an irreducible polynomial $f$ over $\mathbb{Z}$, we give a general unconditional upper bound for the smallest prime number $p$ such that $f$ has a simple root modulo $p$.

Aggregation functions are widely used in answer set programming for representing and reasoning on knowledge involving sets of objects collectively. Current implementations simplify the structure of programs in order to optimize the overall performance. In particular, aggregates are rewritten into simpler forms known as monotone aggregates. Since the evaluation of normal programs with monotone aggregates is in general on a lower complexity level than the evaluation of normal programs with arbitrary aggregates, any faithful translation function must introduce disjunction in rule heads in some cases. However, no function of this kind is known. The paper closes this gap by introducing a polynomial, faithful, and modular translation for rewriting common aggregation functions into the simpler form accepted by current solvers. A prototype system allows for experimenting with arbitrary recursive aggregates, which are also supported in the recent version 4.5 of the grounder gringo, using the methods presented in this paper.

The Mahler measure of a nonzero $n$-variable polynomial $P$ is the integral of $\log \,\left| P \right|$ on the unit $n$-torus. A result of Boyd and Lawton says that the Mahler measure of a multivariate polynomial is the limit of Mahler measures of univariate polynomials. We prove the analogous result for different extensions of Mahler measure such as generalized Mahler measure (integrating the maximum of $\log \,\left| P \right|$ for possibly different ${{P}^{'}}\text{s}$), multiple Mahler measure (involving products of $\log \,\left| P \right|$ for possibly different ${{P}^{'}}\text{s}$), and higher Mahler measure (involving ${{\log }^{k}}\,\left| P \right|$).

The paper describes entire solutions to the eiconal type non-linear partial differential equations, which include the eiconal equations ${{({{X}_{1}}(u))}^{2}}\,+\,{{({{X}_{2}}(u))}^{2}}\,=\,1$ as special cases, where ${{X}_{1}}\,=\,{{p}_{1}}\partial /\partial {{z}_{1}}\,+\,{{p}_{2}}\partial /\partial {{z}_{2}},\,{{X}_{2}}\,=\,{{p}_{3}}\partial /\partial {{z}_{1}}\,+\,{{p}_{4}}\partial /\partial {{z}_{2}}$ are linearly independent operators with ${{p}_{j}}$ being arbitrary polynomials in ${{\mathbf{C}}^{2}}$.

Let 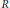$R$ be a dense subring of 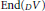$\text{End}\left( _{D}V \right)$, where $V$ is a left vector space over a division ring 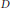$D$. If 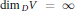$\dim{{\,}_{D}}V\,=\,\infty $, then the range of any nonzero polynomial $f\left( {{X}_{1}},\,\ldots \,,\,{{X}_{m}} \right)$ on 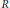$R$ is dense in 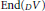$\text{End}\left( _{D}V \right)$. As an application, let 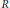$R$ be a prime ring without nonzero nil one-sided ideals and $0\,\ne \,a\,\in \,R$. 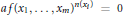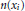If $af{{\left( {{x}_{1}},\ldots ,{{x}_{m}} \right)}^{n\left( {{x}_{i}} \right)}}\,=\,0$ for all ${{x}_{1}},\,\ldots \,,\,{{x}_{m}}\,\in \,R$, where $n\left( {{x}_{i}} \right)$ is a positive integer depending on ${{x}_{1}},\,\ldots \,,\,{{x}_{m}}\,\in \,R$, then $f\left( {{X}_{1}},\,\ldots \,,\,{{X}_{m}} \right)$ is a polynomial identity of 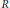$R$ unless 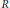$R$ is a finite matrix ring over a finite field.

If is an univariate polynomial with degree n then Bombieri norm of P is defined by

where denotes the binomial coefficient.

In the present paper we give, under assumptions on the roots of P, optimal Bernsteintype inequalities for the ratio between Bombieri norm of P and that of its derivative P′.

We also give such inequalities for the polar derivatives of P defined by

A continuous-time Markov chain on the non-negative integers is called skip-free to the left (right) if the governing infinitesimal generator A = (aij) has the property that aij = 0 for j ≦ i ‒ 2 (i ≦ j – 2). If a Markov chain is skip-free both to the left and to the right, it is called a birth-death process. Quasi-limiting distributions of birth–death processes have been studied in detail in their own right and from the standpoint of finite approximations. In this paper, we generalize, to some extent, results for birth-death processes to Markov chains that are skip-free to the left in continuous time. In particular the decay parameter of skip-free Markov chains is shown to have a similar representation to the birth-death case and a result on convergence of finite quasi-limiting distributions is obtained.

Lower and upper bounds of degree m for the probability of the union of n not necessarily exchangeable events are established. These bounds may be constructed to improve the Bonferroni and the Sobel–Uppuluri bounds.

An application to equi-correlated multivariate normal distribution is given.

R. Paré and W. Schelter (1978) have extended the Cayley-Hamilton theorem by showing that for each n<1 there is an integer k such that all n x n matrices over any (possibly noncommutative) ring satisfy a monic polynomial of degree k. We give a lower bound for this degree, namely π(n), which is defined as the shortest possible length of a sequence with entries from {1, 2, …, n}.