We show that for every 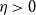$\eta \gt 0$ every sufficiently large $n$-vertex oriented graph $D$ of minimum semidegree exceeding $(1+\eta )\frac k2$ contains every balanced antidirected tree with 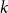$k$ edges and bounded maximum degree, if 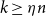$k\ge \eta n$. In particular, this asymptotically confirms a conjecture of the first author for long antidirected paths and dense digraphs.

Further, we show that in the same setting, 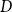$D$ contains every 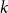$k$-edge antidirected subdivision of a sufficiently small complete graph, if the paths of the subdivision that have length 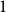$1$ or $2$ span a forest. As a special case, we can find all antidirected cycles of length at most $k$.

Finally, we address a conjecture of Addario-Berry, Havet, Linhares Sales, Reed, and Thomassé for antidirected trees in digraphs. We show that this conjecture is asymptotically true in $n$-vertex oriented graphs for all balanced antidirected trees of bounded maximum degree and of size linear in $n$.

An oriented graph is called singular or nonsingular according as its adjacency matrix is singular or nonsingular. In this note, by a new approach, we determine the singularity of oriented quasi-trees. The main results of Chen et al. [‘Singularity of oriented graphs from several classes’, Bull. Aust. Math. Soc. 102(1) (2020), 7–14] follow as corollaries. Furthermore, we give a necessary condition for an oriented bipartite graph to be nonsingular. By applying this condition, we characterise nonsingular oriented bipartite graphs $B_{m,n}$ when $\min \{m,n\}\leq 3$ .

A digraph is called oriented if there is at most one arc between two distinct vertices. An oriented graph $D$ is nonsingular if its adjacency matrix $A(D)$ is nonsingular. We characterise all nonsingular oriented graphs from three classes: graphs in which cycles are vertex disjoint, graphs in which all cycles share exactly one common vertex and graphs formed by cycles sharing a common path. As a straightforward corollary, the singularity of oriented bicyclic graphs is determined.