As was shown by a part of the authors, for a given 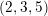 $(2,3,5)$-distribution
$(2,3,5)$-distribution  $D$ on a five-dimensional manifold
$D$ on a five-dimensional manifold  $Y$, there is, locally, a Lagrangian cone structure
$Y$, there is, locally, a Lagrangian cone structure  $C$ on another five-dimensional manifold
$C$ on another five-dimensional manifold 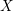 $X$ which consists of abnormal or singular paths of
$X$ which consists of abnormal or singular paths of  $(Y,D)$. We give a characterization of the class of Lagrangian cone structures corresponding to
$(Y,D)$. We give a characterization of the class of Lagrangian cone structures corresponding to 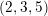 $(2,3,5)$-distributions. Thus, we complete the duality between
$(2,3,5)$-distributions. Thus, we complete the duality between 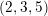 $(2,3,5)$-distributions and Lagrangian cone structures via pseudo-product structures of type
$(2,3,5)$-distributions and Lagrangian cone structures via pseudo-product structures of type  $G_{2}$. A local example of nonflat perturbations of the global model of flat Lagrangian cone structure which corresponds to
$G_{2}$. A local example of nonflat perturbations of the global model of flat Lagrangian cone structure which corresponds to 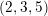 $(2,3,5)$-distributions is given.
$(2,3,5)$-distributions is given.
 $(2,3,5)$-DISTRIBUTIONS TO HIGHER DIMENSIONS
$(2,3,5)$-DISTRIBUTIONS TO HIGHER DIMENSIONS