In the present paper, we characterize the Fredholmness of Toeplitz pairs on Hardy space over the bidisk with the bounded holomorphic symbols, and hence, we obtain the index formula for such Toeplitz pairs. The key to obtain the Fredholmness of such Toeplitz pairs is the $L^p$ solution of Corona Problem over $\mathbb {D}^2$.

A suitable notion of weak amenability for dual Banach algebras, which we call weak Connes amenability, is defined and studied. Among other things, it is proved that the measure algebra M(G) of a locally compact group G is always weakly Connes amenable. It can be a complement to Johnson’s theorem that $L^1(G)$ is always weakly amenable [10].

We show that $\ell ^1(\mathbb {N}_\wedge )$ is $\varphi $-amenable for each multiplicative linear functional $\varphi :\ell ^1(\mathbb {N}_\wedge )\rightarrow \mathbb {C}.$ This is a counterexample to the final corollary of Jaberi and Mahmoodi [‘On $\varphi $-amenability of dual Banach algebras’, Bull. Aust. Math. Soc. 105 (2022), 303–313] and shows that the final theorem in that paper is not valid.

Generalising the concept of injectivity, we study the notion of $\varphi $ -injectivity for dual Banach algebras. It provides a framework for studying $\varphi $ -amenability of enveloping dual Banach algebras.

Motivated by the definition of a semigroup compactication of a locally compact group and a large collection of examples, we introduce the notion of an (operator) homogeneous left dual Banach algebra (HLDBA) over a (completely contractive) Banach algebra $A$. We prove a Gelfand-type representation theorem showing that every HLDBA over A has a concrete realization as an (operator) homogeneous left Arens product algebra: the dual of a subspace of $A^{\ast }$ with a compatible (matrix) norm and a type of left Arens product $\Box$. Examples include all left Arens product algebras over $A$, but also, when $A$ is the group algebra of a locally compact group, the dual of its Fourier algebra. Beginning with any (completely) contractive (operator) $A$-module action $Q$ on a space $X$, we introduce the (operator) Fourier space $({\mathcal{F}}_{Q}(A^{\ast }),\Vert \cdot \Vert _{Q})$ and prove that $({\mathcal{F}}_{Q}(A^{\ast })^{\ast },\Box )$ is the unique (operator) HLDBA over $A$ for which there is a weak$^{\ast }$-continuous completely isometric representation as completely bounded operators on $X^{\ast }$ extending the dual module representation. Applying our theory to several examples of (completely contractive) Banach algebras $A$ and module operations, we provide new characterizations of familiar HLDBAs over A and we recover, and often extend, some (completely) isometric representation theorems concerning these HLDBAs.

It is shown that various definitions of $\unicode[STIX]{x1D711}$-Connes amenability and $\unicode[STIX]{x1D711}$-contractibility are equivalent to older and simpler concepts.

We show that, under special hypotheses, each 3-Jordan homomorphism ${\it\varphi}$ between Banach algebras ${\mathcal{A}}$ and ${\mathcal{B}}$ is a 3-homomorphism.

It is an open question whether every derivation of a Fréchet GB$^{\ast }$-algebra $A[{\it\tau}]$ is continuous. We give an affirmative answer for the case where $A[{\it\tau}]$ is a smooth Fréchet nuclear GB$^{\ast }$-algebra. Motivated by this result, we give examples of smooth Fréchet nuclear GB$^{\ast }$-algebras which are not pro-C$^{\ast }$-algebras.

Let $A$ be a Banach algebra and let 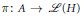$\pi :\,A\,\to \,\mathcal{L}\left( H \right)$ be a continuous representation of $A$ on a separable Hilbert space $H$ with dim $H\,=\,\text{m}$. Let 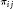${{\pi }_{ij}}$ be the coordinate functions of $\pi $ with respect to an orthonormal basis and suppose that for each 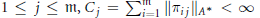$1\,\le \,j\,\le \,\text{m,}\,{{C}_{j}}\,=\,\sum\nolimits_{i=1}^{\text{m}}{\left\| {{\pi }_{ij}} \right\|}{{A}^{*}}\,<\,\infty $ and 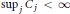${{\sup }_{j}}\,{{C}_{j}}\,<\,\infty $. Under these conditions, we call an element 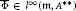$\overline{\Phi }\,\in \,{{\iota }^{\infty }}\,\left( \mathfrak{m},\,{{A}^{**}} \right)$ left $\pi $-invariant if 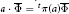$a\,\cdot \overline{\Phi }\,={{\,}^{^{t}\pi }}\left( a \right)\overline{\Phi }$ for all 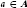$a\in A$ In this paper we prove a link between the existence of left $\pi $-invariant elements and the vanishing of certain Hochschild cohomology groups of $A$. Our results extend an earlier result by Lau on $F$-algebras and recent results of Kaniuth, Lau, Pym, and and the second author in the special case where 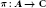$\pi :\,A\,\to \text{C}$ is a non-zero character on $A$.

We define the class of weakly approximately divisible unital ${C}^{\ast } $-algebras and show that this class is closed under direct sums, direct limits, any tensor product with any ${C}^{\ast } $-algebra, and quotients. A nuclear ${C}^{\ast } $-algebra is weakly approximately divisible if and only if it has no finite-dimensional representations. We also show that Pisier’s similarity degree of a weakly approximately divisible ${C}^{\ast } $-algebra is at most five.

We define and study the so-called extreme version of the notion of a projective normed module. The relevant definition takes into account the exact value of the norm of the module in question, in contrast with the standard known definition that is formulated in terms of norm topology.

After the discussion of the case where our normed algebra $A$ is just $\mathbb{C}$, we concentrate on the case of the next degree of complication, where $A$ is a sequence algebra satisfying some natural conditions. The main results give a full characterization of extremely projective objects within the subcategory of the category of non-degenerate normed $A$-modules, consisting of the so-called homogeneous modules. We consider two cases, ‘non-complete’ and ‘complete’, and the respective answers turn out to be essentially different.

In particular, all Banach non-degenerate homogeneous modules consisting of sequences are extremely projective within the category of Banach non-degenerate homogeneous modules. However, neither of them, provided it is infinite-dimensional, is extremely projective within the category of all normed non-degenerate homogeneous modules. On the other hand, submodules of these modules consisting of finite sequences are extremely projective within the latter category.

For a locally compact group G, let LUC(G) denote the space of all left uniformly continuous functions on G. Here, we investigate projectivity, injectivity and flatness of LUC(G) and its dual space LUC(G)* as Banach left modules over the group algebra as well as the measure algebra of G.

Homological properties of several Banach left L1(G)-modules have been studied by Dales and Polyakov and recently by Ramsden. In this paper, we characterize some homological properties of and as Banach left L1(G)-modules, such as flatness, injectivity and projectivity.

We investigate generalized amenability and biflatness properties of various (operator) Segal algebras in both the group algebra, ${{L}^{1}}\left( G \right)$, and the Fourier algebra, $A\left( G \right)$, of a locally compact group $G$.

Let n∈ℕ and let A and B be rings. An additive map h:A→B is called an n-Jordan homomorphism if h(an)=(h(a))n for all a∈A. Every Jordan homomorphism is an n-Jordan homomorphism, for all n≥2, but the converse is false in general. In this paper we investigate the n-Jordan homomorphisms on Banach algebras. Some results related to continuity are given as well.

In this paper, we generalize a result recently obtained by the author. We characterize the cyclic vectors in $L_{a}^{p}\,\left( \mathbb{C},\,\phi \right)$ . Let $f\,\in \,L_{a}^{p}\,\left( \mathbb{C},\,\phi \right)$ and $fC$ be contained in the space. We show that $f$ is non-vanishing if and only if $f$ is cyclic.

The Arens products are the standard way of extending the product from a Banach algebra to its bidual ″. Ultrapowers provide another method which is more symmetric, but one that in general will only give a bilinear map, which may not be associative. We show that if is Arens regular, then there is at least one way to use an ultrapower to recover the Arens product, a result previously known for C*-algebras. Our main tool is a principle of local reflexivity result for modules and algebras.

In this paper, we give a simple criterion for the Arens regularity of a bilinear mapping on normed spaces, which applies in particular to Banach module actions, and then investigate those conditions under which the second adjoint of a derivation into a dual Banach module is again a derivation. As a consequence of the main result, a simple and direct proof for several older results is also included.

Let $G$ be a locally compact group, and let ${{A}_{\text{cb}}}(G)$ denote the closure of $A(G)$, the Fourier algebra of $G$, in the space of completely bounded multipliers of $A(G)$. If $G$ is a weakly amenable, discrete group such that ${{C}^{*}}(G)$ is residually finite-dimensional, we show that ${{A}_{\text{cb}}}(G)$ is operator amenable. In particular, ${{A}_{\text{cb}}}({{\mathbb{F}}_{2}})$ is operator amenable even though ${{\mathbb{F}}_{2}}$, the free group in two generators, is not an amenable group. Moreover, we show that if $G$ is a discrete group such that ${{A}_{\text{cb}}}(G)$ is operator amenable, a closed ideal of $A(G)$ is weakly completely complemented in $A(G)$ if and only if it has an approximate identity bounded in the $\text{cb}$-multiplier norm.

In this paper we give Bessel- and Grüss-type inequalities in an inner product module over a proper $H^*$-algebra or a $C^*$-algebra.