For a prime p and a rational elliptic curve $E_{/\mathbb {Q}}$, set $K=\mathbb {Q}(E[p])$ to denote the torsion field generated by $E[p]:=\operatorname {ker}\{E\xrightarrow {p} E\}$. The class group $\operatorname {Cl}_K$ is a module over $\operatorname {Gal}(K/\mathbb {Q})$. Given a fixed odd prime number p, we study the average nonvanishing of certain Galois stable quotients of the mod-p class group $\operatorname {Cl}_K/p\operatorname {Cl}_K$. Here, E varies over all rational elliptic curves, ordered according to height. Our results are conditional, since we assume that the p-primary part of the Tate–Shafarevich group is finite. Furthermore, we assume predictions made by Delaunay for the statistical variation of the p-primary parts of Tate–Shafarevich groups. We also prove results in the case when the elliptic curve $E_{/\mathbb {Q}}$ is fixed and the prime p is allowed to vary.

A set of complex numbers $S$ is called invariant if it is closed under addition and multiplication, namely, for any $x, y \in S$ we have $x+y \in S$ and $xy \in S$. For each $s \in {\mathbb {C}}$ the smallest invariant set ${\mathbb {N}}[s]$ containing $s$ consists of all possible sums $\sum _{i \in I} a_i s^i$, where $I$ runs over all finite nonempty subsets of the set of positive integers ${\mathbb {N}}$ and $a_i \in {\mathbb {N}}$ for each $i \in I$. In this paper, we prove that for $s \in {\mathbb {C}}$ the set ${\mathbb {N}}[s]$ is everywhere dense in ${\mathbb {C}}$ if and only if $s \notin {\mathbb {R}}$ and $s$ is not a quadratic algebraic integer. More precisely, we show that if $s \in {\mathbb {C}} \setminus {\mathbb {R}}$ is a transcendental number, then there is a positive integer $n$ such that the sumset ${\mathbb {N}} t^n+{\mathbb {N}} t^{2n} +{\mathbb {N}} t^{3n}$ is everywhere dense in ${\mathbb {C}}$ for either $t=s$ or $t=s+s^2$. Similarly, if $s \in {\mathbb {C}} \setminus {\mathbb {R}}$ is an algebraic number of degree $d \ne 2, 4$, then there are positive integers $n, m$ such that the sumset ${\mathbb {N}} t^n+{\mathbb {N}} t^{2n} +{\mathbb {N}} t^{3n}$ is everywhere dense in ${\mathbb {C}}$ for $t=ms+s^2$. For quadratic and some special quartic algebraic numbers $s$ it is shown that a similar sumset of three sets cannot be dense. In each of these two cases the density of ${\mathbb {N}}[s]$ in ${\mathbb {C}}$ is established by a different method: for those special quartic numbers, it is possible to take a sumset of four sets.

This paper goes beyond Katz–Sarnak theory on the distribution of curves over finite fields according to their number of rational points, theoretically, experimentally, and conjecturally. In particular, we give a formula for the limits of the moments measuring the asymmetry of this distribution for (non-hyperelliptic) curves of genus $g\geq 3$. The experiments point to a stronger notion of convergence than the one provided by the Katz–Sarnak framework for all curves of genus $\geq 3$. However, for elliptic curves and for hyperelliptic curves of every genus, we prove that this stronger convergence cannot occur.

For a given genus $g \geq 1$, we give lower bounds for the maximal number of rational points on a smooth projective absolutely irreducible curve of genus g over $\mathbb{F}_q$. As a consequence of Katz–Sarnak theory, we first get for any given $g>0$, any $\varepsilon>0$ and all q large enough, the existence of a curve of genus g over $\mathbb{F}_q$ with at least $1+q+ (2g-\varepsilon) \sqrt{q}$ rational points. Then using sums of powers of traces of Frobenius of hyperelliptic curves, we get a lower bound of the form $1+q+1.71 \sqrt{q}$ valid for $g \geq 3$ and odd $q \geq 11$. Finally, explicit constructions of towers of curves improve this result: We show that the bound $1+q+4 \sqrt{q} -32$ is valid for all $g\ge 2$ and for all q.

Let $d \ge 3$ be an integer and let $P \in \mathbb{Z}[x]$ be a polynomial of degree d whose Galois group is $S_d$. Let $(a_n)$ be a non-degenerate linearly recursive sequence of integers which has P as its characteristic polynomial. We prove, under the generalised Riemann hypothesis, that the lower density of the set of primes which divide at least one non-zero element of the sequence $(a_n)$ is positive.

Using a recent breakthrough of Smith [18], we improve the results of Fouvry and Klüners [4, 5] on the solubility of the negative Pell equation. Let $\mathcal {D}$ denote the set of positive squarefree integers having no prime factors congruent to $3$ modulo $4$ . Stevenhagen [19] conjectured that the density of d in $\mathcal {D}$ such that the negative Pell equation $x^2-dy^2=-1$ is solvable with $x, y \in \mathbb {Z}$ is $58.1\%$ , to the nearest tenth of a percent. By studying the distribution of the $8$ -rank of narrow class groups $\operatorname {\mathrm {Cl}}^+(d)$ of $\mathbb {Q}(\sqrt {d})$ , we prove that the infimum of this density is at least $53.8\%$ .

In this paper, we prove the assertion that the number of monic cubic polynomials $F(x) = x^3 + a_2 x^2 + a_1 x + a_0$ with integer coefficients and irreducible, Galois over ${\mathbb {Q}}$ satisfying $\max \{|a_2|, |a_1|, |a_0|\} \leq X$ is bounded from above by $O(X (\log X)^2)$. We also count the number of abelian monic binary cubic forms with integer coefficients up to a natural equivalence relation ordered by the so-called Bhargava–Shankar height. Finally, we prove an assertion characterizing the splitting field of 2-torsion points of semi-stable abelian elliptic curves.

In this paper we study the family of elliptic curves $E/{{\mathbb {Q}}}$, having good reduction at $2$ and $3$, and whose $j$-invariants are small. Within this set of elliptic curves, we consider the following two subfamilies: first, the set of elliptic curves $E$ such that the quotient $\Delta (E)/C(E)$ of the discriminant divided by the conductor is squarefree; and second, the set of elliptic curves $E$ such that the Szpiro quotient$\beta _E:=\log |\Delta (E)|/\log (C(E))$ is less than $7/4$. Both these families are conjectured to contain a positive proportion of elliptic curves, when ordered by conductor. Our main results determine asymptotics for both these families, when ordered by conductor. Moreover, we prove that the average size of the $2$-Selmer groups of elliptic curves in the first family, again when these curves are ordered by their conductors, is $3$. The key new ingredients necessary for the proofs are ‘uniformity estimates’, namely upper bounds on the number of elliptic curves with bounded height, whose discriminants are divisible by high powers of primes.

We consider the reduction of an elliptic curve defined over the rational numbers modulo primes in a given arithmetic progression and investigate how often the subgroup of rational points of this reduced curve is cyclic.

We propose a framework to prove Malle's conjecture for the compositum of two number fields based on proven results of Malle's conjecture and good uniformity estimates. Using this method, we prove Malle's conjecture for $S_n\times A$ over any number field $k$ for $n=3$ with $A$ an abelian group of order relatively prime to 2, for $n= 4$ with $A$ an abelian group of order relatively prime to 6, and for $n=5$ with $A$ an abelian group of order relatively prime to 30. As a consequence, we prove that Malle's conjecture is true for $C_3\wr C_2$ in its $S_9$ representation, whereas its $S_6$ representation is the first counter-example of Malle's conjecture given by Klüners. We also prove new local uniformity results for ramified $S_5$ quintic extensions over arbitrary number fields by adapting Bhargava's geometric sieve and averaging over fundamental domains of the parametrization space.

The first purpose of our paper is to show how Hooley’s celebrated method leading to his conditional proof of the Artin conjecture on primitive roots can be combined with the Hardy–Littlewood circle method. We do so by studying the number of representations of an odd integer as a sum of three primes, all of which have prescribed primitive roots. The second purpose is to analyse the singular series. In particular, using results of Lenstra, Stevenhagen and Moree, we provide a partial factorisation as an Euler product and prove that this does not extend to a complete factorisation.

Let $p\equiv 1\hspace{0.2em}{\rm mod}\hspace{0.2em}4$ be a prime number. We use a number field variant of Vinogradov’s method to prove density results about the following four arithmetic invariants: (i) $16$-rank of the class group $\text{Cl}(-4p)$ of the imaginary quadratic number field $\mathbb{Q}(\sqrt{-4p})$; (ii) $8$-rank of the ordinary class group $\text{Cl}(8p)$ of the real quadratic field $\mathbb{Q}(\sqrt{8p})$; (iii) the solvability of the negative Pell equation $x^{2}-2py^{2}=-1$ over the integers; (iv) $2$-part of the Tate–Šafarevič group $\unicode[STIX]{x0428}(E_{p})$ of the congruent number elliptic curve $E_{p}:y^{2}=x^{3}-p^{2}x$. Our results are conditional on a standard conjecture about short character sums.

Let $k$ be an imaginary quadratic field with $\operatorname{Cl}_{2}(k)\simeq V_{4}$. It is known that the length of the Hilbert $2$-class field tower is at least $2$. Gerth (On 2-class field towers for quadratic number fields with$2$-class group of type$(2,2)$, Glasgow Math. J. 40(1) (1998), 63–69) calculated the density of $k$ where the length of the tower is $1$; that is, the maximal unramified $2$-extension is a $V_{4}$-extension. In this paper, we shall extend this result for generalized quaternion, dihedral, and semidihedral extensions of small degrees.

We prove an equidistribution theorem for a family of holomorphic Siegel cusp forms for $\mathit{GSp}_{4}/\mathbb{Q}$ in various aspects. A main tool is Arthur’s invariant trace formula. While Shin [Automorphic Plancherel density theorem, Israel J. Math.192(1) (2012), 83–120] and Shin–Templier [Sato–Tate theorem for families and low-lying zeros of automorphic $L$-functions, Invent. Math.203(1) (2016) 1–177] used Euler–Poincaré functions at infinity in the formula, we use a pseudo-coefficient of a holomorphic discrete series to extract holomorphic Siegel cusp forms. Then the non-semisimple contributions arise from the geometric side, and this provides new second main terms $A,B_{1}$ in Theorem 1.1 which have not been studied and a mysterious second term $B_{2}$ also appears in the second main term coming from the semisimple elements. Furthermore our explicit study enables us to treat more general aspects in the weight. We also give several applications including the vertical Sato–Tate theorem, the unboundedness of Hecke fields and low-lying zeros for degree 4 spinor $L$-functions and degree 5 standard $L$-functions of holomorphic Siegel cusp forms.

Let $\psi$ be a generic Drinfeld module of rank $r\,\ge \,2$ . We study the first elementary divisor ${{d}_{1,\,\wp }}\,\left( \psi\right)$ of the reduction of $\psi$ modulo a prime $\wp $ , as $\wp $ varies. In particular, we prove the existence of the density of the primes $\wp $ for which ${{d}_{1,\,\wp }}\,\left( \psi\right)$ is fixed. For $r\,=\,2$ , we also study the second elementary divisor (the exponent) of the reduction of $\psi$ modulo $\wp $ and prove that, on average, it has a large norm. Our work is motivated by J.-P. Serre's study of an elliptic curve analogue of Artin's Primitive Root Conjecture, and, moreover, by refinements to Serre's study developed by the first author and M. R. Murty.

For any finite Galois extension $K$ of $\mathbb{Q}$ and any conjugacy class $C$ in $\text{Gal}\left( {K}/{\mathbb{Q}}\; \right)$, we show that there exist infinitely many Carmichael numbers composed solely of primes for which the associated class of Frobenius automorphisms is $C$. This result implies that for every natural number $n$ there are infinitely many Carmichael numbers of the form ${{a}^{2}}\,+\,n{{b}^{2}}$ with $a,\,b\,\in \,\mathbb{Z}$.

For a number field K and a finite abelian group G, we determine the probabilities of various local completions of a random G-extension of K when extensions are ordered by conductor. In particular, for a fixed prime ℘ of K, we determine the probability that ℘ splits into r primes in a random G-extension of K that is unramified at ℘. We find that these probabilities are nicely behaved and mostly independent. This is in analogy to Chebotarev’s density theorem, which gives the probability that in a fixed extension a random prime of K splits into r primes in the extension. We also give the asymptotics for the number of G-extensions with bounded conductor. In fact, we give a class of extension invariants, including conductor, for which we obtain the same counting and probabilistic results. In contrast, we prove that neither the analogy with the Chebotarev probabilities nor the independence of probabilities holds when extensions are ordered by discriminant.

Let $E$ be an elliptic curve defined over $\mathbb{Q}$ and without complex multiplication. Let $K$ be a fixed imaginary quadratic field. We find nontrivial upper bounds for the number of ordinary primes 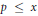$p\,\le \,x$ for which $\mathbb{Q}\left( {{\pi }_{p}} \right)\,=\,K$, where ${{\pi }_{p}}$ denotes the Frobenius endomorphism of $E$ at 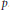$p$. More precisely, under a generalized Riemann hypothesis we show that this number is ${{O}_{E}}\left( {{x}^{17/18}}\,\log x \right)$, and unconditionally we show that this number is ${{O}_{E,K}}\left( \frac{x{{\left( \log \,\log x \right)}^{13/12}}}{{{\left( \log x \right)}^{25/24}}} \right)$ We also prove that the number of imaginary quadratic fields $K$, with − disc $K\,\le \,x$ and of the form $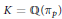K\,=\,\mathbb{Q}({{\pi }_{p}})$, is 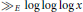${{\gg }_{E}}\,\log \,\log \,\log \,x$ for $x\,\ge \,{{x}_{0}}\left( E \right)$. These results represent progress towards a 1976 Lang–Trotter conjecture.

Let $E$ be an elliptic curve defined over $\mathbb{Q}$, of conductor $N$ and without complex multiplication. For any positive integer $l$, let ${{\phi }_{1}}$ be the Galois representation associated to the $l$-division points of $E$. From a celebrated 1972 result of Serre we know that ${{\phi }_{1}}$ is surjective for any sufficiently large prime $l$. In this paper we find conditional and unconditional upper bounds in terms of $N$ for the primes $l$ for which ${{\phi }_{1}}$ is not surjective.