Hostname: page-component-745bb68f8f-cphqk Total loading time: 0 Render date: 2025-02-06T22:00:29.206Z Has data issue: false hasContentIssue false
  • English
  • Français

Invariant Measure Under the Affine Group Over ${\mathbb{Z}$

Published online by Cambridge University Press:  02 January 2014

DANIELE MUNDICI
DANIELE MUNDICI*
Affiliation:
Department of Mathematics and Computer Science, University of Florence, Viale Morgagni 67/A, 50134 Florence, Italy (e-mail: mundici@math.unifi.it)
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A rational polyhedron$P\subseteq {\mathbb{R^n}}$ is a finite union of simplexes in ${\mathbb{R^n}}$ with rational vertices. P is said to be $\mathbb Z$-homeomorphic to the rational polyhedron 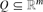$Q\subseteq {\mathbb{R^{\it m}}}$ if there is a piecewise linear homeomorphism η of P onto Q such that each linear piece of η and η−1 has integer coefficients. When n=m, $\mathbb Z$-homeomorphism amounts to continuous $\mathcal{G}_n$-equidissectability, where 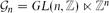$\mathcal{G}_n=GL(n,\mathbb Z) \ltimes \mathbb Z^{n}$ is the affine group over the integers, i.e., the group of all affinities on $\mathbb{R^{n}}$ that leave the lattice $\mathbb Z^{n}$ invariant. $\mathcal{G}_n$ yields a geometry on the set of rational polyhedra. For each d=0,1,2,. . ., we define a rational measure λd on the set of rational polyhedra, and show that any two $\mathbb Z$-homeomorphic rational polyhedra $$P\subseteq {\mathbb{R^n}}$$ and 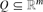$Q\subseteq {\mathbb{R^{\it m}}}$ satisfy 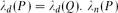$\lambda_d(P)=\lambda_d(Q)$. $\lambda_n(P)$ coincides with the n-dimensional Lebesgue measure of P. If 0 ≤ dim P=d < n then λd(P)>0. For rational d-simplexes T lying in the same d-dimensional affine subspace of 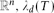${\mathbb{R^{\it n}}, $\lambda_d(T)$$ is proportional to the d-dimensional Hausdorff measure of T. We characterize λd among all unimodular invariant valuations.

Keywords

Primary 20H25Secondary 20H0551M2551N2552B2014E0552A38
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 23 , Issue 2 , March 2014 , pp. 248 - 268
Copyright
Copyright © Cambridge University Press 2014 

References

[1]Barvinok, A. (2002) A Course in Convexity, Vol. 54 of Graduate Studies in Mathematics, AMS.Google Scholar
[2]Betke, U. and Kneser, M. (1985) Zerlegungen und Bewertungen von Gitterpolytopen. J. Reine Angew. Math. 358 202–208.Google Scholar
[3]Birkhoff, G. and Mac Lane, S. (1953) A Survey of Modern Algebra, revised edition, Macmillan.Google Scholar
[4]Boca, F. (2008) An AF algebra associated with the Farey tessellation. Canad. J. Math. 60 975–1000.Google Scholar
[5]Dani, S. G. (1979) On invariant measures, minimal sets and a lemma of Margulis. Inventio. Math. 51 239–260.CrossRefGoogle Scholar
[6]Danilov, V. I. (1983) Birational geometry of toric 3-folds. Math. USSR Izvestiya 21 269–280.Google Scholar
[7]Ehrhart, E. (1962) Sur les polyèdres rationnels homothétiques à n dimensions. CR Acad. Sci. Paris Sér. A 254 616–618.Google Scholar
[8]Ewald, G. (1996) Combinatorial Convexity and Algebraic Geometry, Springer.Google Scholar
[9]Federer, H. (1969) Geometric Measure Theory, Springer.Google Scholar
[10]Fremlin, D. H. (2011) Measure Theory, Vol. 1, second edition. First published in 2000 by Torres Fremlin, 25 Ireton Road, Colchester CO3 3AT, UK. Source files available from: http://www.essex.ac.uk/maths/people/fremlin/mt1.2011/index.htmGoogle Scholar
[11]Glass, A. M. W. and Madden, J. J. (1984) The word problem versus the isomorphism problem. J. London Math. Soc. (2) 30 53–61.CrossRefGoogle Scholar
[12]Gruber, P. M. (2007) Convex and Discrete Geometry, Vol. 336 of Grundlehren der Mathematischen Wissenschaften, Springer.Google Scholar
[13]Lekkerkerker, C. G. (1969) Geometry of Numbers, Wolters-Noordhoff.Google Scholar
[14]McMullen, P. (1993) Valuations and dissections. In Handbook of Convex Geometry, Vol. 2 (Gruber, P. M. and Wills, J. M., eds), Elsevier, pp. 933–988.Google Scholar
[15]Morelli, R. (1996) The birational geometry of toric varieties. J. Algebraic Geometry 5 751–782.Google Scholar
[16]Mundici, D. (1988) Farey stellar subdivisions, ultrasimplicial groups, and K 0 of AF C*-algebras. Adv. Math. 68 23–39.Google Scholar
[17]Mundici, D. (2008) The Haar theorem for lattice-ordered abelian groups with order-unit. Discrete Continuous Dyn. Syst. 21 537–549.CrossRefGoogle Scholar
[18]Mundici, D. (2011) Finite axiomatizability in Ł ukasiewicz logic. Ann. Pure Applied Logic 162 1035–1047.CrossRefGoogle Scholar
[19]Mundici, D. (2011) Revisiting the Farey AF algebra. Milan J. Math. 79 643–656.CrossRefGoogle Scholar
[20]Nogueira, A. (2002) Relatively prime numbers and invariant measures under the natural action of $SL(n,\mathbb Z)$ on ${\mathbb{R^n}}$. Ergodic Theory Dyn. Syst. 22 899–923.Google Scholar
[21]Oda, T. (1988) Convex Bodies and Algebraic Geometry: An Introduction to the Theory of Toric Varieties, Springer.Google Scholar
[22]Panti, G. (2009) Invariant measures in free MV-algebras. Commun. Algebra 36 2849–2861.Google Scholar
[23]Panti, G. (2012) Denominator-preserving maps. Aequationes Math. 84 13–25.Google Scholar
[24]Semadeni, Z. (1982) Schauder Bases in Banach Spaces of Continuous Functions, Vol. 918 of Lecture Notes in Mathematics, Springer.CrossRefGoogle Scholar
[25]Shtan'ko, M. A. (2004) Markov's theorem and algorithmically non-recognizable combinatorial manifolds. Izvestiya RAN, Ser. Math. 68 207–224.Google Scholar
[26]Stallings, J. R. (1968) Lectures on Polyhedral Topology, Vol. 43 of Lectures in Mathematics, Tata Institute of Fundamental Research.Google Scholar
[27]Włodarczyk, J. (1997) Decompositions of birational toric maps in blow-ups and blow-downs. Trans. Amer. Math. Soc. 349 373–411.CrossRefGoogle Scholar