We consider a gradual-impulse control problem of continuous-time Markov decision processes, where the system performance is measured by the expectation of the exponential utility of the total cost. We show, under natural conditions on the system primitives, the existence of a deterministic stationary optimal policy out of a more general class of policies that allow multiple simultaneous impulses, randomized selection of impulses with random effects, and accumulation of jumps. After characterizing the value function using the optimality equation, we reduce the gradual-impulse control problem to an equivalent simple discrete-time Markov decision process, whose action space is the union of the sets of gradual and impulsive actions.

We develop a continuous-time Markov chain (CTMC) approximation of one-dimensional diffusions with sticky boundary or interior points. Approximate solutions to the action of the Feynman–Kac operator associated with a sticky diffusion and first passage probabilities are obtained using matrix exponentials. We show how to compute matrix exponentials efficiently and prove that a carefully designed scheme achieves second-order convergence. We also propose a scheme based on CTMC approximation for the simulation of sticky diffusions, for which the Euler scheme may completely fail. The efficiency of our method and its advantages over alternative approaches are illustrated in the context of bond pricing in a sticky short-rate model for a low-interest environment and option pricing under a geometric Brownian motion price model with a sticky interior point.

We derive the large-sample distribution of the number of species in a version of Kingman’s Poisson–Dirichlet model constructed from an $\alpha$ -stable subordinator but with an underlying negative binomial process instead of a Poisson process. Thus it depends on parameters $\alpha\in (0,1)$ from the subordinator and $r>0$ from the negative binomial process. The large-sample distribution of the number of species is derived as sample size $n\to\infty$ . An important component in the derivation is the introduction of a two-parameter version of the Dickman distribution, generalising the existing one-parameter version. Our analysis adds to the range of Poisson–Dirichlet-related distributions available for modeling purposes.

We investigate the impact of Knightian uncertainty on the optimal timing policy of an ambiguity-averse decision-maker in the case where the underlying factor dynamics follow a multidimensional Brownian motion and the exercise payoff depends on either a linear combination of the factors or the radial part of the driving factor dynamics. We present a general characterization of the value of the optimal timing policy and the worst-case measure in terms of a family of explicitly identified excessive functions generating an appropriate class of supermartingales. In line with previous findings based on linear diffusions, we find that ambiguity accelerates timing in comparison with the unambiguous setting. Somewhat surprisingly, we find that ambiguity may lead to stationarity in models which typically do not possess stationary behavior. In this way, our results indicate that ambiguity may act as a stabilizing mechanism.

Rough volatility is a well-established statistical stylized fact of financial assets. This property has led to the design and analysis of various new rough stochastic volatility models. However, most of these developments have been carried out in the mono-asset case. In this work, we show that some specific multivariate rough volatility models arise naturally from microstructural properties of the joint dynamics of asset prices. To do so, we use Hawkes processes to build microscopic models that accurately reproduce high-frequency cross-asset interactions and investigate their long-term scaling limits. We emphasize the relevance of our approach by providing insights on the role of microscopic features such as momentum and mean-reversion in the multidimensional price formation process. In particular, we recover classical properties of high-dimensional stock correlation matrices.

In open Kelly and Jackson networks, servers are assigned to individual stations, serving customers only where they are assigned. We investigate the performance of modified networks where servers cooperate. A server who would be idle at the assigned station will serve customers at another station, speeding up service there. We assume interchangeable servers: the service rate of a server at a station depends only on the station, not the server. This gives work conservation, which is used in various ways. We investigate three levels of server cooperation, from full cooperation, where all servers are busy when there is work to do anywhere in the network, to one-way cooperation, where a server assigned to one station may assist a server at another, but not the converse. We obtain the same stability conditions for each level and, in a series of examples, obtain substantial performance improvement with server cooperation, even when stations before modification are moderately loaded.

The paper discusses the risk of ruin in insurance coverage of an epidemic in a closed population. The model studied is an extended susceptible–infective–removed (SIR) epidemic model built by Lefèvre and Simon (Methodology Comput. Appl. Prob.22, 2020) as a block-structured Markov process. A fluid component is then introduced to describe the premium amounts received and the care costs reimbursed by the insurance. Our interest is in the risk of collapse of the corresponding reserves of the company. The use of matrix-analytic methods allows us to determine the distribution of ruin time, the probability of ruin, and the final amount of reserves. The case where the reserves are subjected to a Brownian noise is also studied. Finally, some of the results obtained are illustrated for two particular standard SIR epidemic models.

In this work, we study a new model for continuum line-of-sight percolation in a random environment driven by the Poisson–Voronoi tessellation in the d-dimensional Euclidean space. The edges (one-dimensional facets, or simply 1-facets) of this tessellation are the support of a Cox point process, while the vertices (zero-dimensional facets or simply 0-facets) are the support of a Bernoulli point process. Taking the superposition Z of these two processes, two points of Z are linked by an edge if and only if they are sufficiently close and located on the same edge (1-facet) of the supporting tessellation. We study the percolation of the random graph arising from this construction and prove that a 0–1 law, a subcritical phase, and a supercritical phase exist under general assumptions. Our proofs are based on a coarse-graining argument with some notion of stabilization and asymptotic essential connectedness to investigate continuum percolation for Cox point processes. We also give numerical estimates of the critical parameters of the model in the planar case, where our model is intended to represent telecommunications networks in a random environment with obstructive conditions for signal propagation.

We consider a model of a stationary population with random size given by a continuous-state branching process with immigration with a quadratic branching mechanism. We give an exact elementary simulation procedure for the genealogical tree of n individuals randomly chosen among the extant population at a given time. Then we prove the convergence of the renormalized total length of this genealogical tree as n goes to infinity; see also Pfaffelhuber, Wakolbinger and Weisshaupt (2011) in the context of a constant-size population. The limit appears already in Bi and Delmas (2016) but with a different approximation of the full genealogical tree. The proof is based on the ancestral process of the extant population at a fixed time, which was defined by Aldous and Popovic (2005) in the critical case.

We consider stochastic differential equations of the form 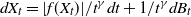$dX_t = |f(X_t)|/t^{\gamma} dt+1/t^{\gamma} dB_t$, where f(x) behaves comparably to 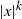$|x|^k$ in a neighborhood of the origin, for $k\in [1,\infty)$. We show that there exists a threshold value $ \,{:}\,{\raise-1.5pt{=}}\, \tilde{\gamma}$ for $\gamma$, depending on k, such that if $\gamma \in (1/2, \tilde{\gamma})$, then 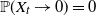$\mathbb{P}(X_t\rightarrow 0) = 0$, and for the rest of the permissible values of 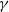$\gamma$, 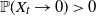$\mathbb{P}(X_t\rightarrow 0)>0$. These results extend to discrete processes that satisfy $X_{n+1}-X_n = f(X_n)/n^\gamma +Y_n/n^\gamma$. Here, $Y_{n+1}$ are martingale differences that are almost surely bounded.

This result shows that for a function F whose second derivative at degenerate saddle points is of polynomial order, it is always possible to escape saddle points via the iteration 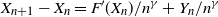$X_{n+1}-X_n =F'(X_n)/n^\gamma +Y_n/n^\gamma$ for a suitable choice of $\gamma$.