Let $(R,\mathfrak {m})$ be a Noetherian local ring and I an ideal of R. We study how local cohomology modules with support in $\mathfrak {m}$ change for small perturbations J of I, that is, for ideals J such that $I\equiv J\bmod \mathfrak {m}^N$ for large N, under the hypothesis that $R/I$ and $R/J$ share the same Hilbert function. As one of our main results, we show that if $R/I$ is generalized Cohen–Macaulay, then the local cohomology modules of $R/J$ are isomorphic to the corresponding local cohomology modules of $R/I$, except possibly the top one. In particular, this answers a question raised by Quy and V. D. Trung. Our approach also allows us to prove that if $R/I$ is Buchsbaum, then so is $R/J$. Finally, under some additional assumptions, we show that if $R/I$ satisfies Serre’s property $(S_n)$, then so does $R/J$.

We develop a dimension theory for coadmissible $\widehat {\mathcal {D}}$-modules on rigid analytic spaces and study those which are of minimal dimension, in analogy to the theory of holonomic $\mathcal {D}$-modules in the algebraic setting. We discuss a number of pathologies contained in this subcategory (modules of infinite length, infinite-dimensional fibres). We prove stability results for closed immersions and the duality functor, and show that all higher direct images of integrable connections restricted to a Zariski open subspace are coadmissible of minimal dimension. It follows that the local cohomology sheaves $\underline {H}^{i}_Z(\mathcal {M})$ with support in a closed analytic subset $Z$ of $X$ are also coadmissible of minimal dimension for any integrable connection $\mathcal {M}$ on $X$.

Let R be a Cohen–Macaulay local ring. It is shown that under some mild conditions, the Cohen–Macaulay property is preserved under linkage. We also study the connection of the (Sn) locus of a horizontally linked module and the attached primes of certain local cohomology modules of its linked module.

Let $A$ be a commutative noetherian ring, let $\mathfrak{a}\subseteq A$ be an ideal, and let $I$ be an injective $A$-module. A basic result in the structure theory of injective modules states that the $A$-module ${{\Gamma }_{\alpha }}\left( I \right)$ consisting of $\mathfrak{a}$-torsion elements is also an injective $A$-module. Recently, de Jong proved a dual result: If $F$ is a flat $A$-module, then the $\mathfrak{a}$-adic completion of $F$ is also a flat $A$-module. In this paper we generalize these facts to commutative noetherian $\text{DG}$-rings: let $A$ be a commutative non-positive $\text{DG}$-ring such that ${{\text{H}}^{0}}\left( A \right)$ is a noetherian ring and for each $i\,<\,0,\,\text{the}\,{{\text{H}}^{0}}\left( A \right)$-module ${{\text{H}}^{i}}\left( A \right)$ is finitely generated. Given an ideal $\overline{\mathfrak{a}}\,\subseteq \,{{\text{H}}^{0}}\left( A \right)$, we show that the local cohomology functor $\text{R}{{\Gamma }_{\overline{\mathfrak{a}}}}$ associated with $\overline{\mathfrak{a}}$ does not increase injective dimension. Dually, the derived $\overline{\mathfrak{a}}$-adic completion functor $\text{L}{{\Lambda }_{\overline{\mathfrak{a}}}}$ does not increase flat dimension.

Let $f$ be a quasi-homogeneous polynomial with an isolated singularity in $\mathbf{C}^{n}$. We compute the length of the ${\mathcal{D}}$-modules ${\mathcal{D}}f^{\unicode[STIX]{x1D706}}/{\mathcal{D}}f^{\unicode[STIX]{x1D706}+1}$ generated by complex powers of $f$ in terms of the Hodge filtration on the top cohomology of the Milnor fiber. When $\unicode[STIX]{x1D706}=-1$ we obtain one more than the reduced genus of the singularity ($\dim H^{n-2}(Z,{\mathcal{O}}_{Z})$ for $Z$ the exceptional fiber of a resolution of singularities). We conjecture that this holds without the quasi-homogeneous assumption. We also deduce that the quotient ${\mathcal{D}}f^{\unicode[STIX]{x1D706}}/{\mathcal{D}}f^{\unicode[STIX]{x1D706}+1}$ is nonzero when $\unicode[STIX]{x1D706}$ is a root of the $b$-function of $f$ (which Saito recently showed fails to hold in the inhomogeneous case). We obtain these results by comparing these ${\mathcal{D}}$-modules to those defined by Etingof and the second author which represent invariants under Hamiltonian flow.

Let $A$ be a complete local ring with a coefficient field $k$ of characteristic zero, and let $Y$ be its spectrum. The de Rham homology and cohomology of $Y$ have been defined by R. Hartshorne using a choice of surjection $R\rightarrow A$ where $R$ is a complete regular local $k$-algebra: the resulting objects are independent of the chosen surjection. We prove that the Hodge–de Rham spectral sequences abutting to the de Rham homology and cohomology of $Y$, beginning with their $E_{2}$-terms, are independent of the chosen surjection (up to a degree shift in the homology case) and consist of finite-dimensional $k$-spaces. These $E_{2}$-terms therefore provide invariants of $A$ analogous to the Lyubeznik numbers. As part of our proofs we develop a theory of Matlis duality in relation to ${\mathcal{D}}$-modules that is of independent interest. Some of the highlights of this theory are that if $R$ is a complete regular local ring containing $k$ and ${\mathcal{D}}={\mathcal{D}}(R,k)$ is the ring of $k$-linear differential operators on $R$, then the Matlis dual $D(M)$ of any left ${\mathcal{D}}$-module $M$ can again be given a structure of left ${\mathcal{D}}$-module, and if $M$ is a holonomic ${\mathcal{D}}$-module, then the de Rham cohomology spaces of $D(M)$ are $k$-dual to those of $M$.

In this paper we study the local cohomology modules of Du Bois singularities. Let $(R,\mathfrak{m})$ be a local ring; we prove that if $R_{\text{red}}$ is Du Bois, then $H_{\mathfrak{m}}^{i}(R)\rightarrow H_{\mathfrak{m}}^{i}(R_{\text{red}})$ is surjective for every $i$. We find many applications of this result. For example, we answer a question of Kovács and Schwede [Inversion of adjunction for rational and Du Bois pairs, Algebra Number Theory 10 (2016), 969–1000; MR 3531359] on the Cohen–Macaulay property of Du Bois singularities. We obtain results on the injectivity of $\operatorname{Ext}$ that provide substantial partial answers to questions in Eisenbud et al. [Cohomology on toric varieties and local cohomology with monomial supports, J. Symbolic Comput. 29 (2000), 583–600] in characteristic $0$. These results can also be viewed as generalizations of the Kodaira vanishing theorem for Cohen–Macaulay Du Bois varieties. We prove results on the set-theoretic Cohen–Macaulayness of the defining ideal of Du Bois singularities, which are characteristic-$0$ analogs and generalizations of results of Singh–Walther and answer some of their questions in Singh and Walther [On the arithmetic rank of certain Segre products, in Commutative algebra and algebraic geometry, Contemporary Mathematics, vol. 390 (American Mathematical Society, Providence, RI, 2005), 147–155]. We extend results on the relation between Koszul cohomology and local cohomology for $F$-injective and Du Bois singularities first shown in Hochster and Roberts [The purity of the Frobenius and local cohomology, Adv. Math. 21 (1976), 117–172; MR 0417172 (54 #5230)]. We also prove that singularities of dense $F$-injective type deform.

Let $(R,\mathfrak{m})$ denote a local Cohen–Macaulay ring and $I$ a non-nilpotent ideal of $R$. The purpose of this article is to investigate Faltings’ finiteness dimension ${{f}_{I}}(R)$ and the equidimensionalness of certain homomorphic images of $R$. As a consequence we deduce that ${{f}_{I}}(R)=\max \{1,\text{ht}I\}$, and if $\text{mAs}{{\text{s}}_{R}}(R/I)$ is contained in $\text{As}{{\text{s}}_{R}}(R)$, then the ring $R/I+{{\bigcup }_{n\ge 1}}(0{{:}_{R}}{{I}^{n}})$ is equidimensional of dimension dim $R-1$. Moreover, we will obtain a lower bound for injective dimension of the local cohomology module $H_{I}^{\text{ht}I}(R)$, in the case where $(R,\mathfrak{m})$ is a complete equidimensional local ring.

Let be a Noetherian local ring and let M be a finitely generated R-module of dimension d. Let be a system of parameters of M and let be a d-tuple of positive integers. In this paper we study the length of generalized fractions M(1/(x1, … , xd, 1)), which was introduced by Sharp and Hamieh. First, we study the growth of the function

Then we give an explicit calculation for the function in the case in which M admits a certain Macaulay extension. Most previous results on this topic are improved in a clearly understandable way.

Harm Derksen made a conjecture concerning degree bounds for the syzygies of rings of polynomial invariants in the non-modular case [Degree bounds for syzygies of invariants, Adv. Math. 185 (2004), 207–214]. We provide counterexamples to this conjecture, but also prove a slightly weakened version. We also prove some general results that give degree bounds on the homology of complexes and of $\text{Tor}\,$ groups.

We compute the characters of the simple $\text{GL}$ -equivariant holonomic ${\mathcal{D}}$ -modules on the vector spaces of general, symmetric, and skew-symmetric matrices. We realize some of these ${\mathcal{D}}$ -modules explicitly as subquotients in the pole order filtration associated to the $\text{determinant}/\text{Pfaffian}$ of a generic matrix, and others as local cohomology modules. We give a direct proof of a conjecture of Levasseur in the case of general and skew-symmetric matrices, and provide counterexamples in the case of symmetric matrices. The character calculations are used in subsequent work with Weyman to describe the ${\mathcal{D}}$ -module composition factors of local cohomology modules with determinantal and Pfaffian support.

Let $\mathfrak{a}$ be an ideal of a Noetherian local ring $R$ and let $C$ be a semidualizing $R$-module. For an $R$-module $X$, we denote any of the quantities 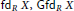$\text{f}{{\text{d}}_{R}}X,\,\text{Gf}{{\text{d}}_{R}}X$ and ${{\text{G}}_{\text{C}}}-\text{f}{{\text{d}}_{R}}\,X\,\text{by}\,\text{T}\left( X \right)$. Let $M$ be an $R$-module such that $\text{H}_{\mathfrak{a}}^{i}\left( M \right)\,=\,0$ for all $i\,\ne \,n$. It is proved that if $T\left( M \right)\,<\,\infty$, then $\text{T}\left( \text{H}_{\mathfrak{a}}^{n}\left( M \right) \right)\,\le \,\text{T}\left( M \right)\,+\,n$, and the equality holds whenever $M$ is finitely generated. With the aid of these results, among other things, we characterize Cohen–Macaulay modules, dualizing modules, and Gorenstein rings.

Let $(S,\mathfrak{m})$ be an $n$-dimensional regular local ring essentially of finite type over a field and let $\mathfrak{a}$ be an ideal of $S$. We prove that if $\text{depth}\,S/\mathfrak{a}\geqslant 3$, then the cohomological dimension $\text{cd}(S,\mathfrak{a})$ of $\mathfrak{a}$ is less than or equal to $n-3$. This settles a conjecture of Varbaro for such an $S$. We also show, under the assumption that $S$ has an algebraically closed residue field of characteristic zero, that if $\text{depth}\,S/\mathfrak{a}\geqslant 4$, then $\text{cd}(S,\mathfrak{a})\leqslant n-4$ if and only if the local Picard group of the completion $\widehat{S/\mathfrak{a}}$ is torsion. We give a number of applications, including a vanishing result on Lyubeznik’s numbers, and sharp bounds on the cohomological dimension of ideals whose quotients satisfy good depth conditions such as Serre’s conditions $(S_{i})$.

Inspired by symplectic geometry and a microlocal characterizations of perverse (constructible) sheaves we consider an alternative definition of perverse coherent sheaves. We show that a coherent sheaf is perverse if and only if $R{\rm\Gamma}_{Z}{\mathcal{F}}$ is concentrated in degree $0$ for special subvarieties $Z$ of $X$. These subvarieties $Z$ are analogs of Lagrangians in the symplectic case.

Let $\left( R,\,m \right)$ be a non-zero commutative Noetherian local ring (with identity) and let $M$ be a non-zero finitely generated $R$-module. In this paper for any $\mathfrak{p}\,\in \,\text{Spec}\left( R \right)$ we show that

1

$$\text{injdi}{{\text{m}}_{{{R}_{\mathfrak{p}}}}}\,H_{\mathfrak{p}{{R}_{\mathfrak{p}}}}^{i-\dim\left( {R}/{\mathfrak{p}}\; \right)}\left( {{M}_{\mathfrak{p}}} \right)$$

1

$$\text{f}{{\text{d}}_{{{R}_{\mathfrak{p}}}}}H_{\mathfrak{p}}^{i-\dim\left( {R}/{\mathfrak{p}}\; \right)}\left( {{M}_{\mathfrak{p}}} \right)$$

are bounded from above by $\text{injdi}{{\text{m}}_{R}}\,H_{\text{m}}^{i}\left( M \right)$ and $\text{f}{{\text{d}}_{R}}\,H_{\text{m}}^{i}\left( M \right)$ respectively, for all integers $i\,\ge \,\dim\left( {R}/{\mathfrak{p}}\; \right)$.

Let $S$ be a polynomial ring over a field $K$ and let $I$ be a monomial ideal of $S$. We say that $I$ is MHC (that is, $I$ satisfies the maximal height condition for the associated primes of $I$) if there exists a prime ideal $\mathfrak{p}\in {\mathrm{Ass} }_{S} \hspace{0.167em} S/ I$ for which $\mathrm{ht} (\mathfrak{p})$ equals the number of indeterminates that appear in the minimal set of monomials generating $I$. Let $I= { \mathop{\bigcap }\nolimits}_{i= 1}^{k} {Q}_{i} $ be the irreducible decomposition of $I$ and let $m(I)= \max \{ \vert Q_{i}\vert - \mathrm{ht} ({Q}_{i} ): 1\leq i\leq k\} $, where $\vert {Q}_{i} \vert $ denotes the total degree of ${Q}_{i} $. Then it can be seen that when $I$ is primary, $\mathrm{reg} (S/ I)= m(I)$. In this paper we improve this result and show that whenever $I$ is MHC, then $\mathrm{reg} (S/ I)= m(I)$ provided $\vert {\mathrm{Ass} }_{S} \hspace{0.167em} S/ I\vert \leq 2$. We also prove that $m({I}^{n} )\leq n\max \{ \vert Q_{i}\vert : 1\leq i\leq ~k\} - \mathrm{ht} (I)$, for all $n\geq 1$. In addition we show that if $I$ is MHC and $w$ is an indeterminate which is not in the monomials generating $I$, then $\mathrm{reg} (S/ \mathop{(I+ {w}^{d} S)}\nolimits ^{n} )\leq \mathrm{reg} (S/ I)+ nd- 1$ for all $n\geq 1$ and $d$ large enough. Finally, we implement an algorithm for the computation of $m(I)$.

Let 𝔞 be an ideal of a Noetherian ring R. Let s be a nonnegative integer and let M and N be two R-modules such that ExtjR(M/𝔞M,Hi𝔞(N)) is finite for all i<s and all j≥0 . We show that HomR (R/𝔞,Hs𝔞(M,N)) is finite provided ExtsR(M/𝔞M,N) is a finite R-module. In addition, for finite R-modules M and N, we prove that if Hi𝔞(M,N) is minimax for all i<s, then HomR (R/𝔞,Hs𝔞(M,N)) is finite. These are two generalizations of the result of Brodmann and Lashgari [‘A finiteness result for associated primes of local cohomology modules’, Proc. Amer. Math. Soc. 128 (2000), 2851–2853] and a recent result due to Chu [‘Cofiniteness and finiteness of generalized local cohomology modules’, Bull. Aust. Math. Soc. 80 (2009), 244–250]. We also introduce a generalization of the concept of cofiniteness and recover some results for it.

Let I be an ideal of a commutative Noetherian local ring R, and M and N two finitely generated modules. Let t be a positive integer. We mainly prove that (i) if HIi(M,N) is Artinian for all i<t, then HIi(M,N) is I-cofinite for all i<t and Hom(R/I,HIt(M,N)) is finitely generated; (ii) if d=pd(M)<∞ and dim N=n<∞, then HId+n(M,N) is I-cofinite. We also prove that if M is a nonzero cyclic R-module, then HIi(N) is finitely generated for all i<t if and only if HIi(M,N) is finitely generated for all i<t.

Let $K$ be a field of characteristic $p$ and let $G$ be a finite group of order divisible by $p$. The regularity conjecture states that the Castelnuovo–Mumford regularity of the cohomology ring $H^*(G,K)$ is always equal to 0. We prove that if the regularity conjecture holds for a finite group $H$, then it holds for the wreath product $H\wr\mathbb{Z}/p$. As a corollary, we prove the regularity conjecture for the symmetric groups $\varSigma_n$. The significance of this is that it is the first set of examples for which the regularity conjecture has been checked, where the difference between the Krull dimension and the depth of the cohomology ring is large. If this difference is at most 2, the regularity conjecture is already known to hold by previous work.

For more general wreath products, we have not managed to prove the regularity conjecture. Instead we prove a weaker statement: namely, that the dimensions of the cohomology groups are polynomial on residue classes (PORC) in the sense of Higman.

We describe an algorithm for computing parameter-test-ideals in certain local Cohen–Macaulay rings. The algorithm is based on the study of a Frobenius map on the injective hull of the residue field of the ring and on the application of Sharp’s notion of ‘special ideals’. Our techniques also provide an algorithm for computing indices of nilpotency of Frobenius actions on top local cohomology modules of the ring and on the injective hull of its residue field. The study of nilpotent elements on injective hulls of residue fields also yields a great simplification of the proof of the celebrated result in the article Generators of D-modules in positive characteristic (J. Alvarez-Montaner, M. Blickle and G. Lyubeznik, Math. Res. Lett. 12 (2005), 459–473).