Let 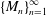$\{R_{k}\}_{k=1}^{\infty }$ be a sequence of expanding integer matrices in $M_{n}(\mathbb {Z})$ , and let $\{D_{k}\}_{k=1}^{\infty }$ be a sequence of finite digit sets with integer vectors in ${\mathbb Z}^{n}$ . In this paper, we prove that under certain conditions in terms of $(R_{k},D_{k})$ for $k\ge 1$ , the Moran measure

$$ \begin{align*} \mu_{\{R_{k}\},\{D_{k}\}}:=\delta_{R_{1}^{-1}D_{1}}\ast\delta_{R_{1}^{-1}R_{2}^{-1}D_{2}}\ast\cdots \end{align*} $$

$(R,D)$

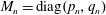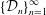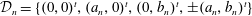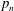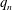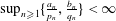Let $\{M_{n}\}_{n=1}^{\infty }$ be a sequence of expanding matrices with $M_{n}=\operatorname{diag}(p_{n},q_{n})$, and let $\{{\mathcal{D}}_{n}\}_{n=1}^{\infty }$ be a sequence of digit sets with ${\mathcal{D}}_{n}=\{(0,0)^{t},(a_{n},0)^{t},(0,b_{n})^{t},\pm (a_{n},b_{n})^{t}\}$, where $p_{n}$, $q_{n}$, $a_{n}$ and $b_{n}$ are positive integers for all $n\geqslant 1$. If $\sup _{n\geqslant 1}\{\frac{a_{n}}{p_{n}},\frac{b_{n}}{q_{n}}\}<\infty$, then the infinite convolution 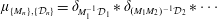$\unicode[STIX]{x1D707}_{\{M_{n}\},\{{\mathcal{D}}_{n}\}}=\unicode[STIX]{x1D6FF}_{M_{1}^{-1}{\mathcal{D}}_{1}}\ast \unicode[STIX]{x1D6FF}_{(M_{1}M_{2})^{-1}{\mathcal{D}}_{2}}\ast \cdots \,$ is a Borel probability measure (Cantor–Dust–Moran measure). In this paper, we investigate whenever there exists a discrete set $\unicode[STIX]{x1D6EC}$ such that 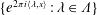$\{e^{2\unicode[STIX]{x1D70B}i\langle \unicode[STIX]{x1D706},x\rangle }:\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}\}$ is an orthonormal basis for 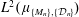$L^{2}(\unicode[STIX]{x1D707}_{\{M_{n}\},\{{\mathcal{D}}_{n}\}})$.

The paper gives a survey of the theory of univariate characteristic functions. These functions were originally introduced as tools in the study of limit theorems but it was later realized that they had an independent mathematical interest. Those parts of the theory which can be found in textbooks are treated only briefly; the main emphasis is placed on more recent developments and areas where active research is still in progress.