Let 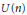G be a finite group and r be a prime divisor of the order of G. An irreducible character of G is said to be quasi r-Steinberg if it is non-zero on every r-regular element of G. A quasi r-Steinberg character of degree $\displaystyle |Syl_r(G)|$ is said to be weak r-Steinberg if it vanishes on the r-singular elements of $G.$ In this article, we classify the quasi r-Steinberg cuspidal characters of the general linear group $GL(n,q).$ Then we characterize the quasi r-Steinberg characters of $GL(2,q)$ and $GL(3,q).$ Finally, we obtain a classification of the weak r-Steinberg characters of $GL(n,q).$

We find an upper bound for the number of groups of order n up to isomorphism in the variety ${\mathfrak {S}}={\mathfrak {A}_p}{\mathfrak {A}_q}{\mathfrak {A}_r}$, where p, q and r are distinct primes. We also find a bound on the orders and on the number of conjugacy classes of subgroups that are maximal amongst the subgroups of the general linear group that are also in the variety $\mathfrak {A}_q\mathfrak {A}_r$.

Let K be an infinite field of characteristic $p>0$ and let $\lambda, \mu$ be partitions, where $\mu$ has two parts. We find sufficient arithmetic conditions on $p, \lambda, \mu$ for the existence of a nonzero homomorphism $\Delta(\lambda) \to \Delta (\mu)$ of Weyl modules for the general linear group $GL_n(K)$. Also, for each p we find sufficient conditions so that the corresponding homomorphism spaces have dimension at least 2.

Let G be a group. A subset X of G is said to be nonnilpotent if for any two distinct elements x and y in X, 〈x,y〉 is a nonnilpotent subgroup of G. If, for any other nonnilpotent subset X′ in G, ∣X∣≥∣X′ ∣, then X is said to be a maximal nonnilpotent subset and the cardinality of this subset is denoted by ω(𝒩G) . Using nilpotent nilpotentizers we find a lower bound for the cardinality of a maximal nonnilpotent subset of a finite group and apply this to the general linear group GL (n,q) . For all prime powers q we determine the cardinality of a maximal nonnilpotent subset of the projective special linear group PSL (2,q) , and we characterize all nonabelian finite simple groups G with ω(𝒩G)≤57 .

Let G be a group. A subset N of G is a set of pairwise noncommuting elements if xy⁄=yx for any two distinct elements x and y in N. If ∣N∣≥∣M∣ for any other set of pairwise noncommuting elements M in G, then N is said to be a maximal subset of pairwise noncommuting elements. In this paper we determine the cardinality of a maximal subset of pairwise noncommuting elements in a three-dimensional general linear group. Moreover, we show how to modify a given maximal subset of pairwise noncommuting elements into another maximal subset of pairwise noncommuting elements that contains a given ‘generating element’ from each maximal torus.

The higher Lie characters of the symmetric group Sn arise from the Poincaré-Birkhoff-Witt basis of the free associative algebra. They are indexed by the partitions of n and sum up to the regular character of Sn. A combinatorial description of the multiplicities of their irreducible components is given. As a special case the Kraśkiewicz-Weyman result on the multiplicities of the classical Lie character is obtained.

Let $U(n)$ be the group of 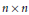$n\,\times \,n$ unitary matrices. We show that if 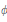$\phi $ is a linear transformation sending 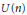$U(n)$ into 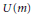$U(m)$, then 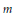$m$ is a multiple of $n$, and 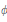$\phi $ has the form

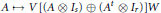$$A\,\mapsto \,V[(A\,\otimes \,{{I}_{s}})\,\otimes \,({{A}^{t}}\,\otimes \,{{I}_{r}})]W$$

for some $V,\,W\,\in \,U(m)$. From this result, one easily deduces the characterization of linear operators that map 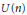$U(n)$ into itself obtained by Marcus. Further generalization of the main theorem is also discussed.

We study modules for the general linear group (over an infinite field of arbitrary characteristic) which are direct summands of tensor products of exterior powers and symmetric powers of the natural module. These modules, which we call listing modules, include the tilting modules and the injective modules for Schur algebras. The modules are studied via their relationship to linear source modules for symmetric groups on the one hand, and simple modules for Schur superalgebras on the other. Listing modules are parametrized by certain pairs of partitions. They are used to describe, by generators and relations, the Grothendieck ring of polynomial functors generated by the symmetric and exterior powers. We also (continuing work of J. Grabmeier) describe the vertices and sources of linear source modules for symmetric groups. 2000 Mathematical Subject Classification: 20G05, 20C30.

Let R be a not necessarily commutative local ring, M a free R-module, and π ∈ GL(M) such that B(π) = im(π –1)is a subspace of M. Then π = σ1…σtρ, where σi are simple mappings of given types, ρ is a simple mapping, B(sgr;i) and B(ρ) are subspaces and t ≤ dim B(π).