We introduce and study the weak Glimm property for 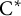$\mathrm{C}^{*}$-algebras, and also a property we shall call (HS$_0$). We show that the properties of being nowhere scattered and residual (HS$_0$) are equivalent for any 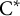$\mathrm{C}^{*}$-algebra. Also, for a 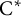$\mathrm{C}^{*}$-algebra with the weak Glimm property, the properties of being purely infinite and weakly purely infinite are equivalent. It follows that for a $\mathrm{C}^{*}$-algebra with the weak Glimm property such that the absolute value of every nonzero, square-zero, element is properly infinite, the properties of being (weakly, locally) purely infinite, nowhere scattered, residual (HS$_0$), residual (HS$_{\text {t}}$), and residual (HI) are all equivalent, and are equivalent to the global Glimm property. This gives a partial affirmative answer to the global Glimm problem, as well as certain open questions raised by Kirchberg and Rørdam.

We show that a virtually residually finite rationally solvable (RFRS) group $G$ of type $\mathtt {FP}_n(\mathbb {Q})$ virtually algebraically fibres with kernel of type $\mathtt {FP}_n(\mathbb {Q})$ if and only if the first $n$ $\ell ^2$-Betti numbers of $G$ vanish, that is, $b_p^{(2)}(G) = 0$ for $0 \leqslant p \leqslant n$. This confirms a conjecture of Kielak. We also offer a variant of this result over other fields, in particular in positive characteristic. As an application of the main result, we show that amenable virtually RFRS groups of type $\mathtt {FP}(\mathbb {Q})$ are virtually Abelian. It then follows that if $G$ is a virtually RFRS group of type $\mathtt {FP}(\mathbb {Q})$ such that $\mathbb {Z} G$ is Noetherian, then $G$ is virtually Abelian. This confirms a conjecture of Baer for the class of virtually RFRS groups of type $\mathtt {FP}(\mathbb {Q})$, which includes (for instance) the class of virtually compact special groups.

A left orderable monster is a finitely generated left orderable group all of whose fixed point-free actions on the line are proximal: the action is semiconjugate to a minimal action so that for every bounded interval I and open interval J, there is a group element that sends I into J. In his 2018 ICM address, Navas asked about the existence of left orderable monsters. By now there are several examples, all of which are finitely generated but not finitely presentable. We provide the first examples of left orderable monsters that are finitely presentable, and even of type $F_\infty $. These groups satisfy several additional properties separating them from the previous examples: they are not simple, they act minimally on the circle, and they have an infinite-dimensional space of homogeneous quasimorphisms. Our construction is flexible enough that it produces infinitely many isomorphism classes of finitely presented (and type $F_{\infty }$) left orderable monsters.

We introduce “braided” versions of self-similar groups and Röver–Nekrashevych groups, and study their finiteness properties. This generalizes work of Aroca and Cumplido, and the first author and Wu, who considered the case when the self-similar groups are what we call “self-identical.” In particular, we use a braided version of the Grigorchuk group to construct a new group called the “braided Röver group,” which we prove is of type $\operatorname {\mathrm {F}}_\infty $. Our techniques involve using so-called d-ary cloning systems to construct the groups, and analyzing certain complexes of embedded disks in a surface to understand their finiteness properties.

Given a commutative unital ring R, we show that the finiteness length of a group G is bounded above by the finiteness length of the Borel subgroup of rank one $\textbf {B}_2^{\circ }(R)=\left ( \begin {smallmatrix} * & * \\ 0 & * \end {smallmatrix}\right )\leq \operatorname {\textrm {SL}}_2(R)$ whenever G admits certain R-representations with metabelian image. Combined with results due to Bestvina–Eskin–Wortman and Gandini, this gives a new proof of (a generalization of) Bux’s equality on the finiteness length of S-arithmetic Borel groups. We also give an alternative proof of an unpublished theorem due to Strebel, characterizing finite presentability of Abels’ groups $\textbf {A}_n(R) \leq \operatorname {\textrm {GL}}_n(R)$ in terms of n and $\textbf {B}_2^{\circ }(R)$ . This generalizes earlier results due to Remeslennikov, Holz, Lyul’ko, Cornulier–Tessera, and points out to a conjecture about the finiteness length of such groups.

We give a complete computation of the Bieri–Neumann–Strebel–Renz invariants Σm(Hn) of the Houghton groups Hn. Partial results were previously obtained by the author, with a conjecture about the full picture, which we now confirm. The proof involves covering relevant subcomplexes of an associated CAT (0) cube complex by their intersections with certain locally convex subcomplexes, and then applying a strong form of the Nerve Lemma. A consequence of the full computation is that for each 1 ≤ m ≤ n − 1, Hn admits a map onto ℤ whose kernel is of type Fm−1 but not Fm; moreover, no such kernel is ever of type Fn−1.

We study basic properties of the category of smooth representations of a p-adic group G with coefficients in any commutative ring R in which p is invertible. Our main purpose is to prove that Hecke algebras are Noetherian whenever R is; this question arose naturally with Bernstein's fundamental work for R = ℂ, in which case he proved this Noetherian property. In a first step, we prove that Noetherianity would follow from a generalization of the so-called second adjointness property between parabolic functors, also due to Bernstein for complex representations. Then, to attack this second adjointness, we introduce and study ‘parahoric functors’ between representations of groups of integral points of smooth integral models of G and of their ‘Levi’ subgroups. Applying our general study to Bruhat-Tits parahoric models, we get second adjointness for minimal parabolic groups. For non-minimal parabolic subgroups, we have to restrict to classical and linear groups, and use smooth models associated with Bushnell-Kutzko and Stevens semi-simple characters. The same strategy should apply to ‘tame’ groups, using Yu's smooth models and generic characters.