We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let $\pi \colon \mathcal {X}\to B$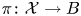
be a family whose general fibre $X_b$
is a $(d_1,\,\ldots,\,d_a)$
-polarization on a general abelian variety, where $1\leq d_i\leq 2$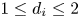
, $i=1,\,\ldots,\,a$
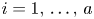and $a\geq 4$
. We show that the fibres are in the same birational class if all the $(m,\,0)$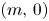
-forms on $X_b$
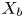are liftable to $(m,\,0)$
-forms on $\mathcal {X}$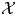
, where $m=1$
and $m=a-1$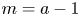
. Actually, we show a general criteria to establish whether the fibres of certain families belong to the same birational class.
