We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
$A_{\infty }$-STRUCTURES
In this paper, for each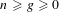 
$n\geqslant g\geqslant 0$ we consider the moduli stack 
$\widetilde{{\mathcal{U}}}_{g,n}^{ns}$ of curves 
$(C,p_{1},\ldots ,p_{n},v_{1},\ldots ,v_{n})$ of arithmetic genus 
$g$ with 
$n$ smooth marked points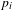 
$p_{i}$ and nonzero tangent vectors 
$v_{i}$ at them, such that the divisor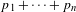 
$p_{1}+\cdots +p_{n}$ is nonspecial (has 
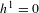$h^{1}=0$) and ample. With some mild restrictions on the characteristic we show that it is a scheme, affine over the Grassmannian 
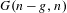$G(n-g,n)$. We also construct an isomorphism of 
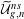$\widetilde{{\mathcal{U}}}_{g,n}^{ns}$ with a certain relative moduli of 
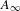$A_{\infty }$-structures (up to an equivalence) over a family of graded associative algebras parametrized by 
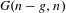$G(n-g,n)$.
