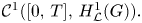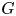Let $G$ be a compact Lie group. In this article, we investigate the Cauchy problem for a nonlinear wave equation with the viscoelastic damping on $G$. More precisely, we investigate some $L^2$-estimates for the solution to the homogeneous nonlinear viscoelastic damped wave equation on $G$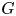 utilizing the group Fourier transform on $G$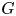. We also prove that there is no improvement of any decay rate for the norm $\|u(t,\,\cdot )\|_{L^2(G)}$ 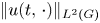by further assuming the $L^1(G)$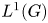-regularity of initial data. Finally, using the noncommutative Fourier analysis on compact Lie groups, we prove a local in time existence result in the energy space $\mathcal {C}^1([0,\,T],\,H^1_{\mathcal {L}}(G)).$

We prove that solutions to the quintic semilinear wave equation with variable coefficients in ${{\mathbb {R}}}^{1+3}$ scatter to a solution to the corresponding linear wave equation. The coefficients are small and decay as $|x|\to \infty$, but are allowed to be time dependent. The proof uses local energy decay estimates to establish the decay of the $L^{6}$ norm of the solution as $t\to \infty$.

This paper is devoted to an extension of the finite-energy condition for extended Runge-Kutta-Nyström (ERKN) integrators and applications to nonlinear wave equations. We begin with an error analysis for the integrators for multi-frequency highly oscillatory systems , where M is positive semi-definite, . The highly oscillatory system is due to the semi-discretisation of conservative, or dissipative, nonlinear wave equations. The structure of such a matrix M and initial conditions are based on particular spatial discretisations. Similarly to the error analysis for Gaustchi-type methods of order two, where a finite-energy condition bounding amplitudes of high oscillations is satisfied by the solution, a finite-energy condition for the semi-discretisation of nonlinear wave equations is introduced and analysed. These ensure that the error bound of ERKN methods is independent of . Since stepsizes are not restricted by frequencies of M, large stepsizes can be employed by our ERKN integrators of arbitrary high order. Numerical experiments provided in this paper have demonstrated that our results are truly promising, and consistent with our analysis and prediction.

The modulation instability of a circularly polarized laser pulse in a magnetized non-Maxwellian plasma is investigated. Based on a relativistic fluid model, the nonlinear interaction of an intense circularly polarized laser beam with a non-Maxwellian magnetized plasma is described. Nonlinear dispersion relation and growth rate of the instability for left- and right-hand polarizations are derived. The effect of temperature, external magnetic field, value of Kappa and state of polarization on the growth rate are analyzed. It is shown that the growth rate increases with increase in the magnetic field for the right-hand polarization and inversely it decreases for the left-hand one. Also it is observed that existence of super-thermal particles causes the decrease in the growth.

Self-focusing of an intense circularly-polarized laser beam in a hot electron-positron-ion magneto-plasma is studied. Using a relativistic fluid model, nonlinear equation describing laser-plasma interaction in the quasi-neutral approximation is derived. Expanding nonlinear current density in terms of normalized vector potential and saving only the parabolic terms, we investigated the self-focusing phenomenon for right- and left-hand circularly polarized laser beams. The evolution of laser beam spot size with Gaussian profile is considered. Effects of the external magnetic field, fraction of electron-positron pairs, and also the kind of polarization on the self-focusing property are studied. It is shown that a mixture of electron-positron pairs to the ion-electron plasma modifies the behavior of plasma with respect to the external magnetic field.

The numerical solution of blow-up problems for nonlinear wave equations on unbounded spatial domains is considered. Applying the unified approach, which is based on the operator splitting method, we construct the efficient nonlinear local absorbing boundary conditions for the nonlinear wave equation, and reduce the nonlinear problem on the unbounded spatial domain to an initial-boundary-value problem on a bounded domain. Then the finite difference method is used to solve the reduced problem on the bounded computational domain. Finally, a broad range of numerical examples are given to demonstrate the effectiveness and accuracy of our method, and some interesting propagation and behaviors of the blow-up problems for nonlinear wave equations are observed.

In this paper, we analyze the relation between the shape of the bounded traveling wave solutions and dissipation coefficient of nonlinear wave equation with cubic term by the theory and method of planar dynamical systems. Two critical values which can characterize the scale of dissipation effect are obtained. If dissipation effect is not less than a certain critical value, the traveling wave solutions appear as kink profile; while if it is less than this critical value, they appear as damped oscillatory. All expressions of bounded traveling wave solutions are presented, including exact expressions of bell and kink profile solitary wave solutions, as well as approximate expressions of damped oscillatory solutions. For approximate damped oscillatory solution, using homogenization principle, we give its error estimate by establishing the integral equation which reflects the relations between the exact and approximate solutions. It can be seen that the error is an infinitesimal decreasing in the exponential form.