Let  $X$ be an
$X$ be an  $n$-dimensional, finite, simply connected
$n$-dimensional, finite, simply connected  $\text{CW}$ complex and set
$\text{CW}$ complex and set
 $${{\alpha }_{X}}\,=\,\underset{i}{\mathop{\lim \,\sup }}\,\frac{\log \,\text{rank}\,{{\pi }_{i}}\left( X \right)}{i}$$
$${{\alpha }_{X}}\,=\,\underset{i}{\mathop{\lim \,\sup }}\,\frac{\log \,\text{rank}\,{{\pi }_{i}}\left( X \right)}{i}$$
When 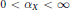 $0<{{\alpha }_{X}}<\infty $, we give upper and lower bounds for
$0<{{\alpha }_{X}}<\infty $, we give upper and lower bounds for 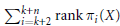 $\sum\nolimits_{i=k+2}^{k+n}{\,\text{rank}}\text{ }{{\pi }_{i}}\left( X \right)$ for
$\sum\nolimits_{i=k+2}^{k+n}{\,\text{rank}}\text{ }{{\pi }_{i}}\left( X \right)$ for 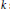 $k$ sufficiently large. We also show for any
$k$ sufficiently large. We also show for any 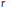 $r$ that
$r$ that 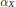 $\alpha x$ can be estimated from the integers
$\alpha x$ can be estimated from the integers 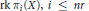 $\text{rk }{{\pi }_{i}}\left( X \right),\,i\,\le \,nr$ with an error bound depending explicitly on
$\text{rk }{{\pi }_{i}}\left( X \right),\,i\,\le \,nr$ with an error bound depending explicitly on  $r$.
$r$.