Furstenberg’s $\times 2\times 3$ theorem asserts that the double sequence $(2^{m}3^{n}\unicode[STIX]{x1D6FC})_{m,n\geq 1}$ is dense modulo one for every irrational $\unicode[STIX]{x1D6FC}$. The same holds with $2$ and $3$ replaced by any two multiplicatively independent integers. Here we obtain the same result for the sequences $((\begin{smallmatrix}m+n\\ d\end{smallmatrix})a^{m}b^{n}\unicode[STIX]{x1D6FC})_{m,n\geq 1}$ for any non-negative integer $d$ and irrational $\unicode[STIX]{x1D6FC}$, and for the sequence $(P(m)a^{m}b^{n})_{m,n\geq 1}$, where $P$ is any polynomial with at least one irrational coefficient. Similarly to Furstenberg’s theorem, both results are obtained by considering appropriate dynamical systems.

Let $G$ be a group acting properly by isometries and with a strongly contracting element on a geodesic metric space. Let $N$ be an infinite normal subgroup of $G$ and let $\unicode[STIX]{x1D6FF}_{N}$ and $\unicode[STIX]{x1D6FF}_{G}$ be the growth rates of $N$ and $G$ with respect to the pseudo-metric induced by the action. We prove that if $G$ has purely exponential growth with respect to the pseudo-metric, then $\unicode[STIX]{x1D6FF}_{N}/\unicode[STIX]{x1D6FF}_{G}>1/2$. Our result applies to suitable actions of hyperbolic groups, right-angled Artin groups and other CAT(0) groups, mapping class groups, snowflake groups, small cancellation groups, etc. This extends Grigorchuk’s original result on free groups with respect to a word metric and a recent result of Matsuzaki, Yabuki and Jaerisch on groups acting on hyperbolic spaces to a much wider class of groups acting on spaces that are not necessarily hyperbolic.

In this paper we study digit frequencies in the setting of expansions in non-integer bases, and self-affine sets with non-empty interior. Within expansions in non-integer bases we show that if $\unicode[STIX]{x1D6FD}\in (1,1.787\ldots )$ then every $x\in (0,1/(\unicode[STIX]{x1D6FD}-1))$ has a simply normal $\unicode[STIX]{x1D6FD}$-expansion. We also prove that if $\unicode[STIX]{x1D6FD}\in (1,(1+\sqrt{5})/2)$ then every $x\in (0,1/(\unicode[STIX]{x1D6FD}-1))$ has a $\unicode[STIX]{x1D6FD}$-expansion for which the digit frequency does not exist, and a $\unicode[STIX]{x1D6FD}$-expansion with limiting frequency of zeros $p$, where $p$ is any real number sufficiently close to $1/2$. For a class of planar self-affine sets we show that if the horizontal contraction lies in a certain parameter space and the vertical contractions are sufficiently close to $1$, then every non-trivial vertical fibre contains an interval. Our approach lends itself to explicit calculation and gives rise to new examples of self-affine sets with non-empty interior. One particular strength of our approach is that it allows for different rates of contraction in the vertical direction.

Suppose $(X,\unicode[STIX]{x1D70E})$ is a subshift, $P_{X}(n)$ is the word complexity function of $X$, and $\text{Aut}(X)$ is the group of automorphisms of $X$. We show that if $P_{X}(n)=o(n^{2}/\log ^{2}n)$, then $\text{Aut}(X)$ is amenable (as a countable, discrete group). We further show that if $P_{X}(n)=o(n^{2})$, then $\text{Aut}(X)$ can never contain a non-abelian free monoid (and, in particular, can never contain a non-abelian free subgroup). This is in contrast to recent examples, due to Salo and Schraudner, of subshifts with quadratic complexity that do contain such a monoid.

We consider a class of skew product maps of interval diffeomorphisms over the doubling map. The interval maps fix the end points of the interval. It is assumed that the system has zero fiber Lyapunov exponent at one endpoint and zero or positive fiber Lyapunov exponent at the other endpoint. We prove the appearance of on–off intermittency. This is done using the equivalent description of chaotic walks: random walks driven by the doubling map. The analysis further relies on approximating the chaotic walks by Markov random walks, that are constructed using Markov partitions for the doubling map.

In this paper, we study an interesting curve, the so-called Manhattan curve, associated with a pair of boundary-preserving Fuchsian representations of a (non-compact) surface; in particular, representations corresponding to Riemann surfaces with cusps. Using thermodynamic formalism (for countable state Markov shifts), we prove the analyticity of the Manhattan curve. Moreover, we derive several dynamical and geometric rigidity results, which generalize results of Burger [Intersection, the Manhattan curve, and Patterson–Sullivan theory in rank 2. Int. Math. Res. Not.1993(7) (1993), 217–225] and Sharp [The Manhattan curve and the correlation of length spectra on hyperbolic surfaces. Math. Z.228(4) (1998), 745–750] for convex cocompact Fuchsian representations.

Let $\unicode[STIX]{x1D6FC}\in \mathbb{R}\backslash \mathbb{Q}$ and $\unicode[STIX]{x1D6FD}(\unicode[STIX]{x1D6FC})=\limsup _{n\rightarrow \infty }(\ln q_{n+1})/q_{n}<\infty$, where 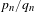$p_{n}/q_{n}$ is the continued fraction approximation to $\unicode[STIX]{x1D6FC}$. Let 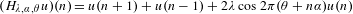$(H_{\unicode[STIX]{x1D706},\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}u)(n)=u(n+1)+u(n-1)+2\unicode[STIX]{x1D706}\cos 2\unicode[STIX]{x1D70B}(\unicode[STIX]{x1D703}+n\unicode[STIX]{x1D6FC})u(n)$ be the almost Mathieu operator on 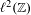$\ell ^{2}(\mathbb{Z})$, where $\unicode[STIX]{x1D706},\unicode[STIX]{x1D703}\in \mathbb{R}$. Avila and Jitomirskaya [The ten Martini problem. Ann. of Math. (2), 170(1) (2009), 303–342] conjectured that, for $2\unicode[STIX]{x1D703}\in \unicode[STIX]{x1D6FC}\mathbb{Z}+\mathbb{Z}$, 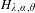$H_{\unicode[STIX]{x1D706},\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}$ satisfies Anderson localization if 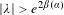$|\unicode[STIX]{x1D706}|>e^{2\unicode[STIX]{x1D6FD}(\unicode[STIX]{x1D6FC})}$. In this paper, we develop a method to treat simultaneous frequency and phase resonances and obtain that, for 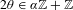$2\unicode[STIX]{x1D703}\in \unicode[STIX]{x1D6FC}\mathbb{Z}+\mathbb{Z}$, $H_{\unicode[STIX]{x1D706},\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}$ satisfies Anderson localization if $|\unicode[STIX]{x1D706}|>e^{3\unicode[STIX]{x1D6FD}(\unicode[STIX]{x1D6FC})}$.

$\mathbb{Z}^{d}$-extensions of probability-preserving dynamical systems are themselves dynamical systems preserving an infinite measure, and generalize random walks. Using the method of moments, we prove a generalized central limit theorem for additive functionals of the extension of integral zero, under spectral assumptions. As a corollary, we get the fact that Green–Kubo’s formula is invariant under induction. This allows us to relate the hitting probability of sites with the symmetrized potential kernel, giving an alternative proof and generalizing a theorem of Spitzer. Finally, this relation is used to improve, in turn, the assumptions of the generalized central limit theorem. Applications to Lorentz gases in finite horizon and to the geodesic flow on Abelian covers of compact manifolds of negative curvature are discussed.

Let $\unicode[STIX]{x1D719}$ be a post-critically finite branched covering of a two-sphere. By work of Koch, the Thurston pullback map induced by $\unicode[STIX]{x1D719}$ on Teichmüller space descends to a multivalued self-map—a Hurwitz correspondence ${\mathcal{H}}_{\unicode[STIX]{x1D719}}$—of the moduli space ${\mathcal{M}}_{0,\mathbf{P}}$. We study the dynamics of Hurwitz correspondences via numerical invariants called dynamical degrees. We show that the sequence of dynamical degrees of ${\mathcal{H}}_{\unicode[STIX]{x1D719}}$ is always non-increasing and that the behavior of this sequence is constrained by the behavior of $\unicode[STIX]{x1D719}$ at and near points of its post-critical set.

We generalize the higher rank rigidity theorem to a class of Finsler spaces, i.e. Berwald spaces. More precisely, we prove that a complete connected Berwald space of finite volume and bounded non-positive flag curvature with rank at least two whose universal cover is irreducible is a locally symmetric space or a locally Minkowski space.