As discovered by W. Thurston, the action of a complex one-variable polynomial on its Julia set can be modeled by a geodesic lamination in the disk, provided that the Julia set is connected. It also turned out that the parameter space of such dynamical laminations of degree two gives a model for the bifurcation locus in the space of quadratic polynomials. This model is itself a geodesic lamination, the so called quadratic minor lamination of Thurston. In the same spirit, we consider the space of all cubic symmetric polynomials $f_\unicode{x3bb} (z)=z^3+\unicode{x3bb} ^2 z$ in three articles. In the first one, we construct the cubic symmetric comajor lamination together with the corresponding quotient space of the unit circle. As is verified in the third paper, this yields a monotone model of the cubic symmetric connectedness locus, that is, the space of all cubic symmetric polynomials with connected Julia sets. In the present paper, the second in the series, we develop an algorithm for generating the cubic symmetric comajor lamination analogous to the Lavaurs algorithm for constructing the quadratic minor lamination.

In this paper, we prove the assertion that the number of monic cubic polynomials $F(x) = x^3 + a_2 x^2 + a_1 x + a_0$ with integer coefficients and irreducible, Galois over ${\mathbb {Q}}$ satisfying $\max \{|a_2|, |a_1|, |a_0|\} \leq X$ is bounded from above by $O(X (\log X)^2)$. We also count the number of abelian monic binary cubic forms with integer coefficients up to a natural equivalence relation ordered by the so-called Bhargava–Shankar height. Finally, we prove an assertion characterizing the splitting field of 2-torsion points of semi-stable abelian elliptic curves.

We consider cubic polynomials 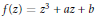$f\left( z \right)\,=\,{{z}^{3}}\,+\,az\,+\,b$ defined over $\mathbb{C}\left( \lambda \right)$, with a marked point of period 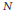$N$ and multiplier 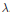$\lambda$. In the case 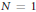$N\,=\,1$, there are infinitely many such objects, and in the case 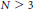$N\,\ge \,3$, only finitely many (subject to a mild assumption). The case 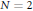$N\,=\,2$ has particularly rich structure, and we are able to describe all such cubic polynomials defined over the field ${{\cup }_{n\ge 1}}\,\mathbb{C}\left( {{\lambda }^{1/n}} \right)$.

This paper explores single-equation nonlinear error correction (NEC) models with linear and nonlinear cointegrated variables. Within the class of semiparametric NEC models, we use smoothing splines. Within the class of parametric models, we discuss the interesting properties of cubic polynomial NEC models and we show how they can be used to identify unknown threshold points in asymmetric models and to check the stability properties of the long-run equilibrium. A new class of rational polynomial NEC models is also introduced. We found multiple long-run money demand equilibria. The stability observed in the money-demand parameter estimates during more than a century, 1878 to 2000, is remarkable.

In this paper, the realization of biped walking on even floor, sloping surfaces, and stairs is studied. The motion trajectories for the biped's body and its two feet are modeled by piecewise cubic polynomials. The main features of the biped robot include two variable-length legs and a moving weight which can be positioned side-to-side for balance. The contribution of this paper is gait synthesis and is experimental verification.