We establish the restricted sumset analog of the celebrated conjecture of Sárközy on additive decompositions of the set of nonzero squares over a finite field. More precisely, we show that if $q>13$ is an odd prime power, then the set of nonzero squares in $\mathbb {F}_q$ cannot be written as a restricted sumset $A \hat {+} A$, extending a result of Shkredov. More generally, we study restricted sumsets in multiplicative subgroups over finite fields as well as restricted sumsets in perfect powers (over integers) motivated by a question of Erdős and Moser. We also prove an analog of van Lint–MacWilliams’ conjecture for restricted sumsets, which appears to be the first analogue of Erdős--Ko–Rado theorem in a family of Cayley sum graphs.

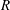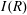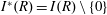Let $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}R$ be a commutative ring, $I(R)$ be the set of all ideals of $R$ and $S$ be a subset of $I^*(R)=I(R)\setminus \{0\}$. We define a Cayley sum digraph of ideals of 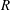$R$, denoted by 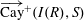$\overrightarrow{\mathrm{Cay}}^+ (I(R),S)$, as a directed graph whose vertex set is the set 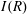$I(R)$ and, for every two distinct vertices 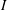$I$ and 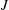$J$, there is an arc from $I$ to 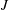$J$, denoted by $I\longrightarrow J$, whenever 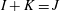$I+K=J$, for some ideal 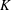$K $ in $S$. Also, the Cayley sum graph $ \mathrm{Cay}^+ (I(R), S)$ is an undirected graph whose vertex set is the set 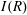$I(R)$ and two distinct vertices 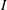$I$ and 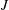$J$ are adjacent whenever 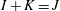$I+K=J$ or 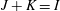$J+K=I$, for some ideal 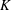$K $ in 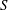$ S$. In this paper, we study some basic properties of the graphs $\overrightarrow{\mathrm{Cay}}^+ (I(R),S)$ and 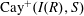$ \mathrm{Cay}^+ (I(R), S)$ such as connectivity, girth and clique number. Moreover, we investigate the planarity, outerplanarity and ring graph of 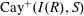$ \mathrm{Cay}^+ (I(R), S)$ and also we provide some characterization for rings 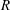$R$ whose Cayley sum graphs have genus one.