For a partially multiplicative quandle (PMQ) ${\mathcal {Q}}$ we consider the topological monoid $\mathring {\mathrm {HM}}({\mathcal {Q}})$ of Hurwitz spaces of configurations in the plane with local monodromies in ${\mathcal {Q}}$. We compute the group completion of $\mathring {\mathrm {HM}}({\mathcal {Q}})$: it is the product of the (discrete) enveloping group ${\mathcal {G}}({\mathcal {Q}})$ with a component of the double loop space of the relative Hurwitz space $\mathrm {Hur}_+([0,1]^2,\partial [0,1]^2;{\mathcal {Q}},G)_{\mathbb {1}}$; here $G$ is any group giving rise, together with ${\mathcal {Q}}$, to a PMQ–group pair. Under the additional assumption that ${\mathcal {Q}}$ is finite and rationally Poincaré and that $G$ is finite, we compute the rational cohomology ring of $\mathrm {Hur}_+([0,1]^2,\partial [0,1]^2;{\mathcal {Q}},G)_{\mathbb {1}}$.

We prove that the classical map comparing Adams’ cobar construction on the singular chains of a pointed space and the singular cubical chains on its based loop space is a quasi-isomorphism preserving explicitly defined monoidal $E_\infty $-coalgebra structures. This contribution extends to its ultimate conclusion a result of Baues, stating that Adams’ map preserves monoidal coalgebra structures.

We exhibit the Hodge degeneration from nonabelian Hodge theory as a $2$-fold delooping of the filtered loop space $E_2$-groupoid in formal moduli problems. This is an iterated groupoid object which in degree $1$ recovers the filtered circle $S^1_{fil}$ of [MRT22]. This exploits a hitherto unstudied additional piece of structure on the topological circle, that of an $E_2$-cogroupoid object in the $\infty $-category of spaces. We relate this cogroupoid structure with the more commonly studied ‘pinch map’ on $S^1$, as well as the Todd class of the Lie algebroid $\mathbb {T}_{X}$; this is an invariant of a smooth and proper scheme X that arises, for example, in the Grothendieck-Riemann-Roch theorem. In particular, we relate the existence of nontrivial Todd classes for schemes to the failure of the pinch map to be formal in the sense of rational homotopy theory. Finally, we record some consequences of this bit of structure at the level of Hochschild cohomology.

We show that under mild conditions, the connected sum $M\# N$ of simply connected, closed, orientable n-dimensional Poincaré Duality complexes M and N is hyperbolic and has no homotopy exponent at all but finitely many primes, verifying a weak version of Moore’s conjecture. This is derived from an elementary framework involving $CW$-complexes satisfying certain conditions.

Reflexive homology is the homology theory associated to the reflexive crossed simplicial group; one of the fundamental crossed simplicial groups. It is the most general way to extend Hochschild homology to detect an order-reversing involution. In this paper we study the relationship between reflexive homology and the $C_2$-equivariant homology of free loop spaces. We define reflexive homology in terms of functor homology. We give a bicomplex for computing reflexive homology together with some calculations, including the reflexive homology of a tensor algebra. We prove that the reflexive homology of a group algebra is isomorphic to the homology of the $C_2$-equivariant Borel construction on the free loop space of the classifying space. We give a direct sum decomposition of the reflexive homology of a group algebra indexed by conjugacy classes of group elements, where the summands are defined in terms of a reflexive analogue of group homology. We define a hyperhomology version of reflexive homology and use it to study the $C_2$-equivariant homology of certain free loop and free loop-suspension spaces. We show that reflexive homology satisfies Morita invariance. We prove that under nice conditions the involutive Hochschild homology studied by Braun and by Fernàndez-València and Giansiracusa coincides with reflexive homology.

We provide a simple condition on rational cohomology for the total space of a pullback fibration over a connected sum to have the rational homotopy type of a connected sum after looping. This takes inspiration from a recent work of Jeffrey and Selick, in which they study pullback fibrations of this type but under stronger hypotheses compared to our result.

Ganea proved that the loop space of $\mathbb{C} P^n$ is homotopy commutative if and only if $n=3$ . We generalize this result to that the loop spaces of all irreducible Hermitian symmetric spaces but $\mathbb{C} P^3$ are not homotopy commutative. The computation also applies to determining the homotopy nilpotency class of the loop spaces of generalized flag manifolds $G/T$ for a maximal torus T of a compact, connected Lie group G.

Let $G$ be a finite group with cyclic Sylow $p$-subgroups, and let $k$ be a field of characteristic $p$. Then $H^{*}(BG;k)$ and $H_*(\Omega BG{{}^{{}^{\wedge }}_p};k)$ are $A_\infty$ algebras whose structure we determine up to quasi-isomorphism.

For the $p$-localized sphere $\mathbb {S}^{2m-1}_{(p)}$ with $p >3$ a prime, we prove that the homotopy nilpotency satisfies $\mbox {nil}\ \mathbb {S}^{2m-1}_{(p)}<\infty$, with respect to any homotopy associative $H$-structure on $\mathbb {S}^{2m-1}_{(p)}$. We also prove that $\mbox {nil}\ \mathbb {S}^{2m-1}_{(p)}= 1$ for all but a finite number of primes $p >3$. Then, for the loop space of the associated $\mathbb {S}^{2m-1}_{(p)}$-projective space $\mathbb {S}^{2m-1}_{(p)}P(n-1)$, with $m,n\ge 2$ and $m\mid p-1$, we derive that $\mbox {nil}\ \Omega (\mathbb {S}^{2m-1}_{(p)}P (n-1))\le 3$.

Greenlees has conjectured that the rational stable equivariant homotopy category of a compact Lie group always has an algebraic model. Based on this idea, we show that the category of rational local systems on a connected finite loop space always has a simple algebraic model. When the loop space arises from a connected compact Lie group, this recovers a special case of a result of Pol and Williamson about rational cofree G-spectra. More generally, we show that if K is a closed subgroup of a compact Lie group G such that the Weyl group WGK is connected, then a certain category of rational G-spectra “at K” has an algebraic model. For example, when K is the trivial group, this is just the category of rational cofree G-spectra, and this recovers the aforementioned result. Throughout, we pay careful attention to the role of torsion and complete categories.

Let S2n+1{p} denote the homotopy fibre of the degree p self map of S2n+1. For primes p ≥ 5, work by Selick shows that S2n+1{p} admits a non-trivial loop space decomposition if and only if n = 1 or p. Indecomposability in all but these dimensions was obtained by showing that a non-trivial decomposition of ΩS2n+1{p} implies the existence of a p-primary Kervaire invariant one element of order p in $\pi _{2n(p-1)-2}^S$. We prove the converse of this last implication and observe that the homotopy decomposition problem for ΩS2n+1{p} is equivalent to the strong p-primary Kervaire invariant problem for all odd primes. For p = 3, we use the 3-primary Kervaire invariant element θ3 to give a new decomposition of ΩS55{3} analogous to Selick's decomposition of ΩS2p+1{p} and as an application prove two new cases of a long-standing conjecture stating that the fibre of the double suspension $S^{2n-1} \longrightarrow \Omega ^2S^{2n+1}$ is homotopy equivalent to the double loop space of Anick's space.

For certain pairs of Lie groups (G, H) and primes p, Harris showed a relation of the p-localized homotopy groups of G and H. This is reinterpreted as a p-local homotopy equivalence G ≃ (p)H × G/H, and so there is a projection G(p) → H(p). We show how much this projection preserves the higher homotopy associativity.

We introduce a notion of Koszul A∞-algebra that generalizes Priddy's notion of a Koszul algebra and we use it to construct small A∞-algebra models for Hochschild cochains. As an application, this yields new techniques for computing free loop space homology algebras of manifolds that are either formal or coformal (over a field or over the integers). We illustrate these techniques in two examples.

In this paper we introduce new local symbols, which we call 4-function local symbols. We formulate reciprocity laws for them. These reciprocity laws are proven using a new method - multidimensional iterated integrals. Besides providing reciprocity laws for the new 4-function local symbols, the same method works for proving reciprocity laws for the Parshin symbol. Both the new 4-function local symbols and the Parshin symbol can be expressed as a finite product of newly defined bi-local symbols, each of which satisfies a reciprocity law. The K-theoretic variant of the first 4-function local symbol is defined in the Appendix. It differs by a sign from the one defined via iterated integrals. Both the sign and the K-theoretic variant of the 4-function local symbol satisfy reciprocity laws, whose proof is based on Milnor K-theory (see the Appendix). The relation of the 4-function local symbols to the double free loop space of the surface is given by iterated integrals over membranes.

Let $X$ be an $n$-dimensional, finite, simply connected $\text{CW}$ complex and set

$${{\alpha }_{X}}\,=\,\underset{i}{\mathop{\lim \,\sup }}\,\frac{\log \,\text{rank}\,{{\pi }_{i}}\left( X \right)}{i}$$

When $0<{{\alpha }_{X}}<\infty $, we give upper and lower bounds for $\sum\nolimits_{i=k+2}^{k+n}{\,\text{rank}}\text{ }{{\pi }_{i}}\left( X \right)$ for $k$ sufficiently large. We also show for any $r$ that $\alpha x$ can be estimated from the integers $\text{rk }{{\pi }_{i}}\left( X \right),\,i\,\le \,nr$ with an error bound depending explicitly on $r$.

The concept of ${{C}_{k}}$-spaces is introduced, situated at an intermediate stage between 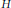$H$-spaces and $T$-spaces. The ${{C}_{k}}$-space corresponds to the $k$-th Milnor–Stasheff filtration on spaces. It is proved that a space 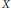$X$ is a ${{C}_{k}}$-space if and only if the Gottlieb set 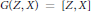$G(Z,\,X)\,=\,[Z,\,X]$ for any space 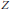$Z$ with cat 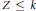$Z\,\le \,k$, which generalizes the fact that 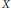$X$ is a $T$-space if and only if $G(\sum B,\,X)\,=\,[\sum B,\,X]$ for any space $B$. Some results on the ${{C}_{k}}$-space are generalized to the 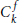$C_{k}^{f}$-space for a map $f\,:\,A\,\to \,X$. Projective spaces, lens spaces and spaces with a few cells are studied as examples of ${{C}_{k}}$-spaces, and non-${{C}_{k}}$-spaces.

The fiber ${{W}_{n}}$ of the double suspension ${{S}^{2n-1}}\,\to \,{{\Omega }^{2}}{{S}^{2n+1}}$ is known to have a classifying space $B{{W}_{n}}$. An important conjecture linking the $EPH$ sequence to the homotopy theory of Moore spaces is that $B{{W}_{n}}\,\simeq \,\Omega {{T}^{2np+1}}(p)$, where ${{T}^{2np+1}}(p)$ is Anick's space. This is known if $n\,=\,1$. We prove the $n\,=\,p$ case and establish some related properties.

The usual constructions of classifying spaces for monoidal categories produce $\text{CW}$-complexes with many cells that,moreover, do not have any proper geometric meaning. However, geometric nerves of monoidal categories are very handy simplicial sets whose simplices have a pleasing geometric description: they are diagrams with the shape of the 2-skeleton of oriented standard simplices. The purpose of this paper is to prove that geometric realizations of geometric nerves are classifying spaces for monoidal categories.

Let $\Omega X$ be the space of Moore loops on a finite, $q$-connected, $n$-dimensional CW complex $X$, and let $R\subset\Q$ be a subring containing 1/2. Let $\rho\left(R\right)$ be the least non-invertible prime in $R$. For a graded $R$-module $M$ of finite type, let $FM = M / {\rm Torsion}\,M$. We show that the inclusion $P \subset FH_{*}\left(\Omega X;R\right)$ of the sub-Lie algebra of primitive elements induces an isomorphism of Hopf algebras $$UP \overset{\cong}{\longrightarrow} FH_{*}\left(\Omega X;R\right)},$$ provided that $\rho\left(R\right) \geqslant n/q$. Furthermore, the Hurewicz homomorphism induces an embedding of $F(\pi_{*}\left(\Omega X\right)\otimes R)$ in $P$, with $P/F(\pi_{*}\left(\Omega X\right)\otimes R)$ torsion. As a corollary, if $X$ is elliptic, then $FH_{*}\left(\Omega X;R\right)$ is a finitely generated $R$-algebra.

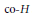James gave an integral homotopy decomposition of $\sum \Omega \sum X$, Hilton-Milnor one for $\Omega (\sum X\,\vee \,\sum Y)$, and Cohen-Wu gave $p$-local decompositions of $\Omega \sum X$ if $X$ is a suspension. All are natural. Using idempotents and telescopes we show that the James and Hilton-Milnor decompositions have analogues when the suspensions are replaced by coassociative $\text{co-}H$ spaces, and the Cohen-Wu decomposition has an analogue when the (double) suspension is replaced by a coassociative, cocommutative $\text{co-}H$ space.