Let H be a real Hilbert space and $\Phi :H\to H$ be a $C^1$ operator with Lipschitzian derivative and closed range. We prove that $\Phi ^{-1}(0)\neq \emptyset $ if and only if, for each $\epsilon>0$, there exist a convex set $X\subset H$ and a convex function $\psi :X\to \mathbf {R}$ such that $\sup _{x\in X}(\|x\|^2+\psi (x))-\inf _{x\in X}(\|x\|^2+\psi (x))<\epsilon $ and $0\in \overline {{\mathrm {conv}}}(\Phi (X))$.

We generalize a classical extension result by Seeley in the context of Bastiani’s differential calculus to infinite dimensions. The construction follows Seeley’s original approach, but is significantly more involved as not only $C^k$ -maps (for ) on (subsets of) half spaces are extended, but also continuous extensions of their differentials to some given piece of boundary of the domains under consideration. A further feature of the generalization is that we construct families of extension operators (instead of only one single extension operator) that fulfill certain compatibility (and continuity) conditions. Various applications are discussed as well.

We discuss an alternative approach to Fréchet derivatives on Banach spaces inspired by a characterisation of derivatives due to Carathéodory. The approach allows many questions of differentiability to be reduced to questions of continuity. We demonstrate how that simplifies the theory of differentiation, including the rules of differentiation and the Schwarz lemma on the symmetry of second-order derivatives. We also provide a short proof of the differentiable dependence of fixed points in the Banach fixed point theorem.

We give precise conditions under which the composition of a norm with a convex function yields a uniformly convex function on a Banach space. Various applications are given to functions of power type. The results are dualized to study uniform smoothness and several examples are provided.

We study classes of mappings between finite and infinite dimensional Banach spaces that are monotone and mappings which are differences of monotone mappings ($\text{DM}$). We prove a Radó–Reichelderfer estimate for monotone mappings in finite dimensional spaces that remains valid for $\text{DM}$ mappings. This provides an alternative proof of the Fréchet differentiability a.e. of $\text{DM}$ mappings. We establish a Morrey-type estimate for the distributional derivative of monotone mappings. We prove that a locally $\text{DM}$ mapping between finite dimensional spaces is also globally $\text{DM}$. We introduce and study a new class of the so-called $\text{UDM}$ mappings between Banach spaces, which generalizes the concept of curves of finite variation.

A characterization of Banach spaces possessing the weak Radon–Nikodým property is given in terms of finitely additive interval functions. Due to that characterization several Banach space valued set functions that are only finitely additive can be represented as integrals.

We prove that for every function $f\,:\,X\,\to \,Y$ , where $X$ is a separable Banach space and $Y$ is a Banach space with RNP, there exists a set 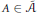$A\,\in \,\overset{\sim }{\mathop{\mathcal{A}}}\,$ such that 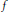$f$ is Gâteaux differentiable at all 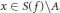$x\,\in \,S\left( f \right)\backslash A$, where $S\left( f \right)$ is the set of points where 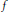$f$ is pointwise-Lipschitz. This improves a result of Bongiorno. As a corollary, we obtain that every 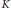$K$-monotone function on a separable Banach space is Hadamard differentiable outside of a set belonging to $\tilde{C}\,;$ this improves a result due to Borwein and Wang. Another corollary is that if $X$ is Asplund, $f\,:\,X\,\to \,\mathbb{R}$ cone monotone, $g\,:\,X\,\to \,\mathbb{R}$ continuous convex, then there exists a point in $X$, where 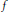$f$ is Hadamard differentiable and $g$ is Fréchet differentiable.

Given a free ideal J of subsets of a set X, we consider games where player ONE plays an increasing sequence of elements of the σ-completion of J, and player TWO tries to cover the union of this sequence by playing one set at a time from J. We describe various conditions under which player TWO has a winning strategy that uses only information about the most recent k moves of ONE, and apply some of these results to the Banach-Mazurgame.

We study the relationships between Gateaux, Fréchet and weak Hadamard differentiability of convex functions and of equivalent norms. As a consequence we provide related characterizations of infinite dimensional Banach spaces and of Banach spaces containing ł1. Explicit examples are given. Some renormings of WCG Asplund spaces are made in this vein.

We generalize the generic single-valuedness and continuity of monotone operators defined on open subsets of Banach spaces of class (S) and Asplund spaces to monotone operators defined on convex subsets of such spaces which may even fail to have non-support points. This yields differentiability theorems for convex Lipschitzian functions on such sets. From a result about minimal convex uscos which are densely single-valued we obtain generic differentiability results for certain Lipschitzian realvalued functions.