A classical theorem of Hermite and Joubert asserts that any field extension of degree  $n\,=\,5\,\text{or}\,\text{6}$ is generated by an element whose minimal polynomial is of the form
$n\,=\,5\,\text{or}\,\text{6}$ is generated by an element whose minimal polynomial is of the form
 ${{\lambda }^{n}}\,+\,{{c}_{1}}{{\lambda }^{n-1}}\,+\,\cdot \cdot \cdot +\,{{c}_{n-1}}\lambda \,+\,{{c}_{n}}$
with
${{\lambda }^{n}}\,+\,{{c}_{1}}{{\lambda }^{n-1}}\,+\,\cdot \cdot \cdot +\,{{c}_{n-1}}\lambda \,+\,{{c}_{n}}$
with 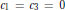 ${{c}_{1\,}}\,=\,\,{{c}_{3}}\,=\,0$. We show that this theorem fails for
${{c}_{1\,}}\,=\,\,{{c}_{3}}\,=\,0$. We show that this theorem fails for
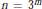 $n\,=\,{{3}^{m}}$ or
$n\,=\,{{3}^{m}}$ or 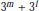 ${{3}^{m}}+{{3}^{l}}$ (and more generally, for
${{3}^{m}}+{{3}^{l}}$ (and more generally, for
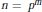 $n={{p}^{m}}$ or
$n={{p}^{m}}$ or 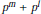 ${{p}^{m}}+{{p}^{l}}$, if 3 is replaced by another prime
${{p}^{m}}+{{p}^{l}}$, if 3 is replaced by another prime  $p$), where
$p$), where  $m\,>\,1\,\ge \,0$. We also prove a similar result for division algebras and use it to study the structure of the universal division algebra
$m\,>\,1\,\ge \,0$. We also prove a similar result for division algebras and use it to study the structure of the universal division algebra 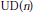 $\text{UD}\left( n \right)$.
$\text{UD}\left( n \right)$.
We also prove a similar result for division algebras and use it to study the structure of the universal division algebra 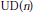 $\text{UD}\left( n \right)$.
$\text{UD}\left( n \right)$.
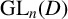 $\mathrm {GL}_n(D)$
$\mathrm {GL}_n(D)$
 $p$-rank
$p$-rank
 $p$-Extensions
$p$-Extensions