Let  $X,\,Y$ be reduced and irreducible compact complex spaces and
$X,\,Y$ be reduced and irreducible compact complex spaces and 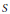 $S$ the set of all isomorphism classes of reduced and irreducible compact complex spaces
$S$ the set of all isomorphism classes of reduced and irreducible compact complex spaces 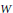 $W$ such that
$W$ such that 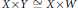 $X\,\times \,Y\,\cong \,X\,\times \,W$. Here we prove that
$X\,\times \,Y\,\cong \,X\,\times \,W$. Here we prove that 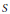 $S$ is at most countable. We apply this result to show that for every reduced and irreducible compact complex space
$S$ is at most countable. We apply this result to show that for every reduced and irreducible compact complex space 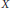 $X$ the set
$X$ the set  $S(X)$ of all complex reduced compact complex spaces
$S(X)$ of all complex reduced compact complex spaces 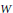 $W$ with
$W$ with  $X\,\times \,{{X}^{\sigma }}\,\cong \,W\,\times \,{{W}^{\sigma }}$ (where
$X\,\times \,{{X}^{\sigma }}\,\cong \,W\,\times \,{{W}^{\sigma }}$ (where 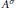 ${{A}^{\sigma }}$ denotes the complex conjugate of any variety
${{A}^{\sigma }}$ denotes the complex conjugate of any variety  $A$) is at most countable.
$A$) is at most countable.
 $p>0$
$p>0$