We give the generating function of split $(n+t)$-colour partitions and obtain an analogue of Euler’s identity for split $n$-colour partitions. We derive a combinatorial relation between the number of restricted split $n$-colour partitions and the function $\unicode[STIX]{x1D70E}_{k}(\unicode[STIX]{x1D707})=\sum _{d|\unicode[STIX]{x1D707}}d^{k}$. We introduce a new class of split perfect partitions with $d(a)$ copies of each part $a$ and extend the work of Agarwal and Subbarao [‘Some properties of perfect partitions’, Indian J. Pure Appl. Math 22(9) (1991), 737–743].

We prove two conjectural congruences on the $(p-1)$th Apéry number, which were recently proposed by Z.-H. Sun.

Let $n$ be a positive integer. We obtain new Menon’s identities by using the actions of some subgroups of $(\mathbb{Z}/n\mathbb{Z})^{\times }$ on the set $\mathbb{Z}/n\mathbb{Z}$. In particular, let $p$ be an odd prime and let $\unicode[STIX]{x1D6FC}$ be a positive integer. If $H_{k}$ is a subgroup of $(\mathbb{Z}/p^{\unicode[STIX]{x1D6FC}}\mathbb{Z})^{\times }$ with index $k=p^{\unicode[STIX]{x1D6FD}}u$ such that $0\leqslant \unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D6FC}$ and $u\mid p-1$, then

$$\begin{eqnarray}\mathop{\sum }_{x\in H_{k}}(x-1,p^{\unicode[STIX]{x1D6FC}})=\frac{\unicode[STIX]{x1D711}(p^{\unicode[STIX]{x1D6FC}})}{k}\bigg(1+k(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})+u\frac{p^{\unicode[STIX]{x1D6FD}}-1}{p-1}\bigg),\end{eqnarray}$$

$\unicode[STIX]{x1D711}(n)$

One of the open questions in the study of Carmichael numbers is whether, for a given $R\geq 3$, there exist infinitely many Carmichael numbers with exactly $R$ prime factors. Chernick [‘On Fermat’s simple theorem’, Bull. Amer. Math. Soc.45 (1935), 269–274] proved that Dickson’s $k$-tuple conjecture would imply a positive result for all such $R$. Wright [‘Factors of Carmichael numbers and a weak $k$-tuples conjecture’, J. Aust. Math. Soc.100(3) (2016), 421–429] showed that a weakened version of Dickson’s conjecture would imply that there are an infinitude of $R$ for which there are infinitely many such Carmichael numbers. In this paper, we improve on our 2016 result by weakening the required conjecture even further.

Let $\mathbb{N}$ be the set of all nonnegative integers. For any set $A\subset \mathbb{N}$, let $R(A,n)$ denote the number of representations of $n$ as $n=a+a^{\prime }$ with $a,a^{\prime }\in A$. There is no partition $\mathbb{N}=A\cup B$ such that $R(A,n)=R(B,n)$ for all sufficiently large integers $n$. We prove that a partition $\mathbb{N}=A\cup B$ satisfies $|R(A,n)-R(B,n)|\leq 1$ for all nonnegative integers $n$ if and only if, for each nonnegative integer $m$, exactly one of $2m+1$ and $2m$ is in $A$.

We improve some previously known deterministic algorithms for finding integer solutions $x,y$ to the exponential equation of the form $af^{x}+bg^{y}=c$ over finite fields.

In this article, we prove the transcendence of certain infinite sums and products by applying the subspace theorem. In particular, we extend the results of Hančl and Rucki [‘The transcendence of certain infinite series’, Rocky Mountain J. Math.35 (2005), 531–537].

We characterise finite unitary rings $R$ such that all Sylow subgroups of the group of units $R^{\ast }$ are cyclic. To be precise, we show that, up to isomorphism, $R$ is one of the three types of rings in $\{O,E,O\oplus E\}$, where $O\in \{GF(q),\mathbb{Z}_{p^{\unicode[STIX]{x1D6FC}}}\}$ is a ring of odd cardinality and $E$ is a ring of cardinality $2^{n}$ which is one of seven explicitly described types.

Let 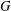$G$ be a finite group and 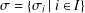$\unicode[STIX]{x1D70E}=\{\unicode[STIX]{x1D70E}_{i}\mid i\in I\}$ some partition of the set of all primes 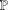$\mathbb{P}$, that is, 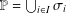$\mathbb{P}=\bigcup _{i\in I}\unicode[STIX]{x1D70E}_{i}$ and 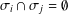$\unicode[STIX]{x1D70E}_{i}\cap \unicode[STIX]{x1D70E}_{j}=\emptyset$ for all 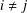$i\neq j$. We say that 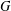$G$ is $\unicode[STIX]{x1D70E}$-primary if 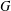$G$ is a 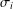$\unicode[STIX]{x1D70E}_{i}$-group for some 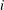$i$. 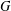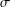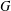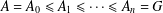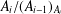A subgroup $A$ of $G$ is said to be: $\unicode[STIX]{x1D70E}$-subnormal in$G$ if there is a subgroup chain $A=A_{0}\leq A_{1}\leq \cdots \leq A_{n}=G$ such that either $A_{i-1}\unlhd A_{i}$ or $A_{i}/(A_{i-1})_{A_{i}}$ is 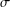$\unicode[STIX]{x1D70E}$-primary for all $i=1,\ldots ,n$; modular in$G$ if the following conditions hold: (i) $\langle X,A\cap Z\rangle =\langle X,A\rangle \cap Z$ for all 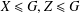$X\leq G,Z\leq G$ such that 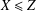$X\leq Z$ and (ii) $\langle A,Y\cap Z\rangle =\langle A,Y\rangle \cap Z$ for all 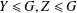$Y\leq G,Z\leq G$ such that 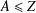$A\leq Z$; and 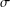$\unicode[STIX]{x1D70E}$-quasinormal in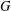$G$ if $A$ is modular and $\unicode[STIX]{x1D70E}$-subnormal in $G$. We study 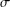$\unicode[STIX]{x1D70E}$-quasinormal subgroups of $G$. In particular, we prove that if a subgroup 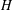$H$ of 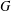$G$ is $\unicode[STIX]{x1D70E}$-quasinormal in 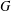$G$, then every chief factor 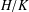$H/K$ of $G$ between $H^{G}$ and $H_{G}$ is $\unicode[STIX]{x1D70E}$-central in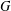$G$, that is, the semidirect product 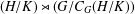$(H/K)\rtimes (G/C_{G}(H/K))$ is $\unicode[STIX]{x1D70E}$-primary.

Dorff et al. [‘Convolutions of harmonic convex mappings’, Complex Var. Elliptic Equ. 57(5) (2012), 489–503] formulated a question concerning the convolution of two right half-plane mappings, where the normalisation of the functions was considered incorrectly. In this paper, we reformulate the problem correctly and provide a solution to it in a more general form. We also obtain two new theorems which correct and improve related results.

We demonstrate the global existence of weak solutions to a class of semilinear strongly damped wave equations possessing nonlinear hyperbolic dynamic boundary conditions. The associated linear operator is $(-\unicode[STIX]{x1D6E5}_{W})^{\unicode[STIX]{x1D703}}\unicode[STIX]{x2202}_{t}u$, where $\unicode[STIX]{x1D703}\in [\frac{1}{2},1)$ and $\unicode[STIX]{x1D6E5}_{W}$ is the Wentzell–Laplacian. A balance condition is assumed to hold between the nonlinearity defined on the interior of the domain and the nonlinearity on the boundary. This allows for arbitrary (supercritical) polynomial growth of each potential, as well as mixed dissipative/antidissipative behaviour.

We consider a convex Lagrangian $L:\mathit{TM}\rightarrow \mathbb{R}$ quadratic at infinity with $L(x,0)=0$ for every $x\in M$ and such that the 1-form $\unicode[STIX]{x1D703}$ defined by $\unicode[STIX]{x1D703}_{x}(v)=L_{v}(x,0)v$ is not closed. We show that for every number $a<0$, there is a contractible (nonconstant) periodic orbit with action $a$. We also obtain estimates of the period and energy of such periodic orbits.

We investigate a dichotomy property for Hardy–Littlewood maximal operators, noncentred $M$ and centred $M^{c}$, that was noticed by Bennett et al. [‘Weak-$L^{\infty }$ and BMO’, Ann. of Math. (2) 113 (1981), 601–611]. We illustrate the full spectrum of possible cases related to the occurrence or not of this property for $M$ and $M^{c}$ in the context of nondoubling metric measure spaces $(X,\unicode[STIX]{x1D70C},\unicode[STIX]{x1D707})$. In addition, if $X=\mathbb{R}^{d}$, $d\geq 1$, and $\unicode[STIX]{x1D70C}$ is the metric induced by an arbitrary norm on $\mathbb{R}^{d}$, then we give the exact characterisation (in terms of $\unicode[STIX]{x1D707}$) of situations in which $M^{c}$ possesses the dichotomy property provided that $\unicode[STIX]{x1D707}$ satisfies some very mild assumptions.

We show that if $(X,\Vert \cdot \Vert )$ is a Banach space that admits an equivalent locally uniformly rotund norm and the set of all norm-attaining functionals is residual then the dual norm $\Vert \cdot \Vert ^{\ast }$ on $X^{\ast }$ is Fréchet at the points of a dense subset of $X^{\ast }$. This answers the main open problem in a paper by Guirao, Montesinos and Zizler [‘Remarks on the set of norm-attaining functionals and differentiability’, Studia Math.241 (2018), 71–86].

Let $u$ and $\unicode[STIX]{x1D711}$ be two analytic functions on the unit disc $D$ such that $\unicode[STIX]{x1D711}(D)\subset D$. A weighted composition operator $uC_{\unicode[STIX]{x1D711}}$ induced by $u$ and $\unicode[STIX]{x1D711}$ is defined by $uC_{\unicode[STIX]{x1D711}}f:=u\cdot f\circ \unicode[STIX]{x1D711}$ for every $f$ in $H^{p}$, the Hardy space of $D$. We investigate compactness of $uC_{\unicode[STIX]{x1D711}}$ on $H^{p}$ in terms of function-theoretic properties of $u$ and $\unicode[STIX]{x1D711}$.

We study linear mappings which preserve vectors at a specific angle. We introduce the concept of $(\unicode[STIX]{x1D700},c)$-angle preserving mappings and define $\widehat{\unicode[STIX]{x1D700}}\,(T,c)$ as the ‘smallest’ number $\unicode[STIX]{x1D700}$ for which $T$ is an $(\unicode[STIX]{x1D700},c)$-angle preserving mapping. We derive an exact formula for $\widehat{\unicode[STIX]{x1D700}}\,(T,c)$ in terms of the norm $\Vert T\Vert$ and the minimum modulus $[T]$ of $T$. Finally, we characterise approximately angle preserving mappings.

We study best proximity points in the framework of metric spaces with $w$-distances. The results extend, generalise and unify several well-known fixed point results in the literature.

An embedding of a metric graph $(G,d)$ on a closed hyperbolic surface is essential if each complementary region has a negative Euler characteristic. We show, by construction, that given any metric graph, its metric can be rescaled so that it admits an essential and isometric embedding on a closed hyperbolic surface. The essential genus $g_{e}(G)$ of $(G,d)$ is the lowest genus of a surface on which such an embedding is possible. We establish a formula to compute $g_{e}(G)$ and show that, for every integer $g\geq g_{e}(G)$, there is an embedding of $(G,d)$ (possibly after a rescaling of $d$) on a surface of genus $g$. Next, we study minimal embeddings where each complementary region has Euler characteristic $-1$. The maximum essential genus $g_{e}^{\max }(G)$ of $(G,d)$ is the largest genus of a surface on which the graph is minimally embedded. We describe a method for an essential embedding of $(G,d)$, where $g_{e}(G)$ and $g_{e}^{\max }(G)$ are realised.