1. Introduction
Perturbations existing on a material interface grow after being accelerated by an incident shock wave or by an external force directed from the heavy fluid to the light fluid, which are known as Richtmyer–Meshkov (RM) instability (Richtmyer Reference Richtmyer1960; Meshkov Reference Meshkov1969) and Rayleigh–Taylor (RT) instability (Rayleigh Reference Rayleigh1882; Taylor Reference Taylor1950), respectively. The pressure gradient caused by the shock wave or the external force does not coincide with the density gradient, which produces a baroclinic source term depositing vorticity around the material interface. The interface is thus rolled up, forming bubbles where the light fluid penetrates into the heavy fluid, and spikes where the heavy fluid penetrates into the light fluid. At late time, the nonlinear effect causes the interfacial instability to transition into turbulent mixing. The evolution of mixing width (![]() $W$), defined as the distance from the bubble front to the spike front, has been investigated extensively, which is reviewed systematically in a series of papers (Brouillette Reference Brouillette2002; Zhou Reference Zhou2017a,Reference Zhoub; Zhai et al. Reference Zhai, Zou, Wu and Luo2018; Zhou et al. Reference Zhou2021).
$W$), defined as the distance from the bubble front to the spike front, has been investigated extensively, which is reviewed systematically in a series of papers (Brouillette Reference Brouillette2002; Zhou Reference Zhou2017a,Reference Zhoub; Zhai et al. Reference Zhai, Zou, Wu and Luo2018; Zhou et al. Reference Zhou2021).
The classical RM instability, which occurs at a planar interface, has been investigated widely. However, in many engineering applications, such as inertial confinement fusion (Thomas & Kares Reference Thomas and Kares2012; Betti & Hurricane Reference Betti and Hurricane2016), the interface is always a collapsing cylinder or sphere. These interface configurations are referred to collectively as convergent geometries. Built on the understanding of planar RM instability, researches have been carried out on convergent RM instability. Early-time linear (Bell Reference Bell1951; Plesset Reference Plesset1954) and weakly nonlinear (Wang et al. Reference Wang, Wu, Guo, Ye, Liu, Zhang and He2015; Zhang et al. Reference Zhang, Wang, Wu, Ye, Zou, Ding, Zhang and He2020) growth of the single-mode perturbation in the convergent geometry are described mathematically. These models have been confirmed by a series of experiments (Ding et al. Reference Ding, Si, Yang, Lu, Zhai and Luo2017; Luo et al. Reference Luo, Zhang, Ding, Si, Yang, Zhai and Wen2018b, Reference Luo, Li, Ding, Zhai and Si2019). Theoretical analyses were extended in recent studies to predict the growth of perturbations in the case of multiple shocks (Flaig et al. Reference Flaig, Clark, Weber, Youngs and Thornber2018), nonlinear stages (Goncharov & Li Reference Goncharov and Li2005; Zhao et al. Reference Zhao, Wang, Liu and Lu2020; Dimonte Reference Dimonte2021), or late-time turbulent mixing (Mikaelian Reference Mikaelian1990, Reference Mikaelian2005; Rafei et al. Reference El Rafei, Flaig, Youngs and Thornber2019; El Rafei & Thornber Reference El Rafei and Thornber2020). Besides, numerical simulations have been carried out to investigate the convergent RM instability. Joggerst et al. (Reference Joggerst, Nelson, Woodward, Lovekin, Masser, Fryer, Ramaprabhu, Francois and Rockefeller2014) presented two-dimensional spherical and cylindrical implosion cases with different grids, and showed that the results obtained from different grids converge under high resolution. Lombardini, Pullin & Meiron (Reference Lombardini, Pullin and Meiron2014a,Reference Lombardini, Pullin and Meironb) simulated numerically the RM instability with multi-mode perturbations in spherical geometry, and divided the growth of the mixing width into three parts based on a linear theory: RT-like contribution, geometric convergence, and compression effects. A series of cylindrical implosion experiments were carried out (Hsing & Hoffman Reference Hsing and Hoffman1997; Hsing et al. Reference Hsing, Barnes, Beck, Hoffman, Galmiche, Richard, Edwards, Graham, Rothman and Thomas1997; Tubbs et al. Reference Tubbs, Barnes, Beck, Hoffman, Oertel, Watt, Boehly, Bradley, Jaanimagi and Knauer1999; Barnes et al. Reference Barnes2002; Lanier et al. Reference Lanier, Barnes, Batha, Day, Magelssen, Scott, Dunne, Parker and Rothman2003). In the experiments, the smooth premixing layer is observed to be thickened during convergence (Lanier et al. Reference Lanier, Barnes, Batha, Day, Magelssen, Scott, Dunne, Parker and Rothman2003), which has not been understood clearly.
One key problem is how to evaluate the difference between the growth of perturbations in convergent geometries and that in the planar geometry. Bell (Reference Bell1951) and Plesset (Reference Plesset1954) extended the single-mode linear theory to the cylindrical and spherical geometry, respectively, which are summarized by Epstein (Reference Epstein2004) as
and
for planar, cylindrical and spherical geometries, respectively. Here, ![]() $\gamma _{R}=-\dot {R}/R$ is the convergence rate of the interface, and
$\gamma _{R}=-\dot {R}/R$ is the convergence rate of the interface, and ![]() $\gamma _{\rho }=-\dot {\rho }/\rho$ is the compression rate of the fluids. The overdot represents the time derivative. Also,
$\gamma _{\rho }=-\dot {\rho }/\rho$ is the compression rate of the fluids. The overdot represents the time derivative. Also, ![]() $a$ is the perturbation amplitude, and
$a$ is the perturbation amplitude, and ![]() $\gamma _{0}$ is the growth rate, where
$\gamma _{0}$ is the growth rate, where
for the planar geometry,
for the cylindrical geometry, and
for the spherical geometry. Here, ![]() $\rho _{h}$ and
$\rho _{h}$ and ![]() $\rho _{l}$ are the densities of heavy fluid and light fluid,
$\rho _{l}$ are the densities of heavy fluid and light fluid, ![]() $L$ is the length of the cross-section in the planar geometry, which is a constant,
$L$ is the length of the cross-section in the planar geometry, which is a constant, ![]() $R$ is the interface radius, which varies with time,
$R$ is the interface radius, which varies with time, ![]() $m$ is the wavenumber of the perturbations, and
$m$ is the wavenumber of the perturbations, and ![]() $g$ is the acceleration of the interface. Compared with the planar cases, the linear theory in convergent geometry is characterized with
$g$ is the acceleration of the interface. Compared with the planar cases, the linear theory in convergent geometry is characterized with ![]() $R$, which varies with time, and
$R$, which varies with time, and ![]() $\dot {\rho }$, which represents the variation of density with time. In the weakly nonlinear model deduced by Wang et al. (Reference Wang, Wu, Guo, Ye, Liu, Zhang and He2015) and Zhang et al. (Reference Zhang, Wang, Wu, Ye, Zou, Ding, Zhang and He2020), geometrical convergence is also verified as an important factor to modify the growth of perturbation. The perturbation growth is found to be amplified in the convergent geometries, which is described as a function of the convergence ratio
$\dot {\rho }$, which represents the variation of density with time. In the weakly nonlinear model deduced by Wang et al. (Reference Wang, Wu, Guo, Ye, Liu, Zhang and He2015) and Zhang et al. (Reference Zhang, Wang, Wu, Ye, Zou, Ding, Zhang and He2020), geometrical convergence is also verified as an important factor to modify the growth of perturbation. The perturbation growth is found to be amplified in the convergent geometries, which is described as a function of the convergence ratio ![]() $C_{r}=R_{0}/R(t)$. For example, by assuming a self-similar growth of perturbation, Mikaelian (Reference Mikaelian1990, Reference Mikaelian2005) extended the linear theory to predict the growth of the turbulent-mixing width in both cylindrical and spherical geometries, where the convergence ratio
$C_{r}=R_{0}/R(t)$. For example, by assuming a self-similar growth of perturbation, Mikaelian (Reference Mikaelian1990, Reference Mikaelian2005) extended the linear theory to predict the growth of the turbulent-mixing width in both cylindrical and spherical geometries, where the convergence ratio ![]() $C_{r}$ also amplifies the growth of the mixing width. Therefore, compared to that in the planar geometry, the perturbation growth in the convergent geometry is modified. This modification caused by the geometry is referred to collectively as the Bell–Plesset (BP) effect (Beck Reference Beck1996; Hsing & Hoffman Reference Hsing and Hoffman1997), which is a function of
$C_{r}$ also amplifies the growth of the mixing width. Therefore, compared to that in the planar geometry, the perturbation growth in the convergent geometry is modified. This modification caused by the geometry is referred to collectively as the Bell–Plesset (BP) effect (Beck Reference Beck1996; Hsing & Hoffman Reference Hsing and Hoffman1997), which is a function of ![]() $R$ and
$R$ and ![]() $\rho$. As suggested in the inertial confinement fusion experiments by Li et al. (Reference Li2004), the BP effect is expected to become important at a much higher convergence ratio
$\rho$. As suggested in the inertial confinement fusion experiments by Li et al. (Reference Li2004), the BP effect is expected to become important at a much higher convergence ratio ![]() $C_{r}>30$.
$C_{r}>30$.
The BP effect is caused mainly by geometrical convergence and fluid compression. However, the relative importance and coupling of the two factors are unclear. On the one hand, by assuming a constant mass in the mixing zone with constant density, the stretching of the mixing width ![]() $W$ (the radial length) is deduced since the azimuthal length of the mixing zone decreases as the interface converges (Luo et al. Reference Luo, Ding, Zhai and Si2018a). On the other hand, supposing that the fluids are compressed uniformly in space, the coupling effect of geometrical convergence and fluid compression turns out to inhibit the growth of perturbations (Epstein Reference Epstein2004), which leads to a different conclusion. More recently, Ge et al. (Reference Ge, Zhang, Li and Tian2020) carried out three-dimensional simulations of cylindrical RM instability. As a result, a stretching effect exists obviously in cylindrical geometry. The stretching effect is defined as the averaged velocity difference between two ends of the mixing zone (Li et al. Reference Li, Tian, He and Zhang2021), which does not exist in planar geometry if there is no wave acting on the mixing zone. Ge et al. (Reference Ge, Zhang, Li and Tian2020) ascribe this stretching effect in cylindrical geometry to ‘an asymmetric geometric environment’. However, there is no detailed explanation of this asymmetric geometric environment. Furthermore, the relation between this stretching effect and the BP effect is still unclear. Therefore, to better understand the BP effect, it is worthwhile to give the exact quantitative contribution of geometrical convergence and fluid compression in the modification caused by the convergent geometry. Besides, an intuitive physical explanation of this modification on the perturbation growth in convergent geometries is also needed, which motivates the present work.
$W$ (the radial length) is deduced since the azimuthal length of the mixing zone decreases as the interface converges (Luo et al. Reference Luo, Ding, Zhai and Si2018a). On the other hand, supposing that the fluids are compressed uniformly in space, the coupling effect of geometrical convergence and fluid compression turns out to inhibit the growth of perturbations (Epstein Reference Epstein2004), which leads to a different conclusion. More recently, Ge et al. (Reference Ge, Zhang, Li and Tian2020) carried out three-dimensional simulations of cylindrical RM instability. As a result, a stretching effect exists obviously in cylindrical geometry. The stretching effect is defined as the averaged velocity difference between two ends of the mixing zone (Li et al. Reference Li, Tian, He and Zhang2021), which does not exist in planar geometry if there is no wave acting on the mixing zone. Ge et al. (Reference Ge, Zhang, Li and Tian2020) ascribe this stretching effect in cylindrical geometry to ‘an asymmetric geometric environment’. However, there is no detailed explanation of this asymmetric geometric environment. Furthermore, the relation between this stretching effect and the BP effect is still unclear. Therefore, to better understand the BP effect, it is worthwhile to give the exact quantitative contribution of geometrical convergence and fluid compression in the modification caused by the convergent geometry. Besides, an intuitive physical explanation of this modification on the perturbation growth in convergent geometries is also needed, which motivates the present work.
In this work, we extract a compression or stretching (S(C)) effect from the perturbation growth. We evaluate quantitatively the S(C) effect, and prove this effect to be an important part of the BP effect. The physical origin underlying the S(C) effect is also given. A series of numerical simulations in planar and cylindrical geometries are performed to verify our theoretical analysis.
The layout of this paper is as follows. The theoretical analysis is presented in § 2, where the S(C) effect is introduced and analysed. In § 3, the physical model and numerical method for numerical simulations are presented. The numerical results can be found in § 4, followed by the discussion about the S(C) effect in § 5. The conclusions are drawn in § 6. The reliability of the numerical simulations is discussed in Appendices A and B.
2. Theoretical analysis on the S(C) effect
2.1. Definition of the S(C) effect
The definition of the S(C) effect is given by applying a decomposition formula. The formula was proposed to investigate the influence of nonlinear waves (Li et al. Reference Li, Tian, He and Zhang2021) and convergent geometry (Ge et al. Reference Ge, Zhang, Li and Tian2020) on the evolution of RM instability. For completeness, we give a brief derivation of the S(C) effect.
The mixing width is defined as the distance from the bubble-zone front to the spike-zone front, i.e.
and hence
where the overdot means the time derivative, ![]() $A=(\rho _{out}-\rho _{in})/(\rho _{out}+\rho _{in})$ is the Atwood number,
$A=(\rho _{out}-\rho _{in})/(\rho _{out}+\rho _{in})$ is the Atwood number, ![]() $R_{B}$ is the radius where the Favre-averaged light-fluid mass fraction profile in the streamwise direction
$R_{B}$ is the radius where the Favre-averaged light-fluid mass fraction profile in the streamwise direction ![]() $\tilde {Y}_{l}(R_{B},t)=0.01$, and
$\tilde {Y}_{l}(R_{B},t)=0.01$, and ![]() $R_{S}$ is the radius where
$R_{S}$ is the radius where ![]() $\tilde {Y}_{l}(R_{S},t)=0.99$.
$\tilde {Y}_{l}(R_{S},t)=0.99$.
For a physical variable ![]() $f$,
$f$, ![]() $\bar {f}$ and
$\bar {f}$ and ![]() $\tilde {f}$ represent the Reynolds-averaged streamwise-direction profile and the Favre-averaged streamwise-direction profile, respectively. Here,
$\tilde {f}$ represent the Reynolds-averaged streamwise-direction profile and the Favre-averaged streamwise-direction profile, respectively. Here, ![]() $\tilde {f}=\bar {\rho f}/\bar {\rho }$, where
$\tilde {f}=\bar {\rho f}/\bar {\rho }$, where ![]() $\rho$ is the fluid density. Correspondingly,
$\rho$ is the fluid density. Correspondingly, ![]() $f^{\prime }=f-\bar {f}$ and
$f^{\prime }=f-\bar {f}$ and ![]() $f^{\prime \prime }=f-\tilde {f}$ are the fluctuating parts of the variable. The averaged velocity is aligned in the streamwise direction. For consistency, in the planar geometry, we use
$f^{\prime \prime }=f-\tilde {f}$ are the fluctuating parts of the variable. The averaged velocity is aligned in the streamwise direction. For consistency, in the planar geometry, we use ![]() $r$ to indicate the streamwise direction.
$r$ to indicate the streamwise direction.
For the Favre-averaged mass fraction profile ![]() $\tilde {Y}(r,t)$, where
$\tilde {Y}(r,t)$, where ![]() $r$ is the streamwise direction, at any given time
$r$ is the streamwise direction, at any given time ![]() $t$, the function of space
$t$, the function of space ![]() $\tilde {Y}(r,t)$ is monotonic when
$\tilde {Y}(r,t)$ is monotonic when ![]() $\tilde {Y}\in ( 0,1)$. Therefore, the inverse function of
$\tilde {Y}\in ( 0,1)$. Therefore, the inverse function of ![]() $\tilde {Y}(r,t)$ can be defined as
$\tilde {Y}(r,t)$ can be defined as ![]() $R_{\tilde {Y}}(Y,t)=\tilde {Y}^{-1}(r,t)$ (
$R_{\tilde {Y}}(Y,t)=\tilde {Y}^{-1}(r,t)$ (![]() $Y\in ( 0,1)$), where
$Y\in ( 0,1)$), where ![]() $R_{\tilde {Y}}(Y,t)$ is the radius with an averaged mass fraction
$R_{\tilde {Y}}(Y,t)$ is the radius with an averaged mass fraction ![]() $Y$ at time
$Y$ at time ![]() $t$. The time derivative of the radius with a certain mass fraction
$t$. The time derivative of the radius with a certain mass fraction ![]() $Y_{0}$ is formulated as
$Y_{0}$ is formulated as
For ![]() $r_{0}=R_{\tilde {Y}}(Y_{0},t+\Delta t)$, it is obvious that at time
$r_{0}=R_{\tilde {Y}}(Y_{0},t+\Delta t)$, it is obvious that at time ![]() $t$, the radius
$t$, the radius ![]() $r_{0}$ corresponds to another value of mass fraction
$r_{0}$ corresponds to another value of mass fraction ![]() $Y_{0}+\Delta Y$, such that
$Y_{0}+\Delta Y$, such that
Substituting (2.3) into (2.2), we have
 \begin{equation} \left.\frac{\partial R_{\tilde{Y}}}{\partial t}\right|_{Y_{0}}= \lim_{\Delta t \to 0}\frac{R_{\tilde{Y}}(Y_{0}+\Delta Y,t)-R_{\tilde{Y}}(Y_{0},t)}{\Delta Y}\,\frac{\Delta Y}{\Delta t}=\left.-\frac{\partial R_{\tilde{Y}}}{\partial Y}\,\frac{\partial \tilde{Y}}{\partial t}\right|_{Y_{0}}. \end{equation}
\begin{equation} \left.\frac{\partial R_{\tilde{Y}}}{\partial t}\right|_{Y_{0}}= \lim_{\Delta t \to 0}\frac{R_{\tilde{Y}}(Y_{0}+\Delta Y,t)-R_{\tilde{Y}}(Y_{0},t)}{\Delta Y}\,\frac{\Delta Y}{\Delta t}=\left.-\frac{\partial R_{\tilde{Y}}}{\partial Y}\,\frac{\partial \tilde{Y}}{\partial t}\right|_{Y_{0}}. \end{equation}
The property of the inverse function indicates that ![]() $\partial R_{\tilde {Y}}/\partial Y=(\partial \tilde {Y}/\partial r)^{-1}$. Therefore, the velocity of the radius with a certain mass fraction
$\partial R_{\tilde {Y}}/\partial Y=(\partial \tilde {Y}/\partial r)^{-1}$. Therefore, the velocity of the radius with a certain mass fraction ![]() $Y_{0}$ can be expressed as
$Y_{0}$ can be expressed as
 \begin{equation} \dot{R}|_{Y_{0}}={-}\left.\frac{\partial \tilde{Y}/\partial t}{\partial \tilde{Y}/\partial r}\right|_{Y_{0}}. \end{equation}
\begin{equation} \dot{R}|_{Y_{0}}={-}\left.\frac{\partial \tilde{Y}/\partial t}{\partial \tilde{Y}/\partial r}\right|_{Y_{0}}. \end{equation}The governing equation of the mass fraction is
where ![]() $D$ is the diffusion coefficient. The Reynolds-averaged equation (2.6) is
$D$ is the diffusion coefficient. The Reynolds-averaged equation (2.6) is
We now consider the averaged mass equation
Subtracting ![]() $\tilde {Y}$ times (2.8) from (2.7), we obtain
$\tilde {Y}$ times (2.8) from (2.7), we obtain
The spatial gradient in the streamwise direction has the same form for the Cartesian, cylindrical and spherical coordinates. Therefore, we use ![]() $\boldsymbol {\nabla }f=\boldsymbol {e_{r}}\,\partial f/\partial r$, where
$\boldsymbol {\nabla }f=\boldsymbol {e_{r}}\,\partial f/\partial r$, where ![]() $\boldsymbol {e_{r}}$ is the unit vector in the streamwise direction, so
$\boldsymbol {e_{r}}$ is the unit vector in the streamwise direction, so ![]() $(\tilde {\boldsymbol {u}}\boldsymbol {\cdot }\boldsymbol {\nabla })f=\tilde {u}\,\partial f/\partial r$. Dividing (2.9) by
$(\tilde {\boldsymbol {u}}\boldsymbol {\cdot }\boldsymbol {\nabla })f=\tilde {u}\,\partial f/\partial r$. Dividing (2.9) by ![]() $\partial \tilde {Y}/\partial r$ and using
$\partial \tilde {Y}/\partial r$ and using ![]() $\tilde {u}=\bar {u}+\overline {\rho ^{\prime }u^{\prime }}/\bar {\rho }$, we obtain
$\tilde {u}=\bar {u}+\overline {\rho ^{\prime }u^{\prime }}/\bar {\rho }$, we obtain
 \begin{align} -\frac{\partial \tilde{Y}/\partial t}{\partial \tilde{Y}/\partial r}=\bar{u}+\frac{1}{\bar{\rho}}\left(\overline{\rho^{\prime}u^{\prime}}+ \frac{\boldsymbol{\nabla}\boldsymbol{\cdot}\overline{\rho Y^{\prime\prime}\boldsymbol{u}^{\prime\prime}}}{\partial \tilde{Y}/\partial r}\right)-\frac{1}{\bar{\rho}\,\partial\tilde{Y}/\partial r}\,\boldsymbol{\nabla}\boldsymbol{\cdot}\left(\bar{\rho}D\,\frac{\partial \tilde{Y}}{\partial r}+\overline{\rho D\,\frac{\partial Y^{\prime\prime}}{\partial r}}\right)\boldsymbol{e}_{\boldsymbol{r}}. \end{align}
\begin{align} -\frac{\partial \tilde{Y}/\partial t}{\partial \tilde{Y}/\partial r}=\bar{u}+\frac{1}{\bar{\rho}}\left(\overline{\rho^{\prime}u^{\prime}}+ \frac{\boldsymbol{\nabla}\boldsymbol{\cdot}\overline{\rho Y^{\prime\prime}\boldsymbol{u}^{\prime\prime}}}{\partial \tilde{Y}/\partial r}\right)-\frac{1}{\bar{\rho}\,\partial\tilde{Y}/\partial r}\,\boldsymbol{\nabla}\boldsymbol{\cdot}\left(\bar{\rho}D\,\frac{\partial \tilde{Y}}{\partial r}+\overline{\rho D\,\frac{\partial Y^{\prime\prime}}{\partial r}}\right)\boldsymbol{e}_{\boldsymbol{r}}. \end{align}By combining (2.5) and (2.10), we obtain
where
 $$\begin{gather}u_{{Diff}}={-}\frac{1}{\bar{\rho}\,\partial\tilde{Y}/\partial r}\,\frac{\partial}{\partial r}\left(\bar{\rho}D\,\frac{\partial \tilde{Y}}{\partial r}+\overline{\rho D\,\frac{\partial Y^{\prime\prime}}{\partial r}}\right)+u_{{Diff,G}}. \end{gather}$$
$$\begin{gather}u_{{Diff}}={-}\frac{1}{\bar{\rho}\,\partial\tilde{Y}/\partial r}\,\frac{\partial}{\partial r}\left(\bar{\rho}D\,\frac{\partial \tilde{Y}}{\partial r}+\overline{\rho D\,\frac{\partial Y^{\prime\prime}}{\partial r}}\right)+u_{{Diff,G}}. \end{gather}$$
Here, ![]() $\bar {u}|_{Y_{0}}$ is the Reynolds-averaged velocity at radius
$\bar {u}|_{Y_{0}}$ is the Reynolds-averaged velocity at radius ![]() $r$ with
$r$ with ![]() $\tilde {Y}_{l}(r)=Y_0$;
$\tilde {Y}_{l}(r)=Y_0$; ![]() $u_{Pen}$ describes the contribution of the fluctuation field,
$u_{Pen}$ describes the contribution of the fluctuation field, ![]() $u_{{Diff}}$ represents the contribution of the molecular diffusion, and
$u_{{Diff}}$ represents the contribution of the molecular diffusion, and ![]() $u_{{Pen,G}}$ and
$u_{{Pen,G}}$ and ![]() $u_{{Diff,G}}$ are the terms caused by the spatial divergence in non-Cartesian coordinates, where
$u_{{Diff,G}}$ are the terms caused by the spatial divergence in non-Cartesian coordinates, where
 $$\begin{gather}u_{{Diff,G}}={-}\frac{\alpha}{\bar{\rho}\,\partial \tilde{Y}/\partial r}\,\frac{1}{r}\left(\bar{\rho}D\,\frac{\partial \tilde{Y}}{\partial r}+\overline{\rho D\,\frac{\partial Y^{\prime\prime}}{\partial r}}\right), \end{gather}$$
$$\begin{gather}u_{{Diff,G}}={-}\frac{\alpha}{\bar{\rho}\,\partial \tilde{Y}/\partial r}\,\frac{1}{r}\left(\bar{\rho}D\,\frac{\partial \tilde{Y}}{\partial r}+\overline{\rho D\,\frac{\partial Y^{\prime\prime}}{\partial r}}\right), \end{gather}$$
where ![]() $\alpha$ is the geometry coefficient, with
$\alpha$ is the geometry coefficient, with ![]() $\alpha =0,1,2$ for the Cartesian, cylindrical and spherical coordinates, respectively.
$\alpha =0,1,2$ for the Cartesian, cylindrical and spherical coordinates, respectively.
At two ends of the mixing zone, the term related to the fluctuation terms, i.e. ![]() $u_{{Pen,G}}$, is negligible compared with the terms related to the spatial gradient of the fluctuation terms (the validation can be found in figures 8 and 9). Combining (2.1) and (2.11), the growth rate of the mixing width is decomposed into
$u_{{Pen,G}}$, is negligible compared with the terms related to the spatial gradient of the fluctuation terms (the validation can be found in figures 8 and 9). Combining (2.1) and (2.11), the growth rate of the mixing width is decomposed into
which is referred to as the decomposition formula. The formula indicates that the growth of the mixing width contains three parts.
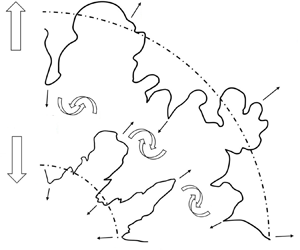
(i) The stretching or compression (S(C)) effect defined as the mean-velocity difference between two edges of the mixing zone, which is presented as the large arrows in figure 1. When this term is not zero, the mixing zone is stretched or compressed.
(ii) The penetration effect defined by the fluctuating field. This effect is illustrated in figure 1 with small arrows. During the growth of the mixing zone,
 $\overline {\rho Y^{\prime \prime }u^{\prime \prime }}$ and
$\overline {\rho Y^{\prime \prime }u^{\prime \prime }}$ and  $\overline {\rho ^{\prime }u^{\prime }}$ are dominated by two important processes: the light fluid penetrates the heavy fluid, and the heavy fluid penetrates the light fluid (Li et al. Reference Li, Tian, He and Zhang2021).
$\overline {\rho ^{\prime }u^{\prime }}$ are dominated by two important processes: the light fluid penetrates the heavy fluid, and the heavy fluid penetrates the light fluid (Li et al. Reference Li, Tian, He and Zhang2021).(iii) The diffusion effect caused by molecular diffusion, which tends to decrease the density gradient at the material interface.

Figure 1. Schematic diagram of the growth of the mixing width in convergent geometry. The large arrows represent the mean velocity calculated at the bubble front and spike front. The small arrows represent the fluctuating velocity of the bubble and spike.
According to the work of Li et al. (Reference Li, Tian, He and Zhang2021), the contribution of the diffusion effect is negligible in the flows at a high Reynolds number. Therefore, in the present work, we consider only the penetration effect and the S(C) effect.
The decomposition formula provides a method to analyse quantitatively the mechanisms controlling the evolution of the mixing width in interfacial fluid mixing. In the planar geometry, the perturbation growth caused by RT/RM instability is attributed to the penetration effect in most cases, while the S(C) effect, which is a growth mechanism independent of RM/RT growth, is significant only when the mixing zone is influenced by wave systems, such as shock, rarefaction and compression waves (Li et al. Reference Li, Tian, He and Zhang2021). However, in the convergent geometry, the S(C) effect is evident even when there is no wave in the mixing zone (Ge et al. Reference Ge, Zhang, Li and Tian2020). Therefore, the S(C) effect is an important difference between the interfacial mixing in planar geometry and that in convergent geometry.
2.2. Quantitative analysis of the S(C) effect
In this subsection, we consider the S(C) effect in planar, cylindrical and spherical geometries. For the fluids inside and outside the interface, the compression rates are assumed to be uniform around the interface, i.e.
The works of Bell (Reference Bell1951) and Epstein (Reference Epstein2004) are inherited in the present work, where the fluid motion is assumed to be irrotational. Therefore, there exist velocity potentials ![]() $\varPhi _{in/out}$ that satisfy a Poisson-type equation,
$\varPhi _{in/out}$ that satisfy a Poisson-type equation, ![]() $\Delta \varPhi _{in/out}=-\gamma _{\rho,in/out}$. The initial perturbation is formulated as a single mode with a small amplitude. These results give the velocity potentials inside and outside the interface expressed as
$\Delta \varPhi _{in/out}=-\gamma _{\rho,in/out}$. The initial perturbation is formulated as a single mode with a small amplitude. These results give the velocity potentials inside and outside the interface expressed as
in Cartesian coordinates ![]() $(r,y)$,
$(r,y)$,
in cylindrical coordinates ![]() $(r,\theta )$, and
$(r,\theta )$, and
in spherical coordinates ![]() $(r,\theta,\varphi )$. For the planar and cylindrical geometries, we disregard the
$(r,\theta,\varphi )$. For the planar and cylindrical geometries, we disregard the ![]() $z$-dependent direction. The potential functions
$z$-dependent direction. The potential functions ![]() $\varPsi _{in/out}(r)$ describe the background flow inside and outside the interface in all geometries, which are represented respectively as
$\varPsi _{in/out}(r)$ describe the background flow inside and outside the interface in all geometries, which are represented respectively as
in the planar geometry,
in the cylindrical geometry, and
in the spherical geometry. For consistency, we use ![]() $R$ to indicate the position of the interface throughout. Here,
$R$ to indicate the position of the interface throughout. Here, ![]() $\psi _{in/out}$ represents the potential of the perturbed flow, which satisfies the Laplace function
$\psi _{in/out}$ represents the potential of the perturbed flow, which satisfies the Laplace function ![]() $\Delta \psi _{in/out}=0$ and thus is formulated as
$\Delta \psi _{in/out}=0$ and thus is formulated as
in the planar geometry (where ![]() $m$ is the wavenumber of the perturbation, and
$m$ is the wavenumber of the perturbation, and ![]() $L$ is the length scale in the spanwise direction
$L$ is the length scale in the spanwise direction ![]() $y$),
$y$),
in the cylindrical geometry, and
in the spherical geometry, where ![]() $m-n$ and
$m-n$ and ![]() $n$ are the latitude and longitude wavenumbers, respectively. Also,
$n$ are the latitude and longitude wavenumbers, respectively. Also, ![]() $A_{in/out}(t)$ is the function determined by the boundary condition and is a function of only time. The perturbed interface equations are
$A_{in/out}(t)$ is the function determined by the boundary condition and is a function of only time. The perturbed interface equations are
in the planar geometry,
in the cylindrical geometry, and
in the spherical geometry, where ![]() $a(t)$ is the amplitude of the perturbation. Therefore, the bubble front
$a(t)$ is the amplitude of the perturbation. Therefore, the bubble front ![]() $R_{B}$ and spike front
$R_{B}$ and spike front ![]() $R_{S}$ of the mixing zone are described as
$R_{S}$ of the mixing zone are described as ![]() $R-a$ and
$R-a$ and ![]() $R+a$, respectively. The Reynolds-averaged velocities at the bubble front and spike front in different geometries are calculated as
$R+a$, respectively. The Reynolds-averaged velocities at the bubble front and spike front in different geometries are calculated as
 \begin{equation} \bar{u}_{B/S}=\left.-\int_{0}^{L}\frac{\partial\varPhi_{in/out}}{\partial r}(R_{B/S},y)\,\mathrm{d} y\right/\int_{0}^{L}\mathrm{d} y \end{equation}
\begin{equation} \bar{u}_{B/S}=\left.-\int_{0}^{L}\frac{\partial\varPhi_{in/out}}{\partial r}(R_{B/S},y)\,\mathrm{d} y\right/\int_{0}^{L}\mathrm{d} y \end{equation}in the planar geometry,
in the cylindrical geometry, and
in the spherical geometry. As a result, we have
in the planar geometry,
in the cylindrical geometry, and
in the spherical geometry. Substituting (2.21) into (2.14) and considering that ![]() $a\ll R$, one obtains that the S(C) effect is formulated as
$a\ll R$, one obtains that the S(C) effect is formulated as
where ![]() $\gamma _{\rho }=(\gamma _{\rho,out}+\gamma _{\rho,in})/2$, the averaged compression rate, and
$\gamma _{\rho }=(\gamma _{\rho,out}+\gamma _{\rho,in})/2$, the averaged compression rate, and ![]() $W=2a$ denotes the mixing width. The geometrical coefficient is
$W=2a$ denotes the mixing width. The geometrical coefficient is ![]() $\alpha =0,1,2$ for the planar, cylindrical and spherical geometries, and
$\alpha =0,1,2$ for the planar, cylindrical and spherical geometries, and ![]() $\dot {W}_{S(C)}>0$ means that the mixing zone is stretched, while
$\dot {W}_{S(C)}>0$ means that the mixing zone is stretched, while ![]() $\dot {W}_{S(C)}<0$ means that the mixing width is compressed.
$\dot {W}_{S(C)}<0$ means that the mixing width is compressed.
The interface convergence gives ![]() $\gamma _{R}>0$, and fluid compression gives
$\gamma _{R}>0$, and fluid compression gives ![]() $\gamma _{\rho }<0$. Therefore, the geometrical convergence leads to the stretching of the mixing zone, while fluid compression leads to the compression of the mixing zone. The coupling of geometrical convergence and fluid compression is realized by the linear superposition. Furthermore, in cylindrical geometry
$\gamma _{\rho }<0$. Therefore, the geometrical convergence leads to the stretching of the mixing zone, while fluid compression leads to the compression of the mixing zone. The coupling of geometrical convergence and fluid compression is realized by the linear superposition. Furthermore, in cylindrical geometry ![]() $\alpha =1$, while in spherical geometry
$\alpha =1$, while in spherical geometry ![]() $\alpha =2$. Therefore, the contribution from the geometrical convergence is more significant in the spherical geometry.
$\alpha =2$. Therefore, the contribution from the geometrical convergence is more significant in the spherical geometry.
2.3. Physical origin of the S(C) effect
According to (2.22), the S(C) effect is determined by three physical quantities: ![]() $\gamma _{R}$,
$\gamma _{R}$, ![]() $\gamma _{\rho }$ and
$\gamma _{\rho }$ and ![]() $W$. As will be shown, the underlying physical origin of (2.22) is that the mass in the mixing zone is conserved except for the mass brought in by the penetration effect.
$W$. As will be shown, the underlying physical origin of (2.22) is that the mass in the mixing zone is conserved except for the mass brought in by the penetration effect.
As shown in figure 2, the mass in the mixing zone is ![]() $\rho V_{t}$ at time
$\rho V_{t}$ at time ![]() $t$, and
$t$, and ![]() $(\rho +\Delta \rho )V_{t+\Delta t}$ at time
$(\rho +\Delta \rho )V_{t+\Delta t}$ at time ![]() $t+\Delta t$. Here,
$t+\Delta t$. Here, ![]() $V_{t}$ denotes the volume of the mixing zone at time
$V_{t}$ denotes the volume of the mixing zone at time ![]() $t$. In this demonstration,
$t$. In this demonstration, ![]() $\rho$ is assumed to be uniform in the mixing zone. For the cylindrical geometry, we have
$\rho$ is assumed to be uniform in the mixing zone. For the cylindrical geometry, we have ![]() $V_{t}={\rm \pi} [(R+W/2)^{2}-(R-W/2)^{2}]=2{\rm \pi} WR$, where the axial length is unity. For the spherical geometry, we have
$V_{t}={\rm \pi} [(R+W/2)^{2}-(R-W/2)^{2}]=2{\rm \pi} WR$, where the axial length is unity. For the spherical geometry, we have ![]() $V_{t}=({4{\rm \pi} }/{3})[(R+W/2)^{3}-(R-W/2)^{3}]=4{\rm \pi} WR^{2}(1+{W}/{12R})$. By assuming that the mass in the mixing zone is conserved, we have
$V_{t}=({4{\rm \pi} }/{3})[(R+W/2)^{3}-(R-W/2)^{3}]=4{\rm \pi} WR^{2}(1+{W}/{12R})$. By assuming that the mass in the mixing zone is conserved, we have ![]() $\rho V_{t}=(\rho +\Delta \rho )V_{t+\Delta t}$, i.e.
$\rho V_{t}=(\rho +\Delta \rho )V_{t+\Delta t}$, i.e.
in the cylindrical geometry, and
in the spherical geometry. In most cases, the radial length of the mixing zone is much smaller than the radius. Therefore, ![]() $W/12R\ll 1$. By disregarding small quantities of any order higher than the first, we obtain
$W/12R\ll 1$. By disregarding small quantities of any order higher than the first, we obtain
where ![]() $\alpha =1,2$ for the cylindrical geometry and spherical geometry, respectively. Dividing (2.24) by
$\alpha =1,2$ for the cylindrical geometry and spherical geometry, respectively. Dividing (2.24) by ![]() $\Delta t$ and applying
$\Delta t$ and applying ![]() $\Delta t\to 0$, we have
$\Delta t\to 0$, we have ![]() $\dot {W}_{S(C)}=(\alpha \gamma _{R}+\gamma _{\rho })W$, which is the same as (2.22). Therefore, we verify that the S(C) effect originates from the idea that the mass in the mixing zone is conserved if we do not consider the mass brought in by the penetration effect. When the mixing zone undergoes fluid compression or interface convergence, the density or the spanwise length of the mixing zone changes, and the mixing width changes as well.
$\dot {W}_{S(C)}=(\alpha \gamma _{R}+\gamma _{\rho })W$, which is the same as (2.22). Therefore, we verify that the S(C) effect originates from the idea that the mass in the mixing zone is conserved if we do not consider the mass brought in by the penetration effect. When the mixing zone undergoes fluid compression or interface convergence, the density or the spanwise length of the mixing zone changes, and the mixing width changes as well.
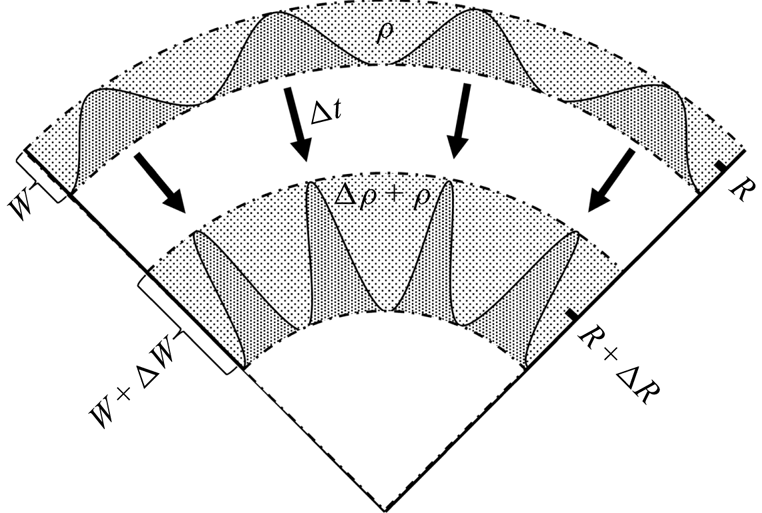
Figure 2. Schematic diagram of the S(C) effect. At time ![]() $t$, the mixing zone is positioned in the radius
$t$, the mixing zone is positioned in the radius ![]() $R$ with width
$R$ with width ![]() $W$, where
$W$, where ![]() $R$ is the centre radius of the mixing zone. The density
$R$ is the centre radius of the mixing zone. The density ![]() $\rho$ in the mixing zone is assumed to be uniform. After
$\rho$ in the mixing zone is assumed to be uniform. After ![]() $t+\Delta t$, the mixing zone converges to radius
$t+\Delta t$, the mixing zone converges to radius ![]() $R+\Delta R$ with width
$R+\Delta R$ with width ![]() $W+\Delta W$ and density
$W+\Delta W$ and density ![]() $\rho +\Delta \rho$.
$\rho +\Delta \rho$.
2.4. The S(C) effect and the BP effect
According to (2.22), the S(C) effect is caused by the geometrical convergence and fluid compression. For the geometrical convergence, it happens in only cylindrical and spherical geometries. For the fluid compression, it happens in a convergent system where the fluids are compressed, or when the mixing zone is influenced by waves, such as shock waves, rarefaction waves and compression waves. Therefore, we know that in planar geometry, ![]() $\dot {W}_{S(C)} = 0$ when there is no wave system acting on the mixing zone (Li et al. Reference Li, Tian, He and Zhang2021), while
$\dot {W}_{S(C)} = 0$ when there is no wave system acting on the mixing zone (Li et al. Reference Li, Tian, He and Zhang2021), while ![]() $\dot {W}_{S(C)} \ne 0$ in convergent geometries (Ge et al. Reference Ge, Zhang, Li and Tian2020). Therefore, we conclude that the S(C) effect is an important part of the BP effect.
$\dot {W}_{S(C)} \ne 0$ in convergent geometries (Ge et al. Reference Ge, Zhang, Li and Tian2020). Therefore, we conclude that the S(C) effect is an important part of the BP effect.
However, the S(C) effect is not equivalent to the BP effect. The BP effect is, in fact, a collective factor for the modification caused by the geometry, which contains multiple mechanisms. For example, as the interface converges, the wavelength of the perturbations changes, and how this factor modifies the instability remains an open question.
In this section, we give the definition of the S(C) effect in (2.14). The quantitative relation between the S(C) effect and the geometrical convergence and fluid compression is given in (2.22), which implies that the S(C) effect originates from the conservation of the mixing mass. Furthermore, we prove that the S(C) effect is one of the mechanisms of the BP effect. In the next section, a series of numerical simulations is provided to support our theoretical analysis.
3. Numerical approach and problem set-up
3.1. Governing equations and computational approach
The flow field is governed by the compressible multi-component Navier–Stokes equations. The mass, momentum, energy and mass fraction equations are
 $$\begin{gather}\frac{\partial\rho E}{\partial t}+\boldsymbol{\nabla}\boldsymbol{\cdot}(\rho E\boldsymbol{u})={-}\boldsymbol{\nabla}\boldsymbol{\cdot}\left(p\boldsymbol{u}-\boldsymbol{\tau}\boldsymbol{\cdot}\boldsymbol{u}-\kappa\,\boldsymbol{\nabla}T-T\sum_{i} C_{p,i}(\rho D\,\boldsymbol{\nabla}Y_{i})\right), \end{gather}$$
$$\begin{gather}\frac{\partial\rho E}{\partial t}+\boldsymbol{\nabla}\boldsymbol{\cdot}(\rho E\boldsymbol{u})={-}\boldsymbol{\nabla}\boldsymbol{\cdot}\left(p\boldsymbol{u}-\boldsymbol{\tau}\boldsymbol{\cdot}\boldsymbol{u}-\kappa\,\boldsymbol{\nabla}T-T\sum_{i} C_{p,i}(\rho D\,\boldsymbol{\nabla}Y_{i})\right), \end{gather}$$
where ![]() $\rho$,
$\rho$, ![]() $\boldsymbol {u}$,
$\boldsymbol {u}$, ![]() $p$, and
$p$, and ![]() $T$ are the density, velocity vector, pressure and temperature, respectively. The energy of the fluid is
$T$ are the density, velocity vector, pressure and temperature, respectively. The energy of the fluid is ![]() $E=e+\boldsymbol {u}\boldsymbol {\cdot }\boldsymbol {u}/2$, where
$E=e+\boldsymbol {u}\boldsymbol {\cdot }\boldsymbol {u}/2$, where ![]() $e$ is the internal energy, and
$e$ is the internal energy, and ![]() $\boldsymbol {u}\boldsymbol {\cdot }\boldsymbol {u}/2$ is the kinetic energy. Here,
$\boldsymbol {u}\boldsymbol {\cdot }\boldsymbol {u}/2$ is the kinetic energy. Here, ![]() $\boldsymbol {\delta }$ is the Kronecker function,
$\boldsymbol {\delta }$ is the Kronecker function, ![]() $Y_i$ is the mass fraction of the
$Y_i$ is the mass fraction of the ![]() $i$th species, where
$i$th species, where ![]() $i=1,2$ and
$i=1,2$ and ![]() $Y_{1}+Y_{2} =1$,
$Y_{1}+Y_{2} =1$, ![]() $\kappa$ and
$\kappa$ and ![]() $D$ are the mixture thermal conductivity and the mixture diffusion coefficient, respectively, and
$D$ are the mixture thermal conductivity and the mixture diffusion coefficient, respectively, and ![]() $C_{p,i}$ is the constant-pressure-specific heat capacity of the
$C_{p,i}$ is the constant-pressure-specific heat capacity of the ![]() $i$th species. To close the equations, the fluids are assumed to be Newtonian to calculate the shear stress, and the equations of state for the ideal gas are applied, i.e.
$i$th species. To close the equations, the fluids are assumed to be Newtonian to calculate the shear stress, and the equations of state for the ideal gas are applied, i.e.
where ![]() $\gamma =C_{p}/C_{V}$ is the heat capacity ratio, and
$\gamma =C_{p}/C_{V}$ is the heat capacity ratio, and ![]() $C_{V}$ is the constant-volume-specific heat capacity.
$C_{V}$ is the constant-volume-specific heat capacity.
The viscosity, thermal conductivity, and diffusivity of the ![]() $i$th species, which describe the physical properties of the component, are obtained as (Sutherland Reference Sutherland1893),
$i$th species, which describe the physical properties of the component, are obtained as (Sutherland Reference Sutherland1893),
 $$\begin{gather} \mu_{i}=\mu_{0,i}\left(\frac{T}{T_{0}}\right)^{3/2}\frac{T_{0}+T_{s}}{T+T_{s}}, \end{gather}$$
$$\begin{gather} \mu_{i}=\mu_{0,i}\left(\frac{T}{T_{0}}\right)^{3/2}\frac{T_{0}+T_{s}}{T+T_{s}}, \end{gather}$$
where ![]() $\mu _{0,i}$ is the viscosity at a reference temperature
$\mu _{0,i}$ is the viscosity at a reference temperature ![]() $T_{0}=273.15\ {\rm K}$,
$T_{0}=273.15\ {\rm K}$, ![]() $T_{s}=124\ {\rm K}$ is an effective temperature (Li et al. Reference Li, He, Zhang and Tian2019), and
$T_{s}=124\ {\rm K}$ is an effective temperature (Li et al. Reference Li, He, Zhang and Tian2019), and ![]() ${Pr}_{i}$ and
${Pr}_{i}$ and ![]() ${Sc}_{i}$ are the Prandtl number and Schmidt number for the
${Sc}_{i}$ are the Prandtl number and Schmidt number for the ![]() $i$th species, respectively.
$i$th species, respectively.
The thermodynamic quantities ![]() $f$ of the mixture in a computational cell are calculated following Reckinger, Livescu & Vasilyev (Reference Reckinger, Livescu and Vasilyev2016):
$f$ of the mixture in a computational cell are calculated following Reckinger, Livescu & Vasilyev (Reference Reckinger, Livescu and Vasilyev2016):
 \begin{equation}
f=\left\{\begin{array}{@{}ll} \displaystyle\sum_{i}f_{i}, &
{\rm for}\ f=\rho, p,\\[3pt]
f_{1}=\dots=f_{i}, & {\rm for}\
f=T,V,\\[3pt]
\displaystyle\sum_{i}Y_{i}f_{i}, & {\rm for}\
f=\mu,\kappa,D,C_{V},C_{p}. \end{array}\right.
\end{equation}
\begin{equation}
f=\left\{\begin{array}{@{}ll} \displaystyle\sum_{i}f_{i}, &
{\rm for}\ f=\rho, p,\\[3pt]
f_{1}=\dots=f_{i}, & {\rm for}\
f=T,V,\\[3pt]
\displaystyle\sum_{i}Y_{i}f_{i}, & {\rm for}\
f=\mu,\kappa,D,C_{V},C_{p}. \end{array}\right.
\end{equation}
The governing equations are solved numerically by the finite difference method with the program APEX (Adaptive-mesh-refinement Program of Eulerian solvers with X-physics). To show the reliability in simulating RM instability with APEX, we simulate the case taken from the work of Thornber et al. (Reference Thornber2017). Appendix A shows the numerical results and their comparison with other algorithms. The fifth-order weighted essentially non-oscillation (WENO) scheme is adopted for spatial reconstruction. The Harten–Lax–van Leer contact (HLLC) approximate Riemann solver is applied to calculate the convection flux. The time advancement is achieved through a third-order Runge–Kutta scheme.
3.2. Initial problem set-up
3.2.1. Computational domain
In this subsubsection, we first simulate the RM instability in a cylindrical geometry. To compare the influence of geometry, RM instability in a planar geometry is also simulated, in which the perturbed interface is the same as that unfolded from the cylindrical interface. Both two-dimensional (2-D) and three-dimensional (3-D) cases are simulated. All cases are simulated under Cartesian grids (![]() $(x,y)$ for 2-D cases, and
$(x,y)$ for 2-D cases, and ![]() $(x,y,z)$ for 3-D cases). The 2-D views of both configurations are depicted in figure 3. By comparing the subsequent growth of the perturbations on the two interfaces after shock time
$(x,y,z)$ for 3-D cases). The 2-D views of both configurations are depicted in figure 3. By comparing the subsequent growth of the perturbations on the two interfaces after shock time ![]() $t_{0}$, we can evaluate the influence caused by the geometry.
$t_{0}$, we can evaluate the influence caused by the geometry.

Figure 3. Planar slice at ![]() $z = 0$ for (a) cylindrical geometry and (b) planar geometry.
$z = 0$ for (a) cylindrical geometry and (b) planar geometry.
The initial setting of the cylindrical case is shown in figure 3(a). At the initial time ![]() $t^{-}$, the material interface is located at the position
$t^{-}$, the material interface is located at the position ![]() $r=R_{0}$. The convergent shock wave radius is
$r=R_{0}$. The convergent shock wave radius is ![]() $R_{s}(t^{-})=R_{s0}=1.1R_{0}$, and the domain size
$R_{s}(t^{-})=R_{s0}=1.1R_{0}$, and the domain size ![]() $\mathcal {V}_{{cylinder}}$ is
$\mathcal {V}_{{cylinder}}$ is
with ![]() $R_{ext}=4{\rm \pi} R_{0}$ to avoid possible influence caused by the boundaries. The symmetric boundaries are set in the axial direction
$R_{ext}=4{\rm \pi} R_{0}$ to avoid possible influence caused by the boundaries. The symmetric boundaries are set in the axial direction ![]() $z$, while outflow conditions are applied in the
$z$, while outflow conditions are applied in the ![]() $x$ and
$x$ and ![]() $y$ directions. The convergence analysis of the size of the computational domain is presented in Appendix B.
$y$ directions. The convergence analysis of the size of the computational domain is presented in Appendix B.
The initial setting of the planar case is shown in figure 3(b). The planar interface is obtained by unfolding the cylindrical interface, including the perturbations. The planar case considered is a shock tube with a constant cross-section. At the initial time ![]() $t^{-}$, the material interface is located at the position
$t^{-}$, the material interface is located at the position ![]() $x=R_{0}$. The initial shock wave locates at
$x=R_{0}$. The initial shock wave locates at ![]() $R_{s}(t^{-})=R_{s0}$, and the domain size
$R_{s}(t^{-})=R_{s0}$, and the domain size ![]() $\mathcal {V}_{{plane}}$ is
$\mathcal {V}_{{plane}}$ is
where ![]() $R_{ext}^{+}-R_{ext}^{-}\;=\;2R_{ext}$. We adjust
$R_{ext}^{+}-R_{ext}^{-}\;=\;2R_{ext}$. We adjust ![]() $R_{ext}^{-}$ to make sure that the re-shock time when the reflected shock wave impacts the interface is identical in the two geometries. An outflow boundary condition is applied at
$R_{ext}^{-}$ to make sure that the re-shock time when the reflected shock wave impacts the interface is identical in the two geometries. An outflow boundary condition is applied at ![]() $x = R_{ext}^{+}$, while a symmetric boundary condition is used at
$x = R_{ext}^{+}$, while a symmetric boundary condition is used at ![]() $x = R_{ext}^{-}$. Periodic boundaries are applied in the
$x = R_{ext}^{-}$. Periodic boundaries are applied in the ![]() $y$ direction. The boundaries in the
$y$ direction. The boundaries in the ![]() $z$ direction are symmetric.
$z$ direction are symmetric.
The streamwise direction refers to the direction in which the shock wave propagates, i.e. the radial direction in the cylindrical cases, and the ![]() $x$ direction in the planar cases. The spanwise direction denotes the direction perpendicular to the streamwise direction.
$x$ direction in the planar cases. The spanwise direction denotes the direction perpendicular to the streamwise direction.
For the 2-D cylindrical cases, the base grid resolution is ![]() $4096^{2}$, and four additional levels of refinement with a factor of two are applied. The finest resolution is therefore
$4096^{2}$, and four additional levels of refinement with a factor of two are applied. The finest resolution is therefore ![]() $65\,536^{2}$, i.e.
$65\,536^{2}$, i.e. ![]() $\Delta x_{min}\approx 3.8\times 10^{-4}R_{0}$. The same
$\Delta x_{min}\approx 3.8\times 10^{-4}R_{0}$. The same ![]() $\Delta x_{min}$ and refinement level are applied for the planar cases, i.e. the base grid resolution is
$\Delta x_{min}$ and refinement level are applied for the planar cases, i.e. the base grid resolution is ![]() $4096\times 1024$ (
$4096\times 1024$ (![]() $x\ {\rm direction}\times y\ {\rm direction}$) for the 2-D planar cases. For the 3-D cylindrical cases, the base grid resolution is
$x\ {\rm direction}\times y\ {\rm direction}$) for the 2-D planar cases. For the 3-D cylindrical cases, the base grid resolution is ![]() $512\times 512\times 128$ (
$512\times 512\times 128$ (![]() $x\ {\rm direction}\times y\ {\rm direction} \times z\ {\rm direction}$), and five additional levels of refinement with a factor of two are applied. The finest resolution is therefore
$x\ {\rm direction}\times y\ {\rm direction} \times z\ {\rm direction}$), and five additional levels of refinement with a factor of two are applied. The finest resolution is therefore ![]() $16\,384\times 16\,384\times 4096$, i.e.
$16\,384\times 16\,384\times 4096$, i.e. ![]() $\Delta x_{min}\approx 1.5\times 10^{-3}R_{0}$ in both domains. The same
$\Delta x_{min}\approx 1.5\times 10^{-3}R_{0}$ in both domains. The same ![]() $\Delta x_{min}$ and refinement level are applied for the planar cases, i.e. the base grid resolution is
$\Delta x_{min}$ and refinement level are applied for the planar cases, i.e. the base grid resolution is ![]() $512\times 128\times 128$ for the 3-D planar cases. The grid convergence analysis is presented in Appendix B.
$512\times 128\times 128$ for the 3-D planar cases. The grid convergence analysis is presented in Appendix B.
3.2.2. Initial fluid state
The two fluids considered here are air (fluid 1, outside the interface) and ![]() ${\rm SF}_{6}$-acetone mixtures (fluid 2, inside the interface). As with Tritschler et al. (Reference Tritschler, Olson, Lele, Hickel, Hu and Adams2014), the unshocked state parameters and other properties of the two fluids are shown in table 1.
${\rm SF}_{6}$-acetone mixtures (fluid 2, inside the interface). As with Tritschler et al. (Reference Tritschler, Olson, Lele, Hickel, Hu and Adams2014), the unshocked state parameters and other properties of the two fluids are shown in table 1.
Table 1. The preshock state parameters and other properties of fluid 1 (air) and fluid 2 (![]() ${\rm SF}_{6}$-acetone).
${\rm SF}_{6}$-acetone).

For the cylindrical configuration, the initialization of the postshock fluid refers to the work of Chisnell (Reference Chisnell1998). In that work, by solving the mass, momentum and entropy equations for postshock symmetric flow, using an adiabatic ideal gas assumption, the self-similar solutions of ![]() $\rho$,
$\rho$, ![]() $p$,
$p$, ![]() $\boldsymbol {u}$ are given in terms of
$\boldsymbol {u}$ are given in terms of ![]() $\xi =r/R_{s}$, where
$\xi =r/R_{s}$, where ![]() $R_{s}$ is the radius of the shock wave. For both geometries, the preshock and postshock states satisfy the Rankine–Hugoniot conditions.
$R_{s}$ is the radius of the shock wave. For both geometries, the preshock and postshock states satisfy the Rankine–Hugoniot conditions.
For the cylindrical geometry, the initial Mach number of the shock wave is set to ensure a large convergence ratio (![]() $C_{r}\sim 10$) before the interface is re-shocked. Meanwhile, the non-dimensional duration (defined in the next section) when the interface converges with a quasi-steady velocity should be long enough so that the cylindrical and planar cases are comparable. Hence the initial Mach number of the cylindrical shock wave is set to be 2.
$C_{r}\sim 10$) before the interface is re-shocked. Meanwhile, the non-dimensional duration (defined in the next section) when the interface converges with a quasi-steady velocity should be long enough so that the cylindrical and planar cases are comparable. Hence the initial Mach number of the cylindrical shock wave is set to be 2.
For the planar geometry, the Mach number is adjusted so that the velocity jump of the interface ![]() $\Delta V$ impacted by the incident shock is the same as that in the cylindrical geometry. In the cylindrical geometry, when the shock wave arrives at the interface at
$\Delta V$ impacted by the incident shock is the same as that in the cylindrical geometry. In the cylindrical geometry, when the shock wave arrives at the interface at ![]() $r = R_{0}$, the Mach number is greater than the Mach number at the initial time
$r = R_{0}$, the Mach number is greater than the Mach number at the initial time ![]() $t^{-}$, i.e.
$t^{-}$, i.e. ![]() ${Ma}(t_{0})>{Ma}(t^{-})$. By referring to the work of Si et al. (Reference Si, Long, Zhai and Luo2015), we can predict the Mach number of the convergent shock wave at the shock time
${Ma}(t_{0})>{Ma}(t^{-})$. By referring to the work of Si et al. (Reference Si, Long, Zhai and Luo2015), we can predict the Mach number of the convergent shock wave at the shock time ![]() $t_{0}$. Based on this theoretical value, the Mach number is adjusted slightly and it is finally set to be
$t_{0}$. Based on this theoretical value, the Mach number is adjusted slightly and it is finally set to be ![]() ${Ma}_{{plane}}=2.14$ in the numerical simulations.
${Ma}_{{plane}}=2.14$ in the numerical simulations.
3.2.3. Initial perturbations
Two types of initial perturbations are applied: (i) single-mode perturbation in 2-D cases, and (ii) multi-mode perturbation in 3-D cases.
The single-mode perturbations at both interfaces are presented as
and
where ![]() $a_{m}$ is the amplitude of perturbation, and
$a_{m}$ is the amplitude of perturbation, and ![]() $k_{m}=mk_{0}$, with
$k_{m}=mk_{0}$, with ![]() $m$ the wavenumber and
$m$ the wavenumber and ![]() $k_{0}=2{\rm \pi} /(2{\rm \pi} R_{0})=1/R_{0}$. Two wavenumbers are applied:
$k_{0}=2{\rm \pi} /(2{\rm \pi} R_{0})=1/R_{0}$. Two wavenumbers are applied: ![]() $m=8$ and
$m=8$ and ![]() $m=16$, which are named SP8 and SP16, respectively. The initial amplitude is
$m=16$, which are named SP8 and SP16, respectively. The initial amplitude is ![]() $a_{m}=0.01\lambda _{m}$, where
$a_{m}=0.01\lambda _{m}$, where ![]() $\lambda _{m}=2{\rm \pi} R_{0}/m$.
$\lambda _{m}=2{\rm \pi} R_{0}/m$.
The multi-mode perturbation is a superposition of a series of modes with a prescribed power spectrum (Dimonte et al. Reference Dimonte2004). The power spectrum is given as
 \begin{equation} P(k)=\begin{cases} Fk^{l}, & k_{min}< k< k_{max}, \\ 0, & \text{otherwise}, \end{cases} \end{equation}
\begin{equation} P(k)=\begin{cases} Fk^{l}, & k_{min}< k< k_{max}, \\ 0, & \text{otherwise}, \end{cases} \end{equation}
where ![]() $k=\sqrt {k_{m}^{2}+k_{n}^{2}}$ denotes the wavenumber of the mode
$k=\sqrt {k_{m}^{2}+k_{n}^{2}}$ denotes the wavenumber of the mode ![]() $(m,n)$, and
$(m,n)$, and ![]() $F$ is the coefficient. In physical space, the perturbations are of the form
$F$ is the coefficient. In physical space, the perturbations are of the form
 \begin{align}
\eta_{{cylinder}}(\theta,z)=\sum_{m,n}&\bigl(a_{mn}\cos(k_{m}\theta
R_{0})\cos (k_{n}z)+b_{mn}\sin(k_{m}\theta
R_{0})\cos(k_{n}z)\nonumber\\ &\quad
+c_{mn}\cos(k_{m}\theta
R_{0})\sin(k_{n}z)+d_{mn}\sin(k_{m}\theta
R_{0})\sin(k_{n}z)\bigr)
\end{align}
\begin{align}
\eta_{{cylinder}}(\theta,z)=\sum_{m,n}&\bigl(a_{mn}\cos(k_{m}\theta
R_{0})\cos (k_{n}z)+b_{mn}\sin(k_{m}\theta
R_{0})\cos(k_{n}z)\nonumber\\ &\quad
+c_{mn}\cos(k_{m}\theta
R_{0})\sin(k_{n}z)+d_{mn}\sin(k_{m}\theta
R_{0})\sin(k_{n}z)\bigr)
\end{align}
and
 \begin{align}
\eta_{{plane}}(y,z)=\sum_{m,n}&\bigl(a_{mn}\cos(k_{m}y)\cos(k_{n}z)+b_{mn}\sin(k_{m}y)\cos(k_{n}z)
\nonumber\\ &\quad
+c_{mn}\cos(k_{m}y)\sin(k_{n}z)+d_{mn}\sin(k_{m}y)\sin(k_{n}z)\bigr),
\end{align}
\begin{align}
\eta_{{plane}}(y,z)=\sum_{m,n}&\bigl(a_{mn}\cos(k_{m}y)\cos(k_{n}z)+b_{mn}\sin(k_{m}y)\cos(k_{n}z)
\nonumber\\ &\quad
+c_{mn}\cos(k_{m}y)\sin(k_{n}z)+d_{mn}\sin(k_{m}y)\sin(k_{n}z)\bigr),
\end{align}
where the amplitude coefficients ![]() $a_{mn}$,
$a_{mn}$, ![]() $b_{mn}$,
$b_{mn}$, ![]() $c_{mn}$ and
$c_{mn}$ and ![]() $d_{mn}$ are determined by the variance
$d_{mn}$ are determined by the variance ![]() $\sigma ^{2}_{mn}\sim P(k_{m},k_{n})\,\Delta k_{m}\Delta k_{n}$, which corresponds to the energy involved in wave space, and
$\sigma ^{2}_{mn}\sim P(k_{m},k_{n})\,\Delta k_{m}\Delta k_{n}$, which corresponds to the energy involved in wave space, and ![]() $k_{m}=mk_{0}$ and
$k_{m}=mk_{0}$ and ![]() $k_{n}=nk_{0}$, where
$k_{n}=nk_{0}$, where ![]() $k_{0}=2{\rm \pi} /(2{\rm \pi} R_{0})=1/R_{0}$. The relevant detailed demonstration of the transformation from the wave space (3.9) to the physical space (3.10) and (3.11) has been given by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2010). In the present work, a narrowband perturbation with wavenumbers ranging from 16 to 48 is used, and the energy spectrum follows
$k_{0}=2{\rm \pi} /(2{\rm \pi} R_{0})=1/R_{0}$. The relevant detailed demonstration of the transformation from the wave space (3.9) to the physical space (3.10) and (3.11) has been given by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2010). In the present work, a narrowband perturbation with wavenumbers ranging from 16 to 48 is used, and the energy spectrum follows ![]() $P(k)=Fk^{0}$. The total standard deviation is
$P(k)=Fk^{0}$. The total standard deviation is ![]() $\sigma =\sqrt {\int P(k)\,{\rm d}k}\approx 0.0067R_{0}$. These cases are named MP16–48.
$\sigma =\sqrt {\int P(k)\,{\rm d}k}\approx 0.0067R_{0}$. These cases are named MP16–48.
3.2.4. Summary of initial conditions
The initial conditions are designed carefully so that the RM instability on a planar interface and a cylindrical interface are comparable. A perturbed cylindrical interface and a planar interface obtained by unfolding the cylindrical interface are impacted by shock waves with the same strength. According to the RM linear model ![]() $\dot {W}=A^{+}k\,\Delta V\,W^{+}$ in the planar geometry (Richtmyer Reference Richtmyer1960), where
$\dot {W}=A^{+}k\,\Delta V\,W^{+}$ in the planar geometry (Richtmyer Reference Richtmyer1960), where ![]() $A^{+}$ and
$A^{+}$ and ![]() $W^{+}$ are the postshock Atwood number and the postshock width of the perturbation, the perturbations in the two geometries should have the same initial growth rate. During the subsequent growth of the perturbations, whether the interface converges or not is the only independent variable, from which we can reasonably compare the difference in the perturbation evolution under the two geometries. Specifically, the set-ups need to meet the following conditions.
$W^{+}$ are the postshock Atwood number and the postshock width of the perturbation, the perturbations in the two geometries should have the same initial growth rate. During the subsequent growth of the perturbations, whether the interface converges or not is the only independent variable, from which we can reasonably compare the difference in the perturbation evolution under the two geometries. Specifically, the set-ups need to meet the following conditions.
(i) The initial preshock fluid states, the initial perturbations and the initial interface size are the same in both geometries.
(ii) The Mach number in the planar geometry is larger than that in the cylindrical geometry at initial time
 $t^{-}$ to ensure that the velocity jumps
$t^{-}$ to ensure that the velocity jumps  $\Delta V$ impacted by the incident shock are equal in the two geometries.
$\Delta V$ impacted by the incident shock are equal in the two geometries.(iii) The convergence ratio
 $C_{r}$ should be high, and the non-dimensional duration when the interface converges with a quasi-steady velocity should be long enough so that the cylindrical and planar cases are comparable. The re-shock time when the reflected shock wave impacts the interface should also be identical in the two geometries.
$C_{r}$ should be high, and the non-dimensional duration when the interface converges with a quasi-steady velocity should be long enough so that the cylindrical and planar cases are comparable. The re-shock time when the reflected shock wave impacts the interface should also be identical in the two geometries.
4. Numerical results
4.1. One-dimensional unperturbed flow
In this section, we inspect the one-dimensional unperturbed cases, with an emphasis on the periods before the re-shock moment ![]() $t_{rs}$. Figure 4 shows the interface motion
$t_{rs}$. Figure 4 shows the interface motion ![]() $R(t)$ in both geometries. The interface has a non-zero width because of the numerical diffusion, therefore the position of the interface
$R(t)$ in both geometries. The interface has a non-zero width because of the numerical diffusion, therefore the position of the interface ![]() $R(t)$ is defined as the radius where
$R(t)$ is defined as the radius where ![]() $Y_{1}(R(t),t)=0.5$. The curves obtained from the planar case are shifted along the time axis so that the shock time
$Y_{1}(R(t),t)=0.5$. The curves obtained from the planar case are shifted along the time axis so that the shock time ![]() $t_s$ is aligned. The interface is shocked at time
$t_s$ is aligned. The interface is shocked at time ![]() $t_{s}=0.4\ {\rm ms}$, then the planar interface moves with a constant velocity
$t_{s}=0.4\ {\rm ms}$, then the planar interface moves with a constant velocity ![]() $\Delta V =340\ {\rm m}\ {\rm s}^{{-1}}$ until the interface is re-shocked at
$\Delta V =340\ {\rm m}\ {\rm s}^{{-1}}$ until the interface is re-shocked at ![]() $t_{rs}=8.5\ {\rm ms}$. For the cylindrical case, before
$t_{rs}=8.5\ {\rm ms}$. For the cylindrical case, before ![]() $t_{1}=5\ {\rm ms}$, the convergence velocity is slightly increased, and after that, the interface begins to decelerate. At re-shock time
$t_{1}=5\ {\rm ms}$, the convergence velocity is slightly increased, and after that, the interface begins to decelerate. At re-shock time ![]() $t_{rs}$, the final convergence ratio of the interface is
$t_{rs}$, the final convergence ratio of the interface is ![]() $C_{r}(t_{rs})\sim 10$.
$C_{r}(t_{rs})\sim 10$.

Figure 4. The displacement (a) and velocity (b) of the interface.
The temporal evolution of the averaged fluid compression rate ![]() $\gamma _{\rho }$ is plotted in figure 5, where the density value is sampled from two sides of the interface. At time
$\gamma _{\rho }$ is plotted in figure 5, where the density value is sampled from two sides of the interface. At time ![]() $t_{s}$, the interface is shocked by the shock wave, which results in a strong compression of the fluids. For time
$t_{s}$, the interface is shocked by the shock wave, which results in a strong compression of the fluids. For time ![]() $t\in [t_{s},t_{0}]$, where
$t\in [t_{s},t_{0}]$, where ![]() $t_{0}=1$ ms, the shock wave continues to affect the fluids near the interface, which results in the negative pulse of the fluid compression. After
$t_{0}=1$ ms, the shock wave continues to affect the fluids near the interface, which results in the negative pulse of the fluid compression. After ![]() $t_{0}$, the planar RM flow is quasi-incompressible before the re-shock, which supports the discussion in § 2.4. Fluid compression is one of the basic features of convergent RM instability. Therefore, for cylindrical cases, after
$t_{0}$, the planar RM flow is quasi-incompressible before the re-shock, which supports the discussion in § 2.4. Fluid compression is one of the basic features of convergent RM instability. Therefore, for cylindrical cases, after ![]() $t_{0}$, a non-zero fluid compression rate can still be observed, and its absolute value continues to rise with the convergence of the interface.
$t_{0}$, a non-zero fluid compression rate can still be observed, and its absolute value continues to rise with the convergence of the interface.

Figure 5. The temporal evolution of the fluid compression rate.
According to the evolution of the fluid parameters mentioned above, the flow evolution is divided into five stages, as shown in table 2. Due to the nature of the convergent shock wave, the acceleration of the convergent interface is not zero. Therefore, the growth of perturbations in the convergent geometries is coupled with RT stability/instability. RT instability, as introduced in § 1, is the phenomenon where the perturbation grows in an acceleration environment (the lighter fluid accelerates the heavier fluid), while the RT stability is the situation when the heavier fluid accelerates the lighter fluid and the perturbations oscillates or attenuates.
Table 2. Definition of five stages of the RM instability until the re-shock time.

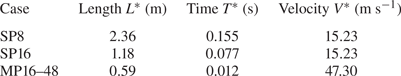
The quantities presented in the following subsections are non-dimensionalized. For the single-mode cases, the characteristic length is the initial wavelength of the perturbation, ![]() $L^{*}=\lambda$. The characteristic velocity is
$L^{*}=\lambda$. The characteristic velocity is ![]() $V^{*}=A^{+}k\,\Delta V\,W^{+}$, where
$V^{*}=A^{+}k\,\Delta V\,W^{+}$, where ![]() $W^{+}=(1-\Delta V/V_{s})\,W(t^{-})$, and
$W^{+}=(1-\Delta V/V_{s})\,W(t^{-})$, and ![]() $V_{s}$ is the velocity of the shock wave. For the multi-mode cases, the characteristic velocity is defined as the growth rate of the perturbations at time
$V_{s}$ is the velocity of the shock wave. For the multi-mode cases, the characteristic velocity is defined as the growth rate of the perturbations at time ![]() $t_{0}$ when the shock wave just passes away from the interface. The characteristic length is
$t_{0}$ when the shock wave just passes away from the interface. The characteristic length is ![]() $L^{*}=\bar {\lambda }$, where
$L^{*}=\bar {\lambda }$, where ![]() $\bar {\lambda }=2{\rm \pi} /\bar {k}$ is the equivalent wavelength of the perturbations at time
$\bar {\lambda }=2{\rm \pi} /\bar {k}$ is the equivalent wavelength of the perturbations at time ![]() $t^{-}$, and
$t^{-}$, and ![]() $\bar {k}$ is calculated by the energy-weighted average of
$\bar {k}$ is calculated by the energy-weighted average of ![]() $k$, which is formulated as
$k$, which is formulated as
 \begin{equation} \bar{k}=\frac{\displaystyle\int_{k_{min}}^{k_{max}}k\,P(k)\,\mathrm{d}k}{\displaystyle\int_{k_{min}}^{k_{max}}P(k) \,\mathrm{d}k}. \end{equation}
\begin{equation} \bar{k}=\frac{\displaystyle\int_{k_{min}}^{k_{max}}k\,P(k)\,\mathrm{d}k}{\displaystyle\int_{k_{min}}^{k_{max}}P(k) \,\mathrm{d}k}. \end{equation}
In this paper, ![]() $P(k)=Ck^{0}$, hence
$P(k)=Ck^{0}$, hence ![]() $\bar {k}=0.5(k_{min}+k_{max})$. The characteristic quantities for all of the cases are listed in table 3.
$\bar {k}=0.5(k_{min}+k_{max})$. The characteristic quantities for all of the cases are listed in table 3.
Table 3. Characteristic quantities for each case.
4.2. Single-mode cases
Figures 6 and 7 show the growth of the perturbations for the cases SP8 and SP16, respectively. After ![]() $\tau =\tau _{0}$ when the shock wave passes away from the interface, the initial growth rates are identical in both geometries, as designed in § 3. Because the initial amplitude of the perturbations is set as
$\tau =\tau _{0}$ when the shock wave passes away from the interface, the initial growth rates are identical in both geometries, as designed in § 3. Because the initial amplitude of the perturbations is set as ![]() $a_{m}=0.01\lambda _{m}$, the growth of the perturbations lies in the linear stage.
$a_{m}=0.01\lambda _{m}$, the growth of the perturbations lies in the linear stage.

Figure 6. For the case SP8, the temporal evolution of the mixing width, as well as its growth rate, in both geometries.

Figure 7. For the case SP16, the temporal evolution of the mixing width, as well as its growth rate, in both geometries.
For the planar configurations, the growth rate of the perturbations increases slowly and is smaller than the growth rate predicted by the linear theory (Richtmyer Reference Richtmyer1960) by the time ![]() $t_{rs}$, i.e. the non-dimensional growth rate is smaller than 1. This shows that the growth of the perturbations is still in the start-up process (Lombardini & Pullin Reference Lombardini and Pullin2009). For the cylindrical configurations, the temporal evolution of the perturbation growth rate is significantly different from that of the planar configurations. The growth rate in the cylindrical geometry is first accelerated and then decelerated to a negative value. This complicated growth behaviour in the cylindrical geometry originates from the coupling of multiple physical effects (for example, the RT instability/stability, geometrical convergence, and fluid compression).
$t_{rs}$, i.e. the non-dimensional growth rate is smaller than 1. This shows that the growth of the perturbations is still in the start-up process (Lombardini & Pullin Reference Lombardini and Pullin2009). For the cylindrical configurations, the temporal evolution of the perturbation growth rate is significantly different from that of the planar configurations. The growth rate in the cylindrical geometry is first accelerated and then decelerated to a negative value. This complicated growth behaviour in the cylindrical geometry originates from the coupling of multiple physical effects (for example, the RT instability/stability, geometrical convergence, and fluid compression).
The decomposition formula (2.14) is applied to decompose the growth of the perturbations, where the contribution of the diffusion is neglected. The results are given in figures 8 and 9. In all configurations, the results obtained by the decomposition formula ![]() $\dot {W}_{formula}$ and the results extracted directly from the numerical simulations
$\dot {W}_{formula}$ and the results extracted directly from the numerical simulations ![]() $\dot {W} _{sim}$ are consistent, which proves the reliability of the decomposition formula. Furthermore, in figures 8(b) and 9(b), we have
$\dot {W} _{sim}$ are consistent, which proves the reliability of the decomposition formula. Furthermore, in figures 8(b) and 9(b), we have ![]() $\dot {W}_{Pen,G}=u_{Pen,G}\vert _{B}-u_{Pen,G}\vert _{S}\approx 0$, where
$\dot {W}_{Pen,G}=u_{Pen,G}\vert _{B}-u_{Pen,G}\vert _{S}\approx 0$, where ![]() $u_{Pen,G}$ is defined in (2.13), which is neglected in the following analysis.
$u_{Pen,G}$ is defined in (2.13), which is neglected in the following analysis.

Figure 8. For the case SP8, the growth rates obtained by the simulation and the decomposition formula for (a) planar geometry and (b) cylindrical geometry.

Figure 9. For the case SP16, the growth rates obtained by the simulation and the decomposition formula for (a) planar geometry and (b) cylindrical geometry.
For the planar cases, one can observe that ![]() $\dot {W}_{S(C)}$, which represents the contribution of the S(C) effect, is not zero only when the wave system acts on the mixing zone. For most of the time in the duration
$\dot {W}_{S(C)}$, which represents the contribution of the S(C) effect, is not zero only when the wave system acts on the mixing zone. For most of the time in the duration ![]() $[\tau _{0},\tau _{rs}]$, the growth of the mixing width is dominated by the penetration effect, as shown in figures 8(a) and 9(a), which is consistent with the previous work of Li et al. (Reference Li, Tian, He and Zhang2021). For the cylindrical geometry, at the initial time,
$[\tau _{0},\tau _{rs}]$, the growth of the mixing width is dominated by the penetration effect, as shown in figures 8(a) and 9(a), which is consistent with the previous work of Li et al. (Reference Li, Tian, He and Zhang2021). For the cylindrical geometry, at the initial time, ![]() $\dot {W}_{S(C)}(\tau _{0})=0$. However, with the evolution of the flow, the contribution of the S(C) effect continues to increase and finally exceeds the penetration effect to dominate the growth of the perturbations. From the numerical results, we know that the S(C) effect is very different in the two geometries, therefore the S(C) effect is an important part of the BP effect.
$\dot {W}_{S(C)}(\tau _{0})=0$. However, with the evolution of the flow, the contribution of the S(C) effect continues to increase and finally exceeds the penetration effect to dominate the growth of the perturbations. From the numerical results, we know that the S(C) effect is very different in the two geometries, therefore the S(C) effect is an important part of the BP effect.
The comparisons of the penetration effect are shown in figure 10. In both geometries, the ![]() $\dot {W}_{Pen}$ values are identical at the initial time. With the coupling effect of geometrical convergence and the RT instability, the fluid penetration effect increases before the convergent velocity reaches its maximum
$\dot {W}_{Pen}$ values are identical at the initial time. With the coupling effect of geometrical convergence and the RT instability, the fluid penetration effect increases before the convergent velocity reaches its maximum ![]() $\tau _{1}$. After that, under the influence of the RT stability, the penetration rate decreases to a negative value at late time.
$\tau _{1}$. After that, under the influence of the RT stability, the penetration rate decreases to a negative value at late time.

Figure 10. For (a) the case SP8 and (b) the case SP16, the different performances of the penetration effect in the two geometries.
The evolution of the S(C) effects is shown in figure 11. The values of ![]() $\gamma _{\rho }$ and
$\gamma _{\rho }$ and ![]() $\gamma _{R}$ used in (2.22) are obtained from the one-dimensional unperturbed simulations. The numerical results and the theory agree well, which proves the reliability of the theory.
$\gamma _{R}$ used in (2.22) are obtained from the one-dimensional unperturbed simulations. The numerical results and the theory agree well, which proves the reliability of the theory.
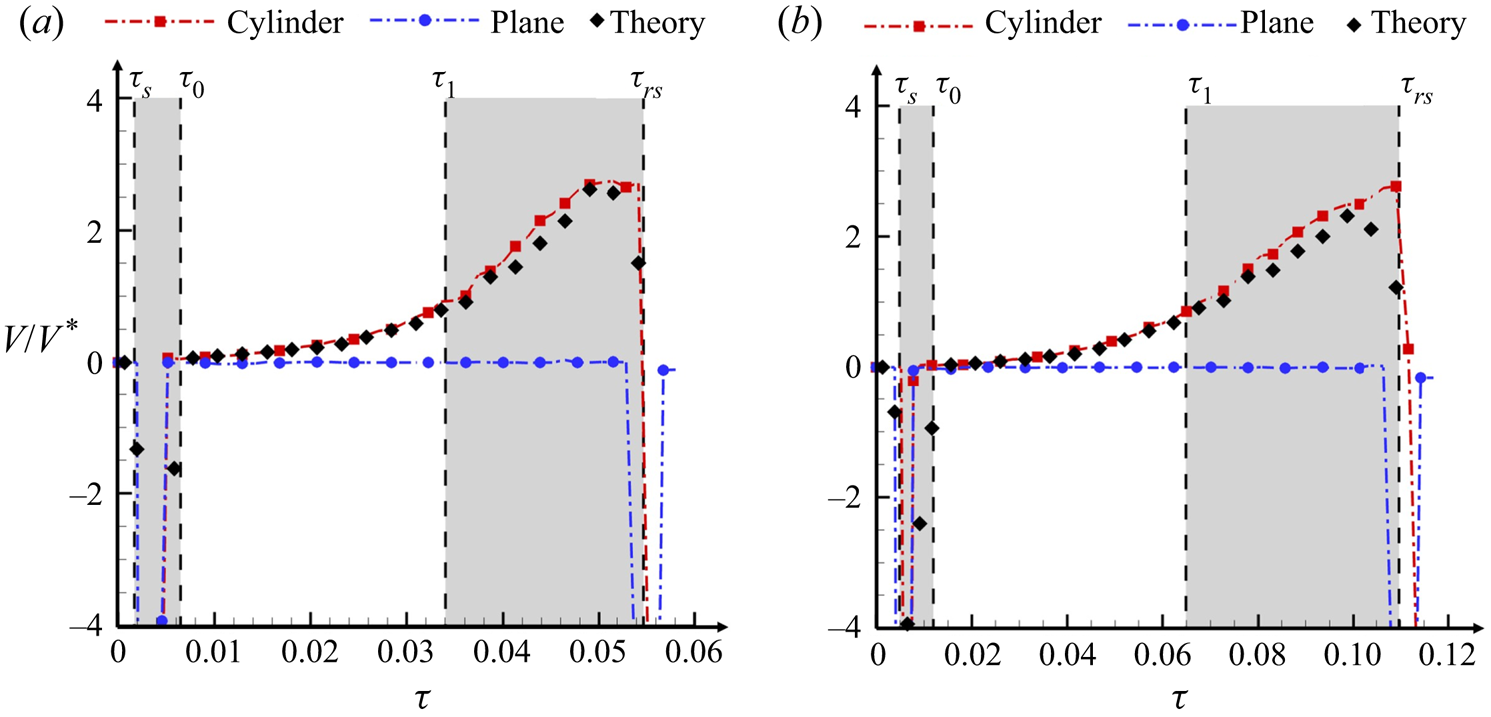
Figure 11. For (a) the case SP8 and (b) the case SP16, the evolution of the S(C) effect in the two geometries. The theoretical prediction for the S(C) effect in the cylindrical geometry is also shown.
The stretching effect (![]() $V_{Stretching}=\gamma _{R}W$) and the compression effect (
$V_{Stretching}=\gamma _{R}W$) and the compression effect (![]() $V_{Compression}=\gamma _{\rho }W$) are given in figure 12. In the short duration
$V_{Compression}=\gamma _{\rho }W$) are given in figure 12. In the short duration ![]() $\tau \in [\tau _{s},\tau _{0}]$, the perturbations are compressed strongly by the shock wave. Therefore, the S(C) effect is dominated by the compression effect caused by the shock wave. In the duration
$\tau \in [\tau _{s},\tau _{0}]$, the perturbations are compressed strongly by the shock wave. Therefore, the S(C) effect is dominated by the compression effect caused by the shock wave. In the duration ![]() $\tau \in [\tau _{0},\tau _{rs}]$, the stretching velocity is always greater than the compression velocity, with the ratio
$\tau \in [\tau _{0},\tau _{rs}]$, the stretching velocity is always greater than the compression velocity, with the ratio ![]() $|V_{Stretching}/V_{Compression}|$ ranging from 1 to 2.
$|V_{Stretching}/V_{Compression}|$ ranging from 1 to 2.

Figure 12. For (a) the case SP8 and (b) the case SP16, the contributions of different components in the S(C) effect of the cylindrical cases.
4.3. Multi-mode case
To examine the universality of the results obtained from the single-mode cases, the multi-mode cases are simulated. Figure 13 gives the growth of the perturbations for the cases MP16–48. For the planar configuration, after ![]() $\tau =\tau _{0}$, there is no more energy injected into the system. Therefore, in the duration
$\tau =\tau _{0}$, there is no more energy injected into the system. Therefore, in the duration ![]() $\tau \in [\tau _{0},\tau _{1}]$, the growth rate of the perturbations first experiences a short-time increase and then attenuates. For the cylindrical configuration, it is obvious that the convergent interface enhances the growth of the perturbations. In the duration
$\tau \in [\tau _{0},\tau _{1}]$, the growth rate of the perturbations first experiences a short-time increase and then attenuates. For the cylindrical configuration, it is obvious that the convergent interface enhances the growth of the perturbations. In the duration ![]() $\tau \in [\tau _{0},\tau _{1}]$, the growth rate of the perturbations does not decay, in contrast with that in the planar geometry. During the subsequent duration
$\tau \in [\tau _{0},\tau _{1}]$, the growth rate of the perturbations does not decay, in contrast with that in the planar geometry. During the subsequent duration ![]() $\tau \in [\tau _{1},\tau _{rs}]$, the acceleration of the interface is pointed from the heavy fluid to the light fluid; hence the growth of the perturbations is influenced by the RT stability, which decelerates the growth of the perturbations.
$\tau \in [\tau _{1},\tau _{rs}]$, the acceleration of the interface is pointed from the heavy fluid to the light fluid; hence the growth of the perturbations is influenced by the RT stability, which decelerates the growth of the perturbations.

Figure 13. For the cases MP16–48, the temporal evolution of the mixing width, as well as its velocity, in both geometries.
Figure 14 shows the growth rates obtained with the decomposition formula and those obtained from the numerical results in both geometries. In both geometries, at time ![]() $\tau _{s}$ when the incident shock wave impacts the interface,
$\tau _{s}$ when the incident shock wave impacts the interface, ![]() $\dot {W}_{S(C)}$ experiences a sharp decrease due to the compression by the shock wave. Meanwhile, the incidence shock wave deposits vorticity on the interface, which results in an increase in the penetration effect
$\dot {W}_{S(C)}$ experiences a sharp decrease due to the compression by the shock wave. Meanwhile, the incidence shock wave deposits vorticity on the interface, which results in an increase in the penetration effect ![]() $\dot {W}_{Pen}$.
$\dot {W}_{Pen}$.

Figure 14. For the cases MP16–48, the growth rates obtained by the simulation and the decomposition formula for (a) planar geometry and (b) cylindrical geometry.
For the planar configuration, ![]() $\dot {W}_{S(C)}$ is always zero when
$\dot {W}_{S(C)}$ is always zero when ![]() $\tau \in [\tau _{0},\tau _{rs}]$, which indicates that the growth of the mixing width is dominated by the penetration effect if there is no influence of the wave system. For the cylindrical configuration, with the convergence of the interface, the stretching effect of the mixing width continues to increase. When
$\tau \in [\tau _{0},\tau _{rs}]$, which indicates that the growth of the mixing width is dominated by the penetration effect if there is no influence of the wave system. For the cylindrical configuration, with the convergence of the interface, the stretching effect of the mixing width continues to increase. When ![]() $\tau >\tau _{1}$, the contribution of the penetration effect decreases due to the RT stability, while the stretching effect surpasses the penetration effect and begins to dominate the perturbation growth.
$\tau >\tau _{1}$, the contribution of the penetration effect decreases due to the RT stability, while the stretching effect surpasses the penetration effect and begins to dominate the perturbation growth.
Figure 15(a) shows the evolution of the penetration effect in the two geometries. After the passage of the shock wave, as the initial growth rates are the same, the penetration rates in both geometries are identical. In the subsequent duration ![]() $\tau \in [\tau _{0},\tau _{1}]$, the penetration rates increase first and then decay with almost the same rate in both geometries. This is because the growth of most perturbation modes enters the nonlinear phase. This phenomenon is different from the single-mode cases, for which the growth of the perturbations lies in the linear stage or the weakly nonlinear stage for most of the time. When
$\tau \in [\tau _{0},\tau _{1}]$, the penetration rates increase first and then decay with almost the same rate in both geometries. This is because the growth of most perturbation modes enters the nonlinear phase. This phenomenon is different from the single-mode cases, for which the growth of the perturbations lies in the linear stage or the weakly nonlinear stage for most of the time. When ![]() $\tau \in [\tau _{1},\tau _{rs}]$, the growth of the perturbations in cylindrical geometries is influenced by the RT stability. As a result, the penetration rate decays faster in cylindrical geometry than in planar geometry. In the late stage, the penetration effect is negative in cylindrical geometry, which indicates a reduction in the mixing width.
$\tau \in [\tau _{1},\tau _{rs}]$, the growth of the perturbations in cylindrical geometries is influenced by the RT stability. As a result, the penetration rate decays faster in cylindrical geometry than in planar geometry. In the late stage, the penetration effect is negative in cylindrical geometry, which indicates a reduction in the mixing width.

Figure 15. For the cases MP16–48, the different performance of (a) the penetration effect and (b) the S(C) effect, in the two geometries. The theoretical prediction of the S(C) effect is also shown.
As shown in figure 15(b), the behaviour of the S(C) effect in the multi-mode case is similar to that in the single-mode cases. For the planar cases, the influence of the S(C) effect is observed only when the shock wave impacts the interface, while ![]() $\dot {W}_{S(C)}$ increases with time in the cylindrical geometry. The ratio between the stretching effect and compression effect is also similar to the single-mode cases, as shown in figure 16.
$\dot {W}_{S(C)}$ increases with time in the cylindrical geometry. The ratio between the stretching effect and compression effect is also similar to the single-mode cases, as shown in figure 16.

Figure 16. For the cases MP16–48, the contribution of different components in the S(C) effect of the cylindrical geometry.
5. Discussion
The numerical results presented in § 4 support our discussion in § 2 that the S(C) effect is an important part of the BP effect. Furthermore, as shown in § 4, for different geometries, there indeed exists a difference in the penetration effect, which verifies that the BP effect has in fact multiple mechanisms. Therefore, the S(C) effect is an important part of the BP effect but not equivalent to the BP effect. Because of the non-zero acceleration in the cylindrical interface, it is hard to evaluate the modification in the penetration effect caused by the BP effect. For our single-mode cases, the growth of perturbation is mainly in the linear and weakly nonlinear phase, and the difference in the penetration effect is obvious. However, in our multi-mode cases, the perturbations grow rapidly into the nonlinear phase. In the duration ![]() $\tau \in [\tau _{0},\tau _{1}]$ when there is no strong RT instability, it is remarkable that the main difference between the growths of the perturbations in the two geometries is contributed by the S(C) effect, and that there is little difference in the penetration effect. In physical reality, the perturbations are always multi-mode, which contains a wide range of modes. Therefore, the results of single-mode cases can be referred to so as to understand the evolution of the low-order modes, while the results of the multi-mode cases can be referred to in order to understand the evolution of the high-order modes. However, the quantitative analysis of the perturbation growth in reality needs further investigations, especially for the penetration effect.
$\tau \in [\tau _{0},\tau _{1}]$ when there is no strong RT instability, it is remarkable that the main difference between the growths of the perturbations in the two geometries is contributed by the S(C) effect, and that there is little difference in the penetration effect. In physical reality, the perturbations are always multi-mode, which contains a wide range of modes. Therefore, the results of single-mode cases can be referred to so as to understand the evolution of the low-order modes, while the results of the multi-mode cases can be referred to in order to understand the evolution of the high-order modes. However, the quantitative analysis of the perturbation growth in reality needs further investigations, especially for the penetration effect.
The S(C) effect can explain the phenomenon of the ‘thickening smooth premixing layer’ in the LANL experiments (Lanier et al. Reference Lanier, Barnes, Batha, Day, Magelssen, Scott, Dunne, Parker and Rothman2003). Since there is no penetration effect for the smooth premixing layer, the mixing layer thickens because of the S(C) effect. The theoretical results of the S(C) effect in different geometries (2.22) does not include explicitly the initial perturbations, interface acceleration and Atwood number. Moreover, as shown in § 4, (2.22) is applicable for different forms of initial perturbations and all stages of flow evolution.
As the S(C) effect is the mechanism independent to the penetration effect, this part of the contribution should be considered additionally in constructing models for the growth of mixing width in the convergent geometries. For example, El Rafei & Thornber (Reference El Rafei and Thornber2020) propose a buoyancy-drag model for the RM instability in the spherical geometry to model the mixing-zone width, where the ‘BP effect’ is taken into account in ‘bubble and spike velocities (![]() $V_{b}, V_{s}$) relative to the fluid’. Mathematically,
$V_{b}, V_{s}$) relative to the fluid’. Mathematically, ![]() $V_{b}$,
$V_{b}$, ![]() $V_{s}$ are calculated as
$V_{s}$ are calculated as
where ![]() $U_{b}$,
$U_{b}$, ![]() $U_{s}$ and
$U_{s}$ and ![]() $U_{I}$ are determined from one-dimensional results at the bubble front, spike front and interface, respectively (El Rafei & Thornber Reference El Rafei and Thornber2020). Essentially, the second term on the right-hand side of each equation in (5.1) can be explained as eliminating the S(C) effect.
$U_{I}$ are determined from one-dimensional results at the bubble front, spike front and interface, respectively (El Rafei & Thornber Reference El Rafei and Thornber2020). Essentially, the second term on the right-hand side of each equation in (5.1) can be explained as eliminating the S(C) effect.
6. Conclusions
The S(C) effect, which is induced by the velocity difference between two ends of the mixing zone, is defined based on the decomposition formula for the growth of the mixing width. Starting from the linear theory, the quantitative relationship between the S(C) effect and the flow parameters is given. The physical origin of the S(C) effect and the relationship between the S(C) effect and the BP effect are discussed. A series of numerical simulations in cylindrical and planar geometries is performed to describe modifications of RM instability induced by the geometry. To ensure that the numerical cases in the two geometries are comparable, the perturbed planar interface is obtained by unfolding the perturbed cylindrical interface. The preshock flow states are identical in both geometries. Moreover, both interfaces are impacted by a shock wave of the same strength. The convergence ratio of the cylindrical cases ![]() $C_{r}\sim 10$ makes sure that the BP effect is shown clearly. The conclusions are listed below.
$C_{r}\sim 10$ makes sure that the BP effect is shown clearly. The conclusions are listed below.
(i) The S(C) effect in planar, cylindrical and spherical geometries is evaluated as
 $\dot {W}_{S(C)}=(\alpha \gamma _{R}+\gamma _{\rho })W$ in (2.22). Further analysis about (2.22) shows that when there is no wave acting on the mixing zone,
$\dot {W}_{S(C)}=(\alpha \gamma _{R}+\gamma _{\rho })W$ in (2.22). Further analysis about (2.22) shows that when there is no wave acting on the mixing zone,  $W_{S(C)} = 0$ in the planar geometry while
$W_{S(C)} = 0$ in the planar geometry while  $W_{S(C)}\ne 0$ in convergent geometries. Therefore, we conclude that the S(C) effect is an important part of the BP effect.
$W_{S(C)}\ne 0$ in convergent geometries. Therefore, we conclude that the S(C) effect is an important part of the BP effect.(ii) The physical origin underlying the S(C) effect is that the mass in the mixing zone is conserved if we do not consider the mass brought in by the penetration effect.
(iii) Both the decomposition formula and the theoretical expression of the S(C) effect agree well with the corresponding numerical results. This agreement is valid for a wide range of initial perturbations and different evolution stages. In the present cases, the contribution of the geometrical effect is always greater than the fluid compression, except for the duration when the shock wave impacts the mixing zone. Therefore, the mixing zone is always stretched.
(iv) The penetration effect is different in the two geometries. Therefore, the S(C) effect is not equivalent to the BP effect. In the present work, the quantitative comparison of the penetration effect in the two geometries is hard because of the existence of the RT stability/instability. The modification on the penetration effect caused by the geometry remains an open question.
(v) The S(C) effect is a growth mechanism that is independent of the RM and RT instabilities (Li et al. Reference Li, Tian, He and Zhang2021). Therefore, for the RM/RT instability in a convergent geometry, additional consideration needs to be given to the stretching or compression of the mixing zone when we model the mixing width by the bubble/spike dynamics (such as the buoyancy-drag model) or extend the understanding in the planar geometry to the convergent geometry.
In the present work, the lighter fluid locates in the outside of the interface, i.e. the initial shock wave propagates from the light fluid to the heavy fluid. For the opposite configuration where the lighter fluid is in the inside, the growth of the perturbation will experience a phase-reversal process, i.e. the amplitude of the perturbation first decreases and then increases in the oppose direction. This process occupies a considerable part of the comparable time, which will influence the comparison between the two geometries. This is why we choose the configuration to put the lighter fluid on the outside. However, the comparison of RM instability in planar and cylindrical geometries where the lighter fluid is in the inside of the interface is still important work worthy of future investigation.
Funding
The authors acknowledge financial support from the National Natural Science Foundation of China (B.T., grant no. 91852207), (H.L., grant no. 11802038). The authors want to extend thanks for the Tianhe-2 computing resources supported by the group of Professor C.-B. Li from Peking University.
Declaration of interests
The authors report no conflict of interest.
Appendix A. Validation of APEX
In the work of Thornber et al. (Reference Thornber2017), eight independent algorithms were employed to test a standard RM problem with multi-mode perturbations. The mathematical expression of the initial perturbations is the same as (3.9). In this problem, The computational domain is Cartesian and measures ![]() $x\times y\times z=2.8{\rm \pi} \times 2{\rm \pi} \times 2 {\rm \pi}\ {\rm m}^{3}$. The initial wavelength is
$x\times y\times z=2.8{\rm \pi} \times 2{\rm \pi} \times 2 {\rm \pi}\ {\rm m}^{3}$. The initial wavelength is ![]() $\lambda \in [L/8,L/4]$, where
$\lambda \in [L/8,L/4]$, where ![]() $L$ is the length of the cross-section. The distribution of energy is
$L$ is the length of the cross-section. The distribution of energy is ![]() $P(k)=Ck^{0}$. The total standard deviation is
$P(k)=Ck^{0}$. The total standard deviation is ![]() $\sigma =\sqrt {\int P(k)\,{\rm d}k}= 0.1\lambda _{min}$, where
$\sigma =\sqrt {\int P(k)\,{\rm d}k}= 0.1\lambda _{min}$, where ![]() $\lambda _{min}=L/8$. The initial pre-mixing width is
$\lambda _{min}=L/8$. The initial pre-mixing width is ![]() $\delta =L/32$. The initial Atwood number is
$\delta =L/32$. The initial Atwood number is ![]() $A=0.5$. The shock wave propagates from the light fluid to the heavy fluid. As a result, the numerical results of all algorithms converge with resolution
$A=0.5$. The shock wave propagates from the light fluid to the heavy fluid. As a result, the numerical results of all algorithms converge with resolution ![]() $\Delta x=L/256$. The same problem is simulated with APEX in the present work. The base resolution is
$\Delta x=L/256$. The same problem is simulated with APEX in the present work. The base resolution is ![]() $23\times 16\times 16$, on which four additional levels of refinement with a factor of 2 are applied. The finest resolution is therefore
$23\times 16\times 16$, on which four additional levels of refinement with a factor of 2 are applied. The finest resolution is therefore ![]() $364\times 256\times 256$. In the work of Thornber et al. (Reference Thornber2017), the integral mixing width is defined as
$364\times 256\times 256$. In the work of Thornber et al. (Reference Thornber2017), the integral mixing width is defined as
where ![]() $\bar {Z}_{1,2}$ indicates the
$\bar {Z}_{1,2}$ indicates the ![]() $(y,z)$ plane (the spanwise directions) averaged volume fraction of species 1, 2, where species 1 is the heavy gas. The results obtained by APEX show good agreement with those of Thornber et al. (Reference Thornber2017), as shown in figure 17, which proves the reliability of APEX for simulating the interfacial instability problem.
$(y,z)$ plane (the spanwise directions) averaged volume fraction of species 1, 2, where species 1 is the heavy gas. The results obtained by APEX show good agreement with those of Thornber et al. (Reference Thornber2017), as shown in figure 17, which proves the reliability of APEX for simulating the interfacial instability problem.

Figure 17. Comparison of the mixing-zone width with the work of Thornber et al. (Reference Thornber2017).
Appendix B. Grid/computation domain convergence analysis
B.1. Grid
The grid convergence is discussed in this subsection. All of the set-ups are summarized in tables 4 and 5. Figures 18, 19 and 20 present the results of the grid convergence test for three cases with different initial perturbations. Good grid convergence is shown between the cases G2048 and G4096 in 2-D simulations. The grid convergence is also shown between the cases G512-4 and G512-5 in 3-D simulations, which indicates that the result of the mixing width is not sensible for our finest grid set-up. Therefore, we present the numerical results of the cases G4096 and G512-5 in the present work.

Figure 18. For the case SP8, grid convergence test for the mixing width in (a) the planar geometry and (b) the cylindrical geometry.

Figure 19. For the case SP16, grid convergence test for the mixing width in (a) the planar geometry and (b) the cylindrical geometry.

Figure 20. For the cases MP16–48, grid convergence test for the mixing width in (a) the planar geometry and (b) the cylindrical geometry.
Table 4. For the cylindrical cases, the grid set-ups for each case.

Table 5. For the planar cases, the grid set-ups for each case.
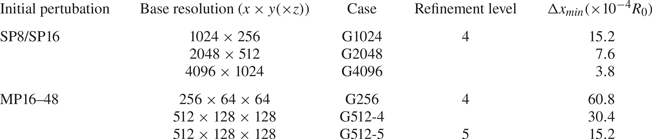
B.2. Computational domain
The fluid quantities behind the cylindrical shock wave are not uniform with radius. For the convergent geometry, at the boundaries of the ![]() $(x,y)$ plane, the gradients of the fluid quantities are not zero. Hence if the outflow boundary condition is applied at the
$(x,y)$ plane, the gradients of the fluid quantities are not zero. Hence if the outflow boundary condition is applied at the ![]() $(x,y)$ plane, the perturbations at the boundaries will propagate to the interior and may influence the numerical results. Therefore, it is necessary to verify the convergence about the computation domain width to make sure that the perturbation does not propagate into the zone in which we are interested. As the convergent shock wave configuration is identical in all the cylindrical cases, here we take the case SP16 as an example. Figure 21 verifies the convergence about the computation domain width. We tested domain widths
$(x,y)$ plane, the perturbations at the boundaries will propagate to the interior and may influence the numerical results. Therefore, it is necessary to verify the convergence about the computation domain width to make sure that the perturbation does not propagate into the zone in which we are interested. As the convergent shock wave configuration is identical in all the cylindrical cases, here we take the case SP16 as an example. Figure 21 verifies the convergence about the computation domain width. We tested domain widths ![]() $R_{ext}={\rm \pi} R$,
$R_{ext}={\rm \pi} R$, ![]() $2{\rm \pi} R$,
$2{\rm \pi} R$, ![]() $4{\rm \pi} R$ and
$4{\rm \pi} R$ and ![]() $8{\rm \pi} R$ with the finest resolution and refinement level the same as G4096. The results show a very good convergence. To avoid the influence of the boundary and to save computation resource at the same time, we choose
$8{\rm \pi} R$ with the finest resolution and refinement level the same as G4096. The results show a very good convergence. To avoid the influence of the boundary and to save computation resource at the same time, we choose ![]() $R_{ext}=4{\rm \pi} R$ in the present work.
$R_{ext}=4{\rm \pi} R$ in the present work.

Figure 21. For the case SP16 in the cylindrical geometry, domain width convergence test for the mixing width.