3.1 Origin of Newtonian and viscoelastic nonlinear Tollmien–Schlichting attractors

In Newtonian flow, a family of nonlinear TS waves bifurcates subcritically from the laminar branch at $Re\approx 5772$ with $L_{x}\approx 2\unicode[STIX]{x03C0}/1.02\approx 6.15$. The lower limit of the parameter regime for which this solution family exists is $Re\approx 2800$, $L_{x}\approx 2\unicode[STIX]{x03C0}/1.3\approx 4.83$ (Jiménez Reference Jiménez1990). Furthermore, in prior work on EIT (Shekar et al. Reference Shekar, McMullen, Wang, McKeon and Graham2019), as well as in more recent simulations in long 2-D domains, a strong peak in the spatial spectrum is found at $L_{x}\approx 5$. Based on these observations, all of the results presented in this study will be at $Re=3000$, $L_{x}=5$. (At $L_{x}=5$, Newtonian channel flow is linearly stable at all $Re$.) In the Newtonian limit at these parameters, there are upper and lower branch solutions (which merge in a saddle-node bifurcation as $Re$ is lowered); the upper branch travelling wave solution is linearly stable with respect to 2-D perturbations and is thus easily computed via DNS. We call this solution branch, including its viscoelastic extension, the Newtonian nonlinear Tollmien–Schlichting attractor (NNTSA). (The word ‘attractor’ is chosen rather than ‘wave’ because, depending on parameters, one can observe a pure travelling wave state or one with periodic or non-periodic modulations.)

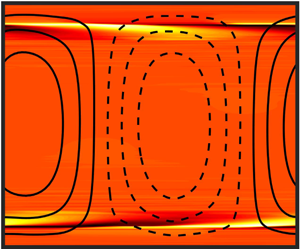
On increasing $Wi$, the self-sustained nonlinear viscoelastic TS wave at $Re=3000$ develops sheets of high polymer stretch that start out from near the wall. These observations are evidence of the capability of nonlinear TS critical layer mechanisms in generating sheets of polymer stretch. Figure 1 illustrates this point with a snapshot of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ on the NNTSA branch at $Wi=3$. The sheets originate in the nonlinear Kelvin cat’s eye kinematics of TS waves at finite amplitude, as detailed in Shekar et al. (Reference Shekar, McMullen, Wang, McKeon and Graham2019). The NNTSA continues to display wall-normal velocity fluctuations that extend across the channel centreline – a signature of TS kinematics. Some of the observations made in Shekar et al. (Reference Shekar, McMullen, Wang, McKeon and Graham2019) are repeated here for completeness, as they form the background for the new results of the present study.

Figure 1. Structure of NNTSA at $Re=3000$, $\mathit{Wi}=3$. Streamlines (blue) in a reference frame moving at the wave speed $c=0.39$ are superimposed on colour contours of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$, where the hat $\hat{\,}$ denotes deviations from the laminar state. Blue dots indicate the locations of hyperbolic stagnation points (in the travelling frame). Black contour lines of $\hat{v}$ are also shown. For $\hat{v}$, dashed $=$ negative and solid $=$ positive.

Figure 2. (a) Bifurcation diagram showing the evolution of the space- and time-averaged wall shear rate with $Wi$ for the 2-D nonlinear NNTSA and EIT branches at $Re=3000$, $L_{x}=5$. Point (a) corresponds to the structure shown in figure 1. Labels ‘L’ and ‘VNTSA’ indicate that initial conditions starting at the arrows evolve to laminar or VNTSA states, respectively. (b) Bifurcation diagram of the $L_{\infty }$-norm of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ with $\mathit{Wi}$ for VNTSA and EIT. Points (a)–(f) correspond to structures shown later in figure 6. The label ‘EIT’ indicates that initial conditions evolve to EIT. In both panels, dashed lines correspond to the unstable solution branches obtained from edge tracking.

At the parameters chosen, the solution branch originating in the self-sustained Newtonian TS wave bifurcates to a periodic orbit at $\mathit{Wi}\approx 3.5$ (cf. Lee & Zaki Reference Lee and Zaki2017) before turning back into a travelling wave and losing existence beyond $\mathit{Wi}=3.875$, evidently in a saddle-node bifurcation yielding a lower branch TS wave solution that becomes the Newtonian solution as $\mathit{Wi}\rightarrow 0$. Consistent with a saddle-node bifurcation, if the solution at $\mathit{Wi}=3.875$ is used as an initial condition for a simulation at slightly higher $\mathit{Wi}$, the flow laminarizes. This bifurcation scenario is shown in figure 2(a) in terms of average wall shear rate versus $\mathit{Wi}$. The unstable lower branch (dashed blue) was found using edge tracking (Zammert & Eckhardt Reference Zammert and Eckhardt2014) between NNTSA and laminar solutions at a given $\mathit{Wi}$. A bisection technique was used to arrive at arbitrarily close initial conditions that are on either side of the edge. DNS trajectories starting from such points stay on the edge for a while before diverging to NNTSA and laminar.

As shown in Shekar et al. (Reference Shekar, McMullen, Wang, McKeon and Graham2019), if $\mathit{Wi}$ is large, sufficiently energetic initial conditions lead to 2-D EIT. Figure 2(a) also shows the mean wall shear rate for the EIT solution branch, which loses existence at finite amplitude when $\mathit{Wi}\lesssim 13$. The bifurcation underlying this transition is presumably also of saddle-node form.

The central observation of the present paper arises from considering what happens just below the onset of the EIT regime at $\mathit{Wi}\approx 13$. We do this by using a velocity and stress field from EIT at $\mathit{Wi}=13$ as an initial condition for a run at $\mathit{Wi}=12$. This initial condition persists as a slowly decaying form of EIT for hundreds of time units (TU), consistent with behaviour just beyond a saddle-node bifurcation. As time increases further, the structure continues to decay, but does not ultimately reach the laminar state. Instead, it evolves to a non-trivial attractor state that is very nearly a travelling wave, and in particular strongly resembles the linear TS mode at these parameters. We call this new state the viscoelastic nonlinear Tollmien–Schlichting attractor (VNTSA).

3.2 Viscoelastic nonlinear Tollmien–Schlichting attractor

To elaborate on the relationship between the VNTSA and the linear TS mode, we describe results at $Re=3000$, $\mathit{Wi}=13$, $L_{x}=5$, i.e. close to the point where the 2-D EIT branch first comes into existence as shown in the bifurcation diagram (figure 2a). EIT and the VNTSA are coexisting attractors at these parameter values. Figure 3 shows the evolution of the $L_{\infty }$-norm of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ starting from an initial condition consisting of the laminar state plus some amplitude $\unicode[STIX]{x1D716}$ of the linear TS mode for this parameter set. This mode, with $\unicode[STIX]{x1D716}=1$, is shown in figure 4(a). The structure of the velocity field is virtually unchanged from the Newtonian case and the polymer conformations are strongly localized to the critical layer positions $y=\pm 0.82$. Sufficiently small perturbations, e.g. $\unicode[STIX]{x1D716}=1$, decay to the laminar state, as they must since that state is linearly stable. However, for larger perturbations, $\unicode[STIX]{x1D716}=5$, 10 and 100, where nonlinear mechanisms play a role, $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ settles to a finite value corresponding to the VNTSA. The initial condition $\unicode[STIX]{x1D716}=5$ that starts from below the VNTSA in $\Vert \hat{\unicode[STIX]{x1D6FC}}_{xx}\Vert _{\infty }$ shows an initial growth phase before saturating onto the VNTSA, whereas $\unicode[STIX]{x1D716}=10$ and 100 relax onto the VNTSA from above.

Figure 3. Time evolution of the $L_{\infty }$-norm of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ for $Re=3000$, $\mathit{Wi}=13$, $L_{x}=5$, starting from an initial condition of $\text{laminar state}~+~\unicode[STIX]{x1D716}\;\times \;\text{TS-mode}$. Dashed lines correspond to linearized runs starting from the same initial conditions for $\unicode[STIX]{x1D716}=10$ and 100.

For comparison, the dashed lines in figure 3 show the linearized evolution starting from the same initial conditions; these all decay to laminar, illustrating the role of nonlinearity in the transition to the VNTSA. This state is robust: initial perturbation amplitudes over a wide range will evolve to it. However, initial conditions with very large magnitudes (e.g. $\unicode[STIX]{x1D716}=6000$) evolve to EIT: as noted above, both EIT and VNTSA are attractors at the chosen parameters (as is the laminar state).

We have also used initial conditions of the laminar state plus velocity perturbations somewhat similar to the ones used by Page & Zaki (Reference Page and Zaki2015). These perturbations satisfied incompressibility and were sinusoidal in nature, with streamwise and wall-normal periods equal to the domain size. When appropriate magnitudes are used, transient growth of polymer stress followed by a decay to the VNTSA is observed.

In 3-D channel flow simulations of Oldroyd-B fluids at $Re=3000$, $\mathit{Wi}=4{-}6$, $\unicode[STIX]{x1D6FD}=0.9$, Min, Choi & Yoo (Reference Min, Choi and Yoo2003) observe a transient (${\sim}500$  TU) before an abrupt jump to the fully developed state. These observations were made starting from turbulent Newtonian initial conditions. In the present work, we used an average of approximately 2500 TU of data, and in some cases more than 5000 TU to calculate the statistics, and have observed no similar transition in any simulations of VNTSA in the parameter space considered here.

Figure 4(b) is a snapshot showing the typical fluctuation structure of the VNTSA at $\mathit{Wi}=13$. The streamwise conformation $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ has tilted sheets highly localized near $y=\pm 0.82$, and contours of wall-normal velocity $\hat{v}$ span the entire channel. This structure bears strong resemblance to the TS mode shown in figure 4(a). The VNTSA is thus a weakly nonlinear self-sustaining state whose primary structure is the viscoelastic TS mode. We elaborate in the following section on the linear TS mode and its connections to the VNTSA.

Figure 4. (a) Structure of the linear TS mode at $Re=3000$, $\mathit{Wi}=13$, $L_{x}=5$. Magnitude of the eigenmode is arbitrary and values shown here correspond to $\unicode[STIX]{x1D716}=1$. (b) Snapshot of the fluctuation structure of the VNTSA at $Re=3000$, $\mathit{Wi}=13$. (c,d) The $kL_{x}/2\unicode[STIX]{x03C0}=1,2$ components, respectively, of the snapshot shown in (b). Shown are contour lines of $\hat{v}$ superimposed on colour contours of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$.

In the VNTSA state, the velocity fluctuations are very weak, and the mean wall shear rate displays a very small change from laminar. This can be understood on the grounds that changes of the mean wall shear rate correspond to fluctuations with $k=0$, which arise only due to nonlinear interactions. Since the primary velocity structure is very weak, the nonlinear effects will be even weaker. To illustrate nonlinear effects, figures 4(c) and 4(d), respectively, show the $kL_{x}/2\unicode[STIX]{x03C0}=1$ and $2$, spatial Fourier components of the snapshot shown in figure 4(b). Figure 4(c) closely resembles the TS mode, with a slight symmetry breaking across the centreline $y=0$. The structure at $kL_{x}/2\unicode[STIX]{x03C0}=2$ also displays polymer stress fluctuations localized around the critical layer position, an observation that also holds for higher wavenumbers.

Having established the structure of the flow on the VNTSA branch, we now illustrate the bifurcation scenario of this solution branch by continuing in $\mathit{Wi}$. The VNTSA branch loses existence at finite amplitude (i.e. in a saddle-node bifurcation) for $\mathit{Wi}\lesssim 6$, as we have confirmed both by using the $\mathit{Wi}=6$ solution as an initial condition for simulations at lower $\mathit{Wi}$ and by running simulations starting from the laminar state perturbed by the TS mode with small $\unicode[STIX]{x1D716}$. For $Wi<6$ all these initial conditions decay to laminar. On increasing $\mathit{Wi}$, the VNTSA branch seems to lose existence beyond $\mathit{Wi}\approx 49$, and initial conditions that land on the VNTSA for $\mathit{Wi}=49$ evolve to EIT at $\mathit{Wi}=50$. Due to its weak nature in relation to EIT, the bifurcation scenario associated with the two solution branches is shown in figure 2(b) in a log scale using the $L_{\infty }$-norm of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ as the amplitude measure. The EIT solutions in figure 2(a) are depicted again in figure 2(b) as the upper stable branch (solid red) with this measure.

Since the VNTSA and EIT are both stable states, we were also able to perform edge tracking to find unstable solutions intermediate between these two states. Five $\mathit{Wi}$ values (13, 15, 20, 40 and 45) were studied. Repeated bisections were performed until trajectories stayed on the edge for an average of 300 TU. The red dashed line in figure 2(a,b) indicates solutions (all time-dependent) on this intermediate branch. The magnitude of fluctuations along this branch monotonically decreases on increasing $\mathit{Wi}$, displaying values close to EIT at $\mathit{Wi}=13$ and values to close to VNTSA at $\mathit{Wi}=45$. Furthermore, this branch also displays fluctuations that resemble the viscoelastic TS mode, as illustrated below. A more detailed link between VNTSA and EIT might be established through numerical continuations of underlying travelling wave solutions; this is a topic of future endeavours.

To complete this discussion, we note that the bifurcation scenario we observe implies the existence of an edge between the VNTSA and the laminar state, which could in principle also be found using edge tracking. However, the weak nature of the VNTSA implies that this edge would be even weaker, thus making this a challenging task.

Figure 5(a) shows the fluctuation structure of the VNTSA at $\mathit{Wi}=8$, close to the point where it first comes into existence. The structure closely resembles the TS mode and does not change appreciably with time. The flow is almost a pure nonlinear travelling wave with some weak non-periodicity, as indicated in the power density plot of the wall-normal velocity at position $(0,0.825)$ shown in figure 5(b). The spectrum is mainly composed of the dominant TS mode frequency and its higher harmonics. The dynamics and structures get more complicated as $\mathit{Wi}$ increases. Figure 5(c) shows a typical snapshot at $\mathit{Wi}=20$, which clearly is more complex than a TS mode. However, at this $\mathit{Wi}$, the VNTSA still intermittently displays clear TS-like structures, such as the snapshot in figure 5(d).

We now turn to the flow structures at various positions on the bifurcation diagram, figure 2(b). Figures 6(a), 6(b) and 6(c) are representative snapshots of VNTSA, the intermediate branch and EIT, respectively, at $\mathit{Wi}=13$, i.e. near the loss of existence of EIT. As detailed in the description of figure 4 and shown again in figure 6(a), VNTSA exhibits a weak structure that strongly resembles the viscoelastic TS mode. At this low $\mathit{Wi}$, the intermediate branch (figure 6b) displays a much stronger fluctuation structure, of the same order of magnitude as the structures seen at EIT (figure 6c). Moreover, the intermediate branch exhibits overlapping sheets of polymer stretch (especially on the bottom side of the channel at the time instant shown) that strongly resemble those seen at EIT.

Figure 5. (a) Fluctuation structure of the VNTSA and (b) power spectral density (PSD) of $v$ at position $(0,0.825)$ at $Re=3000$, $\mathit{Wi}=8$. Frequencies corresponding to the TS mode (red dashed) and its higher harmonics (black dashed) are also shown. (c,d) Snapshots of the VNTSA structure for $Re=3000$, $\mathit{Wi}=20$. Contour plots follow the same format as in figure 4.

Figure 6(d–f) show the structures at $\mathit{Wi}=45$, close to the point where the intermediate branch seems to turn around to merge with VNTSA. The fluctuations on the intermediate branch (figure 6e) decrease in magnitude on increasing $\mathit{Wi}$, and at $\mathit{Wi}=45$ become comparable to those on the VNTSA branch as shown in figure 6(d). Furthermore, the structure on this branch goes from displaying overlapping sheets of polymer stretch at low $\mathit{Wi}$ to localized striations at high $\mathit{Wi}$ similar to those of VNTSA. In sharp contrast to the localized structures just described, EIT (figure 6f) continues to display sheets of polymer stretch, which get stronger on increasing $\mathit{Wi}$. All three solution branches continue to intermittently display wall-normal velocity fluctuations that resemble the TS mode.

3.3 Linear analyses

In this section we elaborate on the linearized problem and its connection to the attractors described above using linear stability and resolvent analyses. The spectrum corresponding to disturbances with wavelengths equal to the DNS box size, i.e. $k=2\unicode[STIX]{x03C0}/5$, has a least stable eigenvalue at $c\approx 0.32{-}0.010\text{i}$, and the associated eigenfunction is the viscoelastic extension of the TS mode. For low values of $\mathit{Wi}$, the mode is less stable than its Newtonian counterpart, while for $\mathit{Wi}\gtrsim 2$, it becomes more stable with increasing elasticity. This non-monotonic behaviour has been reported by Zhang et al. (Reference Zhang, Lashgari, Zaki and Brandt2013), who attribute it to viscoelastic modification of the phase difference between $u$ and $v$. Nevertheless, over the range of $\mathit{Wi}$ considered here, the eigenvalue varies by less than 1 % of the Newtonian value. The linear stability of the laminar state in this range of $\mathit{Wi}$, and the very weak dependence of $c$ on $\mathit{Wi}$, continues up to at least $Re=6000$, confirming the observations in § 3.2 that finite-amplitude disturbances are required to trigger transition to EIT or the VNTSA. However, linear instabilities not related to the TS mode have been found in other regions of parameter space (Garg et al. Reference Garg, Chaudhary, Khalid, Shankar and Subramanian2018; Chaudhary et al. Reference Chaudhary, Garg, Shankar and Subramanian2019), implying the possibility of different attractor families in those regions.

Figure 6. (a–c) Snapshots of VNTSA, intermediate branch and EIT respectively at $\mathit{Wi}=13$. (d–f) Snapshots of the same solution branches at $\mathit{Wi}=45$. Deviations from the laminar base state shown as earlier. Colour scales are intentionally centred about 0 for comparison purposes.

Figure 7. (a) Solid blue line: ratio of peak amplitudes of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ and $\hat{v}$ for the linear TS mode as a function of $\mathit{Wi}$. Circles: amplitude ratio for the VNTSA. (b) Magnitude of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ for the linear TS mode for several values of $\mathit{Wi}$ in the range $[1,20]$. Darker lines indicate higher values of $\mathit{Wi}$. The thick red line shows the averaged magnitude of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ from the VNTSA for $\mathit{Wi}=13$. For comparison, the linear TS mode profile for the same $\mathit{Wi}$ is shown in blue, and the vertical dashed line marks the critical layer location $y_{c}=0.825$. The arrow indicates the value of $\mathit{Wi}$ corresponding to the arrow in (a). (c) First two singular values of the resolvent operator for $k$ and $c$ corresponding to the linear TS mode.

A measure of the relative importance of the conformation tensor and velocity disturbances is the ratio of the peak amplitudes of $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ (the largest component of the conformation tensor) and $\hat{v}$. This ratio is shown in figure 7(a). Two distinct regimes are apparent, with the transition between the two occurring at $\mathit{Wi}\approx 3.1$. The low-$\mathit{Wi}$ regime scales as $\mathit{Wi}^{2}$, which is the same scaling as in linear shear flow. The amplitude ratio above the change in slope does not exhibit power-law scaling. The change in slope at $\mathit{Wi}\approx 3.1$ can be understood by examining the $\hat{\unicode[STIX]{x1D6FC}}_{xx}$ mode shapes, the magnitudes of which are plotted in figure 7(b) for several values of $\mathit{Wi}$ in the range shown in figure 7(a). For small $\mathit{Wi}$, the disturbance is largest at the wall and decays rapidly away from it. Therefore, the $\mathit{Wi}^{2}$ scaling in this regime can be explained by the fact that the leading-order approximation of the base flow very near the wall is simple shear. As $\mathit{Wi}$ increases, this value decreases, while a new local maximum emerges and grows, becoming the global maximum just above $\mathit{Wi}=3$; the arrow in the figure indicates the profile where this occurs. Upon further increase in $\mathit{Wi}$, the maximum gradually shifts away from the wall, and the modes become increasingly localized around the location of the critical layer $y_{c}$, at which the real part of the wave speed equals the base flow velocity. The critical layer for $\mathit{Wi}=13$ is indicated by the vertical dashed line. This suggests that a critical layer mechanism is responsible for the change in scaling at large $\mathit{Wi}$, though at present we do not understand the specific origin of this result. Interestingly, the $\mathit{Wi}$ at which the VNTSA comes into existence is only slightly larger than that at which the transition to critical layer scaling occurs.

Also shown in figure 7(a) is the amplitude ratio computed from the VNTSA for several values of $\mathit{Wi}$. Excellent agreement between the linear and nonlinear results quantitatively reinforces the TS-mode-like nature of the VNTSA. Additionally, the profile of $|\hat{\unicode[STIX]{x1D6FC}}_{xx}|$, averaged in the streamwise direction and over many snapshots, for the VNTSA at $\mathit{Wi}=13$ is shown by the thick red line in figure 7(b), and the blue line highlights the linear mode for the same $\mathit{Wi}$. The VNTSA profile exhibits the same localization, and the location of the peak value is in close agreement with the critical layer location.

Figure 7(c) shows the first two singular values of the resolvent operator for $k$ and $c$ corresponding to the linear TS mode. Shekar et al. (Reference Shekar, McMullen, Wang, McKeon and Graham2019) showed that such modes are the most-amplified 2-D disturbances in this parameter regime and that the leading response mode is nearly identical to the TS eigenmode; for this reason the resolvent modes are not plotted separately. The substantial increase in the leading singular value with $\mathit{Wi}$ indicates that this amplification becomes much stronger with increasing elasticity, and consequently that considerably smaller disturbances may be sufficient to trigger self-sustaining nonlinear mechanisms. Further, the symmetry of the flow geometry about $y=0$ means that resolvent modes typically come in pairs having similar amplification, with one mode having a symmetric $\hat{v}$ response and the other having an antisymmetric $\hat{v}$ response. However, the growing separation between the first and second singular values with increasing $\mathit{Wi}$ indicates that this pairing is broken by elasticity, and that the symmetry exhibited by the TS mode is preferred in terms of linear amplification.

3.4 Broader context: flow geometry and dimensionality

The results described above are limited to 2-D channel flow. Here we describe how they may be viewed in the broader context of turbulent drag reduction, first with regard to how they may relate to the pipe flow geometry and then in the full context of 3-D turbulence.

As noted in the introduction, EIT with very similar features has been observed in both channel and pipe flows. A natural question, then, is to what extent the above channel flow results are relevant to the pipe flow case. While it is true, of course, that there is no linear instability of Newtonian pipe flow, the general structures of the linear stability problem for the pipe and channel are very similar. The A, P and S (wall, centre, strongly decaying) families of modes for the channel flow problem also arise in the pipe, as illustrated in figures 4.18 and 4.19 of Drazin & Reid (Reference Drazin and Reid2004). (In channel flow, one of the wall modes, the TS mode, goes unstable.) Additionally, critical layers are a strong source of linear amplification in pipe flow, as they are in channel flow (McKeon & Sharma Reference McKeon and Sharma2010).

Turning to nonlinear behaviour in Newtonian pipe flow, an important difference from channel flow is the apparent absence of subcritical travelling wave solutions that are analogous to the nonlinear TS waves (Patera & Orszag Reference Patera and Orszag1981). That is, in pipe flow there is no nonlinear solution branch that corresponds to the NNTSA described above. Given this observation, one might wonder whether the results presented here for viscoelastic channel flow are relevant for pipe flow.

To address this issue, we make the following remarks. The key observation of the present work is that the TS mode is nonlinearly excited by viscoelasticity. The resulting solution branch, the VNTSA, is not connected, at least in this part of parameter space under consideration, to the Newtonian branch, indicating that the nonlinear mechanism that sustains it is distinct from the nonlinear mechanisms sustaining the Newtonian branch. So the absence of a Newtonian mechanism for nonlinear sustainment of TS-like travelling waves does not imply the absence of a viscoelastic mechanism. Furthermore, as noted in the introduction, pipe flow simulations of EIT (Lopez et al. Reference Lopez, Choueiri and Hof2019) display essentially 2-D velocity fluctuations localized near the wall that are similar to those reported in channel flow. These are precisely what would be expected in pipe flow based on the observations we report here for channel flow, i.e. a critical layer mechanism associated with excitation of a TS-like mode. At the same time, in more strongly viscoelastic regimes, Garg et al. (Reference Garg, Chaudhary, Khalid, Shankar and Subramanian2018) and Chaudhary et al. (Reference Chaudhary, Garg, Subramanian and Shankar2020) have found a centre mode instability for pipe flow; for the Oldroyd-B model with $Re=3500$, $\unicode[STIX]{x1D6FD}=0.9$, instability occurs when $176.9<\mathit{Wi}<4783.6$. A related instability might be present in the channel flow problem. These results open up the possibility that other states unrelated to the nonlinear excitation of a wall mode may also play a role at EIT in both channels and pipes, especially at high $\mathit{Wi}$.

We now turn to the topic of how the present results are related to the fully 3-D context, beginning with a brief overview of Newtonian near-wall turbulence.

Newtonian turbulence is, of course, strongly 3-D, with the dominant near-wall structure composed of coherent wavy streamwise vortices. In all of the canonical wall-bounded shear flow geometries (pipe flow, channel flow, plane shear (Couette) flow), families of 3-D nonlinear travelling wave solutions to the Navier–Stokes equations have been discovered (Waleffe Reference Waleffe1998, Reference Waleffe2001, Reference Waleffe2003; Hof et al. Reference Hof, van Doorne, Westerweel, Nieuwstadt, Faisst, Eckhardt, Wedin, Kerswell and Waleffe2004; Wedin & Kerswell Reference Wedin and Kerswell2004; Wang, Gibson & Waleffe Reference Wang, Gibson and Waleffe2007; Eckhardt et al. Reference Eckhardt, Schneider, Hof and Westerweel2007, Reference Eckhardt, Faisst, Schmiegel and Schneider2008; Duguet, Willis & Kerswell Reference Duguet, Willis and Kerswell2008b). These solutions are often denoted ‘exact coherent states’ (ECS), and their predominant structure is very similar to that observed in wall turbulence: a mean shear and wavy streamwise vortices. (In particular, they bear no structural resemblance to TS waves and do not arise from a linear instability of the laminar state.) Related, but more complex, states have been found as well, which are not pure travelling waves but rather ‘relative periodic orbits’ that are time-periodic modulo a phase shift in one of the translation-invariant spatial directions (Duguet, Pringle & Kerswell Reference Duguet, Pringle and Kerswell2008a). In minimal domains at Reynolds numbers near transition, the turbulent dynamics has been found to be organized, at least in part, around these solutions (see e.g. Gibson, Halcrow & Cvitanović Reference Gibson, Halcrow and Cvitanović2008, Kawahara, Uhlmann & van Veen Reference Kawahara, Uhlmann and van Veen2012, Park & Graham Reference Park and Graham2015).

It has long been known that one of the effects of viscoelasticity on wall turbulence is to weaken and broaden the near-wall streamwise vortices (Kim et al. Reference Kim, Li, Sureshkumar, Balachandar and Adrian2007; White & Mungal Reference White and Mungal2008). A number of studies have addressed this observation by investigating the effect of viscoelasticity on ECS, which, as noted, capture this streamwise vortex structure (Stone, Waleffe & Graham Reference Stone, Waleffe and Graham2002; Stone & Graham Reference Stone and Graham2003; Stone et al. Reference Stone, Roy, Larson, Waleffe and Graham2004; Li, Stone & Graham Reference Li, Stone and Graham2005; Li, Xi & Graham Reference Li, Xi and Graham2006; Li & Graham Reference Li and Graham2007). Indeed, the effect of viscoelasticity is to weaken these structures; the polymer stresses directly counteract the streamwise vortices.

In particular, Li & Graham (Reference Li and Graham2007) studied the bifurcation scenario for a particular family of channel flow ECS (Waleffe Reference Waleffe1998) in a parameter regime very close to that considered here. At $Re=1500$, this ECS family is sufficiently weakened by viscoelasticity to lose existence at $\mathit{Wi}\approx 16$, somewhat above the value $\mathit{Wi}\approx 10$ beyond which Newtonian turbulence cannot self-sustain in the DNS study of Shekar et al. (Reference Shekar, McMullen, Wang, McKeon and Graham2019). (This discrepancy is consistent with what we know about transition in the Newtonian case: channel flow turbulence is self-sustaining above $Re\approx 1000$, while ECS can exist in that case down to $Re\approx 660$ (Shekar & Graham Reference Shekar and Graham2018).) Extrapolating slightly from the results of Li & Graham (Reference Li and Graham2007), one can estimate that, at $Re=3000$, this ECS family loses existence at $\mathit{Wi}\approx 25$.

Given that, in the present 2-D work with $Re=3000$, EIT is found at $\mathit{Wi}\gtrsim 13$ and the VNTSA above at $\mathit{Wi}\gtrsim 6$, there would appear to be a regime $6\lesssim \mathit{Wi}\lesssim 25$ in which both 2-D and 3-D structures may exist and interact. Furthermore, existing results on the effect of viscoelasticity on ECS are limited to one ECS family, and there certainly may be others that can persist to higher $\mathit{Wi}$. Consistent with this analysis, a number of studies have reported near-wall EIT-like spanwise-oriented structures, with 3-D quasi-streamwise vortices further away from the wall (Dubief et al. Reference Dubief, Terrapon and Soria2013; Choueiri et al. Reference Choueiri, Lopez and Hof2018; Pereira, Thompson & Mompean Reference Pereira, Thompson and Mompean2019a,Reference Pereira, Thompson and Mompeanb). How the 2-D and 3-D structures interact is an important topic for future work.