In this work, we review some properties of twisted partial actions of Hopf algebras on unital algebras and give necessary and sufficient conditions for a twisted partial action to have a globalization. We also elaborate a series of examples.

We provide a comprehensive study of the function $h=h(q)$ defined by

$$\begin{eqnarray}h=q\mathop{\prod }_{j=1}^{\infty }\frac{(1-q^{12j-1})(1-q^{12j-11})}{(1-q^{12j-5})(1-q^{12j-7})}\end{eqnarray}$$

$k=k(q)$

$$\begin{eqnarray}k=q\mathop{\prod }_{j=1}^{\infty }\frac{(1-q^{10j-1})(1-q^{10j-2})(1-q^{10j-8})(1-q^{10j-9})}{(1-q^{10j-3})(1-q^{10j-4})(1-q^{10j-6})(1-q^{10j-7})}.\end{eqnarray}$$

In this erratum we correct a mistake in Ho [‘On differential characteristic classes’, J. Aust. Math. Soc.99(1) (2015), 30–47].

We investigate the function $d_{\mathbf{A}}(n)$, which gives the size of a least size generating set for $\mathbf{A}^{n}$.

In an earlier paper, Romanowska, Ślusarski and Smith described a duality between the category of polytopes (finitely generated real convex sets considered as barycentric algebras) and a certain category of intersections of hypercubes, considered as barycentric algebras with additional constant operations. The present paper provides an extension of this duality to a much more general class of so-called quasipolytopes, that is, convex sets with polytopes as closures. The dual spaces of quasipolytopes are Płonka sums of open polytopes, which are considered as barycentric algebras with some additional operations. In constructing this duality, we use several known and new dualities: the Hofmann–Mislove–Stralka duality for semilattices; the Romanowska–Ślusarski–Smith duality for polytopes; a new duality for open polytopes; and a new duality for injective Płonka sums of polytopes.

In the ambient space of a semidirect product 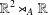$\mathbb{R}^{2}\rtimes _{A}\mathbb{R}$, we consider a connected domain ${\rm\Omega}\subseteq \mathbb{R}^{2}\rtimes _{A}\{0\}$. Given a function 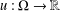$u:{\rm\Omega}\rightarrow \mathbb{R}$, its ${\it\pi}$-graph is 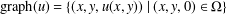$\text{graph}(u)=\{(x,y,u(x,y))\mid (x,y,0)\in {\rm\Omega}\}$. In this paper we study the partial differential equation that $u$ must satisfy so that $\text{graph}(u)$ has prescribed mean curvature 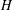$H$. Using techniques from quasilinear elliptic equations we prove that if a ${\it\pi}$-graph has a nonnegative mean curvature function, then it satisfies some uniform height estimates that depend on ${\rm\Omega}$ and on the supremum the function attains on the boundary of 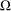${\rm\Omega}$. When 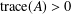$\text{trace}(A)>0$, we prove that the oscillation of a minimal graph, assuming the same constant value $n$ along the boundary, tends to zero when 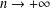$n\rightarrow +\infty$ and goes to 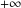$+\infty$ if 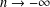$n\rightarrow -\infty$. Furthermore, we use these estimates, allied with techniques from Killing graphs, to prove the existence of minimal ${\it\pi}$-graphs assuming the value zero along a piecewise smooth curve ${\it\gamma}$ with endpoints 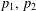$p_{1},\,p_{2}$ and having as boundary 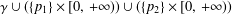${\it\gamma}\cup (\{p_{1}\}\times [0,\,+\infty ))\cup (\{p_{2}\}\times [0,\,+\infty ))$.