2. ANALYSIS

2.1. Propagation

Consider the coaxial propagation of coherent circularly polarized LG and Gaussian beams in a homogeneous plasma with its electric vector polarized along the z-axis; the laser beams are considered to be of same frequency (ω). It is convenient to express the electric field vectors E_l and E_g associated with these beams in cylindrical coordinate system with azimuthal symmetry and can be expressed as

(2)$${\bf E}_{\bi l} \lpar r\comma \; z\comma \; {\rm \theta} _l \rpar = A_l \lpar r\comma \; z\rpar \lpar \hat x + i\hat y\rpar \, \hbox{exp}\, [i\lpar kz - {\rm \omega} t - l{\rm \theta} _l \rpar ] $$

and

(3)$${\bf E}_{\bi g} \lpar r\comma \; z\rpar = A_g \lpar r\comma \; z\rpar \lpar \hat x + i\hat y\rpar \, \hbox{exp}\, [ i\lpar kz - \omega t\rpar ] \comma \; $$

where A _l(r, z) = (E _0l/f _l)(r/r _0lf _l)^lexp[−(r ²/2r _0l²f _l²)]L _p^l(r ²/r _0l²f _l²)exp(−iφ_l), A _g(r, z) = (E _0g/f _g)exp[−(r ²/2r _0g²f _g²)], E _0l and E _0g refers to the maximum amplitude of the LG and Gaussian beams of initial width r _0l & r _0g (in space), L _p^l is the associated Laguerre polynomial, l & p refer the binomial coefficients characterizing intensity modulation on the wavefront and φ_l refers the initial phase difference between the electric field vectors between Gaussian (E_g) and LG (E_l) beams in coaxial propagation. The dispersion relation characterize the em field in a plasma and can be expressed as ω² = (c ²k ² + ω_p²), where ω_p is the plasma frequency, k[=(ω/c)ε_0j^1/2] refers to the wave number associated with the em beam, ε_0j is the dielectric function corresponding to the axis of maximum electric field on the wavefront of the beam and c refers to the speed of light in vacuum; here j stands for LG (L ₀¹ mode) and Gaussian profiles of the beam.

The NLSE) describing the propagation of an em beam in a plasma (Sodha et al., Reference Sodha, Ghatak and Tripathi1976); following the Jeffreys–Wentzel–Kramers–Brillouin (JWKB) approximation (Ghatak & Loknathan, Reference Ghatak and Loknathan2004) for a slowly varying amplitude of the wave with propagation distance z (i.e., the term ∂ ²A _j/∂ z ² is neglected), the wave equation characterizing the electric field vector takes the form

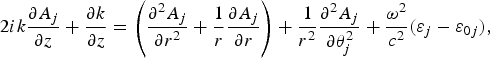(4)$$2ik\displaystyle{{\partial A_j } \over {\partial z}} + \displaystyle{{{\rm \partial} k} \over {\partial z}} = \left({\displaystyle{{\partial^2 A_j } \over {\partial r^2 }} + \displaystyle{1 \over r}\displaystyle{{\partial A_j } \over {\partial r}}} \right)+ \displaystyle{1 \over {r^2 }}\displaystyle{{\partial ^2 A_j } \over {\partial {\rm \theta} _j^2 }} + \displaystyle{{{\rm \omega} ^2 } \over {c^2 }}\lpar {\rm \varepsilon} _j - {\rm \varepsilon} _{0j} \rpar \comma \; $$

where ε_j refers the dielectric function of the plasma and j stands for Gaussian and LG beams.

The first two terms in the right-hand side of the above equation refer to the contribution in field evolution due to diffraction and phase variation; the manifestation of these terms with plasma non-linear effects (last term) causes the transverse focusing/defocusing of the em beam as it propagates in the plasma. The non-linearity in plasma primarily arises on account of electron density modification due to non-uniformity in the irradiance profile of the beam. The solution for Eq. (4) can be written as (Sodha et al., Reference Sodha, Ghatak and Tripathi1976)

(5)$$A_j \lpar r\comma \; z\comma \; {\rm \theta} _j \rpar = A_{0j} \lpar r\comma \; z\rpar \, \hbox{exp} [- i\lpar kS_j \lpar r\comma \; z\rpar + l{\rm \theta} _j \rpar ] \comma \; $$

where S _j(r, z) refers to the eikonal associated with the LG and Gaussian beam propagations.

Substituting for A _j(r, z, θ _j) in Eq. (4) and comparing the real and imaginary terms one gets (Thakur & Berakdar, Reference Thakur and Berakdar2010; Misra et al., Reference Misra, Mishra, Sodha and Tripathi2014)

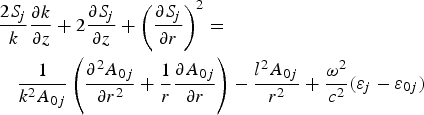(6a)$$\eqalign{&\displaystyle{{2S_j } \over k}\displaystyle{{\partial k} \over {\partial z}} + 2\displaystyle{{\partial S_j } \over {\partial z}} + \left({\displaystyle{{\partial S_j } \over {\partial r}}} \right)^2 = \cr & \quad \displaystyle{1 \over {k^2 A_{0j} }}\left({\displaystyle{{\partial^2 A_{0j} } \over {\partial r^2 }} + \displaystyle{1 \over r}\displaystyle{{\partial A_{0j} } \over {\partial r}}} \right)- \displaystyle{{l^2 A_{0j} } \over {r^2 }} + \displaystyle{{{\rm \omega} _{}^2 } \over {c^2 }}\lpar {\rm \varepsilon} _j - {\rm \varepsilon} _{0j} \rpar } $$

and

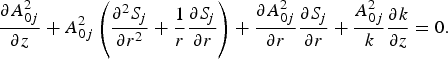(6b)$$\displaystyle{{\partial A_{0j}^2 } \over {\partial z}} + A_{0j}^2 \left({\displaystyle{{\partial^2 S_j } \over {\partial r^2 }} + \displaystyle{1 \over r}\displaystyle{{\partial S_j } \over {\partial r}}} \right)+ \displaystyle{{\partial A_{0j}^2 } \over {\partial r}}\displaystyle{{\partial S_j } \over {\partial r}} + \displaystyle{{A_{0j}^2 } \over k}\displaystyle{{\partial k} \over {\partial z}} = 0. $$

It is noticed that Gaussian and LG beams have different intensity profiles and characterized by on-axis and off-axis maxima, respectively; thus it is instructive that the dynamics of each beam should be analyzed in the vicinity of its intensity maxima.

2.1.1. LG Beam: L₀¹ Mode

As discussed earlier, LG beam displays an off-axis intensity profile and the above set of equations [Eq. (5)] should be transferred to the axis (r ≠ 0) in the vicinity of intensity maximum. For the sake of simplicity in the analysis, the propagation dynamics of a specific LG profile corresponding to L ₀¹ mode has been considered herein. For this particular case (i.e., L ₀¹ mode) the intensity maximum occurs at r = r _0lf _l. To proceed further a paraxial-like approach (Misra & Mishra, Reference Misra and Mishra2008; Sodha et al., Reference Sodha, Mishra and & Misra2009a) analogous to that of paraxial approximation is adopted where the coordinate system is transformed from (r, z) to (η _l, z) space such that

(7)$$\eta _l = [ \lpar r/r_{0l} f_l \rpar - 1] \comma \; $$

where r _0lf _l(z) is the width of the beam and r = r _0lf _l represents the position of the maximum irradiance on the wavefront as it advances in the plasma. In the vicinity of off-axis maxima, it is justified to expand other relevant parameters around intensity maximum, that is, η _l = 0. Using this relation the set of Eqs (6) thus transformed as (Misra & Mishra, Reference Misra and Mishra2008)

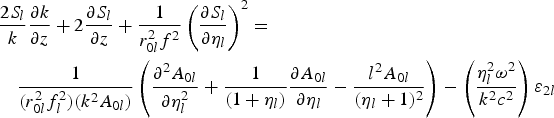(8a)$$\eqalign{& \displaystyle{{2S_l } \over k}\displaystyle{{\partial k} \over {\partial z}} + 2\displaystyle{{\partial S_l } \over {\partial z}} + \displaystyle{1 \over {r_{0l}^2 f^2 }}\left({\displaystyle{{\partial S_l } \over {\partial \eta_l }}} \right)^2 = \cr & \quad \displaystyle{1 \over {\lpar r_{0l}^2 f_l^2 \rpar \lpar k^2 A_{0l} \rpar }}\left({\displaystyle{{\partial^2 A_{0l} } \over {\partial \eta_l^2 }} + \displaystyle{1 \over {\lpar 1 + \eta_l \rpar }}\displaystyle{{\partial A_{0l} } \over {\partial \eta_l }} - \displaystyle{{l^2 A_{0l} } \over {\lpar \eta_l + 1\rpar^2 }}} \right)- \left({\displaystyle{{\eta_l^2 \omega^2 } \over {k^2 c^2 }}} \right)\varepsilon _{2l}} $$

and

(8b)$$\eqalign{\displaystyle{{\partial A_{0l}^2 } \over {\partial z}} + \displaystyle{{A_{0l}^2 } \over {\lpar r_{0l}^2 f_l^2 \rpar }}\left({\displaystyle{{\partial^2 S_l } \over {\partial \eta_l^2 }} + \displaystyle{1 \over {\lpar 1 + \eta_l \rpar }}\displaystyle{{\partial S_l } \over {\partial \eta_l }}} \right)+ \displaystyle{1 \over {r_0^2 f_l^2 }}\displaystyle{{\partial A_{0l}^2 } \over {\partial \eta _l }}\displaystyle{{\partial S_l } \over {\partial \eta _l }} + \displaystyle{{A_{0l}^2 } \over k}\displaystyle{{\partial k} \over {\partial z}} = 0\comma} \; $$

where ε_l (η _l, z) = ε_0l (z) − η _l² ε_2l (z) and like the paraxial theory the present analysis is strictly applicable when η _l≪1. In paraxial regime, the solution of Eqs. (8) can be written as

(9a)$$E_l E_l^\ast = A_{0l}^2 \lpar r\comma \; z\rpar = \lpar 2E_{0l}^2 /f_l^2 \rpar \lpar \eta _l + 1\rpar ^2 \, \hbox{exp}[ - \lpar \eta _l + 1\rpar ^2 ] $$

and

(9b)$$S_l = \lpar 1/2\rpar \lpar \eta _l + 1\rpar ^2 \beta _l \lpar z\rpar + \Theta _l \lpar z\rpar \comma \; $$

where β_l = r _0l²f _l (∂f _l /∂z), Θ_l(z) is the arbitrary phase function describing the departure of the curvature from spherical nature and f _l refers to the beam width parameter that characterizes the irradiance profile as it propagates in the plasma.

The solutions for A _0l² and S _l is consistent with Eq. (8b) and essentially characterize the maintenance of the shape of the beam as it advances through the plasma. Substituting for A _0l², S _l, and ε_l in Eq. (8a) and equating the coefficients of η _l⁰ and η _l² on both sides of the resulting equation one obtains

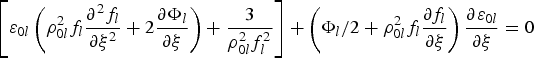(10a)$$\eqalign{\left[{\varepsilon_{0l} \left({\rho_{0l}^2 f_l \displaystyle{{\partial^2 f_l } \over {\partial \xi^2 }} + 2\displaystyle{{\partial \Phi_l } \over {\partial \xi }}} \right)+ \displaystyle{3 \over {\rho_{0l}^2 f_l^2 }}} \right]+ \left({\Phi_l /2 + \rho_{0l}^2 f_l \displaystyle{{\partial f_l } \over {\partial \xi }}} \right)\displaystyle{{\partial \varepsilon _{0l} } \over {\partial \xi }} = 0} $$

and

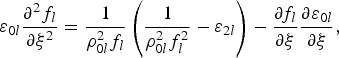(10b)$${\rm \varepsilon} _{0l} \displaystyle{{\partial ^2 f_l } \over {\partial \xi ^2 }} = \displaystyle{1 \over {\rho _{0l}^2 f_l }}\left({\displaystyle{1 \over {\rho_{0l}^2 f_l^2 }} - {\rm \varepsilon}_{2l} } \right)- \displaystyle{{\partial f_l } \over {\partial {\rm \xi} }}\displaystyle{{\partial {\rm \varepsilon} _{0l} } \over {\partial {\rm \xi} }}\comma \; $$

where ρ _0l = (r _0lω/c), Φ_l = (Θ_lω/c), and ξ = (zω/c).

The set of equations [Eq. (10)] characterizes the spatial evolution of the electric field envelop of LG (L ₀¹ mode) beam as it propagates in the plasma; in this course the transverse focusing/defocusing of the beam occurs. It should be noted here that the wave equations [Eqs. (7)–(10)] strictly correspond to the L ₀¹ mode of LG beam; however, the similar analysis based on the paraxial-like approach can be performed for various orders (i.e., arbitrary l & p) of off-axis LG intensity profiles.

2.1.2. Gaussian Beam

In case of Gaussian beam, the intensity maximum occurs at r = 0. Following earlier analyses (Sharma et al., Reference Sharma, Borhanian and Kourakis2009) based on paraxial approximation, the coupled differential equations describing the beam width parameter and phase in corresponding to Gaussian wavefront in the plasma can be written as

(11a)$${\rm \varepsilon} _{0g} \rho _g^2 \lpar d\Phi _g /d{\rm\xi} \rpar + \lpar 1/f_g^2 \rpar + \Phi _g \lpar d{\rm \varepsilon} _{0g} /d{\rm\xi} \rpar = 0 $$

and

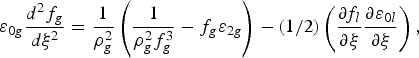(11b)$${\rm \varepsilon} _{0g} \displaystyle{{d^2 f_g } \over {d{\rm\xi} ^2 }} = \displaystyle{1 \over {\rho _g^2 }}\left({\displaystyle{1 \over {\rho_g^2 f_g^3 }} - f_g {\rm \varepsilon}_{2g} } \right)- \lpar 1/2\rpar \left({\displaystyle{{\partial f_l } \over {\partial {\rm\xi} }}\displaystyle{{\partial {\rm \varepsilon}_{0l} } \over {\partial {\rm\xi} }}} \right)\comma \; $$

where ρ _0g = (r _0gω/c) and Φ_g = (Θ_gω/c). The above equations [Eqs (11)] characterizing f _g and Φ_g are consistent with the irradiance profile

(12)$$A_{0g}^2 = \lpar E_{0g}^2 /f_g^2 \rpar \, \hbox{exp}\lpar - \eta _g^2 /f_g^2 \rpar $$

with ε_g (r,z) = ε_0g (z) − η _g² ε_2g (z), η _g = (r/r _0g).

It is interesting to note here that the algebraic form of the focusing equation [i.e., Eqs. (10b)–(11b)] is similar to that obtained in references (Nasalski, Reference Nasalski1995; Reference Nasalski1996; Thakur & Berkdar, Reference Thakur and Berakdar2010), where laser propagation dynamics in Kerr dielectric medium corresponding to Gaussian and LG profiles has been explored. In contrast to quadratic dependence of the dielectric function on the laser field in Kerr medium, the plasma exhibits saturating nature of non-linearity; this causes the oscillatory focusing/defocusing as the laser beam propagates in the plasma.

The set of Eqs. (10) and (11) is coupled through the dielectric function and characterize the coaxial propagation of the LG (L ₀¹ mode) and Gaussian beams. Using appropriate expressions for ε corresponding to plasma with dominant ponderomotive and relativistic non-linearities in addition to initial boundary conditions (corresponding to plane wavefront of the pulse at z = 0) viz.Φ_l(0) = Φ_g(0) = 0, f _l(0) = f _g(0) = 1 and f _l′ (0) = f _g′ (0) = 0, the equations can numerically be solved to evaluate the beam width parameter and phase dependence on the propagation distance ξ; for our computations Mathematica software is used. The knowledge of f _j and Φ_j leads to the information about spatial evolution of intensity profile as it propagates in the plasma.

2.2. Critical Condition for Focusing: Critical Curve

For an initial plane wavefront (i.e., df _j/dξ = 0) of the beam, (d ²f _j/dξ²)_ξ=0 = 0 refers to f _j(ξ) = 1 and the beam can propagate without convergence or divergence in plasma; this refers the critical condition for focusing of the em beam. Thus by substituting (d ²f _j/dξ²)_ξ=0 = 0 in Eqs. (10b) and (11b), one obtains a relation between dimensionless initial width of the beam ρ _j(=r _jω/c) and initial irradiance EE*, as the critical curve that ensures the propagation of the beam in the self-trapped mode. The critical condition is thus given by Sharma et al. (Reference Sharma, Prakash, Verma and Sodha2003)

(13)$$\rho _j^2 = \lpar 1/{\rm \varepsilon} _{2j} \rpar .$$

The beam displays self-focusing for the condition (d ²f _j/dξ²) < 0, whereas in case of (d ²f _j/dξ²)>0 the beam undergoes either oscillatory or steady divergence.

3. DIELECTRIC FUNCTION OF THE PLASMA

The non-linear propagation of any beam in the plasma is characterized by the non-linear dielectric function that is in the present analysis, modified by the coupling of fields (intensities) of both the beams. The spatial profile of the dielectric function as a consequence of the combined intensity profile (derived later) drives the non-linear effects in the plasma and hence propagation dynamics of the beams. Following Sodha et al. (Reference Sodha, Ghatak and Tripathi1974), the effective dielectric function of the plasma can be expressed as

(14)$$\rm \varepsilon \lpar r\comma \; z\rpar = 1 - \Omega ^2 \lpar n_{\rm e} /n_{{\rm e}0} \rpar \comma \; $$

where Ω = (ω_pe/ω), ω_pe = (4π n _e0e ²/m _e)^1/2 is the electron plasma frequency, n _e is the plasma density in the presence of em field, n _e0 refers the undisturbed plasma density, m _e is the mass of the electron, and e is the electronic charge.

As mentioned before, the plasma density redistribution and hence the effective dielectric function is determined by combined intensities of LG and Gaussian beams. The effective irradiance generated by LG (L ₀¹ mode) and Gaussian beams can be written as

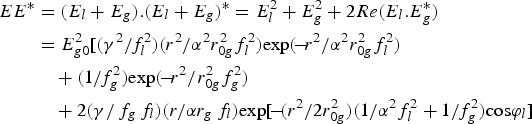(15)$$\eqalign{E{E^{\ast} } &= \lpar {E_l} + {E_g}\rpar .{\lpar {E_l} + {E_g}\rpar ^{\ast}} = E_l^2 + E_g^2 + 2Re\lpar {E_l}.E_g^{\ast} \rpar \cr & = E_{g0}^2 [ {\lpar {\gamma^2}/f_l^2 \rpar \lpar {r^2}/{\alpha^2}r_{0g}^2 f_l^2 \rpar \hbox{exp}\lpar \!-\! {r^2}/{\alpha^2}r_{0g}^2 f_l^2 \rpar } \cr & \quad + \lpar 1/f_g^2 \rpar \hbox{exp}\lpar \!-\! {r^2}/r_{0g}^2 f_g^2 \rpar \cr & \quad + { 2\lpar \gamma /{\,f_g}{\,f_l}\rpar \lpar r/\alpha {r_g}{\,f_l}\rpar \hbox{exp}[ \!-\! \lpar {r^2}/2r_{0g}^2 \rpar \lpar 1/{\alpha^2}f_l^2 + 1/f_g^2 \rpar \hbox{cos}{{\rm \varphi}_l}} ] } $$

with α(=r _0l/r _0g) and γ = E _l0/E _g0.

In the paraxial regime, the effective irradiance [EE*, Eq. (15)] can be expanded around its intensity maximum. Thus for Gaussian beam (r = 0), one gets

(16a)$$EE^{\ast} = a_g + b_g \eta _g + c_g \eta _g^2\comma \; $$

where a _g = (E _g0²/f _g²), b _g = E _g0²(2γ/αf _gf _l²) cosφ_l, and c _g = E _g0²[(γ²/α²f _l⁴) − (1/f _g⁴)].

Similarly in the case of L ₀¹ mode of the LG beam, EE* in the vicinity of irradiance maximum (i.e., η _l = 0) can be written as

(16b)$$EE^{\ast} = a_l + b_l \eta _l + c_l \eta _l^2\comma \; $$

where a _l = E _g0²[(γ ²/f _l²)exp(−1) + (1/f _g²)exp(−α ²f _lg²) + (2γ/f _gf _l) exp[−(1+α ²f _lg²)/2]cosφ_l], b _l = −E _g0²(α ²f _lg²)[(2/f _g²)exp(−α ²f _lg²) + (2γ/f _gf _l)exp[−(1+α ²f _lg²)/2]cosφ_l], c _l = −E _g0²[(2γ ²/f _l²) exp(−1) − (1/f _g²)(α ²f _lg²)exp(−α ²f _lg²) + (2γ/f _gf _l)exp[−(1+ α ²f _lg²)/2]cosφ_l], and f _lg = (f _l/f _g).

3.1. Evaluation of Dielectric Function

3.1.1. Ponderomotive Non-Linearity

In collisionless plasmas under influence of an em radiation, the redistribution of the electron density is determined by the balance of ponderomotive force with electron gas pressure gradient and the space charge electric field; the magnitude of the ponderomotive force is proportional to the gradient of beam irradiance. Such non-linearity sets in a period of the order of ω_pi⁻¹. For a collisionless plasma at moderate power of the beam (when the quiver speed of the electron is much smaller than the speed of light in vacuum), the modified electron density function n _e is given by (Akhmanov et al., Reference Akhmanov, Sukhorukov and Khokhlov1968; Sodha et al., Reference Sodha, Ghatak and Tripathi1976),

(17)$$n_{\rm e} = n_{{\rm e}0} \, \hbox{exp} \lpar \!-\! \beta EE^{\ast} \rpar \comma \; $$

where β = (e ²/8k _bT ₀m _eω²), k _b is the Boltzmann constant, and T ₀ is the temperature of the atoms/ions. By substituting Eq. (17) into (14), one obtains

(18)$$\eqalign{{\rm \varepsilon} _j \lpar r\comma \; z\rpar &= {\rm \varepsilon} _{0j} \lpar z\rpar - \eta _j^2 {\rm \varepsilon} _{2j} \lpar z\rpar = 1 - \Omega ^2 \, \hbox{exp}\lpar - {\rm \beta} EE^ {\ast} \rpar \cr & \approx [ 1 - \Omega ^2 \, \hbox{exp}\lpar - {\rm \beta} a_j \rpar ] - \eta _j^2 [ \Omega ^2 \lpar {\rm \beta} c_j - {\rm \beta} ^2 b_j^2 /2\rpar \, \hbox{exp}\lpar \!-\! {\rm \beta} a_j \rpar ] .} $$

Here ε_j(r, z) refer to the dielectric function for LG (L ₀¹ mode) and Gaussian beams in the vicinity of their intensity maximum.

3.1.2. Relativistic Non-Linearity

In the presence of high-intensity em radiation, the electrons may gain the quiver speed equivalent to the light speed in vacuum. This causes relativistic variation in the electron mass and consequent change in plasma frequency leads to the redistribution of electrons and triggers relativistic non-linearity (Hora, Reference Hora1975). This non-linearity sets in a period of the order of ω_pe⁻¹. The dielectric function in the case of circularly polarized beam can be expressed as (Hora, Reference Hora1991)

(19)$${\rm \varepsilon} _j \lpar r\comma \; z\rpar = 1 - \Omega ^2 \lpar 1 + \varsigma EE^{\ast} \rpar ^{ - 1/2}\comma \; $$

where ς = (e ²/m _e²ω²c ²). The dielectric function in the vicinity of intensity maxima can be written as

(20)$$\eqalign{{\rm \varepsilon} _j \lpar r\comma \; z\rpar &= {\rm \varepsilon} _{0j} \lpar z\rpar - \eta _j^2 {\rm \varepsilon} _{2j} \lpar z\rpar = 1 - \Omega ^2 \lpar 1 + \varsigma EE^{\ast} \rpar ^{ - 1/2} \cr & \approx [ 1 - \Omega ^2 \lpar 1 + \varsigma a_j \rpar ^{ - 1/2} ] - \eta _j^2 \lpar \Omega ^2 /2\rpar \lpar 1 + \varsigma a_j \rpar ^{ - 3/2} \cr & \quad [ \varsigma c_j - \lpar 3/4\rpar \varsigma ^2 b_j^2 \lpar 1 + \varsigma a_j \rpar ^{ - 1} ] .} $$

As can be seen from the final expressions for dielectric function, it is influenced by the irradiance profile of both the beams that are coupled through the phase difference between them; manifesting the appropriate expressions for the dielectric function [Eqs. (18) and (20)] with the beam dynamics equations [Eqs. (10) and (11)], coaxial (or coupled mode) propagation of LG (L ₀¹ mode) and Gaussian beams has been analyzed and discussed in the next section.

4. NUMERICAL RESULTS AND DISCUSSION

For a numerical appreciation of the analytical formulation and physics understanding of the coaxial propagation dynamics of central shadow LG (L ₀¹ mode in particular) and Gaussian em beams, critical curve, and non-linear space evolution are computed for an arbitrarily set of laser–plasma parameters and different kind of basic non-linearities; the estimates have been illustrated graphically. The propagation of the beams in the plasma is primarily characterized by dielectric function that becomes a complex function of electric fields of both the beams in coupled mode. The critical curves viz. the relation between initial irradiance g _j(=β E _0j²orς E _0j²) and initial beam width parameter (ρ _j) has been obtained using Eq. (13) and the appropriate expression for the transverse component of dielectric function and non-linearities. The non-linear space evolution of the beam width parameters (f _j) and eikonal phase (Θ_j) has been obtained by simultaneous numerical integration of Eqs. (10) and (11) along with the suitable laser plasma parameters and appropriate boundary conditions (as stated in the last paragraph of Section 2.1.2). The effect of varying initial laser parameters of both beams viz. initial width (ρ _j), initial irradiance (g _j), and initial phase difference (φ_l) on non-linear coaxial propagation dynamics and transverse compression have been evaluated and presented in the form of curves. The computations have been performed for the following standard set of parameters viz. β E _0g²(=ς E _0g²) = 5, β E _0l²(=ς E _0l²) = 2, γ ² = 2/5, α = 1, Ω² = 0.8, and φ_l = π/4; the choice of these normalized parameters refers to laser propagation in the weakly relativistic plasma regime having uniform background plasma density n _e0~10¹⁸ cm⁻³, laser wavelength λ~10 µm, and intensity I ₀ ≈ 10¹⁷ W/cm², respectively. The effect of various laser–plasma parameters on the critical curves and beam parameters has been studied by varying one and keeping others the same.

The critical curves (cc's) corresponding to dominant ponderomotive non-linearities have been illustrated in the set of Figure 1. The critical curves describe the self-trapping mode of the propagation of the em beams and characterize the em beam propagation in (ρ _j,g _j) space. It can readily be seen from Eq. (13) that the initial beam width (i.e., ρ _j⁻²) follows the behavior similar to that of transverse (azimuthal) component of the dielectric function ε_2j. The critical curve divides the (ρ _j,g _j) space primarily in two regions where the propagation of em beam is characterized by self (oscillatory)-focusing (region below cc) and oscillatory defocusing (region above in proximity of cc), or steady defocusing (region far away from cc). The figures also indicate the fact that the beam having large ρ _j and large irradiance in the self-focusing region, gives rise to larger non-linear effects. The parameters corresponding to an initial point (ρ _j,g _j) lying on critical curves correspond to (d ²f/d ξ ²)_ξ=0 = 0 and for an initially plane wavefront (df/d ξ) = 0, f remains constant and the beam propagates without convergence or divergence in plasma; such self-trapped motion of the beam is termed as stationary spatial soliton propagation. As stated before, the phase difference φ_l describes the coupling of electric field vectors associated with Gaussian and LG (L ₀¹ mode) beams and effectively characterize the intensity pattern and propagation of the coupled mode. The effect of varying φ_l on critical curves (ρ _g⁻² − g _g) corresponding to ponderomotive non-linearity for the given values of parameters α & g _l (=β E _0l²), has been illustrated in Figure 1a. The figure indicates that the self-focusing region decreases with increasing φ_l; this nature can be attributed to large coupling between the coaxial beams with decreasing φ_l which enhances the effective irradiance of the beam and therefore more pronounced non-linear effects. It is seen that the self-focusing region decreases with the increasing value of the parameter α; this behavior has been displayed in Figure 1b and can be understood in terms of decrease in effective intensity [via Eqs. (16) and (18)] with increasing α. The self-focusing region is seen to increase with increasing parameter g _l; this nature has been displayed in Figure 1c. Similarly the critical curves can also be obtained in terms of parameters associated with LG beams, that is (ρ _l⁻² − g _l) for given values of α and g _g; however, these curves are another way of presentation of cc's and carry the same information as described in the case of Figure 1. The dependence of critical curves corresponding to dominant relativistic non-linearity has been displayed in the set of Figure 2; the nature of the curves is similar to that obtained in the case of ponderomotive non-linearity (Fig. 1) and physically interpretable in the similar fashion. These critical curves characterize the regions for self-focusing and oscillatory/steady divergence and valid throughout the propagation dynamics.

Fig. 1. Dependence of cc's (ρ _g⁻² − g _g) on (a) phase φ_l associated with LG beams, (b) parameter α and (c) irradiance of LG beams g _l corresponding to ponderomotive non-linearity for the standard set of parameters stated in the text.

Fig. 2. Dependence of cc's (ρ _g⁻² − g _g) on (a) phase φ_l associated with LG beams, (b) parameter α and (c) irradiance of LG beams g _l corresponding to relativistic non-linearity for the standard set of parameters stated in the text.

The dependence of beam width parameters associated with coaxial propagation of LG (f _l) and Gaussian (f _g) beams in the plasma having dominant ponderomotive non-linearity has been illustrated in Figure 3a (solid lines). It is seen that during coaxial propagation each beam mutually influence the dynamics of the other beam; in order to illustrate this fact the independent propagation of individual beams has been shown by broken lines in the figure. It can be seen from the figure that the additional influence of LG beam in coupled mode propagation causes the focusing of the Gaussian beam as it advances through the plasma. The effect of coaxial propagation on space evolution of the eikonal phase associated with LG (Φ_l) and Gaussian (Φ_g) beams (as in Fig. 3a) has been displayed in Figure 3b. The behavior of the curves corresponding to coaxial propagation is in well conformance with the critical curves as shown in Figure 1. The effect of phase difference (i.e., φ_l) on coaxial propagation of the beams corresponding to φ_l = π/4 & π/8 has been displayed in Figure 4a. The figure reflects the strong coupling in case of φ_l = π/4 as the focusing curves approach each other; this nature is well appreciated with critical curves in Figure 1a. The co-axial beam is seen to exhibit larger focusing with increasing parameter α and is shown in Figure 4b. The increase in α effectively refers to large initial beam width (ρ _l) associated with the LG mode (for constant ρ _g) that enhances non-linearity and hence the focusing. Further the influence of the initial irradiance of the LG mode on the non-linear propagation dynamics of Gaussian beam coaxially has been displayed in Figure 4c; the strong non-linear effects in the case of g _l = 5 can be understood as a consequence of critical curves displayed in Figure 1c. The figures corresponding to the coupled mode propagation suggest that the dynamics of one beam can be controlled up to significant extent by varying the parameters of the other beam.

Fig. 3. Space evolution of the (a) beam width parameters (f _j) and (b) phase (Φ_j) corresponding to ponderomotive non-linearity for the standard set of parameters stated in the text with φ_l = π/6; solid lines refer to coaxial propagation, while the broken curves correspond to separate propagation of Gaussian (f _g, black lines) and LG (f _l, red lines) beams.

Fig. 4. Space evolution of the beam width parameters (f _j) corresponding to ponderomotive non-linearity for the standard set of parameters stated in the text. Panel (a) corresponds to varying phase φ_l(=π/4, solid) and φ_l(=π/8, broken), panel (b) refers to varying parameter α(=1, solid) and α(=1.5, broken), panel (c) refers to varying irradiance of LG beam g _l(=2, solid) and g _l(=5, broken); red and black color lines refer to f _l and f _g, respectively.

The beam width parameters f's certainly describe the evolution of electric field or intensity envelop (i.e., EE*) of the em beam and hence the power (energy) transfer as it advances through the plasma. In order to have an idea of the radial distribution of the intensity during coaxial propagation of LG–Gaussian modes, the space evolution of the effective irradiance profile in non-linear regime of the plasma characterized by dominant ponderomotive non-linearity has been displayed in Figure 5; the distribution is shown at different ξ values for the parameters g _g = 10, g _l = 5, α = 3, and φ_l = π/6. It is shown that during coaxial propagation, the beam dynamics mutually influence each other and gives rise to large non-linear effects; as a consequence the transverse laser field itself gets modified as it advances through the plasma. It can be seen from the figure that on account of mutual focusing/defocusing the radial distribution of intensity displays compression/rarefaction of the beams; the intensity distribution of LG and Gaussian modes independently has been shown by the broken curves. It is also noticed that the coupling of Gaussian mode with annular (L ₀¹ mode) field profile leads to significant enhancement (e.g., ξ = 36) in its irradiance as beam propagates through the plasma; this nature is qualitatively similar as obtained in one of the recent experimental investigation (Scheller et al., Reference Scheller, Mills, Miri, Cheng, Moloney, Kolesik, Polynkin and Christodoulides2014). The spatial evolution of such profiles (characterized by potential dipoles) can also efficiently be utilized for trapping of plasma particles. Furthermore, it is also necessary to point out that a specific case, that is, propagation dynamics of LG em beam corresponding to L ₀¹ mode has been considered herein the analysis however the methodology of paraxial-like approach can be extended to investigate the propagation dynamics of higher order LG modes.

Fig. 5. Space evolution of the radial distribution of the beam irradiance (EE*/E _0g²) as a function of parameters (ξ); the figure corresponds to dominant ponderomotive non-linearity for the parameters g _g = 10, g _l = 5, α = 3, and& ϕ_l = π/6.

5. SUMMARY

A formalism describing the non-linear coaxial propagation dynamics of finite size intense LG (L ₀¹ mode) and Gaussian beams in a plasma characterized by ponderomotive and relativistic non-linearities has been established. In order to analyze the off-axis contribution of annular LG beams the formulation takes account of paraxial-like approach, while usual paraxial approximation is utilized to analyze the dynamics of Gaussian mode propagation. The dynamics of coaxial propagation is coupled through the dielectric function which is considered to be a function of combined electric field of both the propagating modes. The coaxial propagation dynamics is described by NLSE which governs the spatial evolution of the coupled mode as it advances through the plasma. Based on this analysis, the critical curves and space evolution of beam width parameter (f _j) and phase (Φ_j) of coupled mode have been computed and presented graphically. The critical curves predict the regimes of oscillatory (self) focusing/defocusing and steady divergence of the coupled mode propagation; this characteristic feature is a consequence of competing phenomenon of diffraction with non-linear effects. It is shown that the coupling of Gaussian profile with L ₀¹ mode significantly enhances its intensity as the coupled mode advances through the plasma. The focusing dynamics of such profiles (i.e., existence of sharp potential dipoles) is of relevance to the particle/atomic trapping and efficient energy transport which has significantly enhanced due to self-focusing of the coaxial beams.