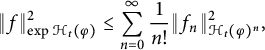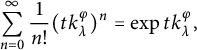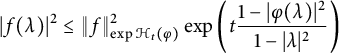1 Introduction
Let
${\mathbb D}$
be the open unit disk in the complex plane
${\mathbb C}$
, and let
$H^{\infty }$
be the Banach algebra consisting of all bounded analytic functions on
${\mathbb D}$
. Then, we set
$$ \begin{align*} {\mathcal S}=\{\varphi\in H^{\infty}:|\varphi(\lambda)|\leq 1\ (\lambda \in {\mathbb D})\}, \end{align*} $$
which is called the Schur class. For any function
$\varphi $
in
$H^{\infty }$
, it is well known that
$\varphi $
belongs to
${\mathcal S}$
if and only if
$$ \begin{align} \dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z} \end{align} $$
is positive semi-definite. This equivalence relation based on the properties of the Szegö kernel is crucial in the operator theory on the Hardy space over
${\mathbb D}$
, in particular, theories of Pick interpolation, de Branges–Rovnyak spaces and sub-Hardy Hilbert spaces (see Agler–McCarthy [Reference Agler and McCarthy2], Ball–Bolotnikov [Reference Ball and Bolotnikov4], Fricain–Mashreghi [Reference Fricain and Mashreghi6], and Sarason [Reference Sarason14]). The kernel (1.1) is called the de Branges–Rovnyak kernel.
Before introducing our study, we should mention that not only the original de Branges–Rovnyak kernel but also its variants have been studied by a number of authors. For example, Zhu [Reference Zhu16, Reference Zhu17] initiated the study on the kernel
$$ \begin{align} \dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{(1-\overline{\lambda}z)^{2}} \end{align} $$
in the Bergman space over
${\mathbb D}$
. The reproducing kernel Hilbert space induced by the kernel (1.2) is called a sub-Bergman Hilbert space (see also Abkar–Jafarzadeh [Reference Abkar and Jafarzadeh1], Ball–Bolotnikov [Reference Ball and Bolotnikov3], Chu [Reference Chu5], Nowak–Rososzczuk [Reference Nowak and Rososzczuk11], and Sultanic [Reference Sultanic15]). Further, powers of the de Branges–Rovnyak kernel
$$ \begin{align} \left(\dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z}\right)^{n}\quad (n\in {\mathbb N}) \end{align} $$
are naturally obtained from the theory of hereditary functional calculus for weighted Bergman spaces on
${\mathbb D}$
(see Example 14.48 in [Reference Agler and McCarthy2] for the case where
$n=2$
) and have appeared also in p. 3672 of Jury [Reference Jury8].
Now, the purpose of this paper is to study the structure of the kernel
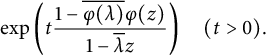$$ \begin{align} \exp\left(t\dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z}\right)\quad (t>0). \end{align} $$
Note that our kernel (1.4) is obtained by binding all kernels in (1.3) together. Thus, we expect that new properties of the de Branges–Rovnyak kernel (1.1) are drawn out from our kernel (1.4). In fact, as the main theorem, we will show that the exponential of the de Branges–Rovnyak kernel is strictly positive definite if
$\varphi $
is nontrivial.
Here, we shall give some remarks on strictly positive definite kernels. In general, it is not difficult to construct positive semi-definite kernels. On the other hand, for strictly positive definite kernels, nontrivial methods depending on each case are often needed (for example, see Micchelli [Reference Micchelli9]). Moreover, it might be worth while mentioning that strictly positive definite kernels have received attention in machine learning (see Rasmussen–Williams [Reference Rasmussen and Williams13]).
This paper is organized as follows. In Section 2, basic properties of the reproducing kernel Hilbert space
$\exp {\mathcal H}_{t}(\varphi )$
constructed from our kernel (1.4) are given. In Section 3, unbounded multipliers on
$\exp {\mathcal H}_{t}(\varphi )$
are introduced and studied. In Section 4, we prove the main theorem.
2 Preliminaries
For
$t>0$
, let
${\mathcal H}_{t}(\varphi )$
denote the reproducing kernel Hilbert space with kernel
$$ \begin{align*} tk^{\varphi}(z,\lambda) =t\dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z}\quad (\varphi \in {\mathcal S}), \end{align*} $$
and we will use notations
$tk_{\lambda }^{\varphi }(z)=tk^{\varphi }(z,\lambda )$
and
${\mathcal H}(\varphi )={\mathcal H}_{1}(\varphi )$
. Then, since
$$ \begin{align*} \langle tk_{\lambda}^{\varphi},tk_{z}^{\varphi} \rangle_{{\mathcal H}_{t}(\varphi)} =tk^{\varphi}(z,\lambda)=t^{-1}\langle tk_{\lambda}^{\varphi},tk_{z}^{\varphi} \rangle_{{\mathcal H}(\varphi)}, \end{align*} $$
the trivial linear mapping
$f\mapsto f$
from
${\mathcal H}(\varphi )$
onto
${\mathcal H}_{t}(\varphi )$
is bounded and invertible. Particularly,
${\mathcal H}_{t}(\varphi )={\mathcal H}(\varphi )$
as vector spaces. In this section, we construct the exponential of
${\mathcal H}_{t}(\varphi )$
and give its basic properties. The contents of this section are well known to specialists. For example, see Exercise (k) in p. 320 of Nikolski [Reference Nikolski10] and Chapter 7 in Paulsen–Raghupathi [Reference Paulsen and Raghupathi12]. However, we give the details for the sake of readers.
2.1 Construction of
$\exp {\mathcal H}_{t}(\varphi )$
Let
${\mathcal H}_{t}(\varphi )^{n}$
be the reproducing kernel Hilbert space obtained by the pull-back construction with the n-fold tensor product space
$$ \begin{align*} {\mathcal H}_{t}(\varphi)^{\otimes n}={\mathcal H}_{t}(\varphi)\otimes \cdots \otimes {\mathcal H}_{t}(\varphi) \end{align*} $$
and the n-dimensional diagonal map
$$ \begin{align*} \Delta_{n}:{\mathbb D}\to {\mathbb D}^{n},\ \lambda\to (\lambda,\ldots,\lambda) \end{align*} $$
(for the pull-back construction, see Theorem 5.7 in [Reference Paulsen and Raghupathi12]). We note that
$ (tk_{\lambda }^{\varphi })^{\otimes n}\circ \Delta _{n}=(tk_{\lambda }^{\varphi })^{n}$
is the reproducing kernel of
${\mathcal H}_{t}(\varphi )^{n}$
. Let
$\oplus _{n=0}^{\infty }{\mathcal H}_{t}(\varphi )^{n}$
denote the Hilbert space with the inner product
$$ \begin{align*} \langle (f_{0},f_{1},\ldots)^{\top},(g_{0},g_{1},\ldots)^{\top} \rangle_{\oplus_{n=0}^{\infty}{\mathcal H}_{t}(\varphi)^{n}} =\sum_{n=0}^{\infty}\dfrac{1}{n!}\langle f_{n},g_{n} \rangle_{{\mathcal H}_{t}(\varphi)^{n}}, \end{align*} $$
where we set
${\mathcal H}_{t}(\varphi )^{0}={\mathbb C}$
. Moreover, we define the linear map
$\Gamma $
as follows:
$$ \begin{align*} \Gamma: \begin{pmatrix} f_0\\ f_1\\ \vdots \end{pmatrix} \mapsto \sum_{n=0}^{\infty}\dfrac{1}{n!}f_n\quad \left( \begin{pmatrix} f_0\\ f_1\\ \vdots \end{pmatrix} \in\oplus_{n=0}^{\infty}{{\mathcal H}}_t(\varphi)^n \right). \end{align*} $$
Proposition 2.1 The following statements hold:
-
(1)
$\Gamma $
is a map from
$\oplus _{n=0}^{\infty }{\mathcal H}_{t}(\varphi )^{n}$
to
$\mathrm {Hol}({\mathbb D})$
. -
(2)
$\ker \Gamma $
is closed.
Proof. For any
$F=(f_{0},f_{1},\ldots )^{\top }$
in
$\oplus _{n=0}^{\infty }{\mathcal H}_{t}(\varphi )^{n}$
, we have
$$ \begin{align} \left| \sum_{\ell=n+1}^{m}\dfrac{1}{\ell !}f_{\ell}(\lambda) \right| &\leq \sum_{\ell=n+1}^{m}\left|\dfrac{1}{\ell !}f_{\ell}(\lambda)\right|\notag \\ &\leq \sum_{\ell=n+1}^{m}\dfrac{1}{\ell !}\|f_{\ell}\|_{{\mathcal H}_{t}(\varphi)^{\ell}}\|(tk_{\lambda}^{\varphi})^{\ell}\|_{{\mathcal H}_{t}(\varphi)^{\ell}}\notag \\ &\leq \left(\sum_{\ell =n+1}^{m}\dfrac{1}{\ell !}\|f_{\ell}\|_{{\mathcal H}_{t}(\varphi)^{\ell}}^{2}\right)^{1/2} \left(\sum_{\ell=n+1}^{m}\dfrac{1}{\ell !}\|(tk_{\lambda}^{\varphi})^{\ell}\|_{{\mathcal H}_{t}(\varphi)^{\ell}}^{2}\right)^{1/2}\notag \\ &= \left(\sum_{\ell=n+1}^{m}\dfrac{1}{\ell !}\|f_{\ell}\|_{{\mathcal H}_{t}(\varphi)^{\ell}}^{2}\right)^{1/2} \left(\sum_{\ell=n+1}^{m}\dfrac{1}{\ell !}\|tk_{\lambda}^{\varphi}\|_{{\mathcal H}_{t}(\varphi)}^{2\ell}\right)^{1/2}. \end{align} $$
Hence,
$\sum _{n=0}^{\infty }\dfrac {1}{n!}f_{n}(\lambda )$
converges uniformly on any compact subset of
${\mathbb D}$
. This concludes (1). Next, let K be a compact set in
${\mathbb D}$
. Then, since
$$ \begin{align*} \|tk_{\lambda}^{\varphi}\|_{{\mathcal H}_{t}(\varphi)}^{2} =t\frac{1-|\varphi(\lambda)|^{2}}{1-|\lambda|^{2}}, \end{align*} $$
there exists a constant
$C_{K}>0$
such that
$$ \begin{align*} \sup_{\lambda \in K}\|tk_{\lambda}^{\varphi}\|_{{\mathcal H}_{t}(\varphi)}^{2n}\leq C_{K}^{n}. \end{align*} $$
Moreover, in (2.1), we essentially showed that
$$ \begin{align} |(\Gamma F)(\lambda)|\leq \|F\|_{\oplus_{n=0}^{\infty}{\mathcal H}_{t}(\varphi)^{n}}\exp \frac{\|tk_{\lambda}^{\varphi}\|_{{\mathcal H}_{t}(\varphi)}^{2}}{2}. \end{align} $$
Hence, we have
$$ \begin{align*} \sup_{\lambda \in K}|(\Gamma F)(\lambda)|\leq \|F\|_{\oplus_{n=0}^{\infty}{\mathcal H}_{t}(\varphi)^{n}}\exp\frac{C_{K}}{2}. \end{align*} $$
Therefore,
$\Gamma $
is continuous. This concludes (2).
By Proposition 2.1, the pull-back construction can be applied to
$\Gamma $
.
Definition 2.1 We define
$\exp {{\mathcal H}_{t}(\varphi )}$
as the reproducing kernel Hilbert space obtained by the pull-back construction with the linear map
$$ \begin{align*} \Gamma: \oplus_{n=0}^{\infty}{\mathcal H}_{t}(\varphi)^{n}\to \text{Hol}({\mathbb D}). \end{align*} $$
2.2 Basic properties of
$\exp {\mathcal H}_{t}(\varphi )$
We summarize basic properties of
$\exp {{\mathcal H}_{t}(\varphi )}$
.
Proposition 2.2
$\exp {{\mathcal H}_{t}(\varphi )}$
is a reproducing kernel Hilbert space consisting of holomorphic functions on
${\mathbb D}$
. More precisely, for any f in
$\exp {\mathcal H}_{t}(\varphi )$
, there exists a vector
$(f_{0},f_{1},\ldots ,)^{\top }$
in
$ \oplus _{n=0}^{\infty }{\mathcal H}_{t}(\varphi )^{n}$
such that
$$ \begin{align*} f=\sum_{n=0}^{\infty}\dfrac{1}{n!}f_{n} \end{align*} $$
converges uniformly on any compact subset of
${\mathbb D}$
. Moreover,
-
(1) the following norm estimate holds:
$$ \begin{align*} \|f\|_{\exp{\mathcal H}_{t}(\varphi)}^{2}\leq \sum_{n=0}^{\infty}\dfrac{1}{n!}\|f_{n}\|_{{\mathcal H}_{t}(\varphi)^{n}}^{2}, \end{align*} $$
-
(2) the reproducing kernel of
$\exp {\mathcal H}_{t}(\varphi )$
is that is,
$$ \begin{align*} \sum_{n=0}^{\infty}\dfrac{1}{n!}(tk_{\lambda}^{\varphi})^{n}= \exp tk_{\lambda}^{\varphi}, \end{align*} $$
for any
$$ \begin{align*} f(\lambda)=\langle f, \exp tk_{\lambda}^{\varphi}\rangle_{\exp{\mathcal H}_{t}(\varphi)} \end{align*} $$
$\lambda $
in
${\mathbb D}$
,
-
(3) the following growth condition holds:
for any
$$ \begin{align*} |f(\lambda)|^{2} \leq \|f\|_{\exp{\mathcal H}_{t}(\varphi)}^{2}\exp\left(t \dfrac{1-|\varphi(\lambda)|^{2}}{1-|\lambda|^{2}}\right) \end{align*} $$
$\lambda $
in
${\mathbb D}$
.
Proof. By the definition of the norm and the inner product of
$\exp {\mathcal H}_{t}(\varphi )$
, we have conclusions.
3 Unbounded multipliers
We shall investigate into unbounded multipliers of
$\exp {\mathcal H}_{t}(\varphi )$
.
Lemma 3.1 Let
$\psi $
be a function in
${\mathcal H}_{t}(\varphi )$
. Then, for any function f in
${\mathcal H}_{t}(\varphi )^{n}$
,
$\psi f$
belongs to
${\mathcal H}_{t}(\varphi )^{n+1}$
.
Proof. We define the bounded linear operator
$\tau _{\psi }$
as follows:
$$ \begin{align*} \tau_{\psi}: {\mathcal H}_{t}(\varphi)^{\otimes n} \to{\mathcal H}_{t}(\varphi)^{\otimes n+1}, \quad F\mapsto \psi \otimes F. \end{align*} $$
Then, the following diagram commutes:
where
$M_{\psi }$
denotes the multiplication operator with symbol
$\psi $
. This concludes the proof.
Theorem 3.2 Let
$\psi $
be a function in
${\mathcal H}_{t}(\varphi )$
. Then, the multiplication operator
$M_{\psi }$
is a densely defined closable linear operator in
$\exp {\mathcal H}_{t}(\varphi )$
.
Proof. Let
$F=(f_{0},f_{1},\ldots ,f_{N},0\ldots )^{\top }$
be a vector having finite support in
$\oplus _{n=0}^{\infty }{\mathcal H}_{t}(\varphi )^{n}$
. We set
$\Gamma F=f$
. Then,
$$ \begin{align*}\psi f=\psi \sum_{n=0}^{N}\dfrac{1}{n!}f_{n}=\sum_{n=0}^{N}\dfrac{1}{n!}\psi f_{n}= \sum_{n=0}^{N}\dfrac{1}{(n+1)!}(n+1)\psi f_{n}=\sum_{n=1}^{N+1}\dfrac{1}{n!}n\psi f_{n-1}, \end{align*} $$
where we note that
$n\psi f_{n-1}$
belongs to
${\mathcal H}_{t}(\varphi )^{n}$
by Lemma 3.1. Hence, setting
$$ \begin{align*} G=(0, \psi f_{0},2\psi f_{1},\ldots, (N+1)\psi f_{N},0,\ldots)^{\top}, \end{align*} $$
G belongs to
$\oplus _{n=0}^{\infty }{\mathcal H}_{t}(\varphi )^{n}$
and
$\Gamma G=\psi f$
, that is,
$\psi f$
belongs to
$\exp {\mathcal H}_{t}(\varphi )$
. Therefore,
$M_{\psi }$
is a densely defined linear operator in
$\exp {\mathcal H}_{t}(\varphi )$
. Moreover, it is easy to see that
$M_{\psi }$
is closable.
Corollary 3.3 Let
$\psi $
be a function in
${\mathcal H}_{t}(\varphi )$
. Then the adjoint operator
$M_{\psi }^{\ast }$
of
$M_{\psi }$
is a densely defined closed linear operator in
$\exp {\mathcal H}_{t}(\varphi )$
, and every
$\exp tk_{\lambda }^{\varphi }$
is an eigenfunction of
$M_{\psi }^{\ast }$
. More precisely,
$$ \begin{align*} M_{\psi}^{\ast}\exp tk_{\lambda}^{\varphi}=\overline{\psi(\lambda)} \exp tk_{\lambda}^{\varphi}. \end{align*} $$
4 Main results
Let X be a set. A function k on
$X\times X$
is called a strictly positive definite kernel on X if
$k(x,y)=\overline {k(y,x)}$
for any x and y in X and
$$ \begin{align*} \sum_{i,j=1}^{n}c_{i}\overline{c_{j}}k(x_{j},x_{i})>0 \end{align*} $$
for any n in
$\mathbb N$
, any
$(c_{1},\ldots , c_{n})^{\top }$
in
${\mathbb C}^{n}\setminus \{ \mathbf {0} \}$
and any n distinct points
$x_{1},\ldots ,x_{n}$
in X. For example, it is well known that
$$ \begin{align*} k(z,\lambda)=\exp(\overline{\lambda}z) \end{align*} $$
is a strictly positive definite kernel on
${\mathbb C}$
. In fact, this is the reproducing kernel of the Segal–Bargmann space. Now, we note that if
$\varphi =z^{2}$
then
$$ \begin{align*} e^{-1}\exp\left(\dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z}\right) =\exp(\overline{\lambda}z). \end{align*} $$
Motivated by this observation, we shall give new examples of strictly positive definite kernels. We consider the following three conditions: (C1)
$\varphi (0)=\varphi ^{\prime }(0)=0$
, (C2)
$\varphi (\mu )=0$
for some
$\mu $
in
${\mathbb D}\setminus \{0\}$
, (C3) the dimension of
${\mathcal H}(\varphi )$
is infinite.
We need the following lemma.
Lemma 4.1 Let
$\lambda _{1},\ldots ,\lambda _{n}$
be n distinct points in
${\mathbb D}$
. Suppose one of (C1), (C2) and (C3). Then there exists a function
$\psi $
in
${\mathcal H}_{t}(\varphi )$
such that
$\psi (\lambda _{i})\neq \psi (\lambda _{j}) (i\neq j)$
.
Proof. Since
${\mathcal H}_{t}(\varphi )={\mathcal H}(\varphi )$
as vector spaces, it suffices to show the statement for
${\mathcal H}(\varphi )$
. First, we assume (C1). Then, since
$\varphi /z$
is in
${\mathcal S}$
by the Schwarz lemma and
$(\varphi /z)(0)=0$
, we have
$$ \begin{align*} (I-T_{\varphi}T_{\varphi}^{\ast})z=z-T_{\varphi}T_{\varphi/z}^{\ast}T_{z}^{\ast}z =z-T_{\varphi}T_{\varphi/z}^{\ast}1=z, \end{align*} $$
where
$T_{\varphi }$
denotes the Toeplitz operator with symbol
$\varphi $
on the Hardy space
$H^{2}$
over
${\mathbb D}$
. Hence z belongs to
${\mathcal H}(\varphi )$
, and we may take
$\psi =z$
.
Secondly, we assume (C2). Let
$\mu $
be a nonzero zero point of
$\varphi $
. Then, we have
$$ \begin{align*} k_{\mu}^{\varphi}=(I-T_{\varphi}T_{\varphi}^{\ast})k_{\mu}=k_{\mu}. \end{align*} $$
Hence,
$(1-\overline {\mu }z)^{-1}$
belongs to
${\mathcal H}(\varphi )$
, and we may take
$\psi =(1-\overline {\mu }z)^{-1}$
.
Thirdly, we assume (C3). Then, by Lemma 31.2 in [Reference Fricain and Mashreghi6], the family
$\{k_{\lambda _{j}}^{\varphi }:1\leq j\leq n\}$
is minimal. Hence, we have that
$\dim \operatorname {span}\{k_{\lambda _{j}}^{\varphi }:1\leq j\leq n\}=n$
. Let T be the linear map defined as follows:
$$ \begin{align*} T: \operatorname{span}\{k_{\lambda_{j}}^{\varphi}:1\leq j\leq n\}\to {\mathbb C}^{n},\quad f\mapsto (f(\lambda_{1}),\ldots,f(\lambda_{n})). \end{align*} $$
Then, it is easy to see that
$\ker T=\{0\}$
. Hence, there exists a function
$\psi $
in
${\mathcal H}(\varphi )$
such that
$\psi (\lambda _{i})\neq \psi (\lambda _{j}) (i\neq j)$
.
Theorem 4.2 Let
$\varphi $
be a function in
${\mathcal S}$
. If
$\varphi $
satisfies one of (C1), (C2) and (C3), then the kernel
$$ \begin{align*} k_{t}(z,\lambda)=\exp\left(t\dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z}\right) \quad (t>0) \end{align*} $$
is strictly positive definite.
Proof. It suffices to show that
$\{\exp tk_{\lambda _{j}}^{\varphi }\}_{j=1}^{n}$
is linearly independent for any n in
$\mathbb N$
and any n distinct points
$\lambda _{1},\ldots , \lambda _{n}$
in
${\mathbb D}$
. Suppose that
$$ \begin{align*} \sum_{j=1}^{n}c_{j}\exp tk_{\lambda_{j}}^{\varphi}=0 \end{align*} $$
for some n in
$\mathbb N$
, some n distinct points
$\lambda _{1},\ldots , \lambda _{n}$
in
${\mathbb D}$
, and some
$c_{1},\ldots ,c_{n}$
in
${\mathbb C}$
. Then, for any function
$\psi $
in
${\mathcal H}_{t}(\varphi )$
, by Corollary 3.3 and the assumption, we have
$$ \begin{align*} \begin{pmatrix} 1 & \cdots & 1\\ \overline{\psi(\lambda_{1})} & \cdots & \overline{\psi(\lambda_{n})}\\ \vdots & \vdots & \vdots \\ \overline{\psi(\lambda_{1})}^{\,{}n-1} & \cdots & \overline{\psi(\lambda_{n})}^{\,{}n-1} \end{pmatrix} \begin{pmatrix} c_{1}\exp tk_{\lambda_{1}}^{\varphi}\\ c_{2}\exp tk_{\lambda_{2}}^{\varphi}\\ \vdots\\ c_{n}\exp tk_{\lambda_{n}}^{\varphi} \end{pmatrix} &= \begin{pmatrix} \sum_{j=1}^{n}c_{j}\exp tk_{\lambda_{j}}^{\varphi}\\ \sum_{j=1}^{n}\overline{\psi(\lambda_{j})}c_{j}\exp tk_{\lambda_{j}}^{\varphi}\\ \vdots\\ \sum_{j=1}^{n}c_{j}\overline{\psi(\lambda_{j})}^{\,{}n-1}\exp tk_{\lambda_{j}}^{\varphi} \end{pmatrix}\\ &= \begin{pmatrix} \sum_{j=1}^{n}c_{j}\exp tk_{\lambda_{j}}^{\varphi}\\ M_{\psi}^{\ast}\sum_{j=1}^{n}c_{j}\exp tk_{\lambda_{j}}^{\varphi}\\ \vdots\\ (M_{\psi}^{\ast})^{n-1}\sum_{j=1}^{n}c_{j}\exp tk_{\lambda_{j}}^{\varphi} \end{pmatrix}\\ &=\mathbf{0}. \end{align*} $$
Further, by Lemma 4.1, there exists a function
$\psi $
in
${\mathcal H}_{t}(\varphi )$
such that
$$ \begin{align*} \prod_{1\leq i<j\leq n}(\psi(\lambda_{i})-\psi(\lambda_{j}))\neq 0. \end{align*} $$
Then, the Vandermonde matrix
$$ \begin{align*} \begin{pmatrix} 1 & \cdots & 1\\ \overline{\psi(\lambda_{1})} & \cdots & \overline{\psi(\lambda_{n})}\\ \vdots & \vdots & \vdots \\ \overline{\psi(\lambda_{1})}^{n-1} & \cdots & \overline{\psi(\lambda_{n})}^{\,{}n-1} \end{pmatrix} \end{align*} $$
is nonsingular. Therefore, we have that
$$ \begin{align*} \begin{pmatrix} c_{1}\exp tk_{\lambda_{1}}^{\varphi}\\ c_{2}\exp tk_{\lambda_{2}}^{\varphi}\\ \vdots\\ c_{n}\exp tk_{\lambda_{n}}^{\varphi} \end{pmatrix}=\mathbf{0}. \end{align*} $$
This concludes that
$c_{1}=\cdots =c_{n}=0$
.
The well-known fact mentioned at the beginning of this section is included in Theorem 4.2.
Corollary 4.3 The kernel function
$$ \begin{align*} k(z,\lambda)=\exp(\overline{\lambda}z) \end{align*} $$
is strictly positive definite on
${\mathbb C}$
.
Proof. For any n distinct points
$\lambda _{1},\ldots \lambda _{n}$
in
${\mathbb C}$
, we set
$R=\max _{1\leq j \leq n} |\lambda _{j}|+1$
. Then
$\lambda _{1}/R,\ldots \lambda _{n}/R$
are in
${\mathbb D}$
. Hence, by Theorem 4.2 in the case where
$\varphi =z^{2}$
and
$t=R^{2}$
, we have
$$ \begin{align*} \sum_{i,j=1}^{n}c_{i}\overline{c_{j}} \exp(\overline{\lambda_{i}}\lambda_{j}) &=e^{-R^{2}}\sum_{i,j=1}^{n}c_{i}\overline{c_{j}} \exp(R^{2}+\overline{\lambda_{i}}\lambda_{j})\\ &=e^{-R^{2}}\sum_{i,j=1}^{n}c_{i}\overline{c_{j}} \exp(R^{2}(1+\overline{(\lambda_{i}/R)}(\lambda_{j}/R)))\\ &=e^{-R^{2}}\sum_{i,j=1}^{n}c_{i}\overline{c_{j}} \exp\left(R^{2}\dfrac{1-\overline{\varphi(\lambda_{i}/R)}\varphi(\lambda_{j}/R)}{1-\overline{(\lambda_{i}/R)}(\lambda_{j}/R)}\right)>0 \end{align*} $$
for any
$(c_{1},\ldots , c_{n})^{\top }$
in
${\mathbb C}^{n}\setminus \{ \mathbf {0} \}$
.
Although the next result is just a simple consequence of Theorem 4.2, from the viewpoint of the theory of model spaces (see Garcia–Mashreghi–Ross [Reference Garcia, Mashreghi and Ross7]), it will be worth while mentioning it as a theorem.
Theorem 4.4 Let
$\varphi $
be an inner function. If
$\varphi $
is neither a constant nor
$e^{i\theta } z$
, then the kernel
$$ \begin{align*} k_{t}(z,\lambda)=\exp\left(t\dfrac{1-\overline{\varphi(\lambda)}\varphi(z)}{1-\overline{\lambda}z}\right) \quad (t>0) \end{align*} $$
is strictly positive definite.
Acknowledgment
The problem discussed in this paper was obtained in conversation about machine learning with Professor Kohtaro Watanabe (National Defense Academy). The referee gave the authors many significant comments on the previous version of this paper. In particular, the referee not only suggested the condition (C3), but also showed the authors the proof of the part related to (C3) in Lemma 4.1. Moreover, the proof of Proposition 2.1 has been simplified by the referee. The authors would like to express gratitude to them. This research was supported by JSPS KAKENHI Grant Number 20K03646.
