1. A hard problem: What is a group?

What is a group? More than a century into the study of groups and group identities, this question continues to vex. Human intuition suggests that groups exist (i.e., as far as we know, all typically developing humans on earth hold the mental concept of a social “group” as part of their perception of the world – it is a universal emic concept; Brown, Reference Brown1991). Research furthermore shows that people hold reliable and consistent intuitions about what is and is not a group (e.g., Hamilton, Sherman, & Castelli, Reference Hamilton, Sherman and Castelli2002). Thousands of scientific papers, hundreds of books (e.g., Brown, Reference Brown2000; Forsyth, Reference Forsyth2014/2019; Sedikides, Schopler, & Insko, Reference Sedikides, Schopler and Insko1998), and dozens of scientific journals (e.g., Advances in Group Processes, Group Dynamics, Group Processes & Intergroup Relations, etc.) are predicated on the existence of this construct. Yet what exactly are we talking about?

Almost all definitions agree that “group” means something more than just an aggregate and more than just a dimension of similarity out in the world (Campbell, Reference Campbell1958; Forsyth, Reference Forsyth2014/2019; Ip, Chiu, & Wan, Reference Ip, Chiu and Wan2006; Wilder & Simon, Reference Wilder, Simon, Sedikides, Schopler and Insko1998). A pile of leaves, for example, is not a group. A group is something more psychological, more agentic. Agency on its own, however, does not seem to be sufficient; people waiting for a bus are classically not a group, for example (Lickel et al., Reference Lickel, Hamilton, Wieczorkowska, Lewis, Sherman and Uhles2000). It seems then, that it is not enough that the entities are agents. There must also be coordination and some psychological representation among the individuals that they are so coordinated (Deutsch, Reference Deutsch and Jones1962; Sherif, Harvey, White, Hood, & Sherif, Reference Sherif, Harvey, White, Hood and Sherif1961; Tajfel, Reference Tajfel1982).

Thus, in more sophisticated definitions, we find a “relationship” (Lewin, Reference Lewin1948), “mutual awareness and interaction” (McGrath, Reference McGrath1984), “obligations,” “influence” (Shaw, Reference Shaw1981), “interdependence” (Lewin, Reference Lewin1948), and “social cohesion” existing between individuals – based on “a common identity” that people “define themselves as members of,” that “is recognized by at least one other” (Brown, Reference Brown2000) and that people “care about” based on shared “values,” “experiences,” “interests,” “kinship,” and so on (see Forsyth, Reference Forsyth2014/2019, for a review).

Undoubtedly, these definitions bring us closer to an adequate definition. The concept “group” of course refers to a very broad set of things. So a satisfactory definition – if it is to be inclusive – must be correspondingly broad and short on details. As Allport famously put it, “It is difficult to define an in-group precisely. Perhaps the best that can be done is to say that members of an in-group all use the term we with the same essential significance” (Allport, Reference Allport1954/1958, p. 30).

Nevertheless, we should not yet be fully satisfied with these current definitions. The problem – correct as they are – is that by relying on one or more additional psychological constructs, these definitions are still not yet grounded in concrete, operationalizable terms. For instance, imagine that an artificial intelligence (AI) engineer approaches us and wants to build a machine intelligence capable of “groups.” What exactly would we tell them? Groups involve the formation of “expectations,” “obligations,” and a meaningful “we.” But expectations and obligations about what, exactly? And what exactly is the essential significance of we? We might answer with obligations to “support one another,” to “come to one another's aid,” and so on. But this kind of explication still appeals to psychological concepts – all of which simply shift the goal posts. Already having an intuition or folk-psychological conception of groups is necessary to understand (and implement) what is meant by “support,” “aid,” “interdependence,” “we,” and so on. We are left then with an infinite regress of appeals to intuition. We know a group when we see it, in other words, but we couldn't say exactly what it is. Or, in the more precise language of the type/token distinction (Peirce, Reference Peirce, Weiss, Hartshorne and Burks1931–1935): We can recognize tokens (particular instances) of groups, but we don't have an explicit notion of what constitutes the type (the class itself).

In fact, there have been roughly two different ways of conceptualizing and studying groups in the behavioral sciences. The first seeks to capture how people intuitively represent and reason about groups, largely borne out of the ground-breaking theorizing of mid-twentieth century social psychology. The problem with this approach – as we have just seen – is that a necessary and sufficient definition of a group has not been forthcoming, and the definitions that do exist tend to remain rather psychological, if not verging on tautological.

A second approach has been to not rely on vague, psychological terms at all, but rather to operationalize the concept of a group as something concrete enough that it can be modeled or studied – which typically involves describing objective behaviors among multiple agents. This formalized study of n-person dynamics has been underway for several decades now – originating from a diverse set of fields including AI (Sandholm, Larson, Andersson, Sherry, & Tohmé, Reference Sandholm, Larson, Andersson, Sherry and Tohmé1999), evolutionary biology (Koykka & Wild, Reference Koykka and Wild2017; Rusch & Gavrilets, Reference Rusch and Gavrilets2020), the evolutionary social sciences (Böhm, Rusch, & Baron, Reference Böhm, Rusch and Baron2018; Glowacki, Wilson, & Wrangham, Reference Glowacki, Wilson and Wrangham2017), primatology (Harcourt & de Waal, Reference Harcourt and deWall1992), international relations (Schelling, Reference Schelling1966), and economics and decision-making (Schelling, Reference Schelling1956). These n-person dynamics have been studied in the lab (e.g., Bornstein, Reference Bornstein2003; Gonzalez, Ben-Asher, Martin, & Dutt, Reference Gonzalez, Ben-Asher, Martin and Dutt2015; Yamagishi, Jin, & Kiyonari, Reference Yamagishi, Jin and Kiyonari1999) and in the field (Bissonnette et al., Reference Bissonnette, Perry, Barrett, Mitani, Flinn, Gavrilets and de Wall2015; De Dreu & Van Vianen, Reference De Dreu and Van Vianen2001), and have been informed by a decades-long enterprise of game theoretic and optimality analyses (e.g., Burani & Zwicker, Reference Burani and Zwicker2003; Gamson, Reference Gamson1961; Mesterton-Gibbons et al., Reference Mesterton-Gibbons, Gavrilets, Gravner and Akcay2011; Shehory & Kraus, Reference Shehory and Kraus1998).

The problem with this second approach, however, is that these concrete implementations have not informed the issue raised by the first approach: namely, what – literally – is being represented in the mind when people are perceiving and reasoning about groups? For the purposes of defining a group, researchers in these areas are typically less concerned with directly assessing people's intuitions of what constitutes a group (as in the first approach), and so simply side-step this issue and declare by fiat that a particular set of behaviors, decisions, or processes constitutes a “group” for their particular purposes (i.e., they posit – either implicitly or explicitly – an operational definition). This declare-by-fiat strategy has afforded great progress, as it allows researchers to use mechanistic techniques (e.g., using agent-based or analytic models; e.g., Goldstone, Roberts, & Gureckis, Reference Goldstone, Roberts and Gureckis2008; Gross & De Dreu, Reference Gross and De Dreu2019).Footnote ¹

Nevertheless, the problem of defining a group sits awkwardly with respect to this second approach, as well. Namely, if the problem of defining a group were simply a matter of rendering the notion of a “group” into something concrete, then this second approach leaves us with an embarrassment of riches: There are dozens, if not hundreds, of concrete operationalizations of the concept “group” within this literature – including behaviors in the lab, the field, and the computerized world of agent-based models. Yet none of these seem to feed back onto the intuitive concept that they instantiate, such that we could point to any one concrete implementation or model and say “this is a group.” Or, more accurately, we would have to say that every single one is an instance of a “group” (at least to the intuition of the researcher and the reviewers who allowed the paper to be published as a study on “groups”) – all of which suggests that this second approach relies just as much on the human intuition of what constitutes a group as the first (albeit in a different way). In particular, intuition is being used to denote particular behaviors or dynamics as being about groups.

Consequently, as a scientific community, we have created a vast universe of group tokens – either described psychologically or operationalized concretely – without having a clear idea of what constitutes the type. Moreover, we now have the additional problem of not having a clear diagnosis of the original problem: that the problem of defining a group cannot simply be a matter of rendering the concept into something concrete. If it were, the second, concrete-operationalization approach would have already solved the problem of “What is a group?” long ago.

2. A solution: A computational theory

What is missing from current approaches to the study of groups – and what will finally resolve the issue of what is a group – is to have what the vision scientist David Marr (Reference Marr1982) referred to as computational theories: explicit information-processing theories of what the mechanisms that make up the mind are representing and operating upon when, pre-theoretically, one is reasoning about some phenomenon (which in this case would be “groups”).Footnote ² Computational theories resolve the long-standing tension between the two approaches described above by (1) explicitly acknowledging the importance of psychological representation – as in the first approach (i.e., they do not naively place the notion of a “group” out in the world, but rather, as a representation within the mind), while at the same time (2) grounding that psychological representation in something concrete, objective, and non-tautological – as in the second approach.

Computational theories highlight the issue of computational adequacy: that if what one has stipulated as going on within some information-processing entity is adequate for creating the phenomenon of interest, without intervention from a god-like agent or intelligence (Chomsky, Reference Chomsky1980; Marr, Reference Marr1982; Minsky, Reference Minsky1961, Reference Minsky1974; Pietraszewski & Wertz, Reference Pietraszewski and Wertz2021; Tooby & Cosmides, Reference Tooby, Cosmides, Barkow, Cosmides and Tooby1992). This issue of computational adequacy differentiates the present approach from what we have described as the second, concrete-operationalization approach to the study of groups. Nearly all of that work takes as its initial starting point the imposition of some sort of predefined structure onto the world – typically a game structure, such as the prisoner's dilemma game (or kindred snow-drift game; see Axelrod, Reference Axelrod1984; Oliver, Reference Oliver1993; Perc & Szolnoki, Reference Perc and Szolnoki2010; Vainstein, Silva, & Arenzon, Reference Vainstein, Silva and Arenzon2007; Worden & Levin, Reference Worden and Levin2007, for reviews). These and other predefined structures render the enterprise tractable, allowing researchers to not have to worry about certain pesky details.

In contrast, on our computational-theoretic approach, we are not allowed to think that the world can come preformed into games or structure. Instead, the representational system itself must impose some sort of framing onto the blooming, buzzing confusion of the real world. That is, the representational system has the job of imposing its own “game” structure onto the real world, and then “playing” an internally represented game, by using cues in the real world to run its simulations. As scientists, we then face the same problem as that faced by the mind: determining what – out of all the things that humans are doing out in the real world – constitute “group” things. Or, in the parlance of AI and philosophy, we are forced to confront the relevance problem (e.g., Dennett, Reference Dennett and Pylyshyn1988).

Colloquially, then, our computational-theoretic approach can be thought of in the following way: We are engineers, and our job is to build a robot that can interface with the real world. The question, “What is a group?”, then becomes a question about what could in principle be a group representation be in such a robot. That is, how does our robot “see” groups? What is the definition of a group within its software?Footnote ³

The proposed computational theory is as follows: The folk-psychological construct “group” corresponds to changes within a framework of event types (what computer scientists and AI researchers would refer to as an event grammar or event calculus; e.g., Mueller, Reference Mueller2015). Within this framework, a group representation corresponds to the probabilistic assignment of agents to particular roles within those event types.

Here, we will consider event types featuring cost impositions. We are thereby addressing what a “group” representation is in the context of conflict. Doing so is not meant to suggest that there are only conflict-based group representations within the mind. Rather, we simply have a plausible account for conflict – which is no trivial matter, as our account must describe what is universally true of all instances of group membership in all instances of real or imagined conflict, all the while describing that representation in its most compressed, reduced form. At the end of the paper, we will speculate as to how to think about other, non-conflict-based event types, and thereby make progress toward a complete computational theory of social groups.

The remainder of the paper will explain the proposed conflict-based event-type computational theory of a group representation – all in a conceptual, non-technical manner – and will try to convey a sense of what kinds of research questions it opens up, all while attempting to head-off a number of likely misunderstandings.

3. Starting with the reduction problem

Any computational theory must first deal with what is called the reduction problem in AI: how to take an apparently unbounded, infinite phenomenon and reduce it to a finite, solvable set (Russell & Norvig, Reference Russell and Norvig1995). For example, vision researchers must postulate a finite set of cognitive primitives that allow a viewer to identify and recognize any and all objects (e.g., Marr & Nishihara, Reference Marr and Nishihara1978). Analogously for groups, we must postulate a finite number of cognitive primitives that allow all tokens of group conflict – from playground fights to international conflict, and everything else in between – to be represented and thereby operated upon within the mind.

A good conceptual starting point for solving the reduction problem for group representation is to start with a broader reduction problem: that of multi-agent (or n-person) conflict, which refers to when more than two agents come into conflict with one another. For decades, the complexity and near-infinite variety of n-person conflict has posed a problem: How can a finite set of mental representations and decision-rules handle the complex dynamics that arise when more than two agents come into conflict with one another (e.g., Byrne & Whiten, Reference Byrne and Whiten1988; Harcourt & deWall, Reference Harcourt and deWall1992; Sherratt & Mesterton-Gibbons, Reference Sherratt, Mesterton-Gibbons, Hardy and Briffa2013)? How, in other words, can the mind “see” all instances of multiperson conflict – of which group-related phenomenon are but a subset?

A solution to this problem was offered recently (Pietraszewski, Reference Pietraszewski2016), and while a solution to this broader reduction problem of n-person conflict does not solve the specific problem of what a group representation is, it does narrow down the scope of possible solutions – in the same way that knowing the answer to the question “Is it bigger than a bread-box?” narrows down the scope of possible solutions in the game of 20 questions. This n-person solution was discovered by asking the question, “What would always be true of n-person conflict over evolutionary time?”Footnote ⁴ From the multigenerational perspective of evolutionary time, the particular details of conflict-related events will always be different. Who is involved, why, and the nature of the conflict itself will not be stable across multiple generations. Therefore, psychological processes for handling conflict cannot be too-tightly tied to any of these particular details, but rather must operate over a class of abstracted invariances (Jackendoff, Reference Jackendoff1992). Once we strip these ever-changing details away, what remains are the following principles:

• • Conflict can be understood as the contingent delivery of costs. An inclusive definition conceptualizes conflict as the contingent delivery of costs between agents; an expectation or realization of the contingency if you do X, I will do Y (Archer, Reference Archer1988; Campbell, Reference Campbell and Buss2015; Daly, Reference Daly and Buss2015; Hardy & Briffa, Reference Hardy and Briffa2013). This conceptualization accommodates exchanging costs in kind – exchanging an eye for an eye and a tooth for a tooth – and accommodates an exchange of different costs. For example, when a child pushes another for stealing a toy, they are exchanging one cost for another, repaying lost enjoyment with physical harm.

• • N-person conflict can be decomposed into triadic – but not dyadic – interactions. What sets multi-agent or group conflict apart from dyadic conflict is the involvement of a third party. For example, if A attacks B, and then in response B attacks A, and so on and so forth, this may become a protracted conflict, but it will only ever become a multi-agent conflict if at least one third party, C, becomes involved (Caplow, Reference Caplow1959; Grammer, Reference Grammer, Harcourt and de Waal1992; Harcourt, Reference Harcourt, Byrne and Whiten1988; Harcourt & deWall, Reference Harcourt and deWall1992; Heider, Reference Heider1958; Liska, Reference Liska1962; Patton, Reference Patton1996, Reference Patton, Cronk, Chagnon and Irons2000; Pietraszewski, Reference Pietraszewski2012, Reference Pietraszewski2016; Strayer & Noel, Reference Strayer, Noel, Zahn-Waxler, Cummings and Iannotti1986; Von Neumann & Morgenstern, Reference Von Neumann and Morgenstern1944).

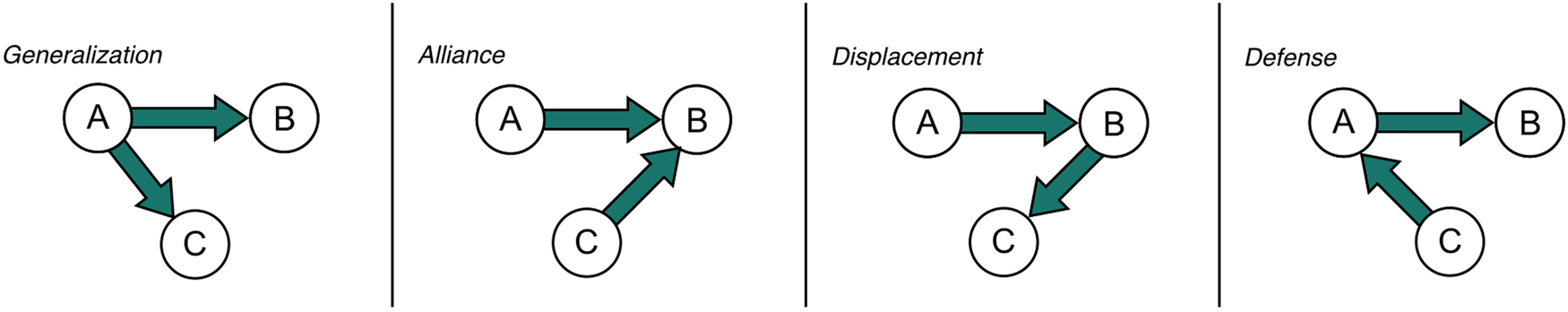
• • There are only four ways that a third party can become drawn into a conflict within these triads. If A attacks B, and a third party, C, becomes involved, there are only four possible ways in which this might happen (Chase, Reference Chase1985; Strayer & Noel, Reference Strayer, Noel, Zahn-Waxler, Cummings and Iannotti1986), depicted in Figure 1:

  1. 1. Generalization: A attacks B, then A attacks C

  2. 2. Alliance: A attacks B, then C attacks B

  3. 3. Displacement: A attacks B, then B attacks C

  4. 4. Defense: A attacks B, then C attacks A

Figure 1. The four ways in which a third party (C) can become involved in an otherwise dyadic conflict between A and B. From left to right, A's attack on B is followed by: A also attacking C; C attacking B; B attacking C; C attacking A. These are Generalization, Alliance, Displacement, and Defense, respectively.

These triadic interactions characterize what is always true of n-person conflict. They are the smallest set of “building block” units that can be strung together over time to describe an infinite number of conflict dynamics – similar to the way a finite number of letters can generate an infinite number of linguistic meanings. Past work had used these four triadic interactions as a coding scheme for documenting real-world multi-agent conflict (Chase, Reference Chase1985; Strayer & Noel, Reference Strayer, Noel, Zahn-Waxler, Cummings and Iannotti1986). However, these triadic interaction types – because they describe all of the ways in which a third party may become involved in a conflict – may also be the computational building blocks out of which all n-person conflicts are cognitively represented (Byrne & Whiten, Reference Byrne and Whiten1988; Harcourt & deWall, Reference Harcourt and deWall1992; Sherratt & Mesterton-Gibbons, Reference Sherratt, Mesterton-Gibbons, Hardy and Briffa2013). We will refer to these as triadic primitives.

Crucial to this proposal is that (1) any one agent can occupy each of the 12 different roles over time (i.e., A, B, and C are representational slots into which particular agents are inserted; they are not agents themselves) and (2) that these triadic interactions will string together or concatenate over time as interactions unfold. Consequently, the human mind need not represent an infinite number of conflict dynamics: it need only reason about each of these four interaction types, and then string them together over time as real or hypothetical events unfold. Such an architecture – described at this high level of abstraction of strings of concatenated triadic primitives – solves the reduction problem with respect to n-person conflict, allowing a finite cognitive architecture to represent an infinite variety of conflict dynamics (see Pietraszewski, Reference Pietraszewski2016, for details).

4. The proposed solution: The mind's definition of a “group” in the context of conflict

With the broader n-person reduction problem tentatively addressed, we can turn back to the original problem of characterizing the mind's definition of group in the context of conflict: If the above four triadic primitives describe all multi-agent conflict, then it would seem likely that the concept of “group” is somehow present in these triadic primitives. But how? Strings of triadic primitives do not yet map onto or produce the intuitive concept “group.” How do we go from these triadic primitives to the concept of “group”?

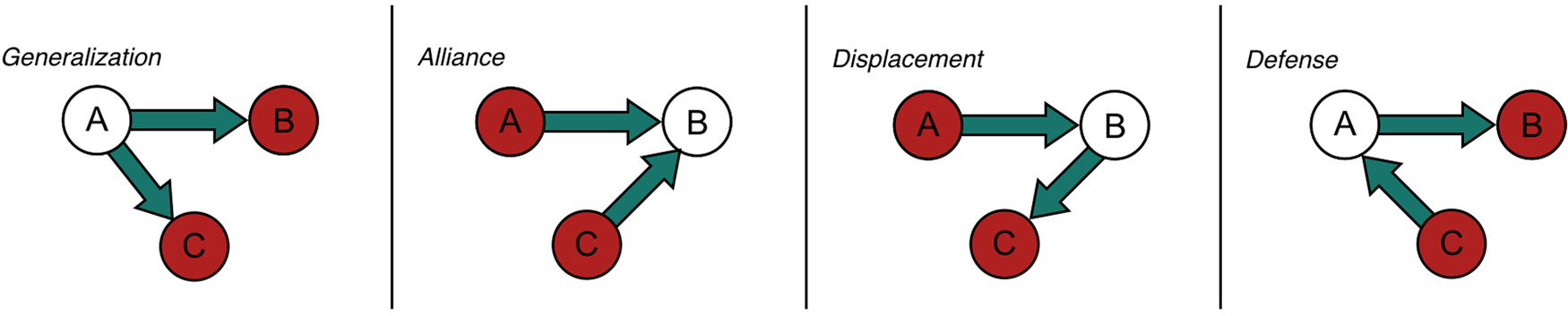
The proposed solution is as follows: a group (in the context of conflict) is a set of specific roles within these four triadic primitives, where role simply refers to one of the three interactant-slots within each triadic primitive (e.g., being A in Generalization). These roles are depicted in Figure 2:

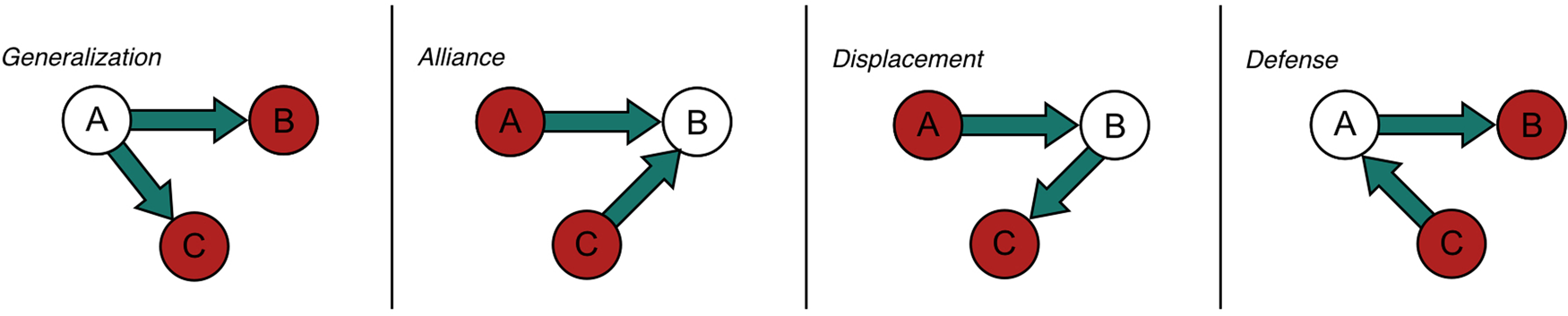
Figure 2. The roles that define the mental concept “group” in the context of conflict (depicted in red). These we will call group-constitutive roles.

What it means that individuals are members of a group, then, is that:

1. (i) in generalization, they are in roles B and C

2. (ii) in alliance, they are in roles A and C

3. (iii) in defense, they are in roles B and C

4. (iv) in displacement, they are in roles A and C

These group-constitutive roles (again, with roles referring to the agent and/or patient roles taken within the triadic primitives) ground “choosing one agent over another” and “taking sides” in a conflict into a finite set of mental representations. The precise, casual claims are as follows:

• • Cost/benefit calculations within each individual agent produce the above triadic interactions.

• • Certain classes of cost/benefit calculations will cause certain agents to occupy the above roles with respect to one another.

• • This class of cost/benefit calculations “hang together” over evolutionary time. That is, the relationships between agents that cause them to occupy the above roles with respect to one another constitute an enduring entity over evolutionary time.

• • This enduring entity allows for selection to create a summary representation in the mind. This is a mental entry for agents who are in the kind of relationship which causes them to occupy these particular roles with respect to one another.

• • That summary representation – precisely, the group-constitutive roles within the four triadic primitives – is our mental concept “group” in the context of conflict.

The claim, then, is that on-the-ground relationships will cause agents to occupy these roles with respect to each other. That there are such relationships creates a conceptual entry or slot in our minds over evolutionary time. What defines this entry – and what is common to all of these relationships – is that agents will occupy these roles with respect to one another.

The order of causation is on-the-ground relationships and cost/benefit calculations first, summary representation second. Individuals are making cost/benefit decisions over evolutionary time, based on a number of factors, such as self-interest, caring about the welfare of another, a formal social contract, and so on. These first-person decisions then create an invariance in the world – a class of events (in this case it is the occupation of group-constitutive roles) – that can in turn select for a conceptual entry that summarizes this class (Shepard, Reference Shepard1994).

This summary representation allows agents to communicate about and refer to this class, both within themselves, and also with others. For instance, an agent can observe their own behavior and motivations, and create a summary representation of who they are in a “group” with, and who they are not. Or they can observe other's behaviors and do the same. Of course, by “agent” we mean a set of information-processing functions (as opposed to a conscious agency within the mind; see Pietraszewski & Wertz, Reference Pietraszewski and Wertz2021). As such, there is no entailment that each (token) summary representation becomes conscious and communicable to others, although undoubtedly a subset does.Footnote ⁵ These communicable summary representations (which would in principle be highly compressed signifiers) should, when communicated, induce an expectation of the class of events to which they refer. That is, if one is told that X and Y are in a “group” within a conflict, then this should lead to the expectation that X and Y will be more likely to occupy the above roles with respect to one another, all else equal.

5. An example: Understanding group-constitutive roles from the perspective of a particular agent

What does it mean that group-constitutive roles are group membership to the human mind? In the examples that follow, we will consider how group-constitutive roles play out from the perspective of a particular agent. These observations, which are entailments of our computational theory, describe additional elements of the overarching information-processing problem of representing, reasoning about, and carrying out the group-constitutive roles. For instance, we will see that occupying group-constitutive roles requires our focal agent to sometimes be motivated to act, to anticipate the actions of others, and to make certain cost/benefit calculations.

We will begin with Figure 3. In the top panel of Figure 3, a third agent, denoted C, observes A attack B. If this agent is to become involved in the conflict, then some constellation of the four possible triadic interaction types will occur. However, as outside observers who do not know if they share a group membership with either A or B, we have no basis for narrowing down which will occur. In contrast, once we know that the onlooking agent is in a group with A and not with B (Fig. 3, bottom left panel), only two of the four interaction types become likely, and the other two become less likely. In contrast, if the onlooking agent is in a group with B and not with A (Fig. 3, bottom right panel), then the other two triadic interaction types become likely. Thus, the representation of the background relationship – the “group” membership – between the direct interactants (A and B) and the onlooker (C) modifies which behaviors are most likely to follow from A attacking B.Footnote ⁶

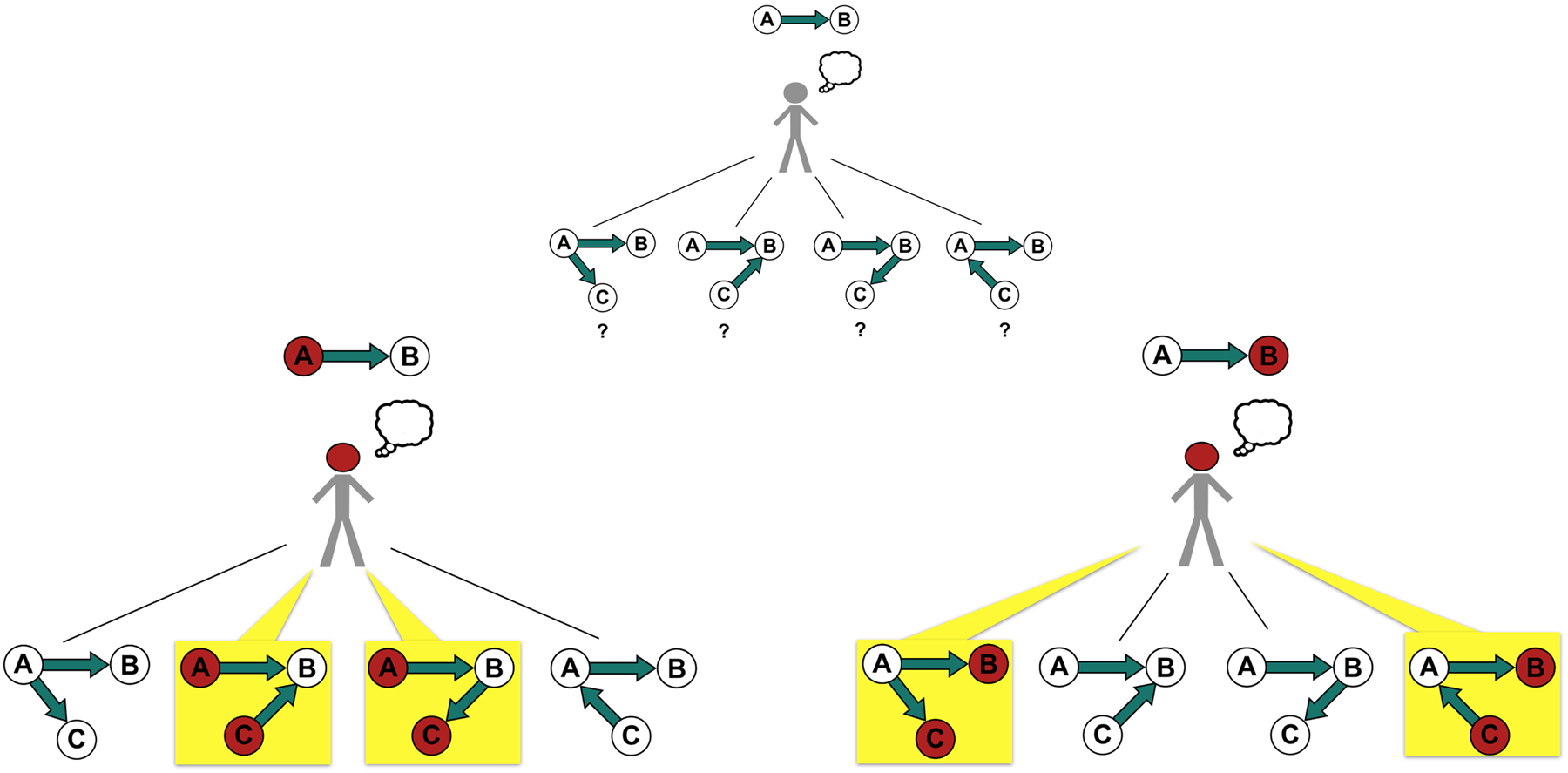
Figure 3. A single agent (depicted as the stylized figure) observes a cost imposition occurring between A and B (top panel). Without knowing the group memberships of A, B, and the agent, we have no basis for anticipating which, if any, of the four possible triadic interactions are likely to happen as a consequence of A attacking B. However, once the group membership of the three agents is marked (group membership is denoted in red in the bottom two panels), certain triadic interactions become more likely to occur (those that are highlighted), whereas others become less likely to occur (those that are not highlighted).

Notice that for these triadic interactions to occur, the initially uninvolved onlooker must at times behave. For example, if the uninvolved agent C shares a group membership with A, then they will join with A in attacking B (the first highlighted interaction type in the bottom left panel of Fig. 3). This means that the psychological implementation of these group-constitutive roles requires motivations to act: In this case, group membership is a relationship between A and C that is causing C to be more likely to act against B. Notice, too, that C must sometimes also generate expectations of other's actions. For example, C in this example is also more likely to be attacked by B, given that A and C are group members (the second highlighted interaction type in the bottom left panel of Fig. 3). This means that the psychological implementation of these group-constitutive roles also requires producing expectations about others' behaviors.

The interplay between one's own behavior and the expectations of other's behavior can also be seen in Figure 4, which again depicts group membership from the perspective of a single individual, who is now in the role of A, the attacker. What happens after A attacks B will depend on the group membership of the uninvolved onlooker C. If that onlooker is in a group with the attacker, then their presence is a benefit from the attacker's perspective (bottom left panel of Fig. 4); they may also join in attacking the victim, helping diffuse the costs and risks of the conflict. Or the victim may retaliate against the attacker's fellow group member rather than the attacker themselves, thereby diffusing the risk of counterattack. In contrast, if the onlooker is in a group with the victim, the likely next steps are costly to the aggressor (bottom right panel of Fig. 4): The aggressor will either be motivated to also attack the onlooker, requiring additional cost and risk. Or, in response to the initial attack on their fellow group member, the onlooker will in turn attack the aggressor. Neither event benefits the aggressor. Consequently, if aggressor A can anticipate these outcomes before attacking B, then A will be more likely to attack when A's group member is present (right panel), but will be less likely to attack when B's group member is present (left panel). This is the causal logic for why the presence or absence of group members changes decision thresholds for engaging in or avoiding conflict. This example demonstrates the strategic benefits of representing the group membership of uninvolved third parties, and how one's own decision-making is aided by being able to anticipate how others will behave.

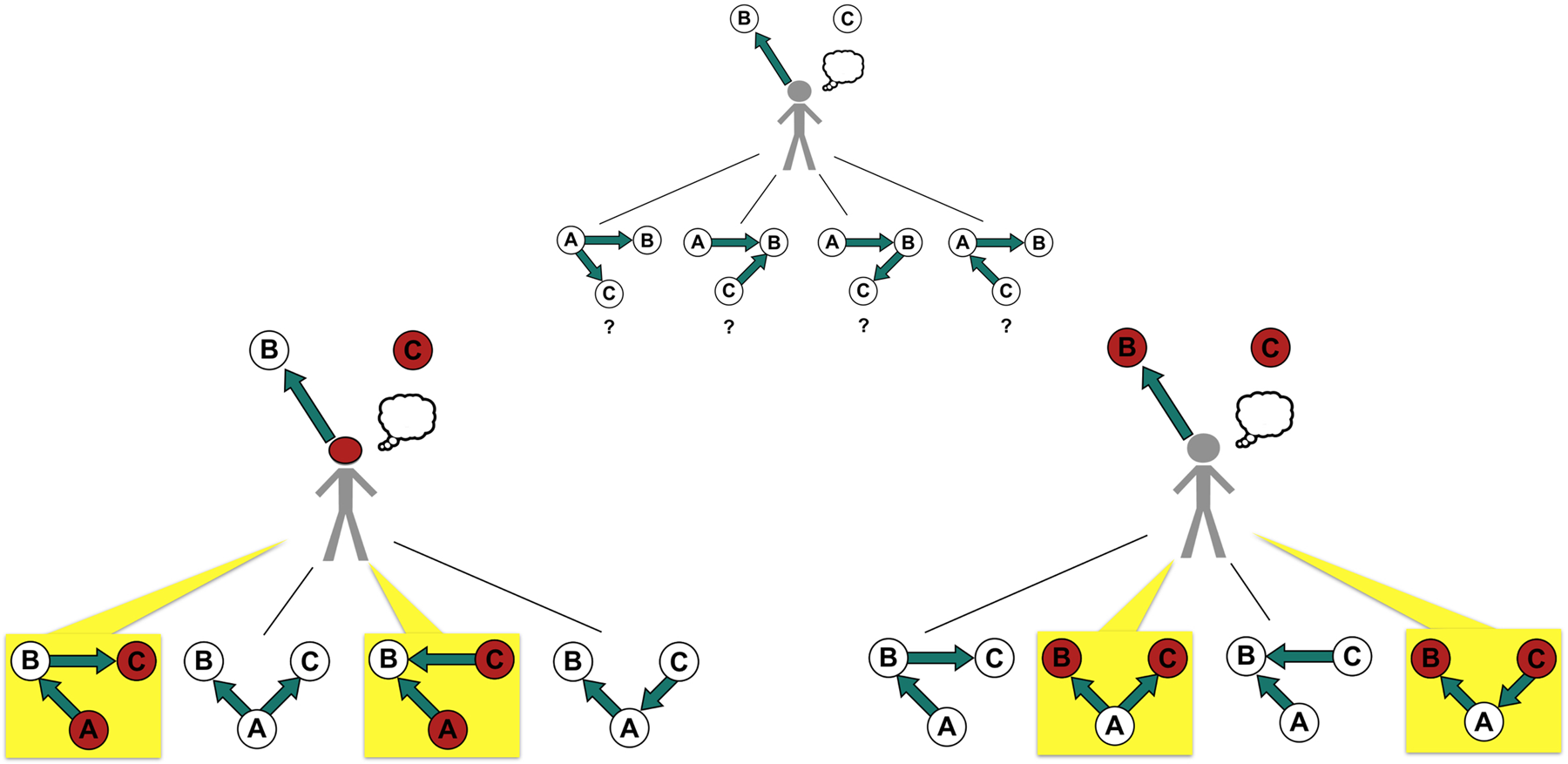
Figure 4. The single agent is now in role A – the cost imposer. Again, without knowing group membership we have no basis for anticipating which, if any, of the four possible triadic interactions are likely to happen (top panel). However, once group membership is marked (in red; bottom panels), certain next steps become more likely than others (those highlighted in yellow).

Finally, Figure 5 depicts the perspective of the initial victim. Here, the initially uninvolved onlooker can either be a group member with B (left panel) or with A (right panel). Again, there is a cost/benefit asymmetry between these two scenarios: It will be less costly for the victim to be attacked in the presence of a fellow group member (left panel) than in the presence of a group member of the attacker (right panel). Furthermore, our victim B will sometimes be motivated to act (e.g., attacking C when A and C are group members) and will sometimes anticipate other's actions (e.g., expecting C to attack A on his or her behalf when B and C are group members). For detailed cost/benefit analysis of why these particular events may unfold, see Pietraszewski (Reference Pietraszewski2016, SOM).

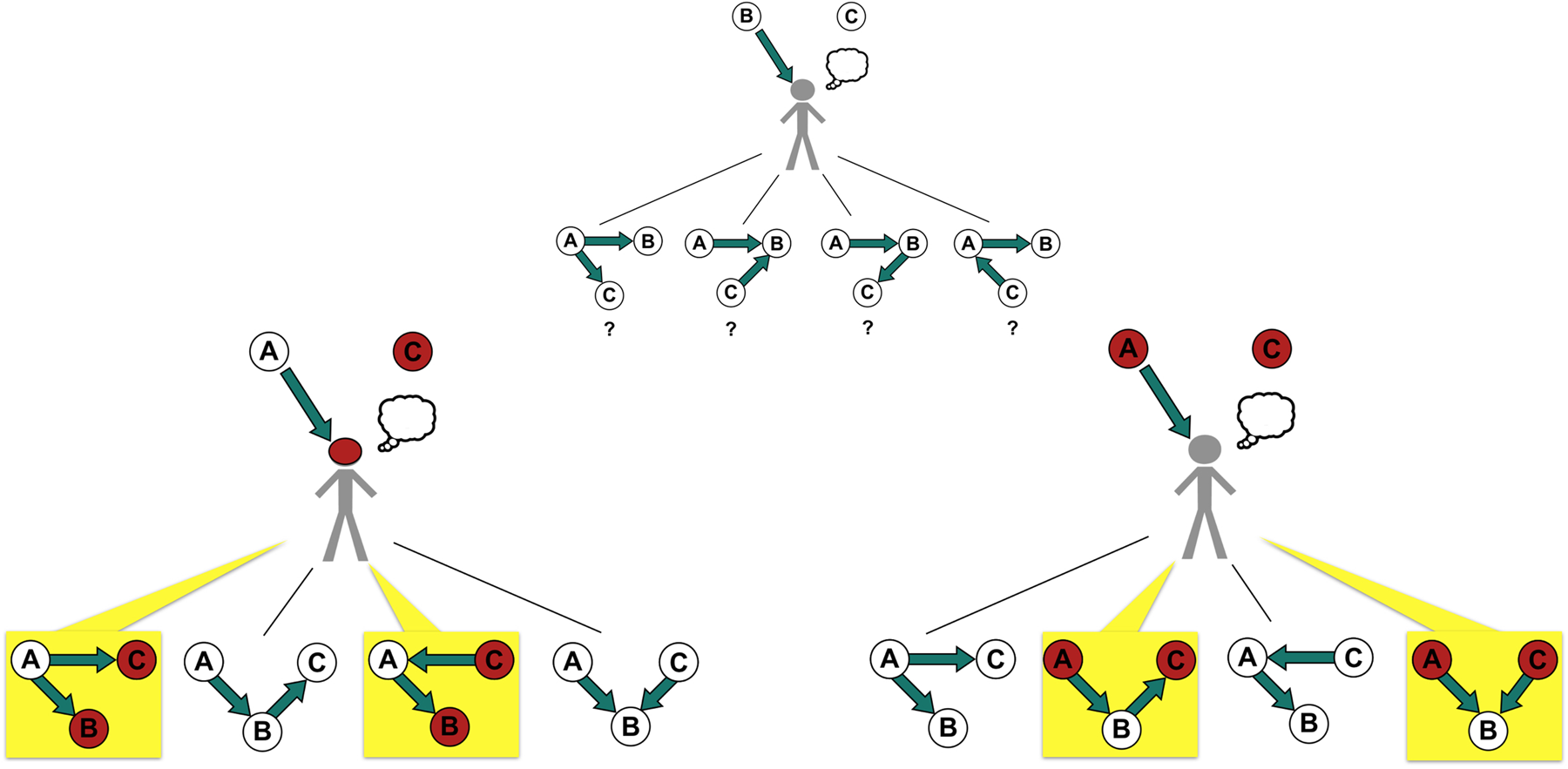
Figure 5. The single agent is now in role B – the victim of the initial cost imposition. Again, with knowing group membership, we have no basis for anticipating which, if any, of the four possible triadic interactions are likely to happen (top panel). However, once group membership is marked (in red; bottom panels), certain next steps become more likely than others (those highlighted in yellow).

6. Clarifying the definition

We can now refine and clarify the definition: Roughly, somewhere in the mind are floating around the representations [group] and [conflict]. When these co-occur, what is bound to this composite [group-in-the-domain-of-conflict] representation are these group-constitutive roles within the triadic primitives. That is, these roles are the mind's operational or functional definition of a group in the context of conflict, and these triads are the skeletal primitives out of which each instance (token) of a group is perceived and built (and we will see in a moment how all of this scales up). In other words – and tautologically, given our definition – to the degree agents belong to the same group, they will be more likely to act according to these roles, and will also anticipate that other group members will do the same.

This definition entails a minimal bound for what the mind considers a group (conflict) dynamic: Minimally, two agents must be within the group, and a single agent must lie outside of it (where “group” is defined non-tautologically by the occupation of group-constitutive roles). For example, if we imagine a conflictual world with only three agents, in which two are allied and a third is not, then we would see over time the two allied individuals occupying each of the roles that define a group when engaging in conflict with the third lone individual. The two in conflict with the lone individual would constitute a group, according to the definition.

Furthermore, the group-constitutive roles define “group” from a third-party perspective. Agents who are not involved in a particular conflict can nevertheless infer these roles by observing the actions of others, and who occupies these roles will define for these third parties who is in a group with whom. Of course, the roles are mental representations and so cannot just be seen (in the same way that an object cannot just be seen; Marr, Reference Marr1982). Therefore, a substantial amount of input analysis is required to go from the detection of on-the-ground behaviors (such as seeing A shooting at B, hearing about X gossiping about Y, and so on) to the inference that these behaviors are an instance of one of these roles; a problem that is far from trivial. Moreover, contingent cost-infliction is often a drawn-out process, with many gaps and lulls between interactions. For example, if you steal my cattle today, it may be a day, a month, or even a year before I retaliate. Therefore, delays between cost-inflictions will have to be allowed for, as will some opacity of who has done what to whom. It follows then that identifying who is in what group requires understanding who has imposed which costs on whom, and in response to what past behaviors. Inferring group membership is then in part an attribution problem.

Crucially, behaviors will not be the only cues used to infer the occupation of the group-constitutive roles. In particular, because the relationships that cause agents to occupy group-constitutive roles with one another constitute an evolutionary invariance (at a particular level of abstraction), natural selection can “see” what cues correlate with these relationships, and can build learning systems that spawn probabilistic group-constitutive role representations in response to them. Consequently, even very sparse cues that do not have much to do with conflict when looked at superficially (e.g., Dunham, Reference Dunham2018; Pietraszewski, Reference Pietraszewski, Banaji and Gelman2013, Reference Pietraszewski, Van Lange, Higgins and Kruglanski2020) can nevertheless probabilistically elicit these group-constitutive role representations. We will refer to these cues as ancillary attributes of groups (ancillary because they are not behaviors corresponding to the group-constitutive roles themselves, but rather are cues that predict the occupation of these roles, either over evolutionary or developmental time).

What attention to ancillary attributes means, is that even in a world in which the only reason to attend to “groups” is to predict conflict-related outcomes, group tokens that superficially have little or nothing to do with conflict will nevertheless be attended to: a point that has interesting consequences for our understanding of the folk concept “group” – namely, it raises the issue of whether or not the concept “group” can ever be divorced from conflict-related representations no matter how apparently peaceful the group token is. In practice, both ancillary attributes and behaviors corresponding to group-constitutive roles are likely to be present in the world. An additional information-processing problem is to then link up these diverse representations to the correct group token.

In sum, the information-processing within the mind that makes it possible to consider, plan, and generate the above group-constitutive roles is what constitutes the psychology of groups-in-conflict. Furthermore, this psychology is recursive in the sense that I not only expect group members to occupy these roles, I expect that others will also expect this, and so on. Finally, we have so far been treating behavior as all-or-none – that if someone is a group member with someone else, they will produce behaviors X, Y, and Z. Of course, this is an oversimplification, so more fleshed out computational theories can incorporate something like a continuously scaled threshold to act, as part of a larger “motivational” goal-tree/difference-reduction architecture.

7. Scaling up

The architecture for representing each of the triadic primitives is based upon interactions between individual agents. However, at times, multiple agents will all behave the same way within a particular triadic primitive (e.g., a set of agents will all occupy role C in Defense). The architecture can therefore scale up the representation by inserting (or substituting) that entire set of agents into the single-agent slot (the group-constitutive role). This one-to-many substitution allows the same (or a slightly modified) architecture to handle much larger numbers of agents simultaneously. Moreover, to the degree that that particular set of agents are in a group – per the definition – they are permitted to be inserted into all of the agent slots within all of the triadic primitives depicted in Figure 2, which enables an infinitely hierarchical scaling up of nested group representations. This kind of architecture allows us to represent, for example, that Japan and Germany occupied group-constitutive roles with respect to one another during World War II, as did Britain and the United States, without having to calculate the actions of the millions of individuals involved (see also Fiske & Neuberg, Reference Fiske and Neuberg1990; Insko & Schopler, Reference Insko, Schopler and Hendrick1987).

Of course, any one of the roles within these triadic primitives may also be produced for reasons other than group membership. For example, an agent, C, who witnesses A attacking B, may retaliate against A, not because she is a group member with B, but because A's attack was morally reprehensible, and should be punished. Indeed, even if multiple agents all decided to attack B, thus filling the role C in Defense, this would still not constitute a group. Groups, instead, are a special class of relationships in which all of the above roles either will occur, or are expected to occur. That is, group membership applies to all of the triadic primitives. If only a subset are shown to hold, this should hurt the representation that such a relationship is a group.

For example, if we were to demonstrate that all of the agents filling role C in the above example are not willing to place themselves in any of the other group-constitutive roles with respect to A, aside from this one case, then they would not be seen as constituting a group with A. That is, they are not group members precisely because these agents do not occupy all of the above roles with respect to one another. Indeed, different collections of agents can occupy some, all, or none of the above group-constitutive roles with respect to one another. This variation must be represented by the mind, and this represented variation may explain why there is a perceived continuum of “groupishness” or entitativity across different collections of people (Campbell, Reference Campbell1958; Hamilton et al., Reference Hamilton, Sherman and Castelli2002; Ip et al., Reference Ip, Chiu and Wan2006; Pietraszewski, Reference Pietraszewski2016) – an idea that is imminently testable.

8. The utility of the definition

So what does having a plausible theory of a group representation in the context of conflict buy us?

9. Next steps

10. Toward a complete computational theory of social groups – including non-conflict-based representations

Finally, because agents can also coordinate and cooperate with one another – and there is nothing in our conflict-based event types to allow for the representation of such events – the current theory of what constitutes a group representation is not yet complete. Therefore, even if correct, the present account will need to be supplemented with (at minimum) additional event types – and there are a number of existing theories and taxonomies that can be repurposed as plausible hints at what these types might be (e.g., Alexander, Reference Alexander1987; Balliet, Tyber, & Van Lange, Reference Balliet, Tybur and Van Lange2017Footnote ¹¹; Heider, Reference Heider1958; Tatone, Geraci, & Csibra, Reference Tatone, Geraci and Csibra2015).

Although the current proposal is silent on what these might be, we can at least speculate that a plausible (if not obvious) set of candidate event types for something like polyadic cooperation might be something akin to sets of direct reciprocity (A gives to B, B repays in kind over time) and indirect reciprocity (A gives to B, as a consequence, B gives to C; see Alexander, Reference Alexander1987). However, this is just speculation, and there may need to be additional elements to make these computationally adequate, or something more specific may be necessary – issues that await future work. What we can say with some certainty is that neither direct nor indirect reciprocity can account for the current conflict-based event types (i.e., these are not isomorphic with respect to one another)Footnote ¹² – which means that a complete computational theory of groups will likely be comprised of multiple event-type frameworks.

In the search for a complete computational theory of group representation, care will have to be taken to conceptually-distinguish between two different enterprises: The first is to provide a computationally adequate account of every group token. In this respect, each token will likely be a composite of a number of event types (in the same way that an object representation is a composite of visual information-processing types, like lines and colors). A second enterprise is to explain why people can think about and understand the over-arching category “group,” and why they agree about a continuum of groupishness across these tokens. The first enterprise will require a proliferation of types. The second will require some unification or integration of those types. There are many more reduction problems to be solved, in other words, than just the problem of multi-agent conflict dynamics covered here. The principle of computational adequacy – combined with considerations of what is both necessary and sufficient – will have to guide these future efforts.

11. Summary and conclusion

Despite an enormous literature on groups and group dynamics, little attention has been paid to explicit computational theories of how the mind represents and reasons about groups. The goal of this paper has been, in a conceptual, non-technical manner, to propose a simple but non-trivial framework for starting to ask questions about the nature of the underlying representations that make the phenomenon of social groups possible – all described at the level of information-processing. This computational theory, when combined with many more such theories – and followed by extensive task analyses and empirical investigations – will eventually contribute to a full accounting of the information-processing required to represent, reason about, and act in accordance with group representations.