Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Conti, Sergio
Fonseca, Irene
and
Leoni, Giovanni
2002.
A Γ‐convergence result for the two‐gradient theory of phase transitions.
Communications on Pure and Applied Mathematics,
Vol. 55,
Issue. 7,
p.
857.
Fonseca, Irene
and
Leoni, Giovanni
2002.
Variational Methods for Discontinuous Structures.
p.
117.
Ambrosio, Luigi
Kirchheim, Bernd
Lecumberry, Myriam
and
Rivière, Tristan
2002.
Nonlinear Problems in Mathematical Physics and Related Topics II.
Vol. 2,
Issue. ,
p.
29.
Alouges, François
Rivière, Tristan
and
Serfaty, Sylvia
2002.
Néel and Cross-Tie Wall Energies for Planar Micromagnetic Configurations.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 8,
Issue. ,
p.
31.
Desimone, Antonio
Kohn, Robert V.
Müller, Stefan
and
Otto, Felix
2002.
A reduced theory for thin‐film micromagnetics.
Communications on Pure and Applied Mathematics,
Vol. 55,
Issue. 11,
p.
1408.
De Lellis, Camillo
2002.
An example in the gradient theory of phase transitions.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 7,
Issue. ,
p.
285.
Conti, Sergio
DeSimone, Antonio
Dolzmann, Georg
Müller, Stefan
and
Otto, Felix
2003.
Trends in Nonlinear Analysis.
p.
375.
De Lellis, Camillo
and
Rivière, Tristan
2003.
The rectifiability of entropy measures in one space dimension.
Journal de Mathématiques Pures et Appliquées,
Vol. 82,
Issue. 10,
p.
1343.
Ercolani, N
Indik, R
Newell, A.C
and
Passot, T
2003.
Global description of patterns far from onset: a case study.
Physica D: Nonlinear Phenomena,
Vol. 184,
Issue. 1-4,
p.
127.
Kohn, Robert V.
and
Yan, Xiaodong
2003.
Upper bound on the coarsening rate for an epitaxial growth model.
Communications on Pure and Applied Mathematics,
Vol. 56,
Issue. 11,
p.
1549.
Ambrosio, Luigi
Lecumberry, Myriam
and
Rivière, Tristan
2003.
A viscosity property of minimizing micromagnetic configurations.
Communications on Pure and Applied Mathematics,
Vol. 56,
Issue. 6,
p.
681.
Rivière, Tristan
and
Serfaty, Sylvia
2003.
Compactness, Kinetic Formulation, and Entropies for a Problem Related to Micromagnetics.
Communications in Partial Differential Equations,
Vol. 28,
Issue. 1-2,
p.
249.
Pisante, Giovanni
2004.
Homogenization of micromagnetics large bodies.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 10,
Issue. 2,
p.
295.
Ercolani, Nick
and
Taylor, Michael
2004.
The Dirichlet-to-Neumann map, viscosity solutions to Eikonal equations, and the self-dual equations of pattern formation.
Physica D: Nonlinear Phenomena,
Vol. 196,
Issue. 3-4,
p.
205.
Conti, Sergio
DeSimone, Antonio
and
Müller, Stefan
2005.
Self-similar folding patterns and energy scaling in compressed elastic sheets.
Computer Methods in Applied Mechanics and Engineering,
Vol. 194,
Issue. 21-24,
p.
2534.
Poliakovsky, Arkady
2005.
A method for establishing upper bounds for singular perturbation problems.
Comptes Rendus. Mathématique,
Vol. 341,
Issue. 2,
p.
97.
Alouges, François
and
Labbé, Stéphane
2006.
Convergence of a ferromagnetic film model.
Comptes Rendus. Mathématique,
Vol. 344,
Issue. 2,
p.
77.
Mayergoyz, Isaak D.
2006.
The Science of Hysteresis.
p.
269.
Glasner, K.B.
2006.
Grain boundary motion arising from the gradient flow of the Aviles–Giga functional.
Physica D: Nonlinear Phenomena,
Vol. 215,
Issue. 1,
p.
80.
Conti, Sergio
and
Schweizer, Ben
2006.
A Sharp-Interface Limit for a Two-Well Problem in Geometrically Linear Elasticity.
Archive for Rational Mechanics and Analysis,
Vol. 179,
Issue. 3,
p.
413.
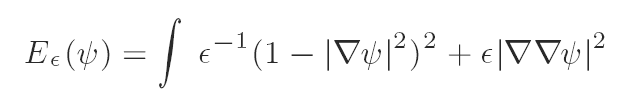 in the plane. As ε → 0, this functional favours |∇ψ| = 1 and penalizes singularities where |∇∇ψ| concentrates. Our main result is a compactness theorem: if {Eε(ψε)}ε↓0 is uniformly bounded, then {∇ψε}ε↓0 is compact in L2. Thus, in the limit ε → 0, ψ solves the eikonal equation |∇ψ| = 1 almost everywhere. Our analysis uses ‘entropy relations’ and the ‘div-curl lemma,’ adopting Tartar's approach to the interaction of linear differential equations and nonlinear algebraic relations.
in the plane. As ε → 0, this functional favours |∇ψ| = 1 and penalizes singularities where |∇∇ψ| concentrates. Our main result is a compactness theorem: if {Eε(ψε)}ε↓0 is uniformly bounded, then {∇ψε}ε↓0 is compact in L2. Thus, in the limit ε → 0, ψ solves the eikonal equation |∇ψ| = 1 almost everywhere. Our analysis uses ‘entropy relations’ and the ‘div-curl lemma,’ adopting Tartar's approach to the interaction of linear differential equations and nonlinear algebraic relations.