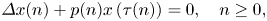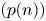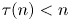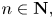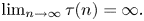Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-02-06T16:59:13.523Z Has data issue: false hasContentIssue false
  • English
  • Français

Upper and lower limit oscillation conditions for first-order difference equations

Part of: Difference and functional equations Difference equations

Published online by Cambridge University Press:  07 July 2022

Özkan Öcalan Open the ORCID record for Özkan Öcalan [Opens in a new window]
Özkan Öcalan*
Affiliation:
Department of Mathematics, Faculty of Science, Akdeniz University, Antalya 07058, Turkey (ozkanocalan@akdeniz.edu.tr)
Rights & Permissions [Opens in a new window]

Abstract

In this work, we consider the first-order difference equation with general argument

\[ \varDelta x(n)+p(n)x\left( \tau (n)\right) =0,\quad n\geq 0, \]
where $(p(n))$ is a sequence of non-negative real numbers, $(\tau (n))$ is a sequence of integers such that $\tau (n)< n$ for$\ n\in \mathbf {N},\,$and$\ \lim _{n\rightarrow \infty }\tau (n)=\infty.$ Under the assumption that the deviating argument is not necessarily monotone, we obtain some new oscillation conditions and improve the all known results for the above equation in the literature, involving only upper and only lower limit conditions. Two examples illustrating the results are also given.

Keywords

delay difference equationgeneral argumentoscillation

MSC classification

Primary: 39A10: Difference equations, additive 39A21: Oscillation theory
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 65 , Issue 3 , August 2022 , pp. 618 - 631
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society

1. Introduction

For a long time, the oscillation theory of differential and difference equations has attracted many researchers. In recent years, there has been much research activity concerning the oscillation and non-oscillation of solutions of delay differential and difference equations. For these oscillatory and non-oscillatory results, we refer, for instance [Reference Braverman and Karpuz1–Reference Zhang and Tian19].

Consider the retarded difference equation

(1.1)\begin{equation} \varDelta x(n)+p(n)x( \tau (n)) =0,\quad n=0,1,\ldots ,\end{equation}

where $(p(n))$ is a sequence of non-negative real numbers, $(\tau (n))$ is a sequence of integers such that

(1.2)\begin{equation} \tau (n)< n\text{ for } n\geq 0,\text{ and }\lim_{n\rightarrow \infty }\tau (n)=\infty . \end{equation}

$\varDelta$ denotes the forward difference operator $\varDelta x(n)=x(n+1)-x(n).$ Define

\[ k={-}\min_{n\geq 0}\tau (n)\quad\text{(clearly, }k\text{ is a positive integer).} \]

By a solution of the difference equation (1.1), we mean a sequence of real numbers $(x(n))$ which satisfies (1.1) for all $n\geq 0$. It is clear that, for each choice of real numbers $c_{-k},\,c_{-k+1},\ldots,\,c_{-1},\,c_{0},$ there exists a unique solution $(x(n))$ of (1.1) which satisfies the initial conditions $x(-k)=c_{-k},\,\ x(-k+1)=c_{-k+1},\,\ldots,\,\ x(-1)=c_{-1},\,\ x(0)=c_{0}.$

A solution $(x(n))$ of the difference equation (1.1) is called oscillatory, if the terms $x(n)$ of the sequence are neither eventually positive nor eventually negative. Otherwise, the solution is said to be non-oscillatory.

When $\tau (n)=n-l$ where $l\geq 0$ an integer number$,$ then Equation (1.1) reduces to

(1.3)\begin{equation} \varDelta x(n)+p(n)x( n-l) =0. \end{equation}

Strong interest in the delay difference equation (1.1) is motivated by the fact that it represents a discrete analogue of the delay differential equation

(1.4)\begin{equation} x^{\prime }(t)+p(t)x( \tau (t)) =0,\quad t\geq t_{0}, \end{equation}

where $p(t)\in C( [t_{0},\,\infty ),\,[0,\,\infty )) ,\,\ \tau (t)\in C( [t_{0},\,\infty ),\,\mathbb {R}) ,\,\ \tau (t)\leq t$ and$\ \lim _{t\rightarrow \infty }\tau (t)=\infty.$

In particular, the delay difference equation (1.3) represents a discrete analogue of the (first-order) delay differential equation

(1.5)\begin{equation} x^{\prime }(t)+p(t)x( t-T) =0,\quad t\geq t_{0}, \end{equation}

where $T$ is a positive real constant. For Equations (1.4) and (1.5), see [Reference Erbe, Kong and Zhang8, Reference Györi and Ladas10, Reference Ladde, Lakshmikantham and Zhang13].

The problem of establishing sufficient conditions for the oscillation of all solutions of the difference equations (1.1) and (1.3) has been the subject of many investigations, for instance, in 1989, Erbe and Zhang [Reference Erbe and Zhang7] proved that each one of the conditions

(1.6)\begin{equation} \liminf_{n\rightarrow \infty }p(n)>\displaystyle\frac{l^{l}}{(l+1)^{l+1}} \end{equation}

or

(1.7)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=n-l}^{n}p(j)>1\end{equation}

is sufficient for all solutions of (1.3) to be oscillatory. In the same year, 1989, Ladas, Philos and Sficas [Reference Ladas, Philos and Sficas12] established that all solutions of (1.3) are oscillatory if

(1.8)\begin{equation} \liminf_{n\rightarrow \infty }\sum\limits_{j=n-l}^{n-1}p(j)>\left(\displaystyle\frac{l}{ l+1}\right)^{l+1}. \end{equation}

Clearly, the condition (1.8) improves the condition (1.6).

We now turn to the general case of the delay difference equation (1.1). The condition (1.7) can be extended to Equation (1.1). More precisely, if the sequence $( \tau (n))$ is assumed to be increasing, then from Chatzarakis et al. [Reference Chatzarakis, Koplatadze and Stavroulakis3], it follows that all solutions of (1.1) are oscillatory if

(1.9)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n}p(j)>1.\end{equation}

In 1991, Philos [Reference Philos14] extended the oscillation criterion (1.8) to the general case of Equation (1.1), by establishing that, if the sequence $( \tau (n))$ is non-decreasing, then the condition

(1.10)\begin{equation} \liminf_{n\rightarrow \infty }[ \displaystyle\frac{1}{n-\tau (n)}\sum\limits_{j= \tau (n)}^{n-1}p(j)] >\limsup\limits_{n\rightarrow \infty }\frac{ (n-\tau (n))^{n-\tau (n)}}{( n-\tau (n)+1) ^{n-\tau (n)+1}}\end{equation}

suffices for the oscillation of all solutions of Equation (1.1).

In 1998, Zhang and Tian [Reference Zhang and Tian19] obtained that if$\ (\tau (n))$ is non-decreasing,

(1.11)\begin{equation} \lim_{n\rightarrow \infty }( n-\tau (n)) =\infty , \end{equation}

and

(1.12)\begin{equation} \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)>\displaystyle\frac{1}{e} , \end{equation}

then all solutions of (1.1) are oscillatory.

In 1998, Zhang and Tian [Reference Zhang and Tian18] obtained that if$\ (\tau (n))$ is not necessarily monotone and

(1.13)\begin{equation} \limsup\limits_{n\rightarrow \infty }p(n)>0\text{ and } \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)>\displaystyle\frac{1}{e} , \end{equation}

then all solutions of (1.1) oscillate.

In 2008, Chatzarakis et al. [Reference Chatzarakis, Koplatadze and Stavroulakis2, Reference Chatzarakis, Koplatadze and Stavroulakis3], when $(\tau (n))$ is not necessarily monotone, studied Equation (1.1) and proved that, if one of the following conditions

(1.14)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)>1,\quad\text{where }h(n)=\max_{0\leq s\leq n}\tau (s), n\geq 0,\end{equation}

or

(1.15)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)<\infty \text{ and }\liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)>\displaystyle\frac{1}{e}, \end{equation}

is satisfied, then all solutions of (1.1) oscillate.

Set,

(1.16)\begin{equation} k(n):=\left(\displaystyle\frac{n-\tau (n)+1}{n-\tau (n)}\right)^{n-\tau (n)+1},\quad n\geq 0. \end{equation}

Clearly,

\[ e< k(n)\leq 4,\quad n\geq 0. \]

In 2006, W. Yan, Q. Meng and J. Yan [Reference Yan, Meng and Yan17] obtained that if$\ (\tau (n))$ is non-decreasing and

(1.17)\begin{equation} \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)\left(\displaystyle\frac{j-\tau (j)+1}{j-\tau (j)}\right) ^{j-\tau (j)+1}>1, \end{equation}

then all solutions of (1.1) are oscillatory.

Observe that, it is easy to see that

\[ \sum\limits_{j=\tau (n)}^{n-1}p(j)k(j)>e\sum\limits_{j=\tau (n)}^{n-1}p(j) \]

and therefore the condition (1.17) is better than the condition (1.12).

In 2016, Öcalan [Reference Öcalan15], when $(\tau (n))$ is not necessarily monotone, established the following result; if

(1.18)\begin{align} & \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{ j-\tau (j)+1}{j-\tau (j)}\right) ^{j-\tau (j)+1} \nonumber\\ & \quad=\liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)\left( \displaystyle\frac{j-\tau (j)+1}{j-\tau (j)}\right) ^{j-\tau (j)+1}>1, \end{align}

where $h(n)=\max _{0\leq s\leq n}\tau (s)$, $n\geq 0$, then all solutions of (1.1) are oscillatory.

In 2011, Braverman and Karpuz [Reference Braverman and Karpuz1] proved that if $(\tau (n))$ is not necessarily monotone and

(1.19)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)\prod\limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{ 1-p(i)}>1, \end{equation}

then all solutions of (1.1) oscillate. Evidently, condition (1.19) has improved condition (1.14).

In [Reference Öcalan16], Öcalan proved that if $(\tau (n))$ is not necessarily monotone and

(1.20)\begin{equation} \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1}{ 1-p(i)}>\frac{1}{e}, \end{equation}

then all solutions of (1.1) oscillate. It can be seen immediately that if $(\tau (n))$ is non-decreasing, then condition (1.20) returns to condition (1.15). However, if $(\tau (n))$ is strictly non-monotone, then condition (1.20) has improved condition (1.15).

The main aim of this paper is to improve, involving only upper and only lower limit conditions, the all known results for Equation (1.1) in the literature.

Throughout this paper, we are going to use the following notation:

\[ \prod\limits_{i=k}^{k-1}A(i)=1\quad\text{and }\sum \limits_{i=k}^{k-1}A(i)=0. \]

2. Main results

We present some new sufficient conditions for the oscillation of all solutions of Equation (1.1), under the assumption that the arguments $(\tau (n))$ is not necessarily monotone.

Let,

(2.1)\begin{equation} h(n)=\max_{s\leq n}\tau (s),\quad n\geq 0. \end{equation}

Clearly, $h(n)$ is non-decreasing and $\tau (n)\leq h(n)$ for all $n\geq 0.$

The following Lemma was given in [Reference Chatzarakis, Koplatadze and Stavroulakis3], which is needed to prove our next theorem.

Lemma 2.1 Assume that (1.2) holds and $p(n)\geq 0$. Thus, we have

\[ \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)=\liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j), \]

where $(h(n))$ is defined by (2.1).

Theorem 2.2 Assume that (1.2) holds and $p(n)\geq 0$. Furthermore, assume that

(2.2)\begin{equation} \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)\left( \displaystyle\frac{j-h(j)+1}{j-h(j)}\right) ^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1} \frac{1}{1-p(i)}>1, \end{equation}

where $(h(n))$ is defined by (2.1). If $\lim _{n\rightarrow \infty }(n-h(n))=\infty$ or $h(n)=n-m,\,\ m\geq 1\in \mathbb {N},$ then all solutions of Equation (1.1) oscillate.

Proof. Assume, for the sake of contradiction, that $(x(n))$ be an eventually positive solution of Equation (1.1). Let $n_{1}\geq -k$ be an integer such that $x(n),\,x(\tau (n))>0$ for all $n\geq n_{1}.$ Thus, from Equation (1.1), we have

\[ \varDelta x(n)={-}p(n)x( \tau (n)) \leq 0,\quad n\geq n_{1}, \]

which means that the sequence $(x(n))$ is eventually non-increasing. In view of this and taking into account that $\tau (n)< n$, Equation (1.1) gives

\[ \varDelta x(n)+p(n)x( n) \leq 0,\quad n\geq n_{1}. \]

If we apply the discrete Grönwall inequality to this inequality, we obtain

\[ x(m)\geq x(n)\prod\limits_{i=m}^{n-1}\displaystyle\frac{1}{1-p(i)},\quad n_{1}\leq m\leq n. \]

On the other hand, we know from Lemma 2.1 that

\begin{align*} & \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)\left( \displaystyle\frac{j-h(j)+1}{j-h(j)}\right) ^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1} \frac{1}{1-p(i)} \\ & =\liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{ j-h(j)+1}{j-h(j)}\right)^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{ 1}{1-p(i)}. \end{align*}

Now, we define

(2.3)\begin{equation} s(n):=\left(\displaystyle\frac{n-h(n)+1}{n-h(n)}\right)^{n-h(n)+1},\quad n\geq 0.\end{equation}

Thus, by (2.2), it follows that there exists a constant $d$ such that

(2.4)\begin{align} \sum\limits_{j=h(n)}^{n}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1}{ 1-p(i)} & \geq \sum\limits_{j=h(n)}^{n}p(j)s(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{1}{1-p(i)} \nonumber\\ & \geq\sum\limits_{j=h(n)}^{n-1}p(j)s(j)\prod\limits_{i=\tau (j)}^{h(j)-1} \displaystyle\frac{1}{1-p(i)}\geq d>1. \end{align}

Now, in view of (2.4), and for all large $n,$ there exists $n^{\ast }\in \lbrack h(n),\,n)$ such that

(2.5)\begin{equation} 4\sum\limits_{j=h(n)}^{n^{{\ast} }}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1} \displaystyle\frac{1}{1-p(i)}\geq \frac{d}{2}\text{ and } 4\sum\limits_{j=n^{{\ast} }}^{n}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{1}{1-p(i)}\geq \frac{d}{2} . \end{equation}

From the fact that $(h(n))$ is non-decreasing and $(x(n))$ is non-increasing, summing up (1.1) from $h(n)$ to $n^{\ast }$and applying the discrete Grönwall inequality, we obtain

\[ x(n^{{\ast} }+1)-x(h(n))+\sum\limits_{j=h(n)}^{n^{{\ast} }}p(j)x(h(j))\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1}{1-p(i)}\leq 0 \]

and

(2.6)\begin{equation} x(n^{{\ast} }+1)-x(h(n))+x(h(n^{{\ast} }))\sum\limits_{j=h(n)}^{n^{{\ast} }}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1}{1-p(i)}\leq 0 \end{equation}

Also, summing up Equation (1.1) from $n^{\ast }$ to $n,$ and using the discrete Grönwall inequality, will yield

(2.7)\begin{equation} x(n+1)-x(n^{{\ast} })+x(h(n))\sum\limits_{j=n^{{\ast} }}^{n}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1}{1-p(i)}\leq 0.\end{equation}

By omitting the first terms in (2.6) and (2.7) and by using (2.5), we obtain

\[{-}x(h(n))+x(h(n^{{\ast} }))\displaystyle\frac{d}{8}\leq 0 \]

and

\[{-}x(n^{{\ast} })+x(h(n))\displaystyle\frac{d}{8}\leq 0. \]

Thus, we have

\[ x(n^{{\ast} })\geq x(h(n))\displaystyle\frac{d}{8}\geq x(h(n^{{\ast} }))\bigg(\frac{d}{8}\bigg) ^{2}, \]

and so

(2.8)\begin{equation} \displaystyle\frac{x(h(n^{{\ast} }))}{x(n^{{\ast} })}\leq \bigg(\frac{8}{d}\bigg)^{2}.\end{equation}

On the other hand, by (1.1), we obtain

(2.9)\begin{equation} \displaystyle\frac{x(n+1)}{x(n)}-1+p(n)\frac{x( \tau (n)) }{x(n)}=0. \end{equation}

Applying the discrete Grönwall inequality to (2.9), we obtain

(2.10)\begin{equation} \displaystyle\frac{x(n+1)}{x(n)}-1+p(n)\frac{x( h(n)) }{x(n)}\prod\limits_{i=\tau (n)}^{h(n)-1}\frac{1}{1-p(i)}\leq 0. \end{equation}

Set

(2.11)\begin{equation} y(n)=1-\displaystyle\frac{x(n+1)}{x(n)}, \end{equation}

then (2.11) yields,

(2.12)\begin{equation} y(n)\geq p(n)\prod\limits_{i=\tau (n)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)} \prod\limits_{j=h(n)}^{n-1}[ 1-y(j)] ^{{-}1}. \end{equation}

Now, using the well-known inequality between the arithmetic and geometric means, we find that

(2.13)\begin{equation} y(n)\geq p(n)\prod\limits_{i=\tau (n)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)}\left[1- \frac{1}{n-h(n)}\sum\limits_{j=h(n)}^{n-1}y(j)\right]^{-( n-h(n)) }. \end{equation}

So, using the inequality

\[ \alpha (1-\alpha )^{k}\leq \displaystyle\frac{k^{k}}{(k+1)^{k+1}},\quad \alpha \in (0,1),\ k\in \mathbf{N} \]

inequality (2.13) gives

(2.14)\begin{equation} y(n)\geq p(n)\prod\limits_{i=\tau (n)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)}\left(\frac{ n-h(n)+1}{n-h(n)}\right) ^{n-h(n)+1}\sum\limits_{j=h(n)}^{n-1}y(j).\end{equation}

Let $\liminf _{n\rightarrow \infty }\sum \nolimits \limits _{j=h(n)}^{n-1}y(j)=c.$ We know that

\[ \displaystyle\frac{x( h(n)) }{x(n)}=\prod\limits_{j=h(n)}^{n-1}[1-y(j)] ^{{-}1}\geq \left[1-\frac{1}{n-h(n)}\sum\limits_{j=h(n)}^{n-1}y(j)\right] ^{-( n-h(n)) } \]

and

(2.15)\begin{equation} \displaystyle\frac{x( h(n)) }{x(n)}\geq \left(\frac{n-h(n)+1}{n-h(n)}\right) ^{n-h(n)+1}\sum\limits_{j=h(n)}^{n-1}y(j)\geq\sum\limits_{j=h(n)}^{n-1}y(j). \end{equation}

From (2.10) and (2.15), we have

(2.16)\begin{equation} \infty >\liminf_{n\rightarrow \infty }\displaystyle\frac{x( h(n)) }{x(n)}\geq \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}y(j)=c. \end{equation}

Now, from (2.16) we get

(2.17)\begin{equation} \sum\limits_{j=h(n)}^{n-1}y(j)\geq c-\varepsilon ,\quad\text{for }n\geq n_{2}, \end{equation}

where $\varepsilon$ is an arbitrary real number with $0<\varepsilon < c.$ So, from (2.14) and (2.17), we have

(2.18)\begin{equation} y(n)\geq p(n)\left(\displaystyle\frac{n-h(n)+1}{n-h(n)}\right)^{n-h(n)+1}(c-\varepsilon )\prod\limits_{i=\tau (n)}^{h(n)-1}\frac{1}{1-p(i)}. \end{equation}

Summing up (2.18) from $h(n)$ to $n-1,$ we have

(2.19)\begin{equation} \sum\limits_{j=h(n)}^{n-1}y(j)\geq (c-\varepsilon )\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{j-h(j)+1}{j-h(j)}\right)^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{1}{1-p(i)}. \end{equation}

Thus, by (2.19), we obtain

\[ c=\liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}y(j)\geq (c-\varepsilon )\liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{j-h(j)+1}{j-h(j)}\right)^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{1}{1-p(i)} \]

and as $\varepsilon \rightarrow 0,$ the above inequality yields

\[ \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{ j-h(j)+1}{j-h(j)}\right)^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{ 1}{1-p(i)}\leq 1, \]

which contradicts to (2.2). The proof of the theorem is complete.

Remark 2.1 It can be seen immediately that if $\tau (n)< n,\,\ \lim _{n\rightarrow \infty }(n-h(n))=\infty$ or $h(n)=n-m,\,\ m\geq 1\in \mathbb {N}$, then

\[ \sum\limits_{j=h(n)}^{n-1}p(j)s(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1 }{1-p(i)}>e\sum\limits_{j=h(n)}^{n-1}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1} \frac{1}{1-p(i)}, \]

and therefore condition (2.2) is better than condition (1.20).

Moreover, when $(\tau (n))$ is strictly non-monotone and $\prod _{i=\tau (j)}^{h(j)-1}\frac {1}{1-p(i)}=1,$ since

\[ \left(\displaystyle\frac{n-h(n)+1}{n-h(n)}\right)^{n-h(n)+1}\geq \left(\frac{n-\tau (n)+1}{n-\tau (n)}\right) ^{n-\tau (n)+1}, \]

condition (2.2) is better than condition (1.18).

Theorem 2.3 Assume that (1.2) holds. Moreover, we suppose that

(2.20)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)\left( \displaystyle\frac{j-h(j)+1}{j-h(j)}\right) ^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(n)-1} \frac{1}{1-p(i)}>e, \end{equation}

where $(h(n))$ is defined by (2.1). If $\lim _{n\rightarrow \infty }(n-h(n))=\infty$ or $h(n)=n-m,\,\ m\geq 1\in \mathbb {N},$ then all solutions of Equation (1.1) oscillate.

Proof. Assume, for the sake of contradiction, that $(x(n))$ be an eventually positive solution of Equation (1.1). Let $n_{1}\geq -k$ be an integer such that $x(n),\,x(\tau (n))>0$ for all $n\geq n_{1}.$ Thus, by equation (1.1), we have

\[ \varDelta x(n)={-}p(n)x( \tau (n)) \leq 0,\quad n\geq n_{1}, \]

which means that the sequence $(x(n))$ is non-increasing. It is clear that if $\tau (n)< n$ and $\lim _{n\rightarrow \infty }(n-h(n))=\infty,\,~$or $h(n)=n-$ $m$ with $m\geq 1\in \mathbb {N}\mathbf {,\,}$ then

\[ e< s(n)\leq 4,\quad n\geq 0. \]

From Equation (1.1), we have

(2.21)\begin{equation} s(n)\varDelta x(n)+p(n)s(n)x( \tau (n)) =0,\quad n\geq n_{1}. \end{equation}

Now, we assume that $\tau (n)< n$ and $\lim _{n\rightarrow \infty }(n-h(n))=\infty.$ Summing up (2.21) from $h(n)$ to $n,$ we obtain

(2.22)\begin{equation} \sum\limits_{j=h(n)}^{n}s(j)\varDelta x(j)+\sum\limits_{j=h(n)}^{n}p(j)s(j)x(\tau (j)=0. \end{equation}

Applying the discrete Grönwall inequality to (2.22), we obtain

\[ \sum\limits_{j=h(n)}^{n}s(j)\varDelta x(j)+\sum\limits_{j=h(n)}^{n}p(j)s(j)x(h(n))\prod\limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)}\leq 0, \]

and from the fact that $(h(n))$ is non-decreasing and $(x(n))$ is non-increasing, we have

(2.23)\begin{equation} s(h(n))x(n+1)-s(h(n))x(h(n))+x(h(n))\sum\limits_{j=h(n)}^{n}p(j)s(j)\prod \limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)}\leq 0, \end{equation}

or

(2.24)\begin{equation} -s(h(n))x(h(n))+x(h(n))\sum\limits_{j=h(n)}^{n}p(j)s(j)\prod\limits_{i= \tau (j)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)}\leq 0. \end{equation}

So, from (2.24), we obtain

(2.25)\begin{equation} x(h(n))\left[\sum\limits_{j=h(n)}^{n}p(j)s(j)\prod\limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{1-p(i)}-s(h(n))\right] \leq 0, \end{equation}

and

(2.26)\begin{equation} \sum\limits_{j=h(n)}^{n}p(j)s(j)\prod\limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{ 1-p(i)}\leq s(h(n). \end{equation}

Since $\lim _{n\rightarrow \infty }(n-h(n))=\infty,$ we have $\lim _{n\rightarrow \infty }s(h(n))=e.$ So, by (2.26), we obtain

\[ \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)\left( \displaystyle\frac{j-h(j)+1}{j-h(j)}\right) ^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(n)-1} \frac{1}{1-p(i)}\leq e, \]

which contradicts to (2.20).

Now, we assume that $h(n)=n-m,\,\ m\geq 1\in \mathbb {N}.$ So, condition (2.20) is equivalent to

(2.27)\begin{equation} \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=n-m}^{n}p(j)\prod\limits_{i=j-m}^{n-m-1}\displaystyle\frac{1}{1-p(i)} >e\left(\frac{m}{m+1}\right)^{m+1}. \end{equation}

In view of (2.23), we have

(2.28)\begin{equation} s(h(n))x(n+1)-s(h(n))x(h(n))+x(h(n))\sum\limits_{j=n-m}^{n}p(j)s(j)\prod \limits_{i=j-m}^{n-m-1}\displaystyle\frac{1}{1-p(i)}\leq 0. \end{equation}

On the other hand, since $\lim _{n\rightarrow \infty }x(n)=l\geq 0,$ we can find a constant $c>0$ such that

(2.29)\begin{equation} c>[ s(h(n))-e] \text{ and }s(h(n))x(n+1)>cx(h(n)). \end{equation}

Thus, from (2.28) and (2.29), we obtain

\[ cx(h(n))-s(h(n))x(h(n))+x(h(n))\left(\displaystyle\frac{m+1}{m}\right)^{m+1}\sum\limits_{j=n-m}^{n}p(j)\prod\limits_{i=j-m}^{n-m-1}\frac{1}{ 1-p(i)}\leq 0, \]

or

\[ x(h(n))[ c-s(h(n))] +x(h(n))\left(\displaystyle\frac{m+1}{m}\right)^{m+1}\sum\limits_{j=n-m}^{n}p(j)\prod\limits_{i=j-m}^{n-m-1}\frac{1}{ 1-p(i)}\leq 0, \]

or

\[{-}ex(h(n))+x(h(n))\left(\displaystyle\frac{m+1}{m}\right)^{m+1}\sum\limits_{j=n-m}^{n}p(j)\prod\limits_{i=j-m}^{n-m-1}\frac{1}{ 1-p(i)}\leq 0, \]

and

(2.30)\begin{equation} x(h(n))\left[\left(\displaystyle\frac{m+1}{m}\right) ^{m+1}\sum\limits_{j=n-m}^{n}p(j)\prod\limits_{i=j-m}^{n-m-1}\frac{1}{ 1-p(i)}-e\right] \leq 0. \end{equation}

So, by (2.30), we obtain

\[ \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=n-m}^{n}p(j)\prod\limits_{i=j-m}^{n-m-1}\displaystyle\frac{1}{1-p(i)} \leq e\left(\frac{m}{m+1}\right) ^{m+1}, \]

which contradicts to (2.20). The proof of theorem is complete.

Remark 2.2 Observe that, it is easy to see that if $\tau (n)< n,\,\ \lim _{n\rightarrow \infty }(n-h(n))=\infty$ or $h(n)=n-m,\,\ m\geq 1\in \mathbb {N}$, then

\[ \sum\limits_{j=h(n)}^{n}p(j)s(j)\prod\limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{ 1-p(i)}>e\sum\limits_{j=h(n)}^{n}p(j)\prod\limits_{i=\tau (j)}^{h(n)-1} \frac{1}{1-p(i)}, \]

and therefore condition (2.20) is better than condition (1.19).

Now, we present two examples to show the significance of our results. The first example is for comparing $\lim \sup$ conditions.

Example 2.1 Consider the following difference equation

(2.31)\begin{equation} \varDelta x(n)+(0.22)x(n-2)=0,\quad n=0,1,\ldots \end{equation}

Let us first show that the $\lim \sup$ tests mentioned in the introduction fail for this equation. Clearly,

\[ \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)=\limsup\limits_{n\rightarrow \infty }\sum\limits_{j=n-2}^{n}p(j)=0.66\ngtr 1, \]

which means that the condition (1.14) is not applicable for this equation. Moreover,

\begin{align*} & \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)\prod\limits_{i=\tau (j)}^{h(n)-1}\displaystyle\frac{1}{ 1-p(i)}=\limsup\limits_{n\rightarrow \infty }\sum\limits_{j=n-2}^{n}p(j)\prod\limits_{i=j-2}^{n-3}\frac{1}{1-p(i)}\\ & =\limsup\limits_{n\rightarrow \infty }\left[p(n-2)\prod\limits_{i=n-4}^{n-3}\displaystyle\frac{1}{1-p(i)}+p(n-1)\prod\limits_{i=n-3}^{n-3}\frac{ 1}{1-p(i)}+p(n)\prod\limits_{i=n-2}^{n-3}\frac{1}{1-p(i)}\right] \\ & =\limsup\limits_{n\rightarrow \infty }\left[p(n-2)\left(\displaystyle\frac{1}{1-p(n-4)}\right)\left(\frac{1}{1-p(n-3)}\right) \!+\!p(n-1)\left(\frac{1}{1-p(n-3)}\right) \!+\!p(n)\right] \\ & =0.863\,66\ngtr 1, \end{align*}

which means that the condition (1.19) is not applicable for this equation. However,

\begin{align*} & \limsup\limits_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n}p(j)\left( \displaystyle\frac{j-h(j)+1}{j-h(j)}\right) ^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(n)-1}\frac{1}{1-p(i)} \\ & \quad=0.863\,66\left(\frac{3}{2}\right)^{3} \\ & \quad= 2. 914\,9>e\cong 2.71. \end{align*}

That is, condition (2.20) of Theorem 2.3 is satisfied. Therefore, all solutions of (2.31) oscillate.

The second example is for comparing $\lim \inf$ conditions.

Example 2.2 Consider the following difference equation

(2.32)\begin{equation} \varDelta x(n)+(0.3)x(\tau (n))=0,\quad n\geq 0, \end{equation}

with

\[ \tau (n)=\left\{ \begin{array}{@{}cc} n-3, & \text{if }n\text{ is even,} \\ n-1 & \text{if }n\text{ is odd.} \end{array} \right. \]

Here, it is clear that (1.2) is satisfied and $(\tau (n))$ is strictly non-monotone. Also, by (2.1), we have

\[ h(n)=\max_{s\leq n}\tau (s)=\left\{ \begin{array}{@{}cc} n-2, & \text{if }n\text{ is even,} \\ n-1 & \text{if }n\text{ is odd.} \end{array} \right. \]

Let us first show that the $\lim \inf$ tests mentioned in the introduction fail for this equation. Clearly,

\[ \liminf_{n\rightarrow \infty }\sum\limits_{j=\tau (n)}^{n-1}p(j)=\liminf_{n\rightarrow \infty }\sum\limits_{j=n-1}^{n-1}(0.3)=0.3\ngtr \displaystyle\frac{1}{e}, \]

which means that condition (1.15) is not applicable for this equation. Moreover,

\begin{align*} \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\prod\limits_{i=\tau (j)}^{h(j)-1}\displaystyle\frac{1}{ 1-p(i)} & =\liminf_{n\rightarrow \infty }\sum\limits_{j=n-1}^{n-1}p(j)\prod\limits_{i=j-1}^{j-2}\frac{1}{1-p(i)} \\ & =0.3\ngtr \frac{1}{e}, \end{align*}

which means that condition (1.20) is not applicable for this equation. Also,

\begin{align*} \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{ j-\tau (j)+1}{j-\tau (j)}\right) ^{j-\tau (j)+1} & =\liminf_{n\rightarrow \infty }\sum\limits_{j=n-1}^{n-1}p(j)\left(\frac{j-\tau (j)+1}{j-\tau (j)}\right)^{j-\tau (j)+1} \\ & =(0.3)\times \left(\frac{4}{3}\right) ^{4}\cong 0.94\ngtr 1, \end{align*}

which means that condition (1.18) is not applicable for this equation. However,

\begin{align*} & \liminf_{n\rightarrow \infty }\sum\limits_{j=h(n)}^{n-1}p(j)\left(\displaystyle\frac{ j-h(j)+1}{j-h(j)}\right)^{j-h(j)+1}\prod\limits_{i=\tau (j)}^{h(j)-1}\frac{ 1}{1-p(i)} \\ & \quad=\liminf_{n\rightarrow \infty }\sum\limits_{j=n-1}^{n-1}p(j)\left(\displaystyle\frac{ j-h(j)+1}{j-h(j)}\right)^{j-h(j)+1} \\ & =(0.3)\times \left(\frac{3}{2}\right)^{3}\cong 1.01>1. \end{align*}

That is, condition (2.2) of Theorem 2.2 is satisfied. Therefore, all solutions of (2.32) oscillate.

References

Braverman, E. and Karpuz, B., On oscillation of differential and difference equations with non-monotone delays, Appl. Math. Comput. 218 (2011), 3880–3887.Google Scholar
Chatzarakis, G. E., Koplatadze, R. and Stavroulakis, I. P., Optimal oscillation criteria for first order difference equations with delay argument, Pacific J. Math. 235 (2008), 15–33.CrossRefGoogle Scholar
Chatzarakis, G. E., Koplatadze, R. and Stavroulakis, I. P., Oscillation criteria of first order linear difference equations with delay argument, Nonlinear Anal. 68 (2008), 994–1005.CrossRefGoogle Scholar
Chatzarakis, G. E., Philos, Ch. G. and Stavroulakis, I. P., On the oscillation of the solutions to linear difference equations with variable delay, Electron. J. Differ. Equ. 50 (2008), 1–5.Google Scholar
Chatzarakis, G. E., Philos, Ch. G. and Stavroulakis, I. P., An oscillation criterion for linear difference equations with general delay argument, Portugal. Math. (N.S.) 66 (4) (2009), 513–533.CrossRefGoogle Scholar
Chen, M. P. and Yu, J. S., Oscillations of delay difference equations with variable coefficients, in Proceedings of the First International Conference on Difference Equations, pp. 105–114 (Gordon and Breach, London, 1994).Google Scholar
Erbe, L. H. and Zhang, B. G., Oscillation of discrete analogues of delay equations, Differ. Int. Equ. 2 (1989), 300–309.Google Scholar
Erbe, L. H., Kong, Q. and Zhang, B. G., Oscillation theory for functional differential equations, (Marcel Dekker, New York, 1995).Google Scholar
Györi, I. and Ladas, G., Linearized oscillations for equations with piecewise constant arguments, Differ. Int. Equ. 2 (1989), 123–131.Google Scholar
Györi, I. and Ladas, G., Oscillation Theory of Delay Differential Equations with Applications (Oxford, Clarendon Press, 1991).Google Scholar
Ladas, G., Explicit conditions for the oscillation of difference equations, J. Math. Anal. Appl 153 (1990), 276–287.CrossRefGoogle Scholar
Ladas, G., Philos, Ch. G. and Sficas, Y. G., Sharp conditions for the oscillation of delay difference equations, J. Appl. Math. Simul. 2 (1989), 101–111.CrossRefGoogle Scholar
Ladde, G. S., Lakshmikantham, V. and Zhang, B. G., Oscillation theory of differential equations with deviating arguments (New York, Marcel Dekker, 1987).Google Scholar
Philos, Ch. G., On oscillations of some difference equations, Funkcial. Ekvac. 34 (1991), 157–172.Google Scholar
Öcalan, Ö., An improved oscillation criterion for first order difference equations, Bull. Math. Soc. Sci. Math. Roumanie (N.S.) 59 (107) (2016), 65–73.Google Scholar
Öcalan, Ö., Oscillation of first-order dynamic equations with nonmonotone delay, Math. Methods Appl. Sci. 43 (2020), 3954–3964.Google Scholar
Yan, W., Meng, Q. and Yan, J., Oscillation criteria for difference equation of variable delays, Dyn. Contin. Discrete Impuls. Syst. Ser. A Math. Anal. 13A (2006), 641–647. Part 2, suppl.Google Scholar
Zhang, B. G. and Tian, C. J., Nonexistence and existence of positive solutions for difference equations with unbounded delay, Comput. Math. Appl. 36 (1998), 1–8.CrossRefGoogle Scholar
Zhang, B. G. and Tian, C. J., Oscillation criteria for difference equations with unbounded delay, Comput. Math. Appl. 35 (4) (1998), 19–26.CrossRefGoogle Scholar