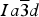Hostname: page-component-745bb68f8f-v2bm5 Total loading time: 0 Render date: 2025-02-10T10:09:47.291Z Has data issue: false hasContentIssue false
  • English
  • Français

The mystery of birefringent garnet: is the symmetry lower than cubic?

Published online by Cambridge University Press:  24 July 2013

Sytle M. Antao
Sytle M. Antao*
Affiliation:
Department of Geoscience, University of Calgary, Calgary, Alberta T2N 1N4, Canada
*
a) Author to whom correspondence should be addressed. Electronic mail: antao@ucalgary.ca
Rights & Permissions [Opens in a new window]

Abstract

The cause of birefringence in several garnet-group minerals with general chemical formula, [8]X3 [6]Y2 [4]Z3 [4]O12, which was observed over 100 years ago, is unknown, although many different reasons were proposed, including symmetry lower than cubic. In this study, electron microprobe analyses (EMPA) were obtained for a Ti-rich andradite, ideally Ca3(Fe2 3+)Si3O12, from Magnet Cove, Arkansas, USA, and the results show that the sample is inhomogeneous with two distinct compositions. The crystal structure was refined by the Rietveld method, cubic space group $Ia\overline 3 d$ , and monochromatic synchrotron high-resolution powder X-ray diffraction (HRPXRD) data, which shows a mixture of three distinct cubic phases that are intergrown together and cause birefringence because of strain arising from small structural mismatch. This mixture of three cubic phases was not observed by any other experimental technique. These results have many implications, including garnet phase transitions from cubic to lower symmetry in the mantle, which has important geophysical consequences.

Keywords

andraditebirefringencethree-phase intergrowthRietveld refinementssynchrotron high-resolution powder X-ray diffraction (HRPXRD)crystal structure
Type
Technical Articles
Information
Powder Diffraction , Volume 28 , Issue 4 , December 2013 , pp. 281 - 288
Copyright
Copyright © International Centre for Diffraction Data 2013 

I. INTRODUCTION

Birefringence in garnet was reported over a century ago (Brauns, Reference Brauns1891), but the origin has remained questionable. Several members of the garnet-group minerals are birefringent when viewed under cross-polarized light in a petrographic microscope, hence optically they are not cubic. Many reasons were given as the cause of the birefringence (Ingerson and Barksdale, Reference Ingerson and Barksdale1943; Chase and Lefever, Reference Chase and Lefever1960; Blanc and Maisonneuve, Reference Blanc and Maisonneuve1973; Lessing and Standish, Reference Lessing and Standish1973; Foord and Mills, Reference Foord and Mills1978; Kitamura and Komatsu, Reference Kitamura and Komatsu1978; Takéuchi et al., Reference Takéuchi, Haga, Umizu and Sato1982; Akizuki, Reference Akizuki1984; Rossman and Aines, Reference Rossman and Aines1986; Allen and Buseck, Reference Allen and Buseck1988; Kingma and Downs, Reference Kingma and Downs1989; Armbruster et al., Reference Armbruster, Geiger and Lager1992; Griffen et al., Reference Griffen, Hatch, Phillips and Kulaksiz1992; Brown and Mason, Reference Brown and Mason1994; Akizuki et al., Reference Akizuki, Takéuchi, Terada and Kudoh1998; Hofmeister et al., Reference Hofmeister, Schaal, Campbell, Berry and Fagan1998; Shtukenberg et al., Reference Shtukenberg, Punin, Frank-Kamenetskaya, Kovalev and Sokolov2001, Reference Shtukenberg, Popov and Punin2005; Wildner and Andrut, Reference Wildner and Andrut2001; Frank-Kamenetskaya et al., Reference Frank-Kamenetskaya, Rozhdestvenskaya, Shtukenberg, Bannova and Skalkina2007), but none of these suggestions provide a unique solution (Allen and Buseck, Reference Allen and Buseck1988). This study shows that a mixture of three cubic phases with slightly different structural and chemical parameters occurs together and gives rise to strain arising from structural mismatch and cause anisotropy in birefringent garnet samples. This is in contrast to ABO3 synthetic garnet samples, such as CaGeO3, CdGeO3 (Prewitt and Sleight, Reference Prewitt and Sleight1969), MnSiO3 (Fujino et al., Reference Fujino, Momoi, Sawamoto and Kumazawa1986), and MgSiO3 (Angel et al., Reference Angel, Finger, Hazen, Kanzaki, Weidner, Liebermann and Veblen1989; Hatch and Ghose, Reference Hatch and Ghose1989; Parise et al., Reference Parise, Wang, Gwanmesia, Zhang, Sinelnikov, Chmielowski, Weidner and Liebermann1996) that undergo a cubic to tetragonal transition, where the tetragonal phase is birefringent. However, the present results cast some doubt on these observations.

Garnet-group minerals have important physical properties because of dense packing of the constituent atoms (high hardness, high density, high refractive index, etc.). Synthetic rare-earth varieties can have any color in the visible spectrum, and some possess good laser properties (Geusic et al., Reference Geusic, Marcos and Van Uitert1964). In solid-state science, garnet-type materials are important because of their ferrimagnetism and antiferromagnetism (Bertaut and Forrat, Reference Bertaut and Forrat1956; Geller and Gilleo, Reference Geller and Gilleo1957). Some garnet varieties spontaneously polarize in electric and magnetic fields, hence they cannot be cubic (Geller, Reference Geller1967). Although the structure of many birefringent garnet samples were refined in non-cubic space groups, this study shows that the structure of a birefringent garnet is cubic and contains an intergrowth of three different cubic phases.

The cubic crystal structure of garnet with general formula, [8]X3 [6]Y2 [4]Z3 [4]O12, consists of alternating ZO4 tetrahedra and YO6 octahedra with X atoms filling cavities to form XO8 dodecahedra. The eight O atoms in the XO8 polyhedra occur at the corners of a distorted cube (Figure 1). The O atom is bonded to two X, one Y, and one Z in a tetrahedral configuration.

Figure 1. (Color online) Projection of the cubic garnet structure down c showing the ZO4 tetrahedra (gray), YO6 octahedra (yellow), and XO8 dodecahedra (blue) that occur as a distorted cubic shape. Dense packing of the polyhedra are obvious from the four unit cells displayed, which shows the prominent edge-sharing and zigzag arrangement of alternating octahedra and dodecahedra.

The flexibility of the garnet structure allows it to accommodate the most abundant divalent (X), trivalent (Y), and tetravalent (Z) cations on Earth, and gives rise to the general formula [8]X3 [6]Y2 [4]Z3 [4]O12, where the eight-coordinated dodecahedral X site, denoted [8], contains Mg, Ca, Mn2+, or Fe2+ cations, the six-coordinated octahedral Y site contains Al, Fe3+, Ti4+, or Zr4+ cations, and the four-coordinated tetrahedral Z site contains Si, Fe3+ or Al cations, or (F, O4H4) groups (Novak and Gibbs, Reference Novak and Gibbs1971; Smyth et al., Reference Smyth, Madel, McCormick, Munoz and Rossman1990; Armbruster et al., Reference Armbruster, Birrer, Libowitzky and Beran1998).

Ti-rich andradite samples are referred to as “melanite”, schorlomite, or morimotoite, depending on the Ti content. The substitution mechanisms and their nomenclature were addressed (Chakhmouradian and McCammon, Reference Chakhmouradian and McCammon2005). Two substitution mechanisms occur at the Z site. One type of Si-deficient Ti-rich andradite can be interpreted as a solid solution between andradite, Ca3(Fe2 3+)Si3O12, and the theoretical end-member morimotoite, Ca3(Ti4+Fe2+)Si3O12, together with minor hydroxy substitution (O4H4) ↔ SiO4 (Lager et al., Reference Lager, Armbruster, Rotella and Rossman1989; Armbruster, Reference Armbruster1995). The second type of substitution on the Z site is (Fe3+, Al) ↔ Si, and charge balance is achieved with Ti4+ on the Y site (Armbruster et al., Reference Armbruster, Birrer, Libowitzky and Beran1998; Locock, Reference Locock2008), and gives rise to the theoretical end-member schorlomite, Ca3Ti2 4+[Fe2 3+Si]O12 (Henmi et al., Reference Henmi, Kusachi and Henmi1995). The exact substitution mechanism is the subject of several controversial debates based mainly on spectroscopic studies (Armbruster et al., Reference Armbruster, Birrer, Libowitzky and Beran1998).

This study examines the chemical composition and crystal structure of a Ti-rich birefringent andradite sample from Magnet Cove using electron microprobe analysis (EMPA) and synchrotron high-resolution powder X-ray diffraction (HRPXRD) data. The latter technique uses a short wavelength [0.41351(2) Å], superior resolution, and high peak-to-background discrimination. Preliminary reports were presented (Antao et al., Reference Antao, Klincker and Round2013a, Reference Antao, Klincker and Round2013b).

II. EXPERIMENTAL

A. Electron microprobe analysis (EMPA) and synchrotron high-resolution powder X-ray diffraction (HRPXRD)

The dark-brown Ti-rich andradite sample (MC9) from Magnet Cove, Hot Spring County, Arkansas, USA appears homogeneous in plane-polarized light and is weakly birefringent under cross-polarized light, where it appears as dark gray instead of black. However, some striking examples of birefringent garnets that contain mottled or tweed-like texture, lamellar, oscillatory, or concentric zoning are available in the recent literature (e.g., Badar et al., Reference Badar, Akizuki and Hussain2010; Antao and Klincker, Reference Antao and Klincker2013; Badar et al., Reference Badar, Niaz, Hussain and Akizuki2013).

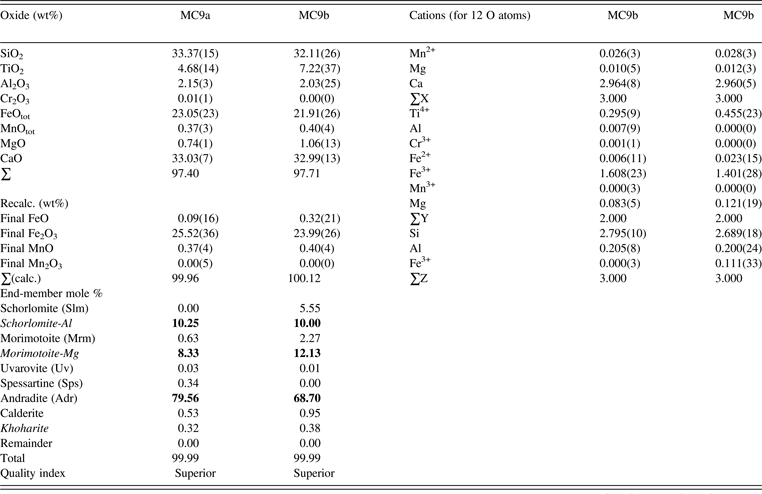
A fragment of the sample (≈0.2 mm in diameter) was analyzed with a JEOL JXA-8200 electron microprobe analyzer (EMPA) using the wavelength-dispersive operating conditions of 15 kV accelerating voltage, 20 nA beam current, a beam diameter of 5 µm, and various mineral standards. The EMPA data were obtained from eight spots from different areas of the crystal and reduced to cations (Table I).

Table I. Electron microprobe analysis a for a Ti-rich andradite (MC9).

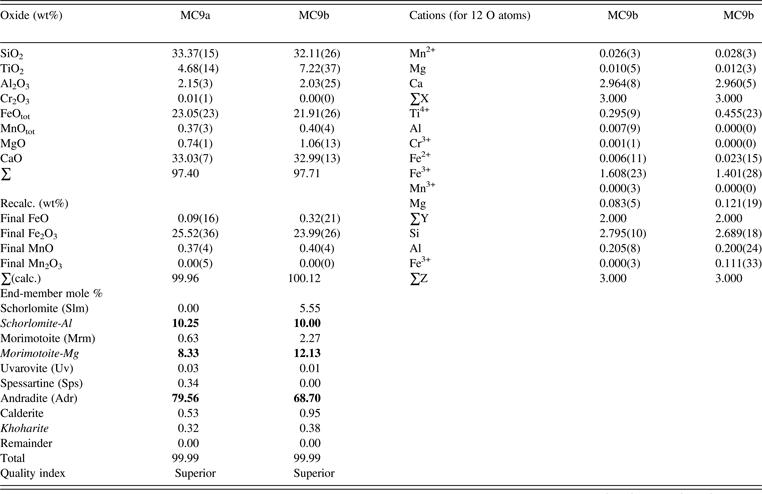
a Electron microprobe data were analyzed using the spreadsheet from Locock (Reference Locock2008), which was also used in calculating the Fe2+/Fe3+ and Mn2+/Mn3+ amounts using the method of Droop (Reference Droop1987). Two phases were detected by EMPA. However, three phases were observed by HRPXRD (MC9a = phase 1, and MC9b = phase 2). Numbers in bold indicate significant end-members.

HRPXRD data were obtained at beamline 11-BM, Advanced Photon Source (APS), Argonne National Laboratory (ANL). A small (≈0.2 mm in diameter) fragment of the sample was crushed to a fine powder using an agate mortar and pestle, and loaded into a Kapton capillary (0.8-mm internal diameter) and rotated at a rate of 90 rotations per second. The data were collected at 23 °C to a maximum 2θ of about 50° with a step size of 0.001° and a step time of 0.1 s per step. The HRPXRD trace was collected with 12 silicon (111) crystal analyzers. A silicon (NIST 640c) and alumina (NIST 676a) standard (ratio of ⅓ Si : ⅔ Al2O3 by weight) was used to calibrate the instrument and refine the monochromatic wavelength (Table II). Additional details of the experimental set-up are given elsewhere (Antao et al., Reference Antao, Hassan, Wang, Lee and Toby2008; Lee et al., Reference Lee, Shu, Ramanathan, Preissner, Wang, Beno, Von Dreele, Ribaud, Kurtz, Antao, Jiao and Toby2008; Wang et al., Reference Wang, Toby, Lee, Ribaud, Antao, Kurtz, Ramanathan, Von Dreele and Beno2008).

Table II. HRPXRD data and Rietveld refinement statistics.

aIn thin film theory (Kitamura and Komatsu, Reference Kitamura and Komatsu1978), both the strain and birefringence between the substrate and film are proportional to Δa (a substrate − a film).

bLY is related to strain and these values are quite large compared to a single phase andradite (MC7), where LY = 3.29 (unpublished result).

c R (F 2) = overall R-structure factor based on observed and calculated structure amplitudes =[∑(F o 2 − F c 2)/∑(F o 2)]1/2.

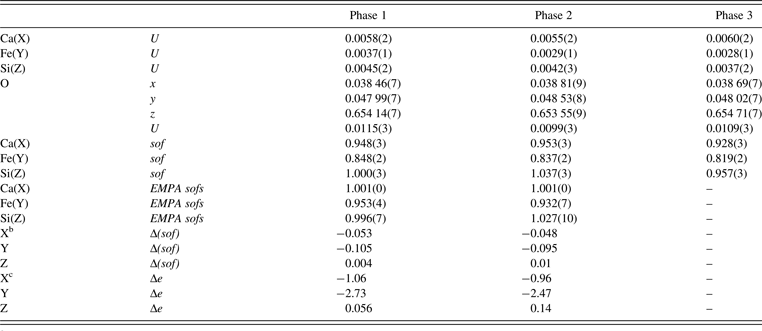
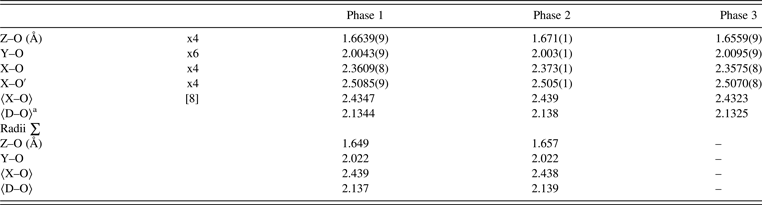
The HRPXRD data were analyzed by the Rietveld method (Rietveld, Reference Rietveld1969) using the GSAS program (Larson and Von Dreele, Reference Larson and Von Dreele2000), EXPGUI interface (Toby, Reference Toby2001), scattering curves for neutral atoms, and a starting structural model from Antao and Klincker (Reference Antao and Klincker2013). A full-matrix least-squares refinement was carried out by varying a scale factor, cell parameter, atom coordinates, and isotropic displacement parameters. The HRPXRD trace shows three separate cubic phases with slightly different unit-cell parameters (Figure 2). The three cubic phases were refined together with the site occupancy factors (sofs) in terms of the dominant atom in the X, Y, and Z sites. At the end of the refinement, all the parameters were varied simultaneously until the refinement converged. The unit-cell parameters and the Rietveld refinement statistics for three cubic phases are listed in Table II. Atom coordinates, isotropic displacement parameters, and sofs are given in Table III. Bond distances are given in Table IV.

Figure 2. HRPXRD trace for a Ti-rich andradite sample (MC9) from Magnet Cove together with the calculated (continuous line) and observed (crosses) profiles. The difference curve (I obs − I calc) is shown at the bottom. The short vertical lines indicate allowed reflection positions. (a) The intensities that are above 20 and 30°2θ are scaled by factors of ×10 and ×30, respectively, for both the trace and difference curves. (b) The fitted HRPXRD trace showing the reflections (840) and (842). Three cubic phases intergrown in the sample are evident, as indicated by the splitting of the diffraction peaks.

Table III. Atom coordinates a , isotropic displacement parameters, U (Å2), and sofs.

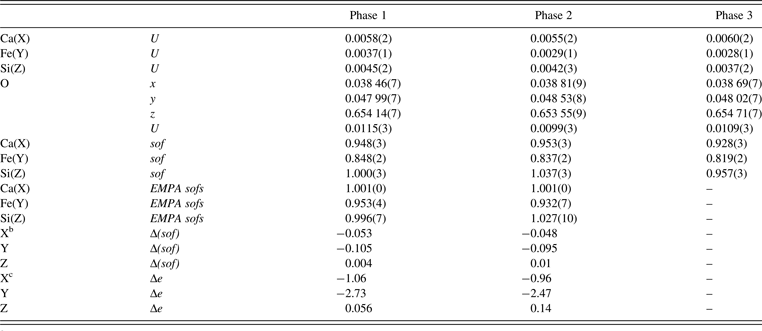
a X at (0, ¼, ⅛) with Ca dominant, Y at (0, 0, 0) with Fe dominant, and Z at (⅜, 0, ¼) with Si dominant.

bΔ(sof) = sof (HRPXRD refinement) − sof (EMPA).

cΔe = electrons (HRPXRD refinement) − electrons (EMPA).

Table IV. Selected distances (Å) in Ti-rich andradite (MC9).

These distances are shown in Figure 3 for comparison with published data. For the calculated radii sum distances, radii from Shannon (Reference Shannon1976) were used (X site: Mn2+ = 0.96, Mg = 0.89 Å; Y site: Ti4+ = 0.605, Al = 0.535, Cr3+ = 0.615, Fe2+ = 0.78, Fe3+ = 0.645, Mn3+ = 0.645, Mg = 0.72 Å; Z site: Si = 0.26, Al = 0.39, Fe3+ = 0.49 Å; O = 1.38 Å). Ca = 1.06 Å instead of 1.12 Å from Shannon (Reference Shannon1976); this gives more realistic 〈X–O〉 distances.

a〈D–O〉 = {(Z–O) + (Y–O) + (X–O) + (X′–O)}/4.

III. RESULTS AND DISCUSSION

The EMPA results show two phases with slightly different compositions of {Ca2.96Mg0.01Mn2+ 0.03}Σ3[Fe3+ 1.61Ti4+ 0.30Mg0.08Al3+ 0.01Fe2+ 0.01]Σ2(Si2.80Al0.21)Σ3O12 (phase-1, 46.5(1) wt% from HRPXRD); and {Ca2.96Mg0.01Mn2+ 0.03}Σ3[Fe3+ 1.40Ti4+ 0.46Mg0.12Fe2+ 0.02]Σ2(Si2.69Al0.20Fe3+ 0.11)Σ3O12 [phase-2, 23.9(1) wt%]. Phase-2 (average of four data points) contains more Ti4+ and less Fe3+ than phase-1 (average of four data points). In the X site, the amount of Ca is quite high (≈2.96 apfu instead of three). In the Y site, Fe3+ is the dominant cation followed by Ti4+. The Z site contains some Al and Fe3+ in phase-2. Distinct compositions for individual phases are not always observed by EMPA because the intergrowth of distinct cubic phases may occur on a fine scale.

The HRPXRD results clearly show that the Ti-rich andradite sample consists of a mixture of three cubic phases intergrown together, and is observed by splitting of diffraction peaks (Figure 2). Some other samples from Magnet Cove are single phase, cubic, optically isotropic, and contain no split diffraction peaks (unpublished results). The unit-cell parameters for each of the three cubic phases are slightly different from each other and each phase occurs in significant quantity, as shown by their weight percentages (wt%) in Table II.

The structural models for the Ti-rich andradite sample were refined quite well, as shown by the Rietveld statistics (Table II). The EMPA analyses show that the Ca(X) site occupancy factors (sofs) are very close to 1.0, and the refinement values are about 0.94 (Table III). The EMPA sofs for the Fe(Y) site are about 0.94, whereas the refinement values vary from about 0.82 to 0.85. The EMPA sofs for the Si(Z) site are close to 1.0, and the refinement values vary from about 0.96 to 1.04, so the hydroxyl substitution, (O4H4) ↔ SiO4, is negligible. The sofs are compared further in terms of differences between electrons and sofs obtained by Rietveld refinement and EMPA (Table III).

Figure 3 was constructed using average 〈X–O〉, Y–O, and Z–O distances that were obtained from single-crystal refinements using cubic space group, $Ia\overline 3 d$ , for single-phase garnet samples that may or may not be birefringent (Novak and Meyer, Reference Novak and Meyer1970; Novak and Gibbs, Reference Novak and Gibbs1971; Weber et al., Reference Weber, Virgo and Huggins1975; Munno et al., Reference Munno, Rossi and Tadini1980; Basso et al., Reference Basso, Dellagiusta and Zefiro1981, Reference Basso, Dellagiusta and Zefiro1983, Reference Basso, Cimmino and Messiga1984a, Reference Basso, Cimmino and Messiga1984b; Sacerdoti and Passaglia, Reference Sacerdoti and Passaglia1985; Lager et al., Reference Lager, Rossman, Rotella and Schultz1987a, Reference Lager, Armbruster and Faber1987b, Reference Lager, Armbruster, Rotella and Rossman1989; Smyth et al., Reference Smyth, Madel, McCormick, Munoz and Rossman1990; Armbruster et al., Reference Armbruster, Geiger and Lager1992, Reference Armbruster, Birrer, Libowitzky and Beran1998; Geiger et al., Reference Geiger, Armbruster, Lager, Jiang, Lottermoser and Amthauer1992; Armbruster and Geiger, Reference Armbruster and Geiger1993; Armbruster, Reference Armbruster1995; Peterson et al., Reference Peterson, Locock and Luth1995; Geiger and Armbruster, Reference Geiger and Armbruster1997; Armbruster et al., Reference Armbruster, Birrer, Libowitzky and Beran1998; Scordari et al., Reference Scordari, Schingaro and Pedrazzi1999; Schingaro et al., Reference Schingaro, Scordari, Capitanio, Parodi, Smith and Mottana2001, Reference Schingaro, Scordari, Pedrazzi and Malitesta2004; Agrosì et al., Reference Agrosì, Schingaro, Pedrazzi, Scandale and Scordari2002; Gramaccioli et al., Reference Gramaccioli, Pilati and Demartin2002; Ferro et al., Reference Ferro, Galli, Papp, Quartieri, Szakall and Vezzalini2003; Chakhmouradian and McCammon, Reference Chakhmouradian and McCammon2005; Adamo et al., Reference Adamo, Gatta, Rotitoti, Diella and Pavese2010). Data for non-cubic, single-phase, single-crystal refinements for birefringent garnet samples are not included in Figure 3 (Takéuchi et al., Reference Takéuchi, Haga, Umizu and Sato1982; Allen and Buseck, Reference Allen and Buseck1988; Angel et al., Reference Angel, Finger, Hazen, Kanzaki, Weidner, Liebermann and Veblen1989; Kingma and Downs, Reference Kingma and Downs1989; Griffen et al., Reference Griffen, Hatch, Phillips and Kulaksiz1992; Nakatsuka et al., Reference Nakatsuka, Yoshiasa, Yamanaka, Ohtaka, Katsura and Ito1999a, Reference Nakatsuka, Yoshiasa, Yamanaka and Ito1999b; Shtukenberg et al., Reference Shtukenberg, Punin, Frank-Kamenetskaya, Kovalev and Sokolov2001, Reference Shtukenberg, Popov and Punin2005; Wildner and Andrut, Reference Wildner and Andrut2001; Frank-Kamenetskaya et al., Reference Frank-Kamenetskaya, Rozhdestvenskaya, Shtukenberg, Bannova and Skalkina2007). The mean 〈D–O〉 distance was calculated using the formula 〈D–O〉 = {(Z–O) + (Y–O) + (X–O) + (X′–O)}/4, and it is linear across the entire garnet series (a varies from about 11.44 to 12.57 Å). The average 〈X–O〉 distance across the garnet series falls on two straight lines that meet at grossular (Grs). The Z–O distance for the anhydrous garnets falls on three straight lines that meet at andradite (Adr) and Ti–Gt, which is a synthetic Ti-rich garnet (Weber et al., Reference Weber, Virgo and Huggins1975). The Y–O distance falls on four straight lines that meet at Grs, Adr, and Ti–Gt. The Y–O variations show the division of the garnet series into four sub-series, as indicated by the dashed vertical lines. For the hydroxy garnets (OH–Gt), the Y–O(OH–Gt) and Z–O(OH–Gt) distances behave differently from other anhydrous garnets, although the behavior of the average 〈X–O〉 distance is the same across the series. Other end members of the garnet series shown in Figure 3 are abbreviated using standard notations (Whitney and Evans, Reference Whitney and Evans2010). The three phases from this study are very close to the end-member andradite, ideally Ca3(Fe2 3+)Si3O12.

Figure 3. (Color online) Structural variations across the silicate garnet series. The Y–O, Z–O, and average 〈X–O〉 distances vary linearly with the a unit-cell parameter in different parts of the series. The mean 〈D–O〉 distance varies linearly with the a parameter across the entire series. The linear lines are based on literature data (see text). Standard abbreviations are used for garnet end members, including slm = schorlomite and kmz = kimzeyite. Data from this study are also shown.

All the bond distances obtained from this study are reasonable and agree with previous results (Table IV; Figure 3). This is not surprising as the single-crystal method samples the dominant phase, but data from possible minor phases are missing. The single-crystal method is an inadequate technique to examine multi-phase samples. The bond distances also compared well with those obtained from the sum of ionic radii (Table IV; Figure 3). The increase in Z–O distance compared to end-member andradite is not the result of (O4H4) ↔ SiO4 substitution, as in hydroxy garnet. Instead, the increase in Z–O is the result of (Fe3+, Al) ↔ Si substitution in the Z site (Table I). Similarly, the decrease in Y–O distance compared to end-member andradite, arises from the replacement of Fe3+ (0.645 Å) by smaller Ti4+ (0.605 Å). Since the mean 〈D–O〉 distance varies as a straight line and the average 〈X–O〉 distances by straight lines, for the Ti-andradite region, when Z–O expands, then Y–O contracts (Figure 3).

The crystal structure of the individual phases in andradite can also be rationalized using bond-valence sums (BVS) calculated in valence units (v.u) (Wills and Brown, Reference Wills and Brown1999). For example, for dominant phase-1, the BVS for Ca atom at X site is 2.30 v.u., whereas BVS for Mn and Mg atoms in this site are low. This means that the Ca atom is a bit large for the X site, whereas the Mg atom is too small, so it rattles and gives rise to large displacement parameters, especially in pyrope; these facts are well known. For Ti4+ at the Y site, the BVS is 3.60 v.u., whereas for Fe3+, it is 2.89 v.u. The BVS for Si at Z site is 3.75 v.u., which is reasonable because there are some Al and Fe atoms in this site (Table I).

The formation of multi-phase cubic intergrowths may be related to changes in oxygen fugacity (f O2), activity of SiO2 (a SiO2), etc., as the crystals grow at low temperature that prevents diffusion or homogenization of the cations. Such intergrowths are similar to hetero-epitaxial or epitaxial growths because of the similarity of the structural and chemical parameters in individual cubic phases. Slight structural differences between the cubic phases give rise to structural mismatch that result in strain and optical anisotropy. A measure of strain can be obtained from the LY profile term in GSAS refinement. For a single-phase cubic garnet, the LY value is small compared to those for the multi-phase andradite, which has larger LY values, indicating larger strain (Table II).

Garnet-group minerals are important as they occur over a range of pressures, temperatures, and chemical compositions. They are common in metamorphic rocks and in xenoliths derived from the Earth's mantle. It is important to know the structural effects that arise from cation substitutions so as to interpret the complex (ideal or non-ideal) thermodynamic behavior observed in garnet solid solutions (Ganguly et al., Reference Ganguly, Cheng and O'Neill1993), and their petrogenetic significances. Most geothermometers and geobarometers involve garnet as one of the phases (Ganguly et al., Reference Ganguly, Cheng and O'Neill1993).

Majorite garnet, (Mg,Fe)SiO3, is considered to be a major constituent of the Earth's transition zone between the 400- and 670-km discontinuities (Ringwood, Reference Ringwood1967; Akaogi and Akimoto, Reference Akaogi and Akimoto1977; Liu, Reference Liu1977). Anisotropic MgSiO3, that is, {Mg3}(MgSi)[Si3O12], garnet accounts for a large fraction of the Earth's upper mantle above the 670-km discontinuity (Ito and Takahashi, Reference Ito, Takahashi, Manghnani and Syono1987), and was reported to be birefringent with tetragonal symmetry (Kato and Kumazawa, Reference Kato and Kumazawa1985; Sawamoto, Reference Sawamoto, Manghnani and Syono1987; Angel et al., Reference Angel, Finger, Hazen, Kanzaki, Weidner, Liebermann and Veblen1989; Parise et al., Reference Parise, Wang, Gwanmesia, Zhang, Sinelnikov, Chmielowski, Weidner and Liebermann1996; Nakatsuka et al., Reference Nakatsuka, Yoshiasa, Yamanaka, Ohtaka, Katsura and Ito1999a, Reference Nakatsuka, Yoshiasa, Yamanaka and Ito1999b). High-pressure synthesis has produced ABO3 tetragonal phases such as CaGeO3, CdGeO3 (Prewitt and Sleight, Reference Prewitt and Sleight1969; Nakatsuka et al., Reference Nakatsuka, Chaya and Yoshiasa2005), and MnSiO3 (Fujino et al., Reference Fujino, Momoi, Sawamoto and Kumazawa1986), in which equal numbers of A and B atoms are ordered on the Y site. If cation order and cubic symmetry reduction occur for birefringent ABO3 garnet, then the thermodynamic properties of MgSiO3 garnet in the mantle will change significantly, and that has important geophysical consequences. The configurational entropy of an ordered tetragonal MgSiO3 garnet would be reduced relative to a disordered cubic phase, and gives rise to a reduction in enthalpy. Therefore, possible cubic to tetragonal phase transition in MgSiO3 garnet and the associated cation ordering on the Y site are of considerable interest in terms of thermodynamic effects and seismic velocities in the transition zone. Contrary to the structural results obtained so far on birefringent ABO3 garnet samples that undergo a cubic to tetragonal transition, the results from this study suggest that they may be cubic and may occur as a mixture of cubic phases, hence further work remains to be done on ABO3 garnet samples. Birefringence in natural samples disappears at about 800 °C and may or may not reappear on cooling (Prewitt and Sleight, Reference Prewitt and Sleight1969).

Natural garnet such as uvarovite, {Ca3}[Cr3+ 2](Si3)O12; grossular, {Ca3}[Al2](Si3)O12; andradite, {Ca3}[Fe3+ 2](Si3)O12; spessartine, {Mn2+ 3}[Al2](Si3)O12; almandine, {Fe2+ 3}[Al2](Si3)O12; and hydrogarnets can be birefringent and consist of a mixture of two or three cubic phases, whereas other garnets such as pyrope, {Mg3}[Al2](Si3)O12, is isotropic, if they occur as a single cubic phase (unpublished results). The hydrogarnet series between grossular, {Ca3}[Al2](SiO4)3 − {Ca3}[Al2](O4H4)3, (Si-free katoite), is known as “hydrogrossular”, and between andradite {Ca3}[Fe3+ 2](SiO4)3 − {Ca3}[Fe3+ 2](O4H4)3, is known as “hydroandradite”. Unusual and elongated atomic displacement parameters along the “Si-O” bond for the O atoms in hydrogarnets are usually modeled by split O-atom positions (e.g., Lager et al., Reference Lager, Armbruster and Faber1987b; Armbruster and Lager, Reference Armbruster and Lager1989; Ferro et al., Reference Ferro, Galli, Papp, Quartieri, Szakall and Vezzalini2003), but they are the result of multiple cubic phases (unpublished results). Similar unusual O-atom features were also observed for the Ice River morimotoite (Peterson et al., Reference Peterson, Locock and Luth1995), and are also the result of multiple phases (unpublished results). The crystal structure of birefringent andradite samples was recently discussed by Antao and Klincker (Reference Antao and Klincker2013) and Antao (Reference Antao2013); the crystal structure of other birefringent garnets will be published elsewhere. Birefringent garnet consists of a mixture of two or more cubic phases. This mixture causes strain that arises from structural mismatch and makes the garnet birefringent. A long standing problem on birefringent garnets now appears to be solved.

ACKNOWLEDGEMENTS

I thank the two anonymous reviewers for their comments. I also thank J. Nicholls for his comments on an earlier draft of this manuscript. J. Michael Howard is thanked for providing a suite of Ti-rich andradite samples from Magnet Cove, Hot Spring County, Arkansas. R. Marr is thanked for help with the EMPA data collection. The HRPXRD data were collected at the X-ray Operations and Research beamline 11-BM, Advanced Photon Source (APS), Argonne National Laboratory (ANL). Use of the APS was supported by the U.S. Dept. of Energy, Office of Science, Office of Basic Energy Sciences, under Contract No. DE-AC02-06CH11357. This work was supported with an NSERC Discovery Grant and an Alberta Ingenuity Award.

References

Adamo, I., Gatta, G. D., Rotitoti, N., Diella, V., and Pavese, A. (2010). “Green andradite stones: gemological and mineralogical characterisation,” Eur. J. Miner. 23, 91–100.Google Scholar
Agrosì, G., Schingaro, E., Pedrazzi, G., Scandale, E., and Scordari, R. (2002). “A crystal chemical insight into sector zoning of a titanian andradite (“melanite”) crystal,” Eur. J. Miner. 14, 785–794.CrossRefGoogle Scholar
Akaogi, M. and Akimoto, S. (1977). “Pyroxene-garnet solid-solution equilibria in the systems Mg4Si4012-Mg3Al2Si3O12 and Fe4Si4O12-Fe3Al2Si3O12 at high pressures and temperatures,” Phys. Earth Planet. Interiors 15, 90–106.CrossRefGoogle Scholar
Akizuki, M. (1984). “Origin of optical variations in grossular-andradite garnet,” Am. Miner. 66, 403–409.Google Scholar
Akizuki, M., Takéuchi, Y., Terada, T., and Kudoh, Y. (1998). “Sectoral texture of a cubo-dodecahedral garnet in grandite,” Neues Jahrbuch für Mineralogie, Monatshefte 12, 565–576.Google Scholar
Allen, F. M. and Buseck, P. R. (1988). “XRD, FTIR, and TEM studies of optically anisotropic grossular garnets,” Am. Miner. 73, 568–584.Google Scholar
Angel, R., Finger, L. W., Hazen, R. M., Kanzaki, M., Weidner, D. J., Liebermann, R. C., and Veblen, D. R. (1989). “Structure and twinning of single-crystal MgSiO3 garnet synthesized at 17 GPa and 1800 °C,” Am. Miner. 74, 509–512.Google Scholar
Antao, S. M. (2013). “Three cubic phases intergrown in a birefringent andradite-grossular garnet and their implications,” Phys. Chem. Miner. DOI: 10.1007/s00269-013-0606-4.Google Scholar
Antao, S. M. and Klincker, A. M. (2013). “Origin of birefringence in andradite from Arizona, Madagascar, and Iran,” Phys. Chem. Miner. 40, 575–586.Google Scholar
Antao, S. M., Hassan, I., Wang, J., Lee, P. L., and Toby, B. H. (2008). “State-of-the-art high-resolution powder X-ray diffraction (HRPXRD) illustrated with Rietveld structure refinement of quartz, sodalite, tremolite, and meionite,” Can. Miner. 46, 1501–1509.Google Scholar
Antao, S. M., Klincker, A. M., and Round, S. A. (2013a). “Origin of birefringence in common silicate garnet: intergrowth of different cubic phases,” Am. Geophys. Union Conference, Cancun, Mexico, 14–17 May, 2013.Google Scholar
Antao, S. M., Klincker, A. M., and Round, S. A. (2013b). “Some garnets are cubic and birefringent, why?,” Conference, Hawaii, USA, 20–24 July, 2013.Google Scholar
Armbruster, T. (1995). “Structure refinement of hydrous andradite, Ca3Fe1.54Mn0.02Al0.26(SiO4)1.65(O4H4)1.35, from the Wessels mine, Kalahari manganese field, South Africa,” Eur. J. Miner. 7, 1221–1225.Google Scholar
Armbruster, T. and Geiger, C. A. (1993). “Andradite crystal chemistry, dynamic x-site disorder and structural strain in silicate garnets,” Eur. J. Miner. 5, 59–71.Google Scholar
Armbruster, T. and Lager, G. A. (1989). “Oxygen disorder and the hydrogen position in garnet-hydrogarnet solid-solutions,” Eur. J. Miner. 1, 363–369.Google Scholar
Armbruster, T., Geiger, C. A., and Lager, G. A. (1992). “Single crystal X-ray structure study of synthetic pyrope-almandine garnets at 100 and 293 K,” Am. Miner. 77, 518–527.Google Scholar
Armbruster, T., Birrer, J., Libowitzky, E., and Beran, A. (1998). “Crystal chemistry of Ti-bearing andradites,” Eur. J. Miner. 10, 907–921.Google Scholar
Badar, M. A., Akizuki, M., and Hussain, S. (2010). “Optical anomaly in iridescent andradite from the Sierra Madre mountains, Sonora, Mexico,” Can. Miner. 48, 1195–1203.Google Scholar
Badar, M. A., Niaz, S., Hussain, S., and Akizuki, M. (2013). “Lamellar texture and optical anomaly in andradite from the Kamaishi mine, Japan,” Eur. J. Miner. 25, 53–60.CrossRefGoogle Scholar
Basso, R., Dellagiusta, A., and Zefiro, L. (1981). “A crystal chemical study of a Ti-containing hydrogarnet,” Neues Jahrbuch Fur Mineralogie-Monatshefte 5, 230–236.Google Scholar
Basso, R., Dellagiusta, A., and Zefiro, L. (1983). “Crystal-structure refinement of plazolite - a highly hydrated hatural hydrogrossular,” Neues Jahrbuch Fur Mineralogie-Monatshefte 6, 251–258.Google Scholar
Basso, R., Cimmino, F., and Messiga, B. (1984a). “Crystal-chemistry of hydrogarnets from three different microstructural sites of a basaltic metarodingite from the Voltri-Massif (Western Liguria, Italy),” Neues Jahrbuch Fur Mineralogie-Abhandlungen 148, 246–258.Google Scholar
Basso, R., Cimmino, F., and Messiga, B. (1984b). “Crystal chemical and petrological study of hydrogarnets from a Fe-gabbro metarodingite (Gruppo Di Voltri, Western Liguria, Italy),” Neues Jahrbuch Fur Mineralogie-Abhandlungen 150, 247–258.Google Scholar
Bertaut, F. and Forrat, F. (1956). “Structures des ferrites ferrimagnétiques des terres rare,” C. R. Acad. Sci. 243, 382–384.Google Scholar
Blanc, Y. and Maisonneuve, J. (1973). “Sur la biréfringence des grenats calciques,” Bull. Soc. Franç. Minér. Cristallogr. 96, 320–321.Google Scholar
Brauns, R. (1891). Die optischen Anomalien der Kristalle. Preisschr. (Jablonowski Ges., Leipzig, Germany).Google Scholar
Brown, D. and Mason, R. A. (1994). “An occurrence of sectored birefringence in almandine from the Gangon terrane, Labrador,” Can. Miner. 32, 105–110.Google Scholar
Chakhmouradian, A. R. and McCammon, C. A. (2005). “Schorlomite: a discussion of the crystal chemistry, formula, and inter-species boundaries,” Phys. Chem. Miner. 32, 277–289.CrossRefGoogle Scholar
Chase, A. B. and Lefever, R. A. (1960). “Birefringence of synthetic garnets,” Am. Miner. 45, 1126–1129.Google Scholar
Droop, G. T. R. (1987). “A general equation for estimating Fe3+ concentrations in ferromagnesian silicates and oxides from microprobe analyses, using stoichiometric criteria,” Miner. Mag. 51, 431–435.Google Scholar
Ferro, O., Galli, E., Papp, G., Quartieri, S., Szakall, S., and Vezzalini, G. (2003). “A new occurrence of katoite and re-examination of the hydrogrossular group,” Eur. J. Miner. 15, 419–426.Google Scholar
Foord, E. E. and Mills, B. A. (1978). “Biaxiality in “isometric” and “dimetric” crystals,” Am. Miner. 63, 316–325.Google Scholar
Frank-Kamenetskaya, O. V., Rozhdestvenskaya, L. V., Shtukenberg, A. G., Bannova, I. I., and Skalkina, Y. A. (2007). “Dissymmetrization of crystal structures of grossular-andradite garnets Ca3(Al, Fe)2(SiO4)3 ,” Struct. Chem. 18, 493–503.CrossRefGoogle Scholar
Fujino, K., Momoi, H., Sawamoto, H., and Kumazawa, M. (1986). “Crystal structure and chemistry of MnSiO3 tetragonal garnet,” Am. Miner. 71, 781–785.Google Scholar
Ganguly, J., Cheng, W., and O'Neill, H. S. C. (1993). “Syntheses, volume, and structural changes of garnets in the pyrope-grossular join: implications for stability and mixing properties,” Am. Miner. 78, 583–593.Google Scholar
Geiger, C. A. and Armbruster, T. (1997). “Mn3Al2Si3O12 spessartine and Ca3Al2Si3O12 grossular garnet: structural dynamic and thermodynamic properties,” Am. Miner. 82, 740–747.Google Scholar
Geiger, C. A., Armbruster, T., Lager, G. A., Jiang, K., Lottermoser, W., and Amthauer, G. (1992). “A combined temperature dependent 57Fe Mössbauer and single crystal X-ray diffraction study of synthetic lmandine: evidence for the Gol'danskii-Karyagin effect,” Phys. Chem. Miner. 19, 121–126.Google Scholar
Geller, S. (1967). “Crystal chemistry of garnets,” Z. Kristal 125, 1–47.Google Scholar
Geller, S. and Gilleo, M. A. (1957). “Structure and ferrimagnetism of yttrium and rare earth iron garnets,” Acta Crystallogr. 10, 239.Google Scholar
Geusic, J. E., Marcos, H. M., and Van Uitert, L. G. (1964). “Laser oscillations in Nd-doped yttrium aluminum, yttrium gallium and gadolinium garnets,” Appl. Phys. Lett. 4, 182–184.CrossRefGoogle Scholar
Gramaccioli, C. M., Pilati, T., and Demartin, F. (2002). “Atomic displacement parameters for spessartine Mn3Al2Si3O12 and their lattice-dynamical interpretation,” Acta Crystallogr. B58, 965–969.CrossRefGoogle Scholar
Griffen, D. T., Hatch, D. M., Phillips, W. R., and Kulaksiz, S. (1992). “Crystal chemistry and symmetry of a birefringent tetragonal pyralspite75-grandite25 garnet,” Am. Miner. 77, 399–406.Google Scholar
Hatch, D. M. and Ghose, S. (1989). “Symmetry analysis of the phase transition and twinning in MgSiO3 garnet: implications to mantle mineralogy,” Am. Miner. 74, 1221–1224.Google Scholar
Henmi, C., Kusachi, I., and Henmi, K. (1995). “Morimotoite, Ca3TiFe2+Si3O12, a new titanian garnet from Fuka, Okayama Prefecture, Japan,” Miner. Mag. 59, 115–120.Google Scholar
Hofmeister, A. M., Schaal, R. B., Campbell, K. R., Berry, S. L., and Fagan, T. J. (1998). “Prevalence and origin of birefringence in 48 garnets from the pyrope-almandine- grossularite-spessartine quaternary,” Am. Miner. 83, 1293–1301.Google Scholar
Ingerson, E. and Barksdale, J. D. (1943). “Iridescent garnet from the Adelaide mining district, Nevada,” Am. Miner. 28, 303–312.Google Scholar
Ito, E. and Takahashi, E. (1987). “Ultrahigh pressure phase transformations and the constitution of the deep mantle,” in High Pressure Research in Mineral Physics: A Volume in Honor of Syun-iti Akimoto, Geophysical Monograph, edited by Manghnani, M. H. and Syono, Y., AGU, Washington, D.C. Vol. 39, pp. 221–229.Google Scholar
Kato, T. and Kumazawa, M. (1985). “Garnet phase of MgSiO3 filling the pyroxene-ilmenite gap at very high temperature,” Nature 316, 803–805.Google Scholar
Kingma, K. J. and Downs, J. W. (1989). “Crystal-structure analysis of a birefringent andradite,” Am. Miner. 74, 1307–1316.Google Scholar
Kitamura, K. and Komatsu, H. (1978). “Optical anisotropy associated with growth striation of yttrium garnet, Y3(Al,Fe)5O12 ,” Kristallogr. Tech. 13, 811–816.Google Scholar
Lager, G. A., Rossman, G. R., Rotella, F. J., and Schultz, A. J. (1987a). “Neutron-diffraction structure of a low-water grossular at 20 K,” Am. Miner. 72, 766–768.Google Scholar
Lager, G. A., Armbruster, T., and Faber, J. (1987b). “Neutron and X-ray-diffraction study of hydrogarnet Ca3Al2(O4H4)3 ,” Am. Miner. 72, 756–765.Google Scholar
Lager, G. A., Armbruster, T., Rotella, F. J., and Rossman, G. R. (1989). “OH substitution in garnets: X-ray and neutron diffraction, infrared, and geometric-modeling studies,” Am. Miner. 74, 840–851.Google Scholar
Larson, A. C. and Von Dreele, R. B. (2000). General Structure Analysis System (GSAS) (Report LAUR 86-748). Los Alamos National Laboratory.Google Scholar
Lee, P. L., Shu, D., Ramanathan, M., Preissner, C., Wang, J., Beno, M. A., Von Dreele, R. B., Ribaud, L., Kurtz, C., Antao, S. M., Jiao, X., and Toby, B. H. (2008). “A twelve-analyzer detector system for high-resolution powder diffraction,” J. Synchrotron Radiat. 15, 427–432.Google Scholar
Lessing, P. and Standish, R. P. (1973). “Zoned garnet from Crested Butte, Colorado,” Am. Miner. 58, 840–842.Google Scholar
Liu, L. (1977). “The system enstatite-pyrope at high pressures and temperatures and the mineralogy of the Earth's mantle,” Earth Planet. Sci. Lett. 36, 237–245.Google Scholar
Locock, A. J. (2008). “An excel spreadsheet to recast analyses of garnet into end-member components, and a synopsis of the crystal chemistry of natural silicate garnets,” Comput. Geosci. 34, 1769–1780.Google Scholar
Munno, R., Rossi, G., and Tadini, C. (1980). “Crystal chemistry of kimzeyite from Stromboli, Aeolian Islands, Italy,” Am. Miner. 65, 188–191.Google Scholar
Nakatsuka, A., Yoshiasa, A., Yamanaka, T., Ohtaka, O., Katsura, T., and Ito, E. (1999a). “Symmetry change of majorite solid-solution in the system Mg3Al2Si3O12-MgSiO3 ,” Am. Miner. 84, 1135–1143.Google Scholar
Nakatsuka, A., Yoshiasa, A., Yamanaka, T., and Ito, E. (1999b). “Structure refinement of a birefringent Cr-bearing majorite Mg3(Mg0.34Si0.34Al0.18Cr0.14)2Si3O12 ,” Am. Miner. 84, 199–202.Google Scholar
Nakatsuka, A., Chaya, H., and Yoshiasa, A. (2005). “Crystal structure of single-crystal CaGeO3 tetragonal garnet synthesized at 3 GPa and 1000 °C,” Am. Miner. 90, 755–757.Google Scholar
Novak, G. A. and Gibbs, G. V. (1971). “The crystal chemistry of the silicate garnets,” Am. Miner. 56, 1769–1780.Google Scholar
Novak, G. A. and Meyer, H. O. A. (1970). “Refinement of the crystal structure of a chrome pyrope garnet: an inclusion in natural diamond,” Am. Miner. 55, 2124–2127.Google Scholar
Parise, J. B., Wang, Y., Gwanmesia, G. D., Zhang, J., Sinelnikov, Y., Chmielowski, J., Weidner, D. J., and Liebermann, R. C. (1996). “The symmetry of garnets on the pyrope (Mg3Al2Si3O12) – majorite (MgSiO3) join,” Geophys. Res. Lett. 23, 3799–3802.Google Scholar
Peterson, R. C., Locock, A. J., and Luth, R. W. (1995). “Positional disorder of oxygen in garnet: the crystal-structure refinement of schorlomite,” Can. Miner. 33, 627–631.Google Scholar
Prewitt, C. T. and Sleight, A. W. (1969). “Garnet-like structures of high-pressure cadmium germanate and calcium germanate,” Science 163, 386–387.Google Scholar
Rietveld, H. M. (1969). “A profile refinement method for nuclear and magnetic structures,” J. Appl. Crystallogr. 2, 65–71.Google Scholar
Ringwood, A. E. (1967). “The pyroxene-garnet transformation in the Earth's mantle,” Earth Planet. Sci. Lett. 2, 255–263.Google Scholar
Rossman, G. R. and Aines, R. D. (1986). “Spectroscopy of a birefringent grossular from Asbestos, Quebec, Canada,” Am. Miner. 71, 779–780.Google Scholar
Sacerdoti, M. and Passaglia, E. (1985). “The crystal structure of katoite and implications within the hydrogrossular group of minerals,” Bull. Miner. 108, 1–8.Google Scholar
Sawamoto, H. (1987). “Phase diagram of MgSiO3 at pressures up to 24 GPa and temperatures up to 2200 °C: phase stability and properties of tetragonal garnet,” in High Pressure Research in Mineral Physics: A Volume in Honor of Syun-iti Akimoto, Geophysical Monograph, edited by Manghnani, M. H. and Syono, Y., AGU, Washington, D.C. Vol. 39, 209–219.Google Scholar
Schingaro, E., Scordari, F., Capitanio, F., Parodi, G., Smith, D. C., and Mottana, A. (2001). “Crystal chemistry of kimzeyite from Anguillara, Mt. Sabatini, Italy,” Eur. J. Miner. 13, 749–759.Google Scholar
Schingaro, E., Scordari, F., Pedrazzi, G., and Malitesta, C. (2004). “Ti and Fe speciation by X-ray photoelectron spectroscopy (XPS) and mössbauer spectroscopy for a full crystal chemical characterisation of Ti-garnets from Colli Albani (Italy),” Ann. Chim. 94, 185–196.Google Scholar
Scordari, F., Schingaro, E., and Pedrazzi, G. (1999). “Crystal chemistry of melanites from Mt. Vulture (Southern Italy),” Eur. J. Miner. 11, 855–869.Google Scholar
Shannon, R. D. (1976). “Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides,” Acta Crystallogr. Sect. A: Cryst. Phys., Diffr., Theor. Gen. Crystallogr. 32, 751–767.Google Scholar
Shtukenberg, A. G., Punin, Y. O., Frank-Kamenetskaya, O. V., Kovalev, O. G., and Sokolov, P. B. (2001). “On the origin of anomalous birefringence in grandite garnets,” Miner. Mag. 65, 445–459.Google Scholar
Shtukenberg, A. G., Popov, D. Y., and Punin, Y. O. (2005). “Growth ordering and anomalous birefringence in ugrandite garnets,” Miner. Mag. 69, 537–550.CrossRefGoogle Scholar
Smyth, J. R., Madel, R. E., McCormick, T. C., Munoz, J. L., and Rossman, G. R. (1990). “Crystal-structure refinement of a F-bearing spessartine garnet,” Am. Miner. 75, 314–318.Google Scholar
Takéuchi, Y., Haga, N., Umizu, S., and Sato, G. (1982). “The derivative structure of silicate garnets in grandite,” Z. Kristallogr. 158, 53–99.Google Scholar
Toby, B. H. (2001). “EXPGUI, a graphical user interface for GSAS,” J. Appl. Crystallogr. 34, 210–213.Google Scholar
Wang, J., Toby, B. H., Lee, P. L., Ribaud, L., Antao, S. M., Kurtz, C., Ramanathan, M., Von Dreele, R. B., and Beno, M. A. (2008). “A dedicated powder diffraction beamline at the advanced photon source: commissioning and early operational results,” Rev. Sci. Instrum. 79, 085105.Google Scholar
Weber, H. P., Virgo, D., and Huggins, F. E. (1975). “A neutron-diffraction and 57Fe Mössbauer study of a synthetic Ti-rich garnet,” Carnegie Inst. Wash. Year Book 74, 575–579.Google Scholar
Whitney, D. L. and Evans, B. W. (2010). “Abbreviations for names of rock-forming minerals,” Am. Miner. 95, 185–187.Google Scholar
Wildner, M. and Andrut, M. (2001). “The crystal chemistry of birefringent natural uvarovites: part II. Single-crystal X-ray structures,” Am. Miner. 86, 1231–1251.Google Scholar
Wills, A. S. and Brown, I. D. (1999). VaList. CEA, France. This is a freely available computer program.Google Scholar
Figure 0

Figure 1. (Color online) Projection of the cubic garnet structure down c showing the ZO4 tetrahedra (gray), YO6 octahedra (yellow), and XO8 dodecahedra (blue) that occur as a distorted cubic shape. Dense packing of the polyhedra are obvious from the four unit cells displayed, which shows the prominent edge-sharing and zigzag arrangement of alternating octahedra and dodecahedra.

Figure 1

Table I. Electron microprobe analysisa for a Ti-rich andradite (MC9).

Figure 2

Table II. HRPXRD data and Rietveld refinement statistics.

Figure 3

Figure 2. HRPXRD trace for a Ti-rich andradite sample (MC9) from Magnet Cove together with the calculated (continuous line) and observed (crosses) profiles. The difference curve (Iobs − Icalc) is shown at the bottom. The short vertical lines indicate allowed reflection positions. (a) The intensities that are above 20 and 30°2θ are scaled by factors of ×10 and ×30, respectively, for both the trace and difference curves. (b) The fitted HRPXRD trace showing the reflections (840) and (842). Three cubic phases intergrown in the sample are evident, as indicated by the splitting of the diffraction peaks.

Figure 4

Table III. Atom coordinatesa, isotropic displacement parameters, U (Å2), and sofs.

Figure 5

Table IV. Selected distances (Å) in Ti-rich andradite (MC9).

Figure 6

Figure 3. (Color online) Structural variations across the silicate garnet series. The Y–O, Z–O, and average 〈X–O〉 distances vary linearly with the a unit-cell parameter in different parts of the series. The mean 〈D–O〉 distance varies linearly with the a parameter across the entire series. The linear lines are based on literature data (see text). Standard abbreviations are used for garnet end members, including slm = schorlomite and kmz = kimzeyite. Data from this study are also shown.