INTRODUCTION
Stimulated Brillouin scattering (SBS) has received general attention owing to its broad applications such as beam combination (Kong et al., Reference Kong, Yoon, Shin and Beak2008, Reference Kong, Shin, Yoon and Beak2009; Wang et al., Reference Wang, Lu, He, Zheng and Zhao2009a, Reference Wang, Lu, Wang, Zheng, He and Lin2009b; Ostermeyer et al., Reference Ostermeyer, Kong, Kovalev, Harrison, Fotiadi, Megret, Kalal, Slezak, Yoon, Shin, Beak, Lee, Lu, Wang, Lin, Knight, Kotova, Straber, Scheikhobeid, Riesbeck, Meister, Eichler, Wang, He, Yoshida, Fujita, Nakatsuka, Hatae, Park, Lim, Omatsu, Nawata, Shiba, Antipov, Kuznetsov and Zakharov2008), Brillouin amplification of weak signals (Gao et al., Reference Gao, Lu, He, Dong and Hasi2009; Lu et al., Reference Lu, Gao, He, Zhang and Hasi2009), optical limiting for laser protection in high-power laser (Hasi et al., Reference Hasi, Lu, Fu, Lu, Gong, Lin and He2009a, Reference Hasi, Guo, Lu, Fu, Gong, Geng, Lu, Lin and He2009b), and slow light in optical fiber (Kovalev et al., Reference Kovalev, Kotova and Harrison2009; Lu et al., Reference Lu, Dong and Li2007). Among the parameters that characterize SBS, the threshold value is of great importance. The definition of this parameter was first suggested to be pump intensity when the SBS amplification of the Stokes radiation overcomes its losses in a medium (Chiao et al., Reference Chiao, Townes and Stoicheff1964). However, the amplification of the Stokes signal corresponding to the point of transition is too weak to detect for most SBS media because of the lower losses. Thus, the threshold is usually considered as the pump intensity when the SBS reflectivity reaches 1%, 2%, or 5% (Boyd et al., Reference Boyd, Rzazewski and Narum1990; Eichler et al., Reference Eichler, Konig, Piitzold and Schwartz1995). As we know, this is a rather confusing experimental practice. Recently, Bai et al. (Reference Bai, Shi, Ouyang, Chen, Gong, Jing, Liu and Liu2008) presented a method to determine the threshold value of SBS by the point of the deviation of the value of the attenuation coefficients of wide- and narrow-line width lasers. We find it is feasible for some media (e.g., CS2, water, etc.), but it is not for other media (e.g., FC-75, FC-72, etc.). Because the SBS still occurs in these media such as FC-75 and FC-72 when the wide-line width laser is used (Lee et al., Reference Lee, Lee and Kong2005), the attenuation coefficients of the media will not be constants. Thus, the point of the deviation can not be determined.
Usually, it is more appropriate and convenient to characterize the steady-state or transient SBS by its threshold exponential gain, G th = gI thL eff, where g is the SBS gain coefficient of the medium, I th is the SBS threshold pump intensity, L eff is the effective interaction length (Kovalev & Harrison, Reference Kovalev and Harrison2007; Bel'dyugin et al., Reference Bel'dyugin, Efimkov, Mikhailov and Zubarev2005). Moreover, the value of G th can provide a practically important reference for the design of a Brillouin amplifier, since the optimum working point of the amplifier is usually near to G th (Sternklar et al., Reference Sternklar, Glick and Jackel1992). When the steady-state approximation is considered and 1% (SBS reflectivity) criterion for threshold is used, Boyd et al. (Reference Boyd, Rzazewski and Narum1990) predicted that the value of G th in organic liquids is in the range 20–25, and Kovalev and Harrison (Reference Kovalev and Harrison2007) found that the value of G th decreases with increasing the interaction length in optical fiber. When a laser system with the nanosecond-order pulse width is used, for the most media (the phonon lifetime is usually between 0.1 ns and 10 ns (Erokhin et al., Reference Erokhin, Kovalev and Faizullov1986; Yoshida et al., Reference Yoshida, Kmetik, Fujita, Nakatsuka, Yamanaka and Yoshida1997)), the transient theory should be use to analyze the SBS process (Bel'dyugin et al., Reference Bel'dyugin, Efimkov, Mikhailov and Zubarev2005). Nevertheless, to our knowledge, the dependence of G th on the pump wavelength and the interaction length in the transient regime has not been observed experimentally or treated theoretically.
In this paper, we present a new method to measure the SBS threshold, which is simple, accurate, and is not confined by the medium characteristics. By this method, we theoretically and experimentally investigate the dependences of the values of G th on the pump wavelength and the interaction length.
MEASUREMENT PRINCIPLE AND THRESHOLD CHARACTERISTICS FOR SBS
We assume a pump wave, 
, propagating in the +z direction, a backward Stokes wave, 
, and a forward acoustic wave, 
, within the SBS medium. The three-wave coupled wave equations to describe transient SBS including its spontaneous initiation from noise are written as (Gaeta & Boyd, Reference Gaeta and Boyd1991)
where E P, E S, and ρ are the amplitudes of the pump, the Stokes, and the acoustic waves, respectively; n is the refractive index of the medium; g 1 and g 2 are the coupling coefficients; α is the absorption coefficient; Γ = 1/τ 0 denotes the phonon decay rate, where τ 0 is the phonon lifetime. Also, f represents the Langevin noise source that describes the thermal excitation of acoustic waves and leads to the initiation of the SBS process. It is spatially and temporally δ-correlated Gaussian random process with zero mean:
where
characterizes the noise intensity. ρ and V are the density and sound speed of the medium, respectively; A is the cross-sectional area of the interaction region. Directly, integrating the ρ phonon from Eq. (1c), substituting it into Eqs. (1a) and (1b), and using the transforms
, and 
, we have
![\eqalign{\left({\partial \over {\partial z}}+{n \over c}{\partial \over {\partial t}} \right)A_{\rm P} & = - {{g\Gamma } \over 4}A_{\rm S} \vint\limits_0^t A_{\rm P} A_{\rm S}^{\ast} \exp \left[- {\Gamma \over 2}\left({t - \tau } \right)\right]{\rm d}\tau \cr & \quad +{\rm i}g_1 A_{\rm S} \vint\limits_0^t f \exp \left[- {\Gamma \over 2}\left({t - \tau } \right)\right]{\rm d}\tau - {1 \over 2}\alpha A_{\rm P}\comma \;}](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20151021110251605-0723:S0263034610000054_eqn6.gif?pub-status=live)
![\eqalign{\left({\partial \over {\partial z}} - {n \over c}{\partial \over {\partial t}} \right)A_{\rm S} & = - {{g\Gamma } \over 4}A_{\rm P} \vint\limits_0^t A_{\rm P}^{\ast} A_{\rm S} \exp \left[- {\Gamma \over 2}\left({t - \tau } \right)\right]{\rm d}\tau \cr & \quad- {\rm i}g_1 A_{\rm P} \vint\limits_0^t {\,f^{\ast}} \exp \left[- {\Gamma \over 2}\left({t - \tau } \right)\right]{\rm d}\tau +{1 \over 2}\alpha A_{\rm S} \comma \;}](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20151021110251605-0723:S0263034610000054_eqn7.gif?pub-status=live)
where g is the SBS gain coefficient. The beam intensity is defined as I P,S = | A P,S|2. By implicit finite difference in time and backward difference in space, Eq. (4) can be numerically solved.
We consider an incident pump pulse of Gaussian temporal shape, which is input at z = 0, and output at z = L (L is the cell length). The boundary conditions are given as: ![$A_{\rm P} \left({0\comma \; t} \right)=A_{{\rm P}0} \exp \left\{{ - 2\ln 2\left[{\left({t - t_0 } \right)/t_{\rm P} } \right]^2 } \right\}\comma \; A_{\rm S} \lpar L\comma \; t\rpar =0$](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20151021110251605-0723:S0263034610000054_inline6.gif?pub-status=live){kind=small}
. The pump output energy can be expressed as 
. The system's exponential gain is defined as G = gI P0L eff, where I P0 = |A P0|2 is the peak intensity of the incident pump pulse. Obviously, for given g and L eff, the value of G is changed with I P0. Figure 1 shows that the dependence of the output energy of the pump on the exponential gain G. In the calculation, the following parameters are used: the wavelength of the incident pump pulse is 532 nm with an 8 ns full width at half maximum, the interaction length is 60 cm. FC-72 is chosen as the SBS medium, its parameters are shown in Table 1. The simulation parameters are closely matched to those that are used for experiments.
Fig. 1. Theoretical simulation of the dependence of the output energy on G. Line AB represents a linear increase of output energy below the SBS threshold. Line CD shows the optical limiting above the SBS threshold. Dashed lines, linear fit of lines AB and CD. The vertical dashed-dotted lines represent intersection points that correspond to the SBS threshold.
Table 1. The parameters of some SBS media
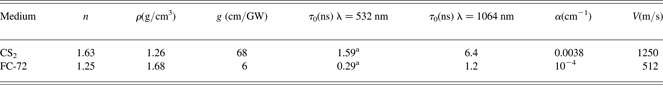
aThe parameters are calculated according to the equation given by Erokhin et al. (Reference Erokhin, Kovalev and Faizullov1986) and the results at 1064 nm (Erokhin et al., Reference Erokhin, Kovalev and Faizullov1986; Yoshida et al., Reference Yoshida, Kmetik, Fujita, Nakatsuka, Yamanaka and Yoshida1997); Others are quoted from Erokhin et al. (Reference Erokhin, Kovalev and Faizullov1986) and Yoshida et al. (Reference Yoshida, Kmetik, Fujita, Nakatsuka, Yamanaka and Yoshida1997).
We see that when the value of G is low, the SBS does not take place, and hence the line AB in Figure 1 shows a linear increase of output energy with G. After the pump intensity exceeds the SBS threshold, the energy quickly transfers from the pump to the Stokes. Consequently, the increase rate of output energy slows down (i.e., line CD), leading to an optical limiting effect. Line CD represents a nonlinear increase process. However, according to Figure 1, it can be considered as an approximately linear change before the gain saturation occurs. Thus, we perform linear fits for lines AB and CD. The value of G corresponding to the intersection point of two straight lines is defined as G th. At this time, the SBS reflectivity is about 2.5%, which agrees with the criterion for threshold generally accepted.
By using the model and method mentioned above, we obtain the values of G th for different wavelengths, λ, and interaction lengths, L eff, using CS2 and FC-72 as the SBS media. Their main medium parameters are listed in Table 1. Figure 2a shows the dependence of G th on λ. According to the literature (Damzen, et al., Reference Damzen, Hutchinson and Schroeder1987), the phonon lifetime of the medium, τ0, is proportional to λ2. Therefore, with the increase of λ, the ratio of the pump pulse width to the phonon lifetime, t P/τ0, decreases, leading to the stronger transient behavior (Bel'dyugin et al., Reference Bel'dyugin, Efimkov, Mikhailov and Zubarev2005; Maier & Renner, Reference Maier and Renner1971). This causes the increase of the values of I th and G th. On the other hand, we can see from Eq. (3) that the noise intensity, Q, is proportional to Γ, i.e., Q ∝ 1/τ0 · G th will be raised because of the reduced Q with increasing λ. Then, we choose λ as 532 nm and study the dependence of G th on L eff, as shown is in Figure 2b. At this time, t P/τ0 is 5 and 27 for CS2 and FC-72, respectively. Strictly speaking, the transient SBS process will occur in these two media (Bel'dyugin et al., Reference Bel'dyugin, Efimkov, Mikhailov and Zubarev2005). In the transient regime, the longer L eff for the same G means the smaller pump intensity, which results in a slower build up of the sound wave and hence a smaller Stokes signal intensity. Therefore, the larger G th is needed to attain the SBS threshold. It can also be seen from Figure 2 that the values of G th are very different for various media, and are larger than that of steady state (~20–25 (Boyd et al., Reference Boyd, Rzazewski and Narum1990)). This also indicates that G th increases as t P/τ0 decreases due to the transient effect.
Fig. 2. Dependences of G th in CS2 and FC-72 on (a) λ for L eff = 60 cm, (b) L eff for λ = 532 nm.
EXPERIMENTAL RESULTS AND DISCUSSIONS
Experimental setup is shown in Figure 3. The laser used is an injection-seeded, Q-switched, pulsed Nd:YAG laser (Continuum Powerlite Precision II 9010) with a line width of 90 MHz and repetition rate of 10 Hz. The output wavelength of the laser is 1064 nm and 532 nm (when the double frequency crystal is inserted), the pulse width is 7–8 ns, and the beam diameter is 8 mm. The pump energy is continuously varied by means of an attenuator, which consisted of a rotatable half-wave plate (λ/2) and a polarizer (P). A quarter-wave plate (λ/4), together with the polarizer P, forms a light isolator, preventing backward SBS light from entering YAG oscillator. A small fraction of the laser energy separated by the beam splitter (BS) and the output energy of the pump is measured by the PE50BB energy detectors (Ophir Optics), ED1 and ED2, respectively.
Fig. 3. Experimental setup for measuring SBS threshold.
CS2 and FC-72 are chosen as SBS media. It has been theoretically and experimentally validated that the scattering occurred in these media could only be SBS if the pulse energy of the laser is controlled in certain range. Other stimulated scatterings can be excluded (Bai et al., Reference Bai, Shi, Ouyang, Chen, Gong, Jing, Liu and Liu2008; Gao et al., Reference Gao, Lu, He, Hasi and Zhang2008; Daree & Kaiser, Reference Daree and Kaiser1971; Sen & Sen, Reference Sen and Sen1986). SBS generally takes place in the free gain length (FGL) determined by the pulse width of the pump beam (Bai et al., Reference Bai, Shi, Ouyang, Chen, Gong, Jing, Liu and Liu2008). In our experiments, the FGLs in CS2 and FC-72 are about 75 cm and 95 cm, respectively. If the cell length, L ≥ FGL, L eff = FGL, or else, L eff = L. Figure 4 shows that the output energy versus G at 532 nm and 1064 nm. The cell lengths are all 60 cm, which are all less than the FGLs. Therefore, L eff = 60 cm. To determine G th, the measured results below and above the SBS threshold are fitted into two straight dash lines. The vertical dash-dot lines denote intersection points that correspond to G th. The measured and theoretical G th and I th are summarized in Table 2. It can be seen that the values of G th and I th increase as λ. The reasons for this trend are discussed in the theoretical analysis.
Fig. 4. Output energy versus G in (a) CS2 at λ = 532 nm, (b) FC-72 at λ = 532 nm, (c) CS2 at λ = 1064 nm, and (d) FC-72 at λ = 1064 nm.
Table 2. Comparison of measured and theoretical threshold exponential gain Gth and the threshold value Ith for SBS
Figure 5 shows the dependence of G th in CS2 and FC-72 on the cell length, L. In the experiment, L are all less than FGLs, so L eff = L. The pump wavelength is 532 nm. It can be seen that G th increases as L. This result is different from that for steady-state SBS (Kovalev Harrison, 2007). The change tendencies of the experimental results are in agreement with those of the theoretical predictions in Figure 2b.
Fig. 5. G thversus L.
However, as seen from Table 2, there are the discrepancies between the experimental and theoretical values. The reasons for the errors may be summarized as follows. First, some main medium parameters such as g and τ0 in the calculation are not completely consistent with those in the experiment, especially for the case of λ = 532 nm. Most of data in Table 1 quoted from the literatures are experimentally obtained at 1064 nm; whereas the parameters at 532 nm are calculated according to the equation given by Erokhin et al. (Reference Erokhin, Kovalev and Faizullov1986) and the results at 1064 nm. Therefore, the relative measurement errors at 532 nm (~8%) are larger than those at 1064 nm (<5%). Second, we find that the absorption coefficient has greater influence on the calculated results. In the theoretical calculation, we introduce the linear absorption coefficients without considering the nonlinear absorption that may occur in the media. Finally, one-dimensional coupled wave equations as shown in Eq. (1) approximately describe the SBS characteristics. If more accurate, Gaussian spatial and spectral distribution of the pump beam should be taken into account.
CONCLUSION
Based on the output energy characteristic of SBS optical limiting, the SBS threshold or its exponential gain can be determined by the intersection point of linear-fitting lines of the output energy below and above the threshold. This method is suitable for various SBS media with the advantages of simple operation and high accuracy. We experimentally and theoretically investigate the dependences of the threshold value of exponential gain, G th, on the pump wavelength and the interaction length. The results indicate that G th increases with the pump wavelength and the interaction length. For Nd:YAG laser commonly used with a nanosecond-order pulse width, the values of G th for various media are very different. Unlike the case of steady-state SBS, transient SBS has no similar criterion for estimating SBS threshold. Therefore, in some applications such as Brillouin amplification, SBS threshold should be estimated according to the medium properties, length, and the wavelength in order to determine the optimum working point of the amplifier.
ACKNOWLEDGMENTS
This work is supported by the National Natural Science Foundation of China (Grant No. 60878005, 60778019) and the Program of Excellent Team in Harbin Institute of Technology.
