A machine learning of the unknown a priori viscous damping, incorporated into the single-dominant nonlinear ‘inviscid’ modal theory by Faltinsen et al. (J. Fluid Mech., vol. 407, 2000, pp. 201–234) on resonant sloshing (the forcing frequency close to the lowest natural sloshing frequency) in a clean (no internal structures) rigid rectangular tank, is proposed. The learning procedure requires a set of measured phase lags between the harmonic horizontal tank excitation and the steady-state resonant wave response. A good consistency with experiments by Bäuerlein & Avila (J. Fluid Mech., vol. 925, 2021, A22) on the liquid-mass centre motions is shown. The latter confirms that the free-surface nonlinearity (causing an energy flow from the primary-excited to higher natural sloshing modes) and viscous damping of the higher natural sloshing modes matter, as well as that the damping rates can depend on the steady-state wave amplitude.

Droplet atomization through aerobreakup is omnipresent in various natural and industrial processes. Atomization of Newtonian droplets is a well-studied area; however, non-Newtonian droplets have received less attention despite their being frequently encountered. By subjecting polymeric droplets of different concentrations to the induced airflow behind a moving shock wave, we explore the role of elasticity in modulating the aerobreakup of viscoelastic droplets. Three distinct modes of aerobreakup are identified for a wide range of Weber number $({\sim }10^2\unicode{x2013}10^4)$ and elasticity number $({\sim }10^{-4}\unicode{x2013}10^2)$ variation: these modes are vibrational, shear-induced entrainment and catastrophic breakup modes. Each mode is described as a three-stage process. Stage I is droplet deformation, stage II is the appearance and growth of hydrodynamic instabilities and stage III is the evolution of liquid mass morphology. It is observed that elasticity plays an insignificant role in the first two stages but a dominant role in the final stage. The results are described with the support of adequate mathematical analysis.

The helix approach is a new individual pitch control method to mitigate wake effects of wind turbines. Its name is derived from the helical shape of the wake caused by a rotating radial force exerted by the turbine. While its potential to increase power production has been shown in previous studies, the physics of the helical wake are not well understood to date. Open questions include whether the increased momentum in the wake stems from an enhanced wake mixing or from the wake deflection. Furthermore, its application to a row of more than two turbines has not been examined before. We study this approach in depth from both an analytical and numerical perspective. We examine large-eddy simulations (LES) of the wake of a single turbine and find that the helix approach exhibits both higher entrainment and notable deflection. As for the application to a row of turbines, we show that the phase difference between two helical wakes is independent of ambient turbulence. Examination of LES of a row of three turbines shows that power gains greatly depend on the phase difference between the helices. We find a maximum increase in the total power of approximately 10 % at a phase difference of $270^\circ$. However, we do not optimise the phase difference any further. In summary, we provide a set of analytical tools for the examination of helical wakes, show why the helix approach is able to increase power production, and provide a method to extend it to a wind farm.

The objective of this paper is to understand the effects of waves and vaporous cavitation upon the hydrodynamic and hydroelastic responses of a flexible surface-piercing hydrofoil, adding to the subcavitating results presented in Part 1. In general, the presence of a sufficiently large vaporous cavitation bubble facilitated the formation of a ventilated cavity, substantially reducing the angle of attack or speed required to induce fully ventilated flow, relative to subcavitating conditions. A new co-analysis procedure was used to isolate synchronous hydrodynamic modes and structural operating deflection shapes. Significant dynamic load amplification occurred when the resonant frequencies of the first twisting and second bending modes coalesced in both fully wetted and partially cavitating flows. The presence of waves did not diminish the effect of frequency coalescence, but did encourage intermittent lock-in with both leading edge cavity shedding and trailing edge vortex shedding at certain speeds. Partial cavity shedding typically had a negligible impact on the power spectral densities of structural motions because of incoherent cavity shedding. However, lock-in between the cavity shedding frequency and modal coalescence frequency led to shifting of the primary frequency peak, as well as amplified harmonics and interactions between the cavity shedding frequency and vortex shedding frequency. The transition from partially cavitating to fully ventilated flow caused sudden and large drops in the mean hydrodynamic loads and deformations, as well as substantial reductions in the intensity of the fluctuations.

The Reynolds number and wall cooling effects on correlations between the thermodynamic variables are systematically investigated in hypersonic turbulent boundary layers by direct numerical simulations. The correlations between the thermodynamic variables and the streamwise velocity are also analysed. The Kovasznay decomposition is introduced to decompose the fluctuating density and temperature into the acoustic and entropic modes. It is found that in the strongly cooled wall cases, the travelling-wave-like alternating positive and negative structures (TAPNSs) are found in the fluctuating pressure and the acoustic modes of density and temperature, and the streaky entropic structures (SESs) are identified in the fluctuating entropy and the entropic modes of density and temperature near the wall. Furthermore, both the acoustic and the entropic modes of density and temperature give significant contributions to the correlations involving density and temperature in the near-wall region, while these correlations are almost totally contributed by the entropic modes in the far-wall region. The entropic modes of the density and temperature are almost linearly correlated with the fluctuating entropy. Therefore, the fact that the fluctuating entropy is strongly correlated with the fluctuating density and temperature far from the wall is mainly due to the dominance of the entropic modes in the fluctuating density and temperature. Moreover, the fluctuating temperature is strongly positively correlated with the fluctuating streamwise velocity near the wall in strongly cooled wall cases, which can be ascribed to the appearance of the TAPNSs and SESs.

We interpret the Taylor–Green cellular vortex model in terms of the Kolmogorov length and velocity scales, in order to study the balance between aggregation and breakup of cohesive sediment in fine-scale turbulence. One-way coupled numerical simulations, which capture the effects of cohesive, lubrication and direct contact forces on the flocculation process, reproduce the non-monotonic relationship between the equilibrium floc size and shear rate observed in previous experiments. The one-way coupled results are confirmed by select two-way coupled simulations. Intermediate shear gives rise to the largest flocs, as it promotes preferential concentration of the primary particles without generating sufficiently strong turbulent stresses to break up the emerging aggregates. We find that the optimal intermediate shear rate increases for stronger cohesion and smaller particle-to-fluid density ratios, and we propose a simple model for the equilibrium floc size that agrees well with experimental data reported in the literature.

Cilia are micro-scale hair-like organelles. They can exhibit self-sustained oscillations which play crucial roles in flow transport or locomotion. Recent studies have shown that these oscillations can spontaneously emerge from dynamic instability triggered by internal stresses via a Hopf bifurcation. However, the flow transport induced by an instability-driven cilium still remains unclear, especially when the fluid is non-Newtonian. This study aims at bridging these gaps. Specifically, the cilium is modelled as an elastic filament, and its internal actuation is represented by a constant follower force imposed at its tip. Three generalized Newtonian behaviours are considered, i.e. the shear-thinning, Newtonian and shear-thickening behaviours. Effects of four key factors, including the filament zero-stress shape, Reynolds number ($Re$), follower-force magnitude and fluid rheology, on the filament dynamics, fluid dynamics and flow transport are explored through direct numerical simulation at $Re$ of 0.04 to 5 and through a scaling analysis at $Re \approx 0$. The results reveal that even though it is expected that inertia vanishes at $Re \ll 1$, inertial forces do alter the filament dynamics and deteriorate the flow transport at $Re\ge 0.04$. Regardless of $Re$, the flow transport can be improved when the flow is shear thinning or when the follower force increases. Furthermore, a linear stability analysis is performed, and the variation of the filament beating frequency, which is closely correlated with the filament dynamics and flow transport, can be predicted.

We study the statistics of passive scalars (either temperature or concentration of a diffusing substance) at friction Reynolds number ${Re}_{\tau }=1140$, for turbulent flow within a smooth straight pipe of circular cross-section, in the range of Prandtl numbers from ${Pr}=0.00625$, to ${Pr}=16$, using direct numerical simulations (DNS) of the Navier–Stokes equations. Whereas the organization of passive scalars is similar to the axial velocity field at ${Pr} = O(1)$, similarity is impaired at low Prandtl number, at which the buffer-layer dynamics is filtered out, and at high Prandtl number, at which the passive scalar fluctuations become confined to the near-wall layer. The mean scalar profiles at ${Pr} \gtrsim 0.0125$ are found to exhibit logarithmic overlap layers, and universal parabolic distributions in the core part of the flow. Near-universality of the eddy diffusivity is exploited to derive accurate predictive formulas for the mean scalar profiles, and for the corresponding logarithmic offset function. Asymptotic scaling formulas are derived for the thickness of the conductive (diffusive) layer, for the peak scalar variance, and its production rate. The DNS data are leveraged to synthesize a modified form of the classical predictive formula of Kader & Yaglom (Intl J. Heat Mass Transfer, vol. 15, 1972, pp. 2329–2351), which is capable of accounting accurately for the dependence on both Reynolds and Prandtl numbers, for ${Pr} \gtrsim 0.25$.

We report the first shock-tube experiments on two-dimensional dual-mode air–SF$_6$ interfaces with different initial spectra subjected to a convergent shock wave. The convergent shock tube is specially designed with a tail opening to highlight the Bell–Plesset (BP) and mode-coupling effects on amplitude development of fundamental mode (FM). The results show that the BP effect promotes the occurrence of mode coupling, and the feedback of high-order modes to the FM also arises earlier in convergent geometry than that in its planar counterpart. Relatively, the amplitude growth of the FM with a higher mode number is inhibited by the feedback, and saturates earlier. The FM with a lower mode number is affected more heavily by the BP effect, and finally dominates the flow. A new model is proposed to well predict the amplitude growths of the FM and high-order modes in convergent geometry. In particular, for FM that reaches its saturation amplitude, the post-saturation relation is introduced in the model to achieve a better prediction.

Indirect noise is a significant contributor to aircraft engine noise, which needs to be minimized in the design of aircraft engines. Indirect noise is caused by the acceleration of flow inhomogeneities through a nozzle. High-fidelity simulations showed that some flow inhomogeneities can be chemically reacting when they leave the combustor and enter the nozzle (Giusti et al., Trans. ASME J. Engng Gas Turbines Power, vol. 141, issue 1, 2019). The state-of-the-art models, however, are limited to chemically non-reacting (frozen) flows. In this work, first, we propose a low-order model to predict indirect noise in nozzle flows with reacting inhomogeneities. Second, we identify the physical sources of sound, which generate indirect noise via two physical mechanisms: (i) chemical reaction generates compositional perturbations, thereby adding to compositional noise; and (ii) exothermic reaction generates entropy perturbations. Third, we numerically compute the nozzle transfer functions for different frequency ranges (Helmholtz numbers) and reaction rates (Damköhler numbers) in subsonic flows with hydrogen and methane inhomogeneities. Fourth, we extend the model to supersonic flows. We find that hydrogen inhomogeneities have a larger impact on indirect noise than methane inhomogeneities. Both the Damköhler number and the Helmholtz number markedly influence the phase and magnitude of the transmitted and reflected waves, which affect sound generation and thermoacoustic stability. This work provides a physics-based low-order model which can open new opportunities for predicting noise emissions and instabilities in aeronautical gas turbines with multi-physics flows.

We present pore-resolved compressible direct numerical simulations of turbulent flows grazing over perforated plates, that closely resemble the acoustic liners found on aircraft engines. Our direct numerical simulations explore a large parameter space including the effects of porosity, thickness and viscous-scaled diameter of the perforated plates, at friction Reynolds numbers $\textit {Re}_\tau = 500\unicode{x2013}2000$, which allows us to develop a robust theory for estimating the added drag induced by acoustic liners. We find that acoustic liners can be regarded as porous surfaces with a wall-normal permeability and that the relevant length scale characterizing their added drag is the inverse of the wall-normal Forchheimer coefficient. Unlike other types of porous surfaces featuring Darcian velocities inside the pores, the flow inside the orifices of acoustic liners is fully turbulent, with a magnitude of the wall-normal velocity fluctuations comparable to the peak in the near-wall cycle. We provide clear evidence of a fully rough regime for acoustic liners, also confirmed by the increasing relevance of pressure drag. Once the fully rough asymptote is reached, canonical acoustic liners provide an added drag comparable to that of sand-grain roughness with viscous-scaled height matching the inverse of the viscous-scaled Forchheimer permeability of the plate.

Spatial linear stability analysis is used to study the axisymmetric screech tones generated by twin converging round nozzles at low supersonic Mach numbers. Vortex-sheet and finite-thickness models allow for identification of the different waves supported by the flow at different conditions. Regions of the frequency–wavenumber domain for which the upstream-propagating guided jet modes are observed to be neutrally stable are observed to vary as a function of solution symmetry, jet separation, $S$, and the velocity profile used. Screech-frequency predictions performed using wavenumbers obtained from both models agree well with experimental data. Predictions obtained from the finite-thickness model better align with the screech tones measured experimentally and so are seen to be an improvement on predictions made with the vortex sheet. Additionally, results from the finite-thickness model predict both symmetric and antisymmetric screech tones for low $S$ that are found in the vortex-sheet model only at greater $S$. The present results indicate that the feedback loop generating these screech tones is similar to that observed for single-jet resonance, with equivalent upstream and downstream modes.

The three-dimensional flow over a low-aspect-ratio (low-$A\!R$) trapezoidal plate is investigated experimentally with a focus on how the tip effects impact the structure and dynamics of the separation bubble. The chord-based Reynolds number is $5800$, and the angle of attack varies from $4^\circ$ to $10^\circ$. Once the flow separates, the separation bubble emerges and features a swallow-tailed structure that shrinks near the midspan, which is first found for the flows over low-$A\!R$ plates. This structure develops into the conventional single-tailed structure as the angle of attack increases. Moreover, the vortex shedding within the swallow-tailed separation bubble is restored from multiple asynchronously measured local velocity fields. It is revealed that the leading-edge vortex undergoes the novel transformation from a C-shape vortex into an M-shape vortex. This vortex transformation stems from the mass transport of the near-wall spanwise flow, which affects the fluid motion on the windward side of the C-shape vortex head, strengthening and accelerating the vortex head. The strengthened vortex head facilitates the entrainment of high-momentum fluid from the outer flow. This is responsible for the formation of the swallow-tailed structure. These findings help to fill the gaps left by the downwash at low angles of attack for low-$A\!R$ wings, and are of value in improving the cruising and gliding performance of micro-air vehicles.

Two kinetic models are proposed for high-temperature rarefied (or non-equilibrium) gas flows with internal degrees of freedom and radiation. One of the models uses the Boltzmann collision operator to model the translational motion of gas molecules, which has the ability to capture the influence of intermolecular potentials, while the other adopts the relaxation time approximations, which has higher computational efficiency. In our kinetic model equations, not only the transport coefficients such as the shear/bulk viscosity and thermal conductivity but also their underlying relaxation processes are recovered. The non-equilibrium dynamics of gas flow and radiation are tightly coupled, where the transport properties of gas molecules and photons are correlatively dependent. The proposed kinetic models are validated by the direct simulation Monte Carlo method in several non-radiative rarefied gas flows (e.g. the normal shock wave, Fourier flow, Couette flow and the creep flow driven by the Maxwell demon), and the experimental data of planar heat transfer and normal shock waves in nitrogen. Then, the rarefied gas flows with strong radiation are studied based on the kinetic models, not only in the above one-dimensional gas flows, but also in the two-dimensional radiative hypersonic flow passing a cylinder. The characteristics of heat transfer in the tightly coupled fields of gas and radiation are systematically investigated, particularly the influence of the non-equilibrium photon transport and their interactions with gas molecules are revealed. It is found that the radiation makes a profound contribution to the total heat transfer in radiative hypersonic flow at an intermediate photon Knudsen number.

The 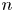$n$th-order velocity structure function 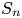$S_n$ in homogeneous isotropic turbulence is usually represented by $S_n \sim r^{\zeta _n}$, where the spatial separation 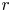$r$ lies within the inertial range. The first prediction for 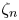$\zeta _n$ (i.e. 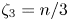$\zeta _3=n/3$) was proposed by Kolmogorov (Dokl. Akad. Nauk SSSR, vol. 30, 1941) using a dimensional argument. Subsequently, starting with Kolmogorov (J. Fluid Mech., vol. 13, 1962, pp. 82–85), models for the intermittency of the turbulent energy dissipation have predicted values of 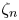$\zeta _n$ that, except for 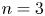$n=3$, differ from $n/3$. In order to assess differences between predictions of 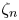$\zeta _n$, we use the Hölder inequality to derive exact relations, denoted plausibility constraints. We first derive the constraint $(p_3-p_1)\zeta _{2p_2} = (p_3 -p_2)\zeta _{2p_1} +(p_2-p_1)\zeta _{2p_3}$ between the exponents 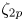$\zeta _{2p}$, where 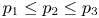$p_1 \leq p_2 \leq p_3$ are any three positive numbers. It is further shown that this relation leads to 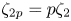$\zeta _{2p} = p \zeta _2$. It is also shown that the relation 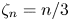$\zeta _n=n/3$, which complies with 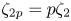$\zeta _{2p} = p \zeta _2$, can be derived from constraints imposed on 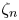$\zeta _n$ using the Cauchy–Schwarz inequality, a special case of the Hölder inequality. These results show that while the intermittency of 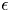$\epsilon$, which is not ignored in the present analysis, is not incompatible with the plausible relation 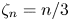$\zeta _n=n/3$, the prediction 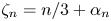$\zeta _n=n/3 +\alpha _n$ is not plausible, unless 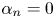$\alpha _n =0$.

In this work, we investigate the characteristics of wind turbine wakes for three different blade designs (i.e. the NREL-Ori, NREL-Root and NREL-Tip designs, where the NREL-Ori refers to the baseline offshore 5 MW wind turbine designed by the US National Renewable Energy Laboratory) under turbulent inflows using large-eddy simulations with the actuator surface model. The load on the blade is higher near the blade root/tip for the NREL-Root/NREL-Tip designs when compared with the NREL-Ori design, while their thrust coefficients are the same. The results show that the blade designs influence the velocity deficit in the near wake, turbulence kinetic energy and wake meandering (both amplitude and frequency). In the near-wake region, the magnitude of the velocity deficit from the NREL-Root design is higher. As for the turbulence kinetic energy, its maximum in the near wake is higher for the NREL-Tip design, while in the far wake, it is higher for the NREL-Root design. Analyses of the instantaneous spanwise wake centre positions show higher meandering amplitude for the NREL-Root design, with the magnitudes of the low-frequency components approximately the same as the other two designs under the same inflow. The dominant meandering frequencies from different designs are different, with lower values for the NREL-Root design for which the vortex structures near the hub of low frequency play leading roles, and higher values for the NREL-Tip design for which the flow structures of high frequency in the tip shear layer are more important.

The perturbations existing on a breaking wavefront can be a potential explanation for the slamming pressure variability in wave impacts. Here, we investigate the effect of these perturbations by forced vertical slamming of a two-dimensional circular cylinder with constant downward velocity on standing waves. Through experimental modelling and numerical simulation, the slamming force is measured for several standing wave amplitudes and wavelengths. The standing wave phase is tuned such that the impact occurs symmetrically at the instant of maximum crest or trough. Our observations show that slamming coefficients vary with the standing wave amplitude when the wavelength is kept constant and vice versa. The trough impact slamming coefficient can be more than two times the flat impact, and up to four times the crest impact. The experimental results are reproduced by numerical simulations and they agree reasonably well in general. Two analytical approaches based on the von Kármán (NACA, vol. 321, 1929, pp. 1–8) and Wagner (Z. Angew. Math. Mech., vol. 12, 1932, pp. 913–215) methods, which consider the effect of water surface curvature, are introduced. The slamming coefficient calculated from these methods can provide a bound in which the slamming coefficient can be found for each standing wave amplitude and wavelength. Further insight is achieved by numerical simulations of impact on the shorter wavelength to diameter ratio of $0.05<\lambda /D<0.4$. As the wavelength to diameter ratio becomes smaller, the cylinder impacts the water surface at several locations. As a result, multiple peaks occur, and the trapped air at different locations between the cylinder and the water surface yields oscillations with different frequencies on the slamming coefficient time history.

The wall dependence of length scales used to describe large- and small-scale structures of turbulence is examined using highly resolved experiments in zero-pressure-gradient turbulent boundary layers and pipe flows spanning the range $2000< Re_\tau <37\ 700$. Of particular interest is the influence of external intermittency on the scaling of these length scales. It is found that when suitable scaling parameters are selected and external intermittency is accounted for, the dissipative motions follow inner scaling even into the outer-scaled regions of the flow, and that certain large-scale descriptions follow outer scaling even in the inner-scaled regions of the flow. The wall dependence is the same for both internal pipe and external boundary layer flows, and the different length scales can be related to recognizable features in the longitudinal wavenumber spectrum.

Modelling the noise emitted by turbulent jets is made difficult by their acoustic inefficiency: only a tiny fraction of the near-field turbulent kinetic energy is propagated to the far field as acoustic waves. As a result, jet-noise models must accurately capture this small, acoustically efficient component hidden among comparatively inefficient fluctuations. In this paper, we identify this acoustically efficient near-field source from large-eddy simulation data and use it to inform a predictive model. Our approach uses the resolvent framework, in which the source takes the form of nonlinear fluctuation terms that act as a forcing on the linearised Navier–Stokes equations. First, we identify the forcing that, when acted on by the resolvent operator, produces the leading spectral proper orthogonal decomposition modes in the acoustic field for a Mach 0.4 jet. Second, the radiating components of this forcing are isolated by retaining only portions with a supersonic phase speed. This component makes up less than 0.05 % of the total forcing energy but generates most of the acoustic response, especially at peak (downstream) radiation angles. Finally, we propose an empirical model for the identified acoustically efficient forcing components. The model is tested at other Mach numbers and flight-stream conditions and predicts noise within 2 dB accuracy for a range of frequencies, downstream angles and flight conditions.

The paper presents a Fluid Structure Interaction (FSI) method for hydroelastic water entry. The method assumes that the momentum exchange between the fluid and solid body can be used for the calculation of pressure, deformation and stresses arising during impact. The flexible fluid–structure interactions of flat plates entering water are solved using a computational code that employs the finite volume method to discretise both fluid and solid equations. This provides a better matching of momentum on the fluid–solid interface. The momentum arising in the solid body that emerges after the impact is defined as the momentum exchange, and is shown to increase linearly under the increase of non-dimensional impact speed. The ratio of the maximum pressure arising in an elastic body entering water to that of a rigid body is termed relative pressure and is shown to decrease linearly as a function of momentum exchange. The latter verifies the main hypothesis of this paper, namely that ‘the pressure acting on an elastic body can be predicted using an unsophisticated equation that uses the momentum exchange.’ The deformation and stresses arising in elastic plates entering water are demonstrated to be functions of momentum exchange and can be found using simple equations formulated via parametrisation of data. It is concluded that subject to further validation, the method could be extended for the prediction of hydroelastic response of other sections/bodies entering water.