2.1. Definition of the present shock reflection problem

The shock reflection problem that we consider is displayed schematically in figure 3(a). A wedge of angle $\theta _{w}$ is immersed in an initially steady supersonic flow with Mach number $M_{0}$ and produces an SOSW with shock angle $\beta _{w}$. A rightward moving normal shock starting at the inlet and moving at constant speed $\phi$ impinges the oblique shock wave to produce a shock reflection between a moving shock wave and an oblique shock.

Figure 3. Reflection between a right-going incident shock wave and an SOSW attached to a sharp wedge: (a) initial state; (b) a typical moment.

The left status of this RMS will be denoted with subscript $l$, and the flow parameters in region $(0)$ will be denoted with subscript $r$. In this paper we consider only the case where $M_{l}>1$, i.e. the inlet remains supersonic behind the RMS. The speed of the shock may also be measured with the shock moving Mach number $M_{s}=\phi /a_{r}$, where $a_{r}$ is the sound speed in region $(0)$.

As shown in figure 3(b), that part of the RMS above the intersection point $P$ of the RMS and the SOSW will be denoted shock $I_{S}$, meaning the incident shock wave. At reflection, the unperturbed part of the SOSW (downstream of $P$) will be denoted shock $S_{OR}$, with the subscript $OR$ meaning the original SOSW. The oblique shock wave newly created with new inflow condition updated by the RMS will be denoted $S_{NE}$.

Both first and second families are considered for the RMS. For an RMS of the first family, one has $p_{r}>p_{l}$, and in the frame co-moving with the RMS, the flow stream is towards the right-hand side. For an RMS of the second family, one has $p_{r}< p_{l}$, and in the frame co-moving with the RMS, the flow stream is towards the left-hand side. Reflection with an RMS of the first family may occur when one supersonic object penetrates the shock wave of another supersonic object that has a smaller speed and is upstream initially. Reflection with an RMS of the second family may occur when two supersonic objects move in the opposite direction, with one penetrating the shock wave of another. In this paper, we consider only the case that the penetrating object (here simplified as a wedge) penetrates perpendicularly to the shock wave of the other.

We will work with a reference frame co-moving with the intersection point $P$. It is obvious that the intersection point $P$ has velocity

(2.1)\begin{equation} \boldsymbol{V}_{P}=(u_{P}, v_{P})=(M_{s}a_{r}, M_{s}a_{r}\tan \beta_{w}), \end{equation}

where $\beta _{w}$ is the shock angle of the undisturbed (original) SOSW.

In the following, we will need repeatedly the oblique shock wave relations, which will, for brevity, be abbreviated as

(2.2a–c)\begin{equation} M_{d}^{2}={f_{M}}(M_{u},\beta_{ud}),\quad p_{d}=p_{u}\,{f_{p}}(M_{u}, \beta_{ud} ),\quad \rho_{d}=\rho_{u}\,{f_{\rho}}(M_{u},\beta_{ud}), \end{equation}

where

(2.3)\begin{equation} \left. \begin{aligned} {f_{M}}(M,\beta) & =\dfrac{{{M^{2}}+\dfrac{2}{{\gamma-1}}}}{{\dfrac{{2\gamma} }{{\gamma-1}}\,{M^{2}}{\sin^{2}}\beta-1}}+\dfrac{{{M^{2}}{\cos^{2}}\beta} }{{\dfrac{{\gamma-1}}{2}{M^{2}}\,{\sin^{2}}\beta+1}},\\ {f_{p}}(M,\beta) & =1+\dfrac{{2\gamma}}{{\gamma+1}}\left( {{{(M\sin \beta)}^{2} }-1}\right),\\ {f_{\rho}}(M,\beta) & =\dfrac{{(\gamma+1){{(M\sin \beta)}^{2}}}}{{2+(\gamma -1){{(M\sin \beta)}^{2}}}}. \end{aligned} \right\} \end{equation}

In (2.2a–c) and (2.3), the subscript $u$ denotes the flow parameters upstream of the shock wave, and $d$ downstream; $\beta _{ud}$ is the shock angle that is related to the flow deflection angle $\theta _{ud}$ by the shock angle relation

(2.4a,b)\begin{equation} \tan \theta_{ud}=f_{\theta}({M}_{u}{,\beta}_{ud}),\quad f_{\theta} ({M,\beta})=\frac{2\left( {M}^{2}\sin^{2}{\beta}-1\right) }{\left( {M}^{2}\left( \gamma+\cos{2\beta}\right) +2\right) \tan{\beta}}. \end{equation}

For a given upstream Mach number $M_{u}$ and flow deflection angle $\theta _{ud}$, there are two solutions for ${\beta }_{ud}$; the smaller one corresponds to a weak solution, and the larger one corresponds to a strong solution. There is a maximum value for the flow deflection angle $\theta =\theta ^{(max)}(M_{u})$, which is determined by ${\partial f_{\theta }({M_{u},\beta }_{ud})}/{\partial {\beta }_{ud}}=0$. The expression for the detached angle is

(2.5)\begin{equation} \left. \begin{aligned} \sin^{2}\beta_{m} & =\frac{1}{\gamma M_{u}^{2}} \left[\frac{\gamma+1}{4}\,M_{u} ^{2}-1+\sqrt{(1+\gamma)\left(1+\frac{\gamma-1}{2}\,M_{u}^{2}+\frac{\gamma+1}{16}\, M_{u}^{4}\right)}\right],\\ \tan \theta^{(max)} & =\frac{2[(M_{u}^{2}-1)\tan^{2}\beta_{m}-1]}{\tan \beta _{m}\,[(\gamma M_{u}^{2}+2)(1+\tan^{2}\beta_{m})+M_{u}(1-\tan^{2}\beta_{m})]}. \end{aligned} \right\} \end{equation}

For a given $M_{u}$, there is a flow deflection angle at which the downstream shock wave has sonic flow (i.e. $M_{d}=1$), beyond which the shock is a strong shock (with $M_{d}<1$), compared to weak shock (with $M_{d}>1$) for a smaller flow deflection angle.

2.2. Shock relations for RMSs of the first and second families

For RMSs of both families, the flow parameters $M_{l},u_{l},\rho _{l},p_{l}$ on the left of an RMS can be related to the flow parameters $M_{r},u_{r},\rho _{r},p_{r}$ on the right of the RMS and the shock speed $\phi$ or the shock Mach number $M_{s}$.

For an RMS of the first family, $p_{l}< p_{r}$ and we have (Ben-Dor et al. Reference Ben-Dor, Igra, Elperin and Lifshitz2001)

(2.6)\begin{equation} \phi=u_{r}-a_{r}\sqrt{\frac{\gamma+1}{2\gamma}\,\frac{p_{l}}{p_{r}}+\frac {\gamma-1}{2\gamma}} \end{equation}

and

(2.7)\begin{equation} {u_{l}}={u_{r}}-\frac{{{a_{r}}}}{\gamma}\left( {\frac{{{p_{l}}}}{{{p_{r}}} }-1}\right) {\left( {\frac{{\gamma+1}}{{2\gamma}}\,\frac{{{p_{l}}}}{{{p_{r}}} }+\frac{{\gamma-1}}{{2\gamma}}}\right) ^{-{1}/{2}}}. \end{equation}

For an RMS of the second family, $p_{r}< p_{l}$ and we have (Ben-Dor et al. Reference Ben-Dor, Igra, Elperin and Lifshitz2001)

(2.8)\begin{equation} \phi=u_{r}+a_{r}\sqrt{\frac{\gamma+1}{2\gamma}\,\frac{p_{l}}{p_{r}}+\frac {\gamma-1}{2\gamma}} \end{equation}

and

(2.9)\begin{equation} {u_{r}}={u_{l}}+\frac{{{a_{l}}}}{\gamma}\left( {\frac{{{p_{r}}}}{{{p_{l}}} }-1}\right) {\left( {\frac{{\gamma+1}}{{2\gamma}}\,\frac{{{p_{r}}}}{{{p_{l}}} }+\frac{{\gamma-1}}{{2\gamma}}}\right) ^{-{1}/{2}}}. \end{equation}

Both (2.6) and (2.8) can be used to express the pressure ratio as a function of the Mach numbers $M_{r}$ and $M_{s}$:

(2.10)\begin{equation} \frac{{{p_{l}}}}{{{p_{r}}}}=\psi, \end{equation}

where

(2.11)\begin{equation} \psi=\frac{2\gamma(M_{r}-M_{s})^{2}-(\gamma-1)}{\gamma+1}. \end{equation}

The shock relation for density and the sound speed expression can be used to give

(2.12a,b)\begin{equation} \frac{\rho_{l}}{\rho_{{{_{r}}}}}=\frac{{1+\dfrac{{\gamma+1}}{{\gamma-1}}}\,\psi }{\psi+\dfrac{{\gamma+1}}{{\gamma-1}}},\quad \frac{a_{l}}{a_{r}}=\sqrt {\psi\, \frac{\psi+\dfrac{{\gamma+1}}{{\gamma-1}}}{{1+\dfrac{{\gamma+1}} {{\gamma-1}}}\,\psi}}. \end{equation}

Insert (2.7) for $u_{l}$ and (2.12a,b) for $a_{l}$ into ${M_{l} =}u_{l}/a_{l}$, and using (2.10) to replace $p_{l}/p_{r}$ by $\psi$, we get, for an RMS of the first family,

(2.13)\begin{equation} {M_{l}}=\dfrac{{M_{r}}-\dfrac{{1}}{\gamma}\,( \psi-1) {\left( {\dfrac{{\gamma+1}}{{2\gamma}}\,\psi+\dfrac{{\gamma-1}}{{2\gamma}}}\right) ^{-{1}/{2}}}}{\sqrt{\psi\, \dfrac{\psi+\dfrac{{\gamma+1}}{{\gamma-1}} }{{1+\dfrac{{\gamma+1}}{{\gamma-1}}}\,\psi}}}. \end{equation}

Similarly, for an RMS of the second family, we have

(2.14)\begin{equation} {M_{l}}=\dfrac{{\left(\dfrac{{\gamma+1}}{2}-\dfrac{{M}_{s}}{{M}_{r}}\right)\dfrac{{1}}{\psi }+\left(\dfrac{{\gamma-1}}{2}+\dfrac{{M}_{s}}{{M}_{r}}\right)}}{{\gamma\left(\dfrac{{M}_{s}} {{M}_{r}}-1\right)\sqrt{\dfrac{{\gamma+1}}{{2\gamma}}\,\dfrac{{1}}{\psi}+\dfrac{{\gamma-1}}{{2\gamma}}}}}. \end{equation}

2.3. Properties of RMSs of both families

Now we display how $M_{l}$ and $p_{l}/p_{r}$ vary with respect to $M_{r}$ or $M_{s}$. The variation of the Mach number $M_{l}$ with respect to $M_{r}$ and $M_{s}$ is computed by (2.13) and is displayed in figures 4(a) for $M_{s}=5$ and 4(b) for $M_{s}=30$.

Figure 4. The Mach number $M_{l}$ as a function of $M_{r}$: (a) $M_{s}=5$; (b) $M_{s}=30$.

For an RMS of the first family, ${M_{l}\rightarrow \infty }$ when $\psi =0$, according to (2.13). By (2.11), $\psi =0$ means $M_{r} =M_{s}+\sqrt {(\gamma -1)/(2\gamma )}$. If $M_{s}=5$, then $M_{r}=5.378$, which is the abscissa of point $c$ in figure 4(a). The value of $M_{l}$ towards the point $c$ is infinite. The condition $p_{l}=p_{r}$ occurs when $\psi =1$. If $M_{s}=5$, then $M_{r}=6$, which is the abscissa of point $d$ in figure 4(a). Thus $M_{r}$ has a finite range of values for an RMS of the first family, and we have $5.378< M_{r}<6$ for $M_{s}=5$. If $M_{s}=30$, then $M_{r}=30.378$, which is the abscissa of point $c$ in figure 4(b). The value of $M_{l}$ towards the point $c$ is infinite. The condition $p_{l}=p_{r}$ occurs when $\psi =1$. If $M_{s}=30$, then $M_{r}=31$, which is the abscissa of point $d$ in figure 4(b). Thus $M_{r}$ has a finite range of values for an RMS of the first family, and we have $30.378< M_{r}<31$ for $M_{s}=30$.

For an RMS of the second family, $M_{r}$ also has a finite range of values. The point $a$ in figure 4(a) corresponds to $M_{r}=1$, at which ${M_{l} =2.05}$ according to (2.14). The point $b$ corresponds to $p_{l}=p_{r}$, i.e. $\psi =1$. With $\psi =1$, (2.11) gives $M_{r}=M_{s}-1=4$ for $M_{s}=5$. The point $a$ in figure 4(b) corresponds to $M_{r}=1$, at which ${M_{l}=1.96}$ according to (2.14). The point $b$ corresponds to $p_{l}=p_{r}$, i.e. $\psi =1$. With $\psi =1$, (2.11) gives $M_{r} =M_{s}-1=29$ for $M_{s}=30$.

In figure 5(a), the variance of $M_{l}$ with the change of $M_{s}$ for $M_{r}=6$ is displayed. For an RMS of the first family, ${M_{l}\rightarrow \infty }$ when $\psi =0$, according to (2.13). By (2.11), $\psi =0$ means $M_{s}=M_{r}-\sqrt {(\gamma -1)/(2\gamma )}$. If $M_{r}=6$, then $M_{s}=5.622$, which is the abscissa of point $b$ in figure 5(a). The value of $M_{l}$ towards the point $b$ is infinite. The condition $p_{l}=p_{r}$ occurs when $\psi =1$. If $M_{r}=6$, then (2.11) gives $M_{s}=5$, which is the abscissa of point $a$ in figure 5(a). Thus $M_{s}$ has a finite range of values for an RMS of the first family, and we have $5< M_{s}<5.622$ for $M_{r}=6$. For an RMS of the second family, the point $d$ in figure 4(a) corresponds to $M_{s}=30$, at which ${M_{l}=}2.443$ according to (2.14). The point $c$ corresponds to $p_{l}=p_{r}$, i.e. $\psi =1$. With $\psi =1$, (2.11) gives $M_{s}=M_{r}+1=7$ for $M_{r}=6$.

Figure 5. (a) The Mach number $M_{l}$ as a function of $M_{s}$ with $M_{r}=6$; (b) $p_{l}/p_{r}$ as a function of $M_{s}$ with $M_{r}=6$.

In figure 5(b), the variance of $p_{l}/p_{r}$ (or $\psi$) with the change of $M_{s}$ for $M_{r}=6$ is displayed. For an RMS of the first family, by (2.11), $\psi =0$ means $M_{s}=M_{r}-\sqrt {(\gamma -1)/(2\gamma )}$. If $M_{r}=6$, then $M_{s}=5.622$, which is the abscissa of point $b$ in figure 5(b). The condition $p_{l}=p_{r}$ occurs when $\psi =1$. If $M_{r}=6$, then (2.11) gives $M_{s}=M_{r}-1=5$, which is the abscissa of point $a$ in figure 5(b). Thus $M_{s}$ has a finite range of values for an RMS of the first family, and we have $5< M_{s}<5.622$ for $M_{r}=6$. For an RMS of the second family, the point $d$ in figure 5(b) corresponds to $M_{s}=7.648$, at which ${M_{l}=}5.761$ according to (2.14). The point $c$ corresponds to $p_{l}=p_{r}$, i.e. $\psi =1$. With $\psi =1$, (2.11) gives $M_{s}=M_{r}+1=7$ for $M_{r}=6$.

2.4. Numerical method

Computational fluid dynamics (CFD) will be used to display shock reflection patterns for specific input conditions. The compressible Euler equations of an ideal gas are solved using the second-order implicit advection upstream splitting method (AUSM) (Liu Reference Liu1996). The computational domain and the boundary conditions are shown in figure 6. The lower boundary is a wedge with wedge angle $\theta _{w}$.

Figure 6. Domain of computation and boundary condition for the numerical simulation.

First we compute a steady supersonic flow with inflow Mach number $M_{0}$. This will give a simple supersonic flow with an oblique shock wave with shock angle $\beta _{w}$. Then, at the inlet upstream of the wedge, we set an RMS moving at a given $M_{s}$. The original steady-state solutions are now considered as the right-hand state of this RMS. The left-hand side flow parameters $M_{l}$, $p_{l}$ and $\rho _{l}$ of this RMS are obtained from the expressions (2.10)–(2.13).

To test the accuracy with a different choice of grid density, we consider an RMS of the first family with $\theta _{w}=25^{\circ },M_{s}=2.45$ and $M_{r}=3$. Three grids are tested. The coarser grid has $250\times 250$ cells, the middle grid has $500\times 500$ cells, and the finest grid has $1000\times 1000$ cells. The Mach contours at the same typical instant are displayed in figure 7 for the three grids. The shock wave patterns calculated with the middle and finest grids have little difference, as shown in figure 7, so the finest grid is used for the subsequent numerical simulations.

Figure 7. Mach contours with different meshes: (a) $250\times 250$ cells, (b) $500\times 500$ cells, (c) $1000\times 1000$ cells.

2.5. Solution and identification of steady and moving triple points

The problem studied in this paper may display a shock reflection configuration with several moving triple points. The typical flow structure for a triple point configuration is shown in figure 8, where we distinguish between an upward triple point and a downward triple point. It involves an incident shock (labelled $i$), a reflected shock (labelled $r$), a Mach stem (labelled $m$) and a slipline (labelled $s$). The flow in the vicinity of a steady triple point is determined by the von Neumann triple point theory: the flow properties in the adjacent regions (regions (0)–(3) in figure 8) are connected by oblique shock wave relations with an assumed slipline angle $\theta _{s}$, and this slipline angle is finally determined by the pressure balance condition ($p_{3}=p_{2}$) and flow parallel condition ($\theta _{03}=\theta _{s}$, $\theta _{02}=\theta _{01}-\theta _{s}$). The pressure balance condition states that the pressure behind the reflected shock wave is balanced with that behind the Mach stem; the flow parallel condition states that the flow streams across the slipline are parallel.

Figure 8. A triple point structure: (a) upward triple point; (b) downward triple point.

In the following, we need an efficient method to identify a moving triple point structure through numerical solution of unsteady flow, i.e. to identify which of the shock waves of a triple point are the incident one, the reflected one and the Mach stem, from numerical solutions. For this, we will use the triple point structure identification (TPSI) method of Wang & Wu (Reference Wang and Wu2021); see the Appendix for how to use it.

3.1. A shock reflection pattern that can be interpreted as type V shock interference

We compute here an initially steady supersonic flow with $M_{0}=6$ and $\theta _{w}=30^{\circ }$. This will give an SOSW. Then, at the inlet, we set an RMS of the first family moving at $M_{s}=5.498$. The original flow parameters in region $(0)$ are now considered as the right-hand state of this RMS. The left-hand side flow parameters of this RMS are set to $M_{l}=10.889$ (obtained from (2.13) for the first family), $p_{l}=0.127p_{r}$ and $\rho _{l}=0.288\rho _{r}$ (obtained from (2.10)–(2.12a,b)).

The Mach contours at some typical instant where the major reflection structure is well formed are displayed in figure 9(a). The shock reflection pattern, with typical triple point structure illustrated, is displayed in figure 9(b). The shock reflection pattern displayed in figure 9(b) has five triple points (labelled $Tp_{1}$, $Tp_{2}$, $Tp_{3}$, $Tp_{4}$, $Tp_{5}$), identified using the TPSI method given in Appendix. The incident shock, reflected shock, Mach stem and slipline are marked with $i$, $r$, $m$ and $S$, with subscripts $1,2,3,4,5$ for the corresponding triple points. For instance, $i_{1}$, $r_{1}$, $m_{1}$, $S_{1}$ mark, respectively, the incident shock, reflected shock, Mach stem and slipline of triple point $Tp_{1}$. Note that this flow structure is similar to the reflection pattern of that given by Wang & Wu (Reference Wang and Wu2021) using $M_{0}=6$, $\theta _{w}=22.5^{\circ }$ and $M_{s}=5.58$. This similarity means that the five triple points structure may occur at close but different conditions. The schematic display in figure 9(b) has more details than that of Wang & Wu (Reference Wang and Wu2021): we display here streamlines for each triple (these streamlines are based on the flow relative to the frame co-moving with that triple point). The direction of a streamline indicates how pressure changes across any shock wave. The pressure downstream of a shock is larger than that upstream. Consider, for instance, triple point $Tp_{3}$. For an RMS of the first family, $p_{l}< p_{r}$ according to figure 5(b), thus one streamline relative to triple point $Tp_{3}$ points from region $(r)$ to region $(l)$ when crossing the RMS (shock ‘$I_{s}$’ in figure 9b) of figure 3(a).

Figure 9. Shock reflection patterns: (a) Mach contours; (b) shock pattern.

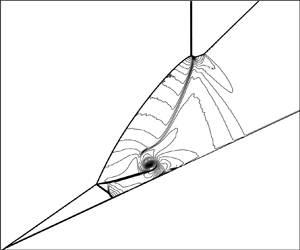
The five triple points structure displayed in figure 9(b) is in fact a type V shock interference, which occurs in Edney's shock interaction problem (Edney Reference Edney1968) and in the double wedge shock reflection problem (cf. Olejniczak et al. Reference Olejniczak, Wright and Candler1997; Hu et al. Reference Hu, Gao, Myong, Dou and Khoo2010; Xiong et al. Reference Xiong, Li, Zhu and Luo2018); see figure 10 for an illustration of type V shock interference produced by double wedge reflection.

Figure 10. Schematic illustration of the double wedge reflection of type V.

The switch of the five triple points structure shown in figure 9(b) to type V shock interference is shown schematically in figure 11. The flow is measured in the reference frame co-moving with the intersection point $P$ of shock $I_{S}$ and shock $S_{OR}$ (see figure 3b). The picture of the original flow structure shown in figure 11(a) is flipped upside down to become the picture shown in figure 11(b), which is further rotated in the clockwise direction to become the picture shown in figure 11(c).

Figure 11. Schematic illustration of the procedure to switch to type V shock interference.

The establishment of equivalence between the original problem and a shock interaction problem allows us to anticipate more shock reflection patterns for other sets of input parameters, as will be discussed in § 3.2. It is well-known that type V shock interference is caused by the interaction of two incident steady shock waves from the same family (Keyes & Hains Reference Keyes and Hains1973; Grasso et al. Reference Grasso, Purpura and Délery2003). The switch of the observed shock reflection pattern of the present problem, as shown in figure 9(b), to the type V shock interference means that the RMS of the first family ($I_{s}$) and the initially steady-state shock wave ($S_{OR}$) constitute two incident shock waves of the same family when the reference frame co-moving with the intersection point $P$ of $I_{S}$ and $S_{OR}$ is used.

It is also well-known that the interaction of two shock waves of the same family may also lead to type VI and type IV shock interference (Keyes & Hains Reference Keyes and Hains1973; Grasso et al. Reference Grasso, Purpura and Délery2003). By switching between the original problem (present shock reflection with an RMS of the first family) and the equivalent problem (steady shock interaction problem), as illustrated in figure 12, we anticipate that the present shock reflection problem may produce a shock reflection pattern other than the type V shock reflection pattern, i.e. we may also have type VI and type IV shock reflection patterns as shown in figure 12(a). However, as we will see in § 3.4, the problem is even more complex than this.

Figure 12. Correspondence between possible shock reflection patterns of the original problem (a) and possible shock interference patterns of the equivalent problem (b).

3.2. Link of parameters between the original problem and the equivalent problem: flow deflection angle reversal

To anticipate the shock reflection patterns of the original problem (reflection between the RMS and the SOSW with input parameters $M_{s}$, $M_{r}$ and $\theta _{w}$) using the knowledge of the equivalent problem (steady shock interaction between two incident shock waves with input parameters $\theta _{1}$, $\theta _{2}$ and $M$), we need to make a link between the input parameters of the present problem and those of the equivalent problem. The parameters used to establish such a link are labelled in figure 13. The link is obtained directly by using the frame co-moving with the intersection point $P$ of $I_{S}$ and $S_{OR}$. Here, $\theta _{1}$ is the flow deflection angle across the first incident shock wave $I_{S}$ (or the first wedge angle as shown in figure 10), and $\theta _{2}$ is that across the second incident shock wave $S_{OR}$ and measured with respect to the free-stream flow direction (or the second wedge angle as shown in figure 10). The difference

(3.1)\begin{equation} \Delta \theta=\theta_{2}-\theta_{1} \end{equation}

measures the flow deflection angle across the shock wave $S_{OR}$. Now we provide the expressions for the link between $\theta _{1}$, $\theta _{2}$, $M$ and $M_{s}$, $M_{r}$, $\theta _{w}$. The parameters $\theta _{1}$, $\theta _{2}$, $M$ follow from $M_{s}$, $M_{r}$, $\theta _{w}$ by switching flow parameters from the ground frame to the frame co-moving with the intersection point $P$.

Figure 13. Flow parameters in the frame co-moving with the intersection point $P$ of $I_{S}$ and $S_{OR}$ for an RMS of the first family. Only type V shock interference is considered.

On the ground frame, the Mach number $M_{d}$ and speed of sound $a_{d}$ in zone $(d)$ (i.e. downstream of the steady shock wave $S_{OR}$) follow from the steady shock wave relation of $S_{OR}$. The flow velocities $(u_{d},v_{d})$ of zone $(d)$ in the ground frame are given by $u_{d}=M_{d}a_{d}\cos \theta _{w}$, $v_{d}=M_{d}a_{d}\sin \theta _{w}$. In regions $(r)$ and $(l)$, the velocities are $u_{r}=M_{r}a_{r}$ and $u_{l}=M_{l}a_{l}$, where $M_{r}$ and $M_{l}$ are given input flow parameters.

Now we derive the flow parameters in the frame co-moving with $P$. The superscript $(P)$ is used to denote flow parameters in this co-moving frame. The velocity of the intersection point $P$ of $I_{S}$ and $S_{OR}$ is given by (2.1). Thus the flow velocities of region $(r)$, region $(l)$ and region $(d)$ in the co-moving frame are

(3.2)\begin{equation} \left. \begin{aligned} & (u_{r}^{(P)},v_{r}^{(P)})=(u_{r}-u_{P},-v_{P})\quad \text{region }(r),\\ & (u_{l}^{(P)},v_{l}^{(P)})=(u_{l}-u_{P},-v_{P})\quad \text{region }(l),\\ & (u_{d}^{(P)},v_{d}^{(P)})=(u_{d}-u_{P},v_{d}-v_{P})\quad \text{region }(d). \end{aligned} \right\} \end{equation}

Putting (3.2) into $M_{l}^{(P)}=\sqrt {(u_{l}^{(P)})^{2}+(v_{l}^{(P)})^{2} }/a_{l}$ and $\theta _{l}^{(P)}=\arctan |v_{l}^{(P)}/u_{l}^{(P)}|$, and using the definition of the Mach number, we get the following expressions for the Mach number $M_{l}^{(P)}$ and flow deflection angle $\theta _{l}^{(P)}$ in region $(l)$:

(3.3)\begin{equation} \left. \begin{aligned} M_{l}^{(P)} & =\frac{\sqrt{(M_{l}a_{l}-M_{s}a_{r})^{2}+(M_{s}a_{r}\tan \beta _{w})^{2}}}{a_{l}},\\ \theta_{l}^{(P)} & =\arctan\left(\left|\frac{M_{s}a_{r}\tan \beta_{w}}{M_{l}a_{l}-M_{s} a_{r}}\right|\right). \end{aligned} \right\} \end{equation}

Putting (3.2) into $\theta _{r}^{(P)}=\arctan |v_{r}^{(P)}/u_{r}^{(P)}|$, we get the following expressions for the flow deflection angle $\theta _{r}^{(P)}$ in region $(r)$:

(3.4)\begin{equation} \theta_{r}^{(P)}=\arctan\left(\left|\frac{M_{s}\tan \beta_{w}}{M_{s}-M_{r}}\right|\right). \end{equation}

The flow deflection angle $\theta _{d}^{(P)}$ in region $(d)$ is then computed by

(3.5)\begin{equation} \theta_{d}^{(P)}=\begin{cases} \arctan\left|\dfrac{v_{d}^{(P)}}{u_{d}^{(P)}}\right|, & \text{if } u_{d}^{(P)}>0, v_{d}^{(P)}<0,\\ {\rm \pi}-\arctan\left|\dfrac{v_{d}^{(P)}}{u_{d}^{(P)}}\right|, & \text{if } u_{d}^{(P)}<0, v_{d}^{(P)}<0,\\ {\rm \pi}+\arctan\left|\dfrac{v_{d}^{(P)}}{u_{d}^{(P)}}\right|, & \text{if }u_{d}^{(P)}<0, v_{d}^{(P)}>0,\\ 2{\rm \pi}-\arctan\left|\dfrac{v_{d}^{(P)}}{u_{d}^{(P)}}\right|, & \text{if }u_{d}^{(P)}>0, v_{d}^{(P)}>0. \end{cases} \end{equation}

The expression (3.2) is used to compute the right-hand sides of the above expressions.

The corresponding parameters $\theta _{1}$, $\theta _{2}$ and $M$ in the equivalent problem, as shown in figure 13, can thus be expressed as

(3.6)\begin{equation} \left. \begin{aligned} \theta_{1} & =\theta_{r}^{(P)}-\theta_{l}^{(P)},\\ \theta_{2} & =\theta_{d}^{(P)}-\theta_{l}^{(P)},\\ M & =M_{l}^{(P)}. \end{aligned} \right\} \end{equation}

To identify the co-moving flow field, apart from parameters $\theta _{1}$, $\theta _{2}$ and $M$, the Mach number in the co-moving frame with point $P$ in region $(r)$ and region $(d)$, denoted $M_{1}$ (or $M_{r}^{(P)}$) and $M_{2}$ (or $M_{d}^{(P)}$) are also needed. With the required flow velocity components given by (3.2), the Mach numbers $M_{1}$ (or $M_{r}^{(P)}$) and $M_{2}$ (or $M_{d}^{(P)}$) in the equivalent problem are obtained as

(3.7)\begin{equation} \left. \begin{aligned} M_{1} & =M_{r}^{(P)}=\frac{\sqrt{(u_{r}^{(P)})^{2}+(v_{r}^{(P)})^{2}}}{a_{r}},\\ M_{2} & =M_{d}^{(P)}=\frac{\sqrt{(u_{d}^{(P)})^{2}+(v_{d}^{(P)})^{2}}}{a_{d}}. \end{aligned} \right\} \end{equation}

For a given set of $M_{s}$, $M_{r}$ and $\theta _{w}$, (3.6) provides the final set of relations to find the input parameters $\theta _{1}$, $\theta _{2}$ and $M$ of the equivalent shock interaction problem, and (3.7) provides the final set of relations to find Mach numbers $M_{1}$ and $M_{2}$. Recall that we have defined $\Delta \theta =\theta _{1}-\theta _{2}$, which can be regarded as the flow deflection angle of the second attached shock wave of the equivalent double wedge shock reflection.

For $M_{r}=6$ and $M_{s}=5.498$, the variations of $\theta _{1}$, $\theta _{2}$ and $\Delta \theta$ for $\theta _{w}\in \lbrack 0^{\circ },40^{\circ }]$ are displayed in figure 14(a), and the variations of $M$, $M_{1}$ (or $M_{r}^{(P)}$) and $M_{2}$ (or $M_{d}^{(P)}$) for the same range of $\theta _{w}$ are displayed in figure 14(b). It is seen that, with increasing $\theta _{w}$, the angle $\theta _{1}$ decreases monotonically from $33.36^{\circ }$ to $8.19^{\circ }$, and both $M$ and $M_{1}$ increase monotonically. However, the curves for $\theta _{2}$, $\Delta \theta =\theta _{2} -\theta _{1}$ and $M_{2}$ are non-monotonic. For increasing $\theta _{w}$, they first decrease, then increase, and finally decrease. The Mach number $M_{2}$ is smaller than $M_{1}$. When $\theta _{w}\rightarrow 0$, the SOSW is an infinitely weak oblique shock wave, and the flow parameters in region $(r)$ are almost the same as those in region $(d)$.

Figure 14. Variation of parameters with respect to $\theta _{w}$ for $M_{r}=6$ and $M_{s}=5.498$: (a) variation of $\theta _{1}$, $\theta _{2}$ and $\Delta \theta$; (b) variation of $M$, $M_{1}$ and $M_{2}$.

Two important phenomena are observed. The first is that $M_{2}$ is below 1 for $\theta _{w}\in \lbrack 0^{\circ },24.18^{\circ }]$, and above 1 for $\theta _{w}\in \lbrack 24.18^{\circ },40^{\circ }]$. This defines a condition that the incident shock wave $S_{OR}$ becomes a strong one in the moving frame, which would be a condition for transition between types V and IV. In § 3.3, we indeed observe type IV shock interaction. The second phenomenon is that the difference $\Delta \theta$ is negative for $\theta _{w}\in \lbrack 0^{\circ },9.18^{\circ }]$, while it is positive for $\theta _{w}\in \lbrack 9.18^{\circ },40^{\circ }]$. This will be referred to flow deflection angle reversal, and this reversal will make the shock wave $S_{OR}$ no longer an incident one but a reflected one, and type I and type II shock interference will occur; see § 3.4 for more details.

Similar phenomena are observed for other sets of conditions, as can be seen from figure 15 for $M_{r}=6$ and $M_{s}=5.2$.

Figure 15. Variation of parameters with respect to $\theta _{w}$ for $M_{r}=6$ and $M_{s}=5.2$: (a) variation of $\theta _{1}$, $\theta _{2}$ and $\Delta \theta$; (b) variation of $M$, $M_{1}$ and $M_{2}$.

3.4. Type I and type II shock interference due to flow deflection angle reversal

The line $\theta _{w}=\theta _{CE}(M_{r},M_{s})$ in figure 16, which separates regions $C$ and $E$, is of particular interest. This line corresponds to $\Delta \theta =0$, where $\Delta \theta$, defined by (3.1), is the flow deflection angle across shock $S_{OR}$. Thus the line $\theta _{w}=\theta _{CE}(M_{r},M_{s})$ corresponds to the condition with flow deflection angle reversal discussed in § 3.2; see figure 14(a). Below this line, the shock $S_{OR}$, which is the downstream incident shock for type IV, V and VI shock interference when $\theta _{w}>\theta _{CE}(M_{r},M_{s})$, can no longer be regarded as an incident shock wave of the equivalent problem, but should be regarded as a reflected shock one. This means that the newly generated shock oblique shock wave $S_{NE}$ (see figure 3b) should be one incident shock wave, apart from the RMS ($I_{S}$). The shock $I_{S}$ and the shock $S_{NE}$ then constitute two incident shock waves of different families, which should have type I and type II shock interaction (Keyes & Hains Reference Keyes and Hains1973; Grasso et al. Reference Grasso, Purpura and Délery2003). The line $\theta _{w}=\theta _{EF}(M_{r},M_{s})$ in figure 16 is the transition condition between type I and type II shock interference. Region $E$ above $\theta _{w}=\theta _{EF}(M_{r},M_{s})$ should have type II shock interference, and region $F$ below $\theta _{w}=\theta _{EF}(M_{r},M_{s})$ should have type I shock interference.

Case 4 in table 1 lies in region $E$, where we should have type II shock interference. The numerical solution for case 4 is displayed in figure 21, which is indeed type II shock interference. Figure 21(a) shows the Mach contours, figure 21(b) gives the pressure contours, and figure 21(c) shows the Mach contours and streamlines seen from the frame co-moving with the intersection point $P$. In this case, shock $S_{OR}$ is one reflected shock of the type II shock interference, and shock $I_{S}$ and the newly generated SOSW($S_{NE}$) are the two incident shock waves according to the streamlines shown in figure 21(c). The shock $S_{R2}$ is the other reflected shock wave.

Figure 21. Type II shock interaction for $\theta _{w}=5^{\circ }$, $M_{r}=6$, $M_{s}=5.498$: (a) Mach contours; (b) pressure contours; (c) Mach contours and streamlines in the co-moving frame.

Case 5 in table 1 lies in region $F$, where we should have type I shock interference. The numerical solution for case 5 is displayed in figure 22, which is indeed type I shock interference. Figure 22(a) shows the Mach contours, figure 22(b) gives the pressure contours, and figure 22(c) shows the Mach contours and streamlines seen from the frame co-moving with the intersection point $P$ of $I_{S}$ and $S_{OR}$. In this case, shock $S_{OR}$ is one reflected shock of the type I shock interference, and shock $I_{S}$ and the newly generated SOSW($S_{NE}$) are the two incident shock waves according to the streamlines shown in figure 22(c). The shock $S_{R2}$ is the other reflected shock wave.

Figure 22. Type I shock interaction for $\theta _{w}=2^{\circ }$, $M_{r}=6$, $M_{s}=5.498$: (a) Mach contours; (b) pressure contours; (c) Mach contours and streamlines in the co-moving frame.

Note that type I and type II shock interactions are called regular reflection and Mach reflection in asymmetric shock reflections (Li et al. Reference Li, Chpoun and Ben-Dor1999; Ivanov et al. Reference Ivanov, Ben-Dor, Elperin, Kudryavtsev and Khotyanovsky2002).

4.1. Some shock reflection patterns: primary and secondary reflections

As noted in § 1, shock reflection for an RMS of the second family has been studied before within the context of shock-on-shock interaction. Li & Ben-Dor (Reference Li and Ben-Dor1997) considered an oblique incident shock wave and derived analytical solutions for various shock reflection patterns. Law et al. (Reference Law, Felthun and Skews2003) performed some numerical simulation for shock-on-shock interaction, considering both oblique and normal incident shock waves. These studies have considered the influence of shock angle of the incident shock. Here, we study shock patterns and transition conditions for a normal shock, as for an RMS of the first family, with the emphasis on the role of shock speed. More subtypes of shock reflection patterns are expected to be found here.

Since the shock reflection patterns may be rather complex, it is convenient to consider the global reflection pattern to be a combination of primary reflection and secondary reflection. The primary reflection is the direct reflection at the intersection of the RMS ($I_{S}$) and the SOSW ($S_{OR}$). It may produce regular reflection (RR) and Mach reflection (MR). The secondary reflection is the reflection of reflected shock waves from primary reflection. It may also produce regular reflection and Mach reflection; the latter will be called irregular reflection (IR) to follow the terminology in past studies of pseudo-steady reflection.

Three properly chosen test cases showing different primary shock reflection structures, with $\theta _{w}$, $M_{s}$ and $M_{r}$ as given in table 2, are used for numerical simulation.

Table 2. Test cases for an RMS of the second family. The parameters $\theta _{w}$, $M_{s}$ and $M_{r}$ are specified. The pressure ratio $p_{l}/p_{r}$ is computed by (2.10).

For case 1, the Mach contours at a typical instant are displayed in figure 23(a), and the reflection pattern, displaying the main flow structures and ignoring some details about interaction between shock waves and sliplines, is displayed in figure 23(b). The primary reflection, i.e. the direct reflection at the intersection of the RMS ($I_{S}$) and the SOSW ($S_{OR}$), is a Mach reflection, with Mach stem $Tp_{1}Tp_{2}$. The secondary reflection of the lower reflected shock wave, reflected from the triple point $Tp_{2}$, is an irregular reflection on the wedge, which produces another triple point $Tp_{3}$. There is a kink ($K$) on the reflected shock wave from triple point $Tp_{1}$.

Figure 23. Shock reflection patterns for case 1: (a) Mach contours; (b) shock pattern.

For case 2, the Mach contours at a typical instant are displayed in figure 24(a), and the reflection pattern, with some details ignored, is displayed in figure 24(b). The primary reflection is a regular reflection. The secondary reflection of the lower reflected shock wave is an irregular reflection on the wedge, which produces another triple point $Tp_{3}$. There is an additional triple point $Tp_{4}$ on the reflected shock from triple point $Tp_{1}$.

Figure 24. Shock reflection patterns for case 2: (a) Mach contours; (b) shock pattern.

For case 3, the Mach contours at a typical instant are displayed in figure 25(a) and the reflection pattern is displayed in figure 25(b). The primary reflection at point $P$ is a regular reflection. The secondary reflection of the lower reflected shock wave produces a regular reflection at point $R$ on the wedge. The reflected shock from point $R$ further interacts with the slipline from the primary interaction point $P$, and this interaction produces a transmitted shock wave above that slipline. Similarly to case 2, there is an additional triple point $Tp_{4}$ on the reflected shock from the primary interaction point $P$. The reflected shock from $Tp_{4}$ will interact with the transmitted shock from $R$. The full details are not displayed here since in this study we are concerned mainly with the primary and secondary reflection structures.

Figure 25. Shock reflection patterns for case 3: (a) Mach contours; (b) shock pattern.

For an RMS of the second family, four possible primary–secondary reflection configurations, labelled RR-RR, RR-IR, MR-RR, MR-IR, are shown in figure 26. The RR-RR configuration (figure 26a) means that the RMS ($I_{S}$) and the SOSW ($S_{OR}$) have regular reflection at their direct intersection point ($P$), and the reflected shock ($R_{S2}$) of this reflection has regular reflection on the wall (at point $R$). The RR-IR configuration (figure 26b) means that the RMS ($I_{S}$) and the SOSW ($S_{OR}$) still have regular reflection at their direct intersection point ($P$) , and the reflected shock ($R_{S2}$) of this reflection has irregular reflection on the wall (with its Mach stem at point $N$ on the wall). The MR-RR configuration (figure 26c) means that the RMS ($I_{S}$) and the SOSW ($S_{OR}$) have Mach reflection (with Mach stem $Tp_{1}Tp_{2}$), and the reflected shock ($R_{S2}$) of this reflection has regular reflection on the wall (at point $R$). The MR-IR configuration (figure 26d) means that the RMS ($I_{S}$) and the SOSW ($S_{OR}$) also have Mach reflection (with Mach stem $Tp_{1}Tp_{2}$) , and the reflected shock ($R_{S2}$) of this reflection has irregular reflection on the wall (with its Mach stem at point $N$ on the wall). Types RR-RR and RR-IR have been predicted and found numerically before (cf. Li & Ben-Dor Reference Li and Ben-Dor1997; Law et al. Reference Law, Felthun and Skews2003). The MR-RR configuration is not observed in our numerical simulation, as will be further mentioned at the end of § 4.4. The MR-IR configuration shown in figure 23(a) is different from the case reported before. (Previous studies appear to have reported not an $R_{S2}$ that further produces Mach reflection on the wall, but a curved $R_{S2}$ directly connected to the wall, the condition for which will be studied in § 4.5.)

Figure 26. Possible flow patterns for an RMS of the second family. The reflection at the intersection point $P$ is the primary reflection. The reflection of one reflected shock from primary reflection on the wedge is secondary reflection.

Apart from the secondary reflection of $R_{S2}$ on the wall, the reflected shock $R_{S1}$ may also have secondary reflection structure; see § 4.5 for further discussion.

4.2. Method to find transition condition for primary reflection

The primary reflection, i.e. reflection between $I_{S}$ and $S_{OR}$ at their intersection point ($P$), is an asymmetric reflection, and the reflection type can be decided in the frame co-moving with the intersection point $P$ of $I_{S}$ and $S_{OR}$, through looking for the von Neumann criterion and detachment criterion as by Li et al. (Reference Li, Chpoun and Ben-Dor1999) and Ivanov et al. (Reference Ivanov, Ben-Dor, Elperin, Kudryavtsev and Khotyanovsky2002) for steady asymmetric reflection. The flow parameters that need to be used for deriving the transition conditions are displayed in figure 27. We will use the superscript $(P)$ for flow parameters in the frame co-moving with the intersection point $P$.

Figure 27. Schematic illustration of the asymmetric shock reflection in the co-moving frame with intersection point $P$ (case RR-RR).

The flow velocity of region (0) in figure 27 is

(4.1)\begin{equation} (u_{0}^{(P)},v_{0}^{(P)})=(u_{r}-u_{P},-v_{P})=((M_{r}-M_{s})a_{r},-M_{s} a_{r}\tan \beta_{w}), \end{equation}

where $(u_{P},v_{P})$, the velocity of the intersection point $P$, is given by (2.1). Putting (4.1) into $M_{0}^{(P)}=\sqrt {(u_{0}^{(P)})^{2} +(v_{0}^{(P)})^{2}}/a_{r}$ and $\theta _{0}^{(P)}=\arctan |{v_{0}^{(P)}/} {u_{0}^{(P)}}|$, and using (2.1), we get the following expressions for the Mach number and flow angle in region (0):

(4.2)\begin{equation} \left. \begin{aligned} M_{0}^{(P)} & =\sqrt{(M_{r}-M_{s})^{2}+(M_{s}\tan \beta_{w})^{2}},\\ \theta_{0}^{(P)} & =\arctan\left(\frac{M_{s}\tan \beta_{w}}{M_{s}-M_{r}}\right). \end{aligned} \right\} \end{equation}

The shock angles $\beta _{1}^{(P)}$ and $\beta _{2}^{(P)}$ for the shocks $I_{S}$ and $S_{OR}$ in the frame co-moving with $P$ are related to $(u_{0} ^{(P)},v_{0}^{(P)})$ by

(4.3)\begin{equation} \left. \begin{aligned} & \beta_{1}^{(P)}=\arccos \left( \frac{|v_{0}^{(P)}|}{|(u_{0}^{(P)},v_{0} ^{(P)})|}\right),\\ & \beta_{2}^{(P)}=\arccos \left( \frac{|u_{0}^{(P)}\cos \beta_{w}+v_{0}^{(P)} \sin \beta_{w}|}{|(u_{0}^{(P)},v_{0}^{(P)})|}\right). \end{aligned} \right\} \end{equation}

The expressions (4.2) and (4.3) provide the input parameters $M_{0}^{{(P)}}$, $\theta _{0}^{{(P)}}$, $\beta _{1}^{{(P)}}$ and $\beta _{2}^{{(P)}}$ to be used for deriving the von Neumann condition and detachment condition using the method to find the transition condition of steady asymmetric reflection. In this paper, the shock waves $I_{S}$ and $S_{OR}$ are assumed to be weak shock waves viewing from the co-moving frame with point $P$. The case that they are strong shock waves is not considered.

The flow deflection angles $\theta _{1}^{(P)}$ and $\theta _{2}^{(P)}$ across the shocks $I_{S}$ and $S_{OR}$ shown in figure 27 are obtained from

(4.4)\begin{equation} \tan{{\theta}_{k}^{(P)}}={{f}_{{{\theta}}}}(M_{0}^{(P)},\beta_{k} ^{(P)}),\quad k=1,2\text{ (weak solution)}, \end{equation}

and the Mach number and pressure in regions (1) and (2) are computed as

(4.5)\begin{equation} (M_{k}^{(P)})^{2}=f_{M}(M_{0}^{(P)},\beta_{k}^{(P)}), \quad p_{k}^{(P)} =p_{r}\,{f_{p}}(M_{0}^{(P)},\beta_{k}^{(P)}),\quad k=1,2. \end{equation}

Referring to figure 27 for notations for shock angles and flow deflection angles, the pressures ${p_{3}^{(P)}}$ and ${p_{4}^{(P)}}$ downstream of the reflected shock waves are computed by

(4.6)\begin{equation} \left. \begin{aligned} & \tan{\theta_{13}^{(P)}}={{f}_{{{\theta}}}}(M_{1}^{(P)},\beta_{13}^{(P)}),\quad{p_{3}^{(P)}}={p_{1}^{(P)}\,{f}_{p}}(M_{1}^{(P)},\beta_{13}^{(P)}),\\ & \tan{\theta_{24}^{(P)}}={{f}_{{{\theta}}}}(M_{2}^{(P)},\beta_{24} ^{(P)}),\quad{p_{4}^{(P)}}={p_{2}^{(P)}\,{f}_{p}}(M_{2}^{(P)},\beta_{24}^{(P)}). \end{aligned} \right\} \end{equation}

The expressions in (4.6) are solved along with the flow parallel condition

(4.7)\begin{equation} \left. \begin{aligned} & {\theta_{13}^{(P)}={\theta}_{1}^{(P)}-\theta}_{s}^{(P)},\\ & {\theta_{24}^{(P)}={\theta}_{2}^{(P)}+\theta}_{s}^{(P)}, \end{aligned} \right\} \end{equation}

and pressure balance condition

(4.8)\begin{equation} {p_{3}^{(P)}=p_{4}^{(P)}}. \end{equation}

In (4.7), ${\theta }_{s}^{(P)}$ is the slipline angle as shown in figure 27, and is assumed positive if it deflects in the anticlockwise direction relative to the direction of $(u_{0}^{(P)} ,v_{0}^{(P)})$.

For given $M_{r}$ and $M_{s}$, the detachment condition ${{\theta }_{w}}= \theta _{w}^{(D)}(M_{r},{M_{s}})$ is the condition of ${{\theta }}_{w}$ at which either ${\theta _{13}^{(P)}}$ reaches its detached angle or ${\theta _{24}^{(P)}}$ reaches its detached angle, i.e.

(4.9)\begin{equation} {\theta_{13}^{(P)}=\theta}_{max}{(M_{1}^{(P)})}\quad\text{or}\quad{\theta_{24} ^{(P)}=\theta}_{max}{(M_{2}^{(P)})}. \end{equation}

See (2.5) for the expression for the detached angle.

For given $M_{r}$ and $M_{s}$, the von Neumann condition ${{\theta }_{w}}=\theta _{w}^{(N)}(M_{r},{M_{s}})$ is the condition of ${{\theta }}_{w}$ at which

(4.10)\begin{equation} {p_{m}^{(P)}}={p_{3}^{(P)}}\quad\text{or}\quad{p_{m}^{(P)}}={p_{4}^{(P)}}, \end{equation}

where the pressure ${p_{m}^{(P)}}$ downstream of a strong shock wave with flow deflection angle ${\theta }_{s}^{(P)}$ and with the upstream Mach number $M_{0}^{(P)}$ is calculated from

(4.11)\begin{equation} {p_{m}^{(P)}}={{p}}_{r}\left( 1+\frac{2\gamma}{\gamma+1}\left( {{\left( M_{0}^{(P)}\sin \beta_{s}^{(P)}\right) }^{2}}-1\right) \right), \end{equation}

with $\beta _{s}^{(P)}$ being the strong shock wave solution of $\tan {\theta }_{s}^{(P)}=f_{\theta}(M_{0}^{(P)},\beta _{s}^{(P)})$.

4.3. Method for transition condition of secondary reflection of $R_{S2}$

The secondary reflection, i.e. reflection of the reflected shock $R_{S2}$ on the wedge, at point $R$ as shown in figure 26, is a pseudo-steady reflection similar to that shown in figures 1(c,d). To find the transition condition of such a reflection, we need to know the velocity of $R_{S2}$. For RR-IR and RR-RR as shown in figure 26, this velocity can be found easily, and the transition condition will be considered in this subsection. For MR-IR and MR-RR in figure 26 this velocity can not be found easily.

Now consider pseudo-steady reflection of $R_{S2}$ on the wedge. In a conventional study of pseudo-steady reflection, as shown in figures 1(c,d), the incident shock is in a vertical direction, i.e. the angle $\beta _{R}$ displayed in figure 27 satisfies $\beta _{R}+\theta _{w}=90^{\circ }$. Here it satisfies

(4.12)\begin{equation} \beta_{R}=\theta_{0}^{(P)}-\theta_{2}^{(P)}+\beta_{24}^{(P)}-\theta_{w}. \end{equation}

To find the transition condition, we need the speed $V_{R}$ of the intersection point $R$ between $R_{S2}$ and the wedge surface. Note that $R$ moves along the surface of the wedge. The flow parameters used for this are displayed schematically in figure 28, from which it can be seen that the following geometrical relation holds:

(4.13)\begin{equation} \frac{RA}{\sin \omega}=\frac{PA}{\sin \beta_{R}}, \end{equation}

with $\omega =\beta _{R}-(\beta _{w}-\theta _{w})$. The speed of $R$ is related to the speed $V_{P}$ of point $P$ by $V_{R}=V_{P}({RA}/{PA})$, where $V_{P}=M_{s}a_{r}/\cos (\beta _{w})$ is the modulus of $\boldsymbol {V}_{P}$ already given by (2.1). Using (4.13) for ${RA}/{PA}$, we get

(4.14)\begin{equation} V_{R}=\frac{\sin(\beta_{R}-(\beta_{w}-\theta_{w}))}{\cos(\beta_{w})\sin (\beta_{R})}\,M_{s}a_{r}. \end{equation}

Figure 28. Flow parameters for determining the velocity of $R$.

Now we use superscript $(R)$ to denote flow parameters in the frame co-moving with $R$. The Mach number $M_{d}^{(R)}$ in region $(d)$ is thus

(4.15)\begin{equation} M_{d}^{(R)}=\frac{V_{R}}{a_{d}}-M_{d}, \end{equation}

where $M_{d}$ is the ground frame Mach number in region $(d)$ calculated by $M_{d}^{2}=f_{M}(M_{r},\beta _{w})$, and $a_{d}$ is the sound speed in region $(d)$ that follows from the sound speed expression and the oblique shock wave relation for density and pressure.

Now, with $M_{d}^{(R)}$ and $\beta _{R}$ determined, the transition condition between RR and IR at point $R$ can be decided as for pseudo-steady reflection. Out of various suggested criteria for the termination of RR, Ben-Dor (Reference Ben-Dor2006) pointed out that the one that best agrees with pseudo-steady shock tube experimental data is from the length scale concept of Hornung et al. (Reference Hornung, Oertel and Sandeman1979). This concept suggests that in pseudo-steady flows, RR terminates when the flow behind the reflection point, $R$, of the RR becomes sonic in a frame co-moving with $R$. With $M_{k}^{(R)}$ ($k=1,2$) denoting the Mach number in region $(k)$ ($k=1,2$) of figure 28(a) in the frame co-moving with $R$, this criterion implies that the transition occurs when

(4.16)\begin{equation} M_{2}^{(R)}=1, \end{equation}

where $M_{2}^{(R)}$ can be derived from the oblique shock wave relations

(4.17)\begin{equation} (M_{2}^{(R)})^{2}={f_{M}}(M_{1}^{(R)},\beta_{12}^{(R)}), \end{equation}

where $M_{1}^{(R)}$ is calculated from $(M_{1}^{(R)})^{2}={f_{M}}(M_{d} ^{(R)},\beta _{R})$, and $\beta _{12}^{(R)}$ is the weak solution of $\tan {{\theta }_{d1}^{(P)}}={{f}_{{{\theta }}}}(M_{1}^{(R)},\beta _{12}^{(R)})$, with ${{\theta }_{d1}^{(P)}}$ determined by $\tan {{\theta }_{d1}^{(P)}} ={{f}_{{{\theta }}}}(M_{d}^{(R)},\beta _{R})$.

4.4. Transition conditions in the $M_{s}-\theta _{w}$ and $M_{r} -\theta _{w}$ planes

The transition conditions are expressed in either the $M_{s}$–$\theta _{w}$ plane for fixed $M_{r}$, or the $M_{r}$–$\theta _{w}$ plane for fixed $M_{s}$. The transition conditions in the $M_{s}$–$\theta _{w}$ plane for $M_{r}=5$ are displayed in figure 29(a), and the transition conditions in the $M_{r}$–$\theta _{w}$ plane for $M_{s}=10$ are displayed in figure 29(b). The shock polars for primary reflection in three cases (cases 1 and 2 shown in figure 29(a), and case 3 in figure 29(b) – see also table 2) are displayed in figure 30, with the $I$ polar obtained from inflow Mach number $M_{0}^{(P)}$, and the $R_{1}$ and $R_{2}$ polars obtained from shock deflection angles $\theta _{1}^{(P)}$ and $\theta _{2}^{(P)}$.

Figure 29. Transition criteria: (a) in the $M_{s}$–$\theta _{w}$ plane for $M_{r}=5$; (b) in the $M_{r}$–$\theta _{w}$ plane for $M_{s}=10$.

Figure 30. Shock polars for the primary reflection (in the frame co-moving with $P$): (a) case 1; (b) case 2; (c) case 3.

In figure 29(a), the range of $M_{s}$ is $M_{s}\in \lbrack 6.1,32]$. In figure 29(b), the range of $M_{r}$ is $M_{r}\in \lbrack 2,8.9]$. This range is chosen from the requirement that $M_{s}>M_{r}+1$ for an RMS of the second family.

Regions with RR, double solution (DSD) and MR shown in figure 29 are reflection types for primary reflection. Inside region $C$ (Mach reflection), the line $\theta _{w}=\theta _{min}(M_{r},M_{s})$ is the critical angle to have one or more strong incident shock wave in the co-moving frame of the intersection point $P$ of $I_{s}$ and $S_{OR}$. The flow pattern below $\theta _{w}=\theta _{min}(M_{r},M_{s})$ will be discussed further in § 4.5. The dual solution domain is bounded below by the von Neumann criterion ${{\theta }_{w}}=\theta _{w}^{(N)}(M_{r},{M_{s}})$ computed by (4.10), and bounded above by the detachment criterion ${{\theta }_{w}}=\theta _{w}^{(D)}(M_{r},{M_{s}})$ computed by (4.9). It is interesting to note that regular reflection occurs for large $\theta _{w}$, while MR occurs for small $\theta _{w}$, which appears to be counter-intuitive. A similar phenomenon is observed for an RMS of the first family, where type IV shock interference occurs at smaller $\theta _{w}$ while type VI shock interference occurs at larger $\theta _{w}$.

The line ${{\theta }_{w}}=\theta _{RR,IR}(M_{r},{M_{s}})$ in figure 29 is the critical condition for transition of secondary reflection, i.e. for transition of the reflected shock wave $R_{S2}$ over the wedge, in a region where the primary reflection is a regular reflection. On the left-hand side of ${{\theta }_{w}}=\theta _{RR,IR}(M_{r},{M_{s}})$, we have RR-IR. On the right-hand side of ${{\theta }_{w}}=\theta _{RR,IR}(M_{r},{M_{s}})$, we have RR-RR. In a region where the primary reflection is a Mach reflection, there is no simple way to obtain a similar transition criterion for the secondary reflection, since the velocity of the triple point $Tp_{2}$ is not known. As stated in § 4.1, the MR-RR configuration (figure 26c) did not appear in our numerical simulation. Since there is no simple way to derive the velocity of triple point $Tp_{2}$, no conclusion can be drawn about whether MR-RR is possible.

Now let us return to the shock polars displayed in figure 30. For case 1, the $R_{1}$ and $R_{2}$ polars have no intersection so the reflection type is MR. For case 2, the $R_{1}$ and $R_{2}$ polars have an intersection that is above the other two intersection points (the intersection point of the $R_{1}$ and $I$ polars, and the intersection point of the $R_{2}$ and $I$ polars), which means that case 2 is in the DSD domain. For case 3, the intersection point of the $R_{1}$ and $R_{2}$ polars is below the other two intersection points (the intersection point of the $R_{1}$ and $I$ polars, and the intersection point of the $R_{2}$ and $I$ polars), so the reflection type for case 3 is RR.

For another set of conditions, the results are similar. For instance, figure 31(a) presents the transition conditions in the $M_{r}$–$\theta _{w}$ plane with $M_{s}=20$. It can be seen that the transition criteria are similar to those shown in figure 29(b) for $M_{s}=10$. It is interesting to note that there is a non-monotonic variation for the detachment condition especially after $M_{r}\approx 17$. This non-monotonicity is made evident through displaying, in figure 31(b), the shock polars at points 1, 2 and 3 (with different $M_{r}$ but with $\theta _{w}=10.3^{\circ }$, $M_{s}=20$) from figure 31(a). We observe MR for point 1, DSD for point 2, and MR again for point 3.

Figure 31. (a) Transition criteria for $M_{s}=20$. (b) Shock polars (for points 1, 2 and 3).

The non-monotonicity might be due to the combined effects of the variations of the equivalent flow parameters ($M_{0}^{(p)}$, $\beta _{1}^{(P)}$ and $\beta _{2}^{(P)}$, computed by (4.2) and (4.3)) with respect to $M_{r}$, and these variations are shown in figure 32.

Figure 32. Dependence of some equivalent flow parameters on $M_{r}$ for $M_{s}=20$: (a) equivalent Mach number $M_{0}^{(P)}$; (b) equivalent shock angles $\beta _{1}^{(P)}$ and $\beta _{2}^{(P)}$.

4.5. Brief discussion of refection of secondary shock waves $R_{S1}$ and $R_{S2}$

The reflection of the secondary shock waves $R_{S1}$ and $R_{S2}$ shown in figure 26 may involve more patterns than discussed above. However, a complete study of all the possibilities is beyond the scope of this paper. Here we simply make an incomplete discussion.

4.5.1. Reflection of the secondary shock wave $R_{S1}$

In conventional pseudo-steady shock reflection, where a moving shock wave reflects over a wedge immersed initially in a still gas (as shown in figure 1d), the reflected shock wave (like $R_{S1}$ here) in the case of Mach reflection may be smooth (a situation called single Mach reflection, SMR), have a kink (a situation called transitional Mach reflection, TMR), or have a new triple point (a situation called double Mach reflection, DMR); see figures 12 and 22 of Ben-Dor (Reference Ben-Dor2006) for more detailed classification of subtypes (such as direct, stationary, inverse Mach reflections, DMR$+$, DMR$-$, or terminated RR) and transition conditions between various types of shock reflection.

It would be possible that for the present problem, the reflected shock wave $R_{S1}$ from point $Tp_{1}$ of figure 23(b), or point $P$ of figures 24(b) and 25(b), may also have a kink (point $K$) or an additional triple point. Indeed, in case 1 (MR-IR), we observe a kink according to figure 23(a), and this kink is marked $K$ in figure 23(b). In this case, the primary reflection may be regarded as transitional Mach reflection. Previous studies (cf. Li & Ben-Dor Reference Li and Ben-Dor1997; Law et al. Reference Law, Felthun and Skews2003) showed cases not with such a kink, but with a smooth $R_{S1}$. Now we also show a case with a smooth $R_{S1}$. This case has $M_{s}=20$, $M_{r}=8$, $\theta _{w}=5^{\circ }$, corresponding to point 4 in figure 31(a), and this point is in the region below $\theta _{w}=\theta _{min }(M_{r},M_{s})$ (below which the incident shock is strong). The Mach contours and pressure contours for this condition are displayed in figures 33(a,b), respectively. In this case, $R_{S1}$ is smooth and the primary reflection can be regarded as a single Mach reflection. However, we are unable to find a case for which the primary reflection (in the case of Mach reflection) is a double Mach reflection.

Figure 33. Shock reflection patterns for point 4 in figure 31(a) ($M_{s}=20$, $M_{r}=8$, ${{\theta }_{w}=5}^{\circ }$): (a) Mach contours; (b) pressure contours.

The finding that we may have a smooth $R_{S1}$ or a kink on $R_{S1}$ raises the issue of determining further transition criteria to distinguish the conditions for SMR, TMR or possibly DMR for the present problem. Since TMR is observed above $\theta _{w}=\theta _{min}(M_{r},M_{s})$, and SMR is observed below, one may wonder whether $\theta _{w}=\theta _{min}(M_{r},M_{s})$ is the transition condition between SMR and TMR. However, this requires further study since transition conditions between SMR, TMR and DMR are far more complex than this even in the conventional pseudo-steady shock reflection problem, according to the studies by Li & Ben-Dor (Reference Li and Ben-Dor1995) and Semenov et al. (Reference Semenov, Berezkina and Krassovskaya2012).

In the conventional pseudo-steady shock reflection problem, the entire reflection process was treated as a combination of the shock-reflection process and the flow-deflection process (Li & Ben-Dor Reference Li and Ben-Dor1995). The transition condition between TMR and DMR requires knowledge of the velocity of the kink or the additional triple point, and simplified models, as given in the appendices of Li & Ben-Dor (Reference Li and Ben-Dor1995), are already complex in the conventional pseudo-steady shock reflection case. If we follow the same approach, then the present problem is much more complicated since we need to know in addition the velocity of the triple point ($Tp_{1}$) of figures 26(c,d). Li & Ben-Dor (Reference Li and Ben-Dor1997) provides a theory to estimate the velocity or trajectory in the equivalent shock-on-shock interaction problem. Thus, to derive transition conditions for various types of $R_{S1}$ following the same approach as Li & Ben-Dor (Reference Li and Ben-Dor1995) and Li & Ben-Dor (Reference Li and Ben-Dor1997), one would have to combine the triple point trajectory theory of Li & Ben-Dor (Reference Li and Ben-Dor1995) for a kink or a second triple point on $R_{S1}$, and the theory of Li & Ben-Dor (Reference Li and Ben-Dor1997) for the triple point $Tp1$ shown in figures 26(c,d). It appears that such an analytical study would be highly complex, if not impossible, if one wished to derive transition conditions for various possible types of $R_{S1}$, similar to that given in figure 10 of Li & Ben-Dor (Reference Li and Ben-Dor1995) for the conventional pseudo-steady problem. Such a study will thus not be considered in the present paper, but may be considered in the future.

4.5.2. Reflection of the secondary shock wave $R_{S2}$ on the wall

The reflection pattern of $R_{S2}$ on the wall might also have more subtypes than shown in figure 26, if it were considered as the incident shock in the conventional pseudo-steady shock reflection problem. We immediately have the same difficulty as shown above for $R_{S1}$ if one wants to investigate more details about the possible flow structure and the transition conditions, so such an issue is not considered in this study. We simply consider the subcase when $R_{S1}$ becomes strong with respect to its reflection point on the wall.

The Mach contours displayed in figure 23(a) correspond to case 1 in figure 29(a), for which $R_{S2}$ has Mach reflection, while the work of Li & Ben-Dor (Reference Li and Ben-Dor1997) and Law et al. (Reference Law, Felthun and Skews2003) shows only a curved $R_{S2}$. Here we examine under which conditions this might occur.

In figure 26, $R_{S2}$ is considered as a weak shock. In fact, $R_{S2}$ may be also a strong shock wave seen from the reference frame attached to its intersection point on the wedge (point $R$ of figure 26), according to the critical line $\theta _{w}=\omega (M_{r} ,M_{s})$ shown in figures 34(a,b) (which are obtained when the primary reflection is RR), which use the same conditions as figures 29(b) and 31(a). The critical line $\theta _{w} =\omega (M_{r},M_{s})$ is the condition above which $R_{S2}$ is weak and below which $R_{S2}$ is strong, in the frame co-moving with point $R$ of figure 26. Shock reflection with $R_{S2}$ strong happened to be computed by Law et al. (Reference Law, Felthun and Skews2003), who used a reference frame in which both shock waves are moving. Using the present frame (attached to the wedge), their cases in their figure 13(a,c) correspond to $M_{s}=7.077$, $M_{r} =4.431$, ${{\theta }_{w}=6}^{\circ }$ (called case 1 here), and $M_{s}=7.077$, $M_{r} =4.431$, ${{\theta }_{w}=12}^{\circ }$ (called case 2 here). These two cases will be used for comparison here.

Figure 34. For primary reflection of RR, the region with $R_{S2}$ being strong is displayed as the dashed region. Transition criteria in the $M_{r}$–$\theta _{w}$ plane for (a) $M_{s}=10$, (b) $M_{s}=20$.

For $M_{s}=7.077$, the transition conditions, similar to those shown in figure 34, are displayed in figure 35(a). Figure 35(b) is the anticipated flow pattern. Cases 1 and 2 are shown as circles in figure 35(a). The mentioned two cases of Law et al. (Reference Law, Felthun and Skews2003) are indeed below the critical line $\theta _{w}=\omega (M_{r},M_{s})$, i.e. $R_{S2}$ is strong in the reference frame co-moving with $R$.

Figure 35. Shock reflection with strong $R_{S2}$: (a) transition criteria showing the critical line below which $R_{S2}$ is strong (for $M_{s}=7.077$); (b) hypothetical flow pattern (NR means no reflection).

The Mach contours for both cases computed here are displayed in figure 36(a,b). They compare well with numerical results of figure 13(a,c) of Law et al. (Reference Law, Felthun and Skews2003). The flow pattern in figure 36(a) is indeed similar to the anticipated flow pattern shown in figure 35(b).

Figure 36. (a) Mach contours for case 1 ($M_{s}=7.077$, $M_{r}=4.431$, ${{\theta }_{w} =6}^{\circ }$). (b) Mach contours for case 2 ($M_{s}=7.077$, $M_{r}=4.431$, ${{\theta } _{w}=12}^{\circ }$).

Thus, at the condition well below the critical line $\theta _{w}=\omega (M_{r},M_{s})$, we recover the shock reflection part of previous studies, for which the reflected shock $R_{S2}$ is a curved strong shock.