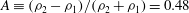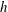2. Experimental apparatus

Experiments were performed using a drop tower, in which a liquid-filled tank is accelerated downwards at a rate greater than gravity to produce the instability. A square acrylic tank, having inside dimensions of $76~\text{mm}\times 76~\text{mm}$ in the two horizontal directions and with a height of 178 mm, was used to contain the liquids. With this set-up, the aspect ratio is large enough that the instability should be unaffected by the top and bottom walls at the end of the self-similar stage, when the mixing-layer thickness is of the order of the tank width. At later times, one would expect a transition to $t^{2/5}$ growth, as found by Lawrie & Dalziel (Reference Lawrie and Dalziel2011). However, this regime is not the focus of the present study. The drop tower consists of two $102~\text{mm}\times 102~\text{mm}$ , 3.05 m long, thick-walled vertical steel columns on which are mounted precision linear rails. Four Thompson Roundway linear roller bearings (two for each guide rail) are attached to a test sled that travels down the rails. The liquid-filled tank and imaging system are mounted to the test sled, which is made from Techno-Isel $30~\text{mm}\times 250~\text{mm}$ extruded aluminium panels that have a system of slots that allow for easy fastening of the required instrumentation (Waddell Reference Waddell1999).

In order to accelerate the test sled downwards at a rate greater than that of gravity, a system of weights and pulleys is used (Waddell et al. Reference Waddell, Niederhaus and Jacobs2001). Renderings of the set-up are shown in figure 4. A cable is fed through five pulleys so that the downward acceleration of the much more massive weight pulls the test sled downwards. This system is similar to a block-and-tackle system operated in reverse. The small distance traversed by the large mass is translated into a larger distance at the test sled, which acquires a larger velocity and acceleration. The mass of the weight-and-pulley system can be altered by changing the number of lead bricks contained in it and the corresponding weight can be increased up to approximately 450 kg, yielding a test sled acceleration of approximately $2g$ . Subtracting the $1g$ downward acceleration due to gravity from the $2g$ acceleration experienced by the liquids results in a net acceleration of approximately $1g$ . An I-beam is attached to the bottom of the test sled that provides the attachment point for the cable to direct its force through. At the bottom of the drop tower are two Enidine shock absorbers to stop the sled at the end of its travel. In addition to the shock absorbers, clay is used to add extra impact absorption. This deceleration system was chosen to keep the acceleration below $70g$ , which is the camera’s maximum acceleration limit.

It is necessary to have a method to quickly and repeatably release the sled initiated by an electronic trigger. To accomplish this, a release mechanism with an unstable linkage secures a $1/4$  in. bolt attached to the test sled. The unstable linkage quickly releases the bolt when a small force is applied by a Dormeyer Industries P10-201L solenoid. Since only a small force is required for release, a P6-101L ‘safety’ solenoid is also used. This second solenoid effectively stabilizes the unstable linkage and must be disengaged before the test sled can be released. A SolidWorks rendering of the release mechanism is depicted in figure 2. More details of this mechanism can be found in Waddell et al. (Reference Waddell, Niederhaus and Jacobs2001).

Figure 2. Rendering of the weight-and-pulley system release mechanism.

A typical acceleration profile measured by an accelerometer that has been attached to the tank lid is shown in figure 3. An average through the main acceleration region between approximately $100$ and $400$  ms gives approximately $1g$ of acceleration. While time-dependent acceleration measurements were obtained for each experiment, only time averages over the main acceleration region were used in the analysis that follows.

Figure 3. Typical acceleration profile during an experiment for the weight-and-pulley apparatus. The acceleration is represented in multiples of $g$ (the ordinate) and time is represented as milliseconds (the abscissa).

Figure 4. Renderings of the weight-and-pulley system.

Figure 5. Rendering of the weight-and-pulley test sled with the experimental equipment attached.

The apparatus used in this study is identical to that used before (Olson & Jacobs Reference Olson and Jacobs2009), but there have been instrumentation improvements. The 60 f.p.s. framing rate of the analogue camera used in the earlier studies has been upgraded with a faster 200 f.p.s. Pulnix TM-6740CL camera with $640\times 480$ resolution and a Cameralink interface connected to a National Instruments PCIe-1427 frame grabber. This system provides more than a threefold increase in camera frame rate, thus increasing the temporal resolution of the data that can be acquired. In addition, the new camera has a bit depth of 10 bits rather than 8 bits, yielding a fourfold increase in greyscale resolution. A rendering of the test sled with the tank and camera attached is shown in figure 5.

Acceleration measurements are also acquired during each experiment. Two accelerometers were used: a Silicon Designs Model 2210-005 capacitive accelerometer with a $\pm 5g$ range and a PCB model JQ353-B32 constant-current piezoelectric accelerometer with a $\pm 100g$ range. The accelerometer measurements are used both for data analysis and to verify that the system is operating within its design limits. Data from the accelerometers are acquired and synchronized with the camera using a National Instruments PCIe-6251 multifunction Data Acquisition (DAQ) card.

3. Liquids and imaging

The experiments in this study were performed using either an immiscible or a miscible liquid combination, where care was taken to match both the Atwood number and the viscosity so that only miscibility and forcing was varied. The Atwood number here was $0.48$ for both of the fluid combinations. In accordance with viscous linear stability theory (Roberts Reference Roberts2012, A.31), the important quantity governing instability growth is the ratio of the dynamic viscosity difference to the sum of the densities. This quantity was matched to within 10 % for both the miscible and immiscible experiments (0.491 cSt for the miscible case and 0.496 cSt for the immiscible case). The immiscible combination consists of lithium polytungstate (LST Heavy Liquid) as the heavy liquid and silicone oil (Clearco 5cSt) as the light liquid. LST Heavy Liquid is a proprietary liquid solution consisting of a polytungstate salt water solution. The concentration of the salt (and thus the density) can be varied, but in the experiments presented here the solution was close to the saturation point. The silicone oil used in this study was chosen because of its low viscosity and non-toxic properties. Surfactant was also added to the liquid combination to reduce the interfacial tension. The surfactant used in this study was AOT (dioctyl sulfosuccinate sodium salt). It should be noted that using surfactant to reduce the surface tension in mixing problems can produce unwanted effects in situations with large interfacial strain, such as occurs in the turbulent RTI. In these situations, highly strained regions of the interface may become depleted of surfactant, thus potentially locally increasing the surface tension. In order to mitigate this effect, a relatively large concentration of surfactant was used. Thus $1~\text{g}~\text{l}^{-1}$ of AOT was added to the LST Heavy Liquid, and whatever did not dissolve was filtered out, thus assuring that the saturation limit was reached. Using this combination to achieve an Atwood number of $0.48$ , the LST Heavy Liquid was diluted to have a specific gravity of $2.61$ and a viscosity of 6.34 cP (calculated using an exponential fit of the manufacturer’s supplied data). The silicone oil has a specific gravity of $0.918$ and a dynamic viscosity of 4.59 cP. Before an experiment, mixed liquids were allowed to equilibrate to room temperature by leaving them undisturbed overnight or longer.

For the miscible configuration, LST Heavy Liquid is used in conjunction with a 90 % ethanol–10 % water mixture. The use of large ethanol concentrations can lead to nonlinear mixing with the water-based heavy liquid solution, resulting in volume not being conserved during the mixing process. In fact, the liquid volume was observed to decrease during an experiment. Since the tank is rigid and sealed, this creates a vacuum, which causes the light liquid to cavitate and bubbles to form within the mixing region during an experiment. To prevent bubbles from forming, an ethanol mixture-filled bladder was affixed on the outside of the tank to provide a reservoir of fluid that could flow in through an opening as the volume decreases. It should be noted that this reduction in volume is consistent with what is observed with pure water mixing with alcohol, where the decrease in volume associated with water with pure ethanol to a 50 % mixture is 7.5 % (Parsons & Estrada Reference Parsons and Estrada1942). Using this information, an estimate was derived to determine how much extra volume of liquid would be required for the heavy-liquid mixing case. Using the fact that the maximum mixing-layer width observed in the miscible experiments was approximately 40 mm and the tank has inside dimensions of 76 mm $\times$ 76 mm, the volume of the mixing region at the end of the experiment was 230 ml. Assuming that the 7.5 % reduction applies to this entire volume, this results in a 17 ml decrease. However, this value represents an upper bound and the actual required volume will be significantly less than this value. Note that this small volume should produce very minimal effect on the evolution of the instability. Although bubbles still occasionally formed, they were normally far enough from the interface not to affect the instability. Also, the bladder is situated at the top of the tank far from the interface to mitigate any effects on the RTI development.

Using LST Heavy Liquid in combination with a 90 % ethanol–10 % water mixture, an Atwood number of $0.48$ was achieved. The LST Heavy Liquid was diluted to have a specific gravity of $2.31$ and a viscosity of 3.02 cP (calculated using an exponential fit of the manufacturer’s supplied data). In addition, pure ethanol was diluted with distilled water to a mole fraction of $0.735$ , yielding a specific gravity of $0.810$ and a viscosity of 1.493 cP (as calculated from Tanaka et al. (Reference Tanaka, Yamamoto, Satomi, Kubota and Makita1977)). Again, before the start of an experiment, mixed liquids were allowed to equilibrate to room temperature by leaving the liquids undisturbed overnight or longer.

The very large differences in the indices of refraction of the liquids used in this study precluded the use of planar imaging techniques. This resulted in backlit imaging being the only optical diagnostic possible for this study. Imaging was therefore accomplished by placing an LED backlight behind the tank and observing the resulting light transmission from the front, as shown in figure 6. Here the distance from the centre of the tank to the camera was 317 mm. Thus, parallax error was always less than 12 %.

Figure 6. Illustration of the imaging system.

When turbulent RTI experiments with unmatched refractive index are performed, the turbulent mixing region between the two pure liquids appears darker than the two pure liquids, making it visible during backlit visualization (Roberts Reference Roberts2012, 3.1.2). Since both fluids are nearly transparent, the mixing region becomes visible due to redirection of light from the refractive-index gradients within the turbid mixing region preventing light from the backlight from reaching the camera. This mechanism is a form of the shadowgraph principle when considering an extended light source (Settles Reference Settles1999). It is also important to note that, as the number of refraction events that a light ray encounters increases, the mixed region will appear darker. Thus, smaller turbulent length scales will result in a darker-appearing mixing region. In all cases, the backlight and camera field of view extends beyond the tank sidewalls. Thus the horizontal extent of all images shown in what follows is always the full tank width.

In addition to the unmatched refractive-index experiments, it was found that, by using trans-anethole as the light liquid, it was possible to match the refractive index with LST Heavy Liquid in an immiscible combination. trans-Anethole is difficult to use owing to its ability to dissolve plastic. However, a small set of matched-index experiments were performed in order to validate the measurements from experiments having unmatched refractive index. Using this combination to achieve an Atwood number of $0.48$ , the LST Heavy Liquid was diluted to have a specific gravity of $2.81$ and a viscosity of 10.21 cP (calculated using an exponential fit of the manufacturer’s supplied data). The trans-anethole has a specific gravity of $0.988$ . Tests were also performed to confirm the validity of Beer’s law for these experiments, and this can be found in Roberts (Reference Roberts2012, 3.2).

4. Initial perturbations

Since it is desired to study the instability in the self-similar regime, small-wavelength initial perturbations when compared to the tank width are required (Cook, Cabot & Miller Reference Cook, Cabot and Miller2004). The self-similar flow is characterized by a progression in time from smaller to larger scales as the mixing-layer width grows in time. Scales cannot become larger than the tank width, and therefore self-similarity ends when the largest scale reaches that point. Thus, the initial perturbation wavelength must be small enough to allow sufficient time to study the self-similar flow. In the experiments presented here, the small initial perturbations imposed on the interface are either forced or left unforced where background noise acts as the source of the perturbations.

In the past, forced initial perturbations were created in our laboratory by horizontally oscillating the fluid-filled tank, which was affixed to a set of horizontal bearings (Waddell et al. Reference Waddell, Niederhaus and Jacobs2001; Wilkinson & Jacobs Reference Wilkinson and Jacobs2007). This method works well to produce a large-wavelength perturbation (with a maximum of approximately five wavelengths spanning the tank width). However, when perturbations with greater than five wavelengths are produced, viscous damping results in amplitude non-uniformity, where the amplitude at the centre is less than that at the edges. An alternative method to produce interfacial perturbations is to parametrically (where gravity is the parameter being varied) excite the two stratified fluids to produce Faraday waves. This technique produces three-dimensional perturbations with uniform amplitude across the container. However, the perturbations produced are more complex and are no longer single-mode. This method was first implemented in this experimental set-up by Olson & Jacobs (Reference Olson and Jacobs2009) using a large stepper motor to oscillate the entire tank containing the liquids in the vertical direction. The production of large-wavenumber disturbances requires a higher frequency of oscillation that proved to be very difficult to accomplish with Olson’s set-up. Also, the large mass of the motor resulted in large forces applied to connecting joints by the nearly $70g$ acceleration that occurs at the end of the experiment. Because of these difficulties, different mechanisms were considered and tested.

In order to determine the displacement of the tank oscillation amplitude necessary to create waves at the interface, an analysis of parametric wave theory was performed, and the details are available in Roberts (Reference Roberts2012, A.3). It was determined that using a spring–mass system driven at resonance would be the best way to produce tank motion of this amplitude without using an exceedingly large motor. The tank and backlight are therefore mounted on an open enclosure that is affixed on the sides to the test sled using crossed roller bearings so that it is constrained to move only in the vertical direction. Equating the expression for Faraday-wave resonance frequency, ${\it\omega}_{w}=\sqrt{4kAg+{\it\gamma}k^{3}/({\it\rho}_{2}+{\it\rho}_{1})}$ , with that of the spring resonance frequency, ${\it\omega}_{s}=\sqrt{K/m}$ , where $K$ is the spring constant, $m$ is the mass of the system, $k$ is the wavenumber and ${\it\gamma}$ is the interfacial tension, allows for the sizing of the required springs. The system uses three springs in parallel, which are held in place on the bottom of the open enclosure and the test sled. These springs provide a large enough spring constant to achieve the desired perturbation with approximately 20 wavelengths across the tank width. Small counterweights that can be adjusted in the front-to-back direction help balance the system and allow for fine tuning of the resonance frequency, allowing for up to an approximately 10 % variation in the resonance frequency. A voice coil is used to produce the force to drive the spring–mass system, allowing the acceleration amplitude to be easily adjusted without changing the frequency by increasing or decreasing the current passing through the coil. A rendering of the shaker system is shown in figure 7.

Figure 7. Rendering of the resonant enclosure used to create the vertical oscillations used for the parametric excitation.

Parametrically forced immiscible and miscible experiments were performed. The smallest-wavelength perturbations that could be produced in the immiscible experiments with this set-up were approximately 3 mm, which required a forcing frequency of approximately 37 Hz. This is the initial perturbation that was used for all the experiments of this type presented in this study. The amplitude of the waves produced is approximately 0.5 mm. The sled release could not be synchronized with the tank oscillation, so each experiment was initiated at a different phase of oscillation. The visualization method used in these experiments did not allow measurement of perturbation spectra. However, the perturbation spectrum should be similar to that measured by Olson & Jacobs (Reference Olson and Jacobs2009), who utilized a similar forcing technique. In the forced miscible experiments, the tank was oscillated vertically at approximately 25 Hz, producing Faraday waves having an amplitude of approximately 1 mm and a wavelength of approximately 3 mm.

In the unforced experiments, the instability was allowed to evolve from background noise of unmeasurable size. However, the initial amplitude can be approximated using linear stability analysis. At an early time in the progression of the experiment, at the point where perturbations first become visible (at approximately 100 ms for the immiscible experiments and 175 ms for the miscible ones), the wavelength and amplitude were noted. Using this measured wavenumber and amplitude, along with the growth rate computed from viscous linear stability analysis, an approximate initial amplitude was determined by extrapolating backwards in time using the linear instability solution. The resulting initial amplitude, found using this technique, was determined to be in the range of $1\times 10^{-7}$ to $1\times 10^{-6}~\text{m}$ (for both miscible and immiscible experiments). It should be noted that the calculation of the predicted initial perturbation amplitude for the miscible case required the inclusion of the effects of a diffuse interface. It is known that diffusion reduces the initial growth rate of the RTI as shown by Duff, Harlow & Hirt (Reference Duff, Harlow and Hirt1962), which was accounted for in the calculated value for initial amplitude. For this calculation, an initial diffusion thickness of 0.5–1 mm was used as obtained by assuming that the development of the diffusion region grows as $\sqrt{Dt}$ , and that 5–10 min has elapsed between the time when the tank was filled until when the experiment is performed. The diffusion coefficient used here ( $1.12\times 10^{-10}~\text{m}^{2}~\text{s}^{-1}$ (Poling Reference Poling2001)) is approximated by taking the average of the diffusion coefficients for ethanol into water and water into ethanol. Further details of this calculation can be found in Roberts (Reference Roberts2012, 4.2.1).

It should also be noted that, while the form of the noise spectrum that resulted in the first observed perturbations is unknown, the many e-foldings that have occurred during this process result in a nearly single-scale perturbation at the fastest-growing wavelength. Thus, the primary difference between the forced and unforced experiments is the many orders-of-magnitude difference in the amplitude of the perturbations. In addition, forced experiments have a smaller wavelength with a smaller bandwidth, while the unforced experiments have a larger bandwidth centred around a wavelength that is slightly larger. However, in both cases we obtain a small-wavelength, finite-bandwidth initial perturbation that one would expect to result in the mode-coupling case described by Ramaprabhu et al. (Reference Ramaprabhu, Dimonte and Andrews2005).

One might expect components of the initial perturbation to be on the scale of the tank. However, in all of the experiments performed, none were observed, except for the effect of slight tilting of the tank with respect to the vertical direction. This tilting, which was impossible to remove entirely, produces the equivalent of a very small-amplitude triangular perturbation. In the cases with forced perturbations, the effects of this triangular perturbation are much less than that of the much faster-growing shorter-wavelength forced perturbation and thus are not observable. However, in the cases with unforced perturbations and particularly those with miscible fluid combinations that have much slower growth rates, the effects of tilting are more pronounced. But, nevertheless, they are always confined to regions near the walls.

Figure 8. Experimental images progressing in time in which a diluted LST Heavy Liquid with AOT as a surfactant (bottom liquid) and 5 cSt silicone oil (top liquid) combination, giving an Atwood number of $0.48$ , was used. These experiments are unforced. Here the horizontal extent of the images is the full tank width (76 mm) at $t=0~\text{ms}$  (a), 25 ms (b), 50 ms (c), 75 ms (d), 100 ms (e), 125 ms (f), 150 ms (g), 175 ms (h), 200 ms (i), 225 ms (j), 250 ms (k), 275 ms (l), 300 ms (m), 325 ms (n), 350 ms (o) and 375 ms (p).

5. Results and discussion

5.1. Qualitative results

For the immiscible experiments performed in this study, the fluids were initially added to the tank (heavy liquid first) with care taken to remove all air bubbles. Because the fluids remain unmixed, experiments were performed repeatedly without emptying the tank. Enough time was allowed to pass (approximately 10 min) between experiments to allow the emulsion layer that develops at the interface to dissipate. Shown in figure 8 is a sequence of images of a typical unforced immiscible experiment. It can be observed in this sequence that a range of scales develops at late time, beginning at approximately 300 ms, where both large and small wavelengths are observed. The initially small structures can be observed to merge into larger and larger structures as time progresses. Since a range of small scales is initially present, once the linear regime is no longer valid, nonlinear mode coupling will allow the creation of additional wavelengths, which is also observed. As the mixing-layer width becomes larger, so does the largest turbulent length scale, which is consistent with the concept of self-similarity. It should be noted that all experiments showed very slight run-to-run variations, such as slight asymmetry or variation in the mixing-layer thickness across the tank width. However, these were not consistent from experiment to experiment and thus were assumed to be the result of randomness in the initial perturbation. For this reason, ensemble averages were used to obtain the mixing-layer widths along with the associated ${\it\alpha}$ values. Using the eigenvalue expression from a viscous linear stability analysis that includes the effects of interfacial tension to obtain the fastest-growing wavelength (Roberts Reference Roberts2012, A.31), one can estimate the interfacial tension of the fluid combination by assuming that the observed dominant wavelength at the onset of the instability is equal to the fastest-growing wavelength from the linear stability analysis. At a time of approximately 100 ms, the instability is still in the linear regime and a dominant wavelength of approximately 4.3 mm is clearly visible. Using this wavelength along with the known viscosities of the two liquids, the interfacial tension is found to be $2.1~\text{mN}~\text{m}^{-1}$ . This value is also consistent with the value of $1.6~\text{mN}~\text{m}^{-1}$ found for the heptane/calcium nitrate solution combination with AOT added that was used by Waddell et al. (Reference Waddell, Niederhaus and Jacobs2001).

Figure 9. Experimental images progressing in time in which a diluted LST Heavy Liquid without a surfactant (bottom liquid) and 5 cSt silicone oil (top liquid) combination, giving an Atwood number of 0.48, was used. These experiments are unforced. Here the horizontal extent of the images is the full tank width (76 mm) at $t=0~\text{ms}$  (a), 25 ms (b), 50 ms (c), 75 ms (d), 100 ms (e), 125 ms (f), 150 ms (g), 175 ms (h), 200 ms (i), 225 ms (j), 250 ms (k), 275 ms (l), 300 ms (m), 325 ms (n), 350 ms (o) and 375 ms (p).

Although it was expected that surfactant was required to produce small scales, an experiment was performed without surfactant to verify this assumption. In figure 9, an experiment with the same fluid combination and parameters as that used in figure 8 is presented, where the only difference is the lack of surfactant. In this experiment, only a few wavelengths across the tank were observed, and thus there is not enough domain size to display a large range of scales. Once again, an attempt to determine the interfacial tension was made using the experimentally observed wavelength. Although it was difficult to determine a dominant wavelength in this experiment, it was approximated to be 11 mm, which yields an approximate interfacial tension of $20~\text{mN}~\text{m}^{-1}$ . Thus, adding surfactant appears to produce a factor of $10$ decrease in the interfacial tension.

Figure 10 shows an immiscible experiment in which parametric forcing is used to produce the initial perturbation. It is observed that the initial wavelength is much less than the tank width. In addition, as with the unforced experiments (figure 8), there appears to be a large range of scales present at late time, as can be seen at 300 ms and later. The Faraday forcing used in this study does not produce a monochromatic initial perturbation. However, the perturbation that is produced has a bandwidth narrower than that which evolves from background noise. Therefore, the forced experiments might be expected to have a decreased amount of mode coupling when compared to the unforced experiments, which logically would result in a reduction in the degree of self-similarity observed. This difference, therefore, might be expected to affect the measured growth constant ${\it\alpha}$ . It is also apparent that the forced experiments develop into the possibly turbulent self-similar regime earlier than the unforced ones. With a larger initial amplitude, the forced experiments should be expected to progress into turbulence (and therefore self-similarity) more rapidly. All of these observations suggest that the effects of forcing on the growth of the turbulent mixing region deserve further study.

Figure 10. Parametrically forced experimental images progressing in time in which a diluted LST Heavy Liquid with surfactant (bottom liquid) and 5 cSt silicone oil (top liquid) combination, giving an Atwood number of 0.48, was used. Here the horizontal extent of the images is the full tank width (76 mm) at $t=0~\text{ms}$  (a), 25 ms (b), 50 ms (c), 75 ms (d), 100 ms (e), 125 ms (f), 150 ms (g), 175 ms (h), 200 ms (i), 225 ms (j), 250 ms (k), 275 ms (l), 300 ms (m), 325 ms (n), 350 ms (o) and 375 ms (p).

As with the immiscible experiments, miscible experiments were performed by first adding the heavy liquid and then the light one. Owing to the miscible nature of the liquids, a piece of balsa wood was rested atop the heavy liquid and allowed to float while the light liquid was added. This had the effect of spreading the liquid flow and thus decreasing the velocity to minimize mixing between the two fluids. Unlike with the immiscible experiments, the tank had to be refilled with new pure fluids after every experiment.

Figure 11. Miscible unforced liquid experiments performed on the weight-and-pulley apparatus with diluted LST Heavy Liquid (bottom liquid) and 90 % ethanol–10 % water (top liquid), having an Atwood number of 0.48. Here the horizontal extent of the images is the full tank width (76 mm) at $t=0~\text{ms}$  (a), 25 ms (b), 50 ms (c), 75 ms (d), 100 ms (e), 125 ms (f), 150 ms (g), 175 ms (h), 200 ms (i), 225 ms (j), 250 ms (k), 275 ms (l), 300 ms (m), 325 ms (n), 350 ms (o) and 375 ms (p).

Shown in figure 11 is a sequence of images from a miscible unforced experiment. In these experiments, it is apparent that the first observable interfacial waves appear after twice as much time has passed when compared to the immiscible experiments. In addition, these first observed perturbations appear to have a smaller wavelength than their immiscible counterparts and are of varying scale. It should be noted that a linear stability calculation including viscosity but neglecting diffusion yields a fastest-growing wavelength of 0.9 mm, which agrees well with that observed here. Adding diffusion to the analysis would further increase the fastest-growing wavelength, bringing it even closer to the observed value (1.8 mm). At late time, the development of a range of scales also is observed. Without the stabilizing effect of interfacial tension on small wavelengths, a larger range of wavelengths is present at late time. It is therefore more likely that the miscible experiments will have achieved true self-similarity because of the larger range of scales achieved.

Figure 12. Miscible forced liquid experiments performed on the weight-and-pulley apparatus with diluted LST Heavy Liquid (bottom liquid) and 90 % ethanol–10 % water (top liquid), having an Atwood number of 0.48. The frequency of forcing here is 25 Hz. Here, the horizontal extent of the images is the full tank width (76 mm) at $t=0~\text{ms}$  (a), 25 ms (b), 50 ms (c), 75 ms (d), 100 ms (e), 125 ms (f), 150 ms (g), 175 ms (h), 200 ms (i), 225 ms (j), 250 ms (k), 275 ms (l), 300 ms (m), 325 ms (n), 350 ms (o) and 375 ms (p).

In figure 12, a sequence of images from a forced miscible experiment is shown. In these forced experiments, it is observed that the growth of the mixing region develops nearly immediately, as was observed in the forced immiscible experiments. Similar to the immiscible experiments, at late time we also observe the development of a range of scales.

Shown in figure 13 is a comparison of the late-time behaviour of all four cases, all taken at a time in which the mixing-layer width is approximately the same. When comparing the two immiscible experiments, it is observed that the dominant wavelength (as observed in the profile of the bubble and spike fronts) appears similar in size, which is consistent with the assumption that forcing small wavelengths does not alter the flow once self-similarity is reached and implies that initial conditions do not influence the self-similar regime. Comparing the dominant length scales between the two miscible experiments yields a similar conclusion. However, one significant difference between the miscible and immiscible experiments is that the miscible experiments have noticeably darker mixing regions than the immiscible ones, thus implying that significantly smaller wavelengths are present in the miscible experiments than in the immiscible ones. Note that the lack of surface tension in the miscible experiments would allow the formation of smaller scales that would be suppressed by the presence of surface tension in the immiscible experiments. Thus the presence of shorter length scales is not the result of diffusion but instead is the result of the lack of surface tension. This observation, however, contradicts the fact that miscibility also results in molecular mixing, which should act to reduce refractive-index gradients. Thus the fact that the miscible experiments have darker mixing zones implies that the lack of surface tension is more dominant than the effect of diffusion.

Figure 13. Comparison between forced, unforced, miscible and immiscible experiments performed on the weight-and-pulley apparatus for the 0.48 Atwood number case at $t=320~\text{ms}$ for immiscible unforced (a), 380 ms for miscible unforced (b), 275 ms for immiscible forced (c) and 300 ms for miscible forced (d) cases. Images were chosen at times in which the mixing-layer width is approximately matched between experiments.

5.2. Quantitative results

The primary measured quantity in this study is the mixing-layer width, $h$ , and it is desired to determine how this quantity progresses in time. The mixing-layer width can be divided into that of the bubble (the portion in which the less dense mixing region grows into the heavy liquid), $h_{b}$ , and that of the spike (the portion in which the more dense mixing region grows into the light liquid), $h_{s}$ . The widths of the bubble and spike regions are found by subtracting the locations of the spike and bubble extents, respectively, from the initial interface location. Image analysis was performed using programs written in Java utilizing the ImageJ and JExcel libraries as the main components (Roberts Reference Roberts2012, 6.2).

The first step in the process was to obtain an intensity average across each row for each frame in the usable mixing region area. The usable mixing region area was determined by excluding structures created by interaction with the sidewalls of the tank. At the latest usable frame for an experiment, the usable region was determined by excluding obvious wall effects, and this is the region used for averaging throughout the experiment. The mixing region width measurements also had to be measured while accounting for inhomogeneities in the tank and in the backlight. Ideally, one could divide each experimental image by an image in which the tank was empty to account for these non-uniformities. However, in addition to the backlight intensity being non-uniform in space, it was also found to vary in time due to temperature variations. For this reason, all of the experimental images for a particular run were scaled by the first image in the sequence and then the top and bottom fluid regions were re-multiplied by an average of a representative area of the corresponding fluid. This area was chosen to be near the interface to account for the backlight intensity variations that occur near the edges of each fluid.

It is important to point out that there is no standardized way of determining the mixing-layer width from backlit images such as used here. However, since we do not obtain concentration measurements, a consistent way of extracting the mixing-layer widths had to be developed. After the initial rescaling process has been performed, the data from each image were then row-averaged. Marching from both the light and heavy liquid towards the interface, for the bubble and spike, respectively, the row-averaged intensity value is compared to the average intensity value in the pure liquid region. The edge of the mixing layer is determined when the intensity ( $I$ ) drops below a threshold percentage value ( $P$ ) defined by

(5.1) $$\begin{eqnarray}I_{thresh}=I_{drk}+(I_{lght}-I_{drk})\times \frac{P}{100}.\end{eqnarray}$$

It was found that a threshold value of 80 % produces the most consistent results. Other threshold values (70 % and 90 %) were considered, but since they did not always yield a smoothly increasing mixing width across a set of experiments, they were not chosen. A 95 % threshold is often used in experiments in which concentration is measured, but this value showed inconsistent results in the present study and was thus found to be unsuitable. Also, because transmitted light intensity is not an indicator of concentration, using a 95 % threshold would not necessarily correspond to an equivalent concentration threshold. In figure 14, a montage of images is shown. Superimposed on each image are a scaled row-averaged profile along with horizontal lines indicating the determined edge locations found from using 70 %, 80 % and 90 % threshold values. Also, one line is present that represents the intersection of a least-squares curve fit of the intensity profile with the quiescent intensity value. Note that the evolution in time of the profiles appears to have the characteristics of self-similarity: as the profile becomes wider with time, its shape remains similar. It is obvious from the figure that using a 90 % or 95 % threshold value would result in only a slight increase in ${\it\alpha}$ . However, doing so would also bring with it significantly increased error due to increased run-to-run variation.

Figure 14. A sequence of images where the images of an ensemble-averaged refractive-index mismatch experiment have been post-processed. Horizontally averaged intensity values are superimposed on the images. The profiles have the characteristics of a self-similar flow as time progresses. Also on the images are horizontal lines representing different bubble and spike extent measurements so they can be compared. Times are (a)  $t=100~\text{ms}$ , (b)  $t=125~\text{ms}$ , (c)  $t=150~\text{ms}$ , (d)  $t=175~\text{ms}$ , (e)  $t=200~\text{ms}$ , (f)  $t=225~\text{ms}$ , (g)  $t=250~\text{ms}$ , (h)  $t=275~\text{ms}$ , (i)  $t=300~\text{ms}$ , (j)  $t=325~\text{ms}$ , (k)  $t=350~\text{ms}$ and (l)  $t=375~\text{ms}$ .

Plots showing the time evolution of mixing-layer width are shown in figure 15. Note that the parametrically forced experiments obtain a mixing-layer width larger than their unforced counterparts. As was shown in the image sequences, forcing produces larger-amplitude initial perturbations that also produce faster initial growth. Also, there is a very obvious difference between the immiscible experiments and the miscible ones. The miscible experiments grow significantly more slowly than the immiscible ones. This is to be expected since a diffuse interface will result in a reduction in the local Atwood number and thus growth rate as dictated by linear stability analysis.

Figure 15. Mixing-layer width for ensemble-averaged experiments where 80 % of the pure liquid intensity was taken as the mixing-layer width cutoff. Data are for LST Heavy Liquid experiments for the four cases discussed previously: (a) immiscible unforced, (b) immiscible forced, (c) miscible unforced and (d) miscible forced.

We would like to determine the effects of forcing on the growth parameter ${\it\alpha}$ . We are not able to determine conclusively whether self-similarity is achieved in our experiments. However, since turbulence is required for self-similarity, it is useful to compute the Reynolds number in our experiments to determine whether it is at least as large as those of other studies that observed self-similar flow, i.e. the results of Dalziel et al. (Reference Dalziel, Linden and Youngs1999) and Ramaprabhu & Andrews (Reference Ramaprabhu and Andrews2004). The Reynolds number obtained by Ramaprabhu & Andrews was approximately $1000$ . This value was calculated using

(5.2) $$\begin{eqnarray}Re=\frac{h{\dot{h}}}{{\it\nu}},\end{eqnarray}$$

where $h$ represents the full mixing-layer width and ${\dot{h}}$ is its temporal derivative. In the present study, bubble and spike Reynolds numbers are calculated individually,

(5.3) $$\begin{eqnarray}Re=\frac{h_{b/s}{\dot{h}}_{b/s}}{{\it\nu}},\end{eqnarray}$$

so the corresponding Reynolds numbers of Ramaprabhu & Andrews becomes a factor of four smaller, yielding a value of $250$ . An approximate bubble Reynolds number in the experiments of Dalziel et al. at a time where the flow was verified, by internal mixing region measurements, to be turbulent was $3700$ (Dalziel et al. Reference Dalziel, Linden and Youngs1999; S. B. Dalziel 2013, personal communication). Plots of Reynolds number versus time for the cases studied here are shown in figure 16. The approximate maximum values, excluding the scatter towards the ends of the experiments, are approximately $2000$ , $4000$ , $1500$ and $4000$ for the unforced immiscible forced immiscible unforced miscible and forced miscible experiments, respectively, where the values for the spikes are approximately 20 % larger than those for the bubbles. It is observed that the maximum Reynolds numbers for the forced experiments are twice those for the unforced ones. Since these values are larger than those obtained by Ramaprabhu & Andrews and similar to those of Dalziel et al. (both of whom found their flows to be self-similar and turbulent by internal mixing-layer measurements), we will assume our experiments are self-similar.

Figure 16. Reynolds-number data for ensemble-averaged experiments where 80 % of the pure liquid intensity was taken as the mixing-layer width cutoff. Data are for the four cases of the LST Heavy Liquid experiments: (a) immiscible unforced, (b) immiscible forced, (c) miscible unforced and (d) miscible forced.

Under the premise that we have a large enough Reynolds number to achieve self-similarity, we will proceed with the determination of the growth factor ${\it\alpha}$ . As shown by Dimonte & Schneider (Reference Dimonte and Schneider2000), one way to measure ${\it\alpha}$ is to plot $\sqrt{h_{b/s}}$ versus $t\sqrt{Ag_{eff}}$ and fit a straight line (by the method of least squares) through the part of the curve that appears linear at late time. Squaring the slope of this line will yield an averaged value of ${\it\alpha}$ . Another way of determining ${\it\alpha}$ is to use a method proposed by Cabot & Cook (Reference Cabot and Cook2006) using an expression developed by Ristorcelli & Clark (Reference Ristorcelli and Clark2004) where

(5.4) $$\begin{eqnarray}{\it\alpha}=\frac{{\dot{h}}_{b/s}^{2}}{4Ag_{eff}h_{b/s}}.\end{eqnarray}$$

This gives a time-dependent expression for ${\it\alpha}$ which can be compared to the average value.

5.2.1. Immiscible experiments

In using the fitting method for the calculation of ${\it\alpha}$ as used by Dimonte & Schneider (Reference Dimonte and Schneider2000), it is desirable to employ a fixed fitting region for consistency in comparing values from different sets of experiments. For the immiscible fluid combination in the present study, the fitting region was chosen to be $5~\text{mm}^{1/2}<t\sqrt{Ag_{eff}}<25~\text{mm}^{1/2}$ and the curve fits are shown in figure 17. For the ${\it\alpha}$ calculations, $g_{eff}$ was found by averaging over the main acceleration region between approximately $100$ and $400$  ms, which yielded $11.06\pm 0.02~\text{m}~\text{s}^{-2}$ for the unforced case and $10.87\pm 0.33~\text{m}~\text{s}^{-2}$ for the forced case, where uncertainties represent 95 % confidence intervals of measurements from individual experiments. The calculated values of ${\it\alpha}$ are displayed on the plots. At the end of the unforced experiments, ${\it\alpha}$ is nearly constant, indicated by the linearity of the data at late time, with a value of $0.059\pm 0.002$ for the spike and $0.047\pm 0.007$ for the bubble. For the forced case, ${\it\alpha}$ is $0.057\pm 0.014$ for the spike and $0.044\pm 0.009$ for the bubble. These ${\it\alpha}$ values represent an ensemble average of $13$ experiments for the unforced case and $10$ experiments for the forced case. Here the uncertainties were calculated using a 95 % confidence interval from ${\it\alpha}$ values determined from experiments analysed individually. Note that the ${\it\alpha}$ values obtained in both cases are smaller than those obtained in earlier experimental studies. For example, when comparing the experiments that match the Atwood number used in our experiments, Youngs & Read (Reference Youngs and Read1983) obtained ${\it\alpha}$ values of $0.086$ and $0.066$ for spike and bubble, respectively, Kucherenko et al. (Reference Kucherenko, Shibarshov, Chitaikin, Balabin and Pylaev1991) obtained values of $0.070$ and $0.055$ , respectively, and Dimonte & Schneider (Reference Dimonte and Schneider2000) obtained values of $0.063$ and $0.050$ , respectively. However, it should be noted that the data of figure 17 have significantly greater time resolution and are an average over a much larger ensemble than in previous studies. As a result, the measurements shown in this figure yield much smoother curves than in the earlier experiments and thus could explain the small difference in ${\it\alpha}$ values measured here. In addition to attaining smaller ${\it\alpha}$ values than previously found, the results presented here indicate that imposing forced initial perturbations with a wavelength smaller than the fastest-growing wavelength and larger than the cutoff wavelength (as is done in the forced experiments) yields an ${\it\alpha}$ value similar to that obtained in the unforced experiments. The larger amplitude of the forced case extends the experimental time in the nonlinear regime for the flow to evolve, but the similar ${\it\alpha}$ values imply the extra evolution time is not necessary to achieve the same final result. The spike to bubble ratios are also very similar for the forced and unforced experiments, where the ratio ${\it\alpha}_{s}/{\it\alpha}_{b}$ was found to be $1.26\pm 0.19$ and $1.30\pm 0.41$ for the unforced and forced cases, respectively. Here the error estimates were obtained by propagating error estimates from the bubble and spike values. These ratios compare well with past experiments having similar Atwood number, where Youngs & Read (Reference Youngs and Read1983) found a ratio of $1.3$ , Dimonte & Schneider (Reference Dimonte and Schneider2000) found a ratio of $1.26$ and Kucherenko et al. (Reference Kucherenko, Shibarshov, Chitaikin, Balabin and Pylaev1991) found a ratio of $1.27$ .

Figure 17. Measurements of ${\it\alpha}$ determined by fitting a straight line to the $\sqrt{h_{b/s}}$ versus $t\sqrt{Ag_{eff}}$ plot for ensemble-averaged experiments where 80 % of the pure liquid intensity was taken as the mixing-layer width cutoff. Data are for the two LST Heavy Liquid immiscible cases: (a) immiscible unforced; ${\it\alpha}^{b}=0.047$ , ${\it\alpha}^{s}=0.059$ and (b) immiscible forced; ${\it\alpha}^{b}=0.044$ , ${\it\alpha}^{s}=0.057$ .

Using (5.4), ${\it\alpha}$ is plotted in figure 18, where ${\dot{h}}_{b/s}$ is determined using a central difference approximation and $g_{eff}$ are the average values used in the $\sqrt{h}$ versus $t$ method described above. A weighted running average of ${\it\alpha}$ of 20 % of the total number of points in the particular experiment is also performed (represented as solid and dashed lines on the plot) to smooth the data. The weighted running average function performs a nonlinear regression using a locally weighted regression method, where more weight is given to the central data point. Horizontal dashed lines are also drawn representing the average ${\it\alpha}$ values obtained by the square-root method, described above, showing that it agrees well with (5.4) at late time. It is observed that ${\it\alpha}$ for the forced and unforced cases show similar values.

Figure 18. Measurements of ${\it\alpha}$ determined by the method of Cabot & Cook (Reference Cabot and Cook2006) for ensemble-averaged immiscible experiments where 80 % of the pure liquid intensity was taken as the mixing-layer width cutoff. Here ${\dot{h}}_{b/s}$ was calculated using a central difference approximation. Data are for the two LST Heavy Liquid cases: (a) immiscible unforced and (b) immiscible forced. The horizontal line here represents ${\it\alpha}$ obtained from the square-root method and is drawn to show the consistency between methods of determining ${\it\alpha}$ : the top line represents the spike and the bottom represents the bubble for both plots. Also, a weighted running average of 20 % of the total number of points in the particular experiment is also performed (represented as solid and dashed lines on the plot) to smooth the data.

As mentioned above, in addition to the unmatched refractive-index experiments, a small set of experiments were performed using a liquid combination in which LST Heavy Liquid is the heavy liquid and trans anethole is the light liquid, where the refractive indices were matched. In addition, these experiments were unforced. The analysis of these experiments utilized Beer’s law absorption to obtain integrated concentration profiles, from which the 10 % and 90 % concentration thresholds of LST Heavy Liquid were used to determine the spike and bubble front locations. From these, ${\it\alpha}$ measurements were obtained utilizing the same techniques as used in the unmatched-index experiments. For these matched-index experiments, ${\it\alpha}$ was found to have a value of $0.046\pm 0.011$ for the spike and $0.039\pm 0.015$ for the bubble. These ${\it\alpha}$ values represent an ensemble average of nine experiments. Here the uncertainties were calculated using a 95 % confidence interval from ${\it\alpha}$ values determined from experiments analysed individually. These measurements compare well with the non-index-matched experiments, thus validating our results. More information on these experiments can be found in Roberts (Reference Roberts2012, 6.2).

Figure 19. Measurements of ${\it\alpha}$ determined by fitting a straight line to the $\sqrt{h_{b/s}}$ versus $t\sqrt{Ag_{eff}}$ plot for ensemble-averaged experiments where 80 % of the pure liquid intensity was taken as the mixing-layer width cutoff. Data are for the two LST Heavy Liquid miscible cases: (a) miscible unforced; ${\it\alpha}^{b}=0.030$ , ${\it\alpha}^{s}=0.047$ and (b) miscible forced; ${\it\alpha}^{b}=0.023$ , ${\it\alpha}^{s}=0.017$ .

5.2.2. Miscible experiments

The fitting region for the miscible experiments was chosen to be $15~\text{mm}^{1/2}<t\sqrt{Ag_{eff}}<25~\text{mm}^{1/2}$ , which is slightly later in time than for the immiscible experiments due to the difficulty in making measurements at early time. The curve fits for extracting ${\it\alpha}$ are shown in figure 19, where the values of ${\it\alpha}$ are displayed on the plots. For the ${\it\alpha}$ calculations, the average acceleration was computed and used for each set of experiments in the same manner as was done for the immiscible experiments. The acceleration for the unforced and forced cases is $10.12\pm 2.21~\text{m}~\text{s}^{-2}$ and $10.73\pm 0.34~\text{m}~\text{s}^{-2}$ , respectively, where the uncertainties represent 95 % confidence intervals of measurements from individual experiments. These result in unforced spike and bubble ${\it\alpha}$ values of $0.047\pm 0.023$ and $0.030\pm 0.008$ , respectively, and forced spike and bubble values of $0.017\pm 0.008$ and $0.023\pm 0.007$ , respectively. These ${\it\alpha}$ values represent an ensemble average of nine experiments for the unforced case and nine experiments for the forced case as well. Again, the uncertainties here were calculated using a 95 % confidence interval from ${\it\alpha}$ values determined from experiments analysed individually. Thus, as was similarly found for the immiscible experiments, the ${\it\alpha}$ values obtained for the miscible experiments are smaller than those obtained in past experiments. But, unlike the immiscible experiments that yielded ${\it\alpha}$ values only slightly smaller than previous measurements, values for the miscible experiments are significantly smaller than those previously found. Thus the miscible experiments yield ${\it\alpha}$ values that are significantly less than those of their immiscible counterparts. Differences with the immiscible experiments’ spike to bubble ratios for the growth rates are also observed. For the miscible unforced and miscible forced experiments, the ratio ${\it\alpha}_{s}/{\it\alpha}_{b}$ was found to be $1.57\pm 0.87$ and $0.74\pm 0.41$ , respectively. Here the error estimates were obtained by propagating error estimates from the bubble and spike values. Note that the forced miscible ratio is less than unity, indicating a larger bubble growth rate than for the spike. However, the very large error estimate associated with this value indicates that this discrepancy can probably be attributed to experimental uncertainty caused by imaging limitations.

The time-dependent ${\it\alpha}$ value obtained using (5.4) is shown in figure 20, where, as was done for the immiscible experiments, ${\dot{h}}_{b/s}$ is determined using a central difference approximation. Also, as with the immiscible results, a weighted running average of 20 % of the total number of points in the particular experiment is also performed (represented as solid and dashed lines on the plot) to smooth the data and a dashed line is drawn representing the average ${\it\alpha}$ value obtained by the square-root method. As was similarly shown in the immiscible experiments, the square-root method agrees well with (5.4) at late time.

Figure 20. Measurements of ${\it\alpha}$ determined by the method of Cabot & Cook (Reference Cabot and Cook2006) for ensemble-averaged miscible experiments where 80 % of the pure liquid intensity was taken as the mixing-layer width cutoff. Here ${\dot{h}}_{b/s}$ was calculated using a central difference approximation. Data are for the two LST Heavy Liquid cases: (a) miscible unforced and (b) miscible forced. The horizontal line here represents ${\it\alpha}$ obtained from the square-root method and is drawn to show the consistency between methods of determining ${\it\alpha}$ : on the left, the top line is the spike and the bottom the bubble, while it is opposite for the right panel. Also, a weighted running average of 20 % of the total number of points in the particular experiment is also performed (represented as solid and dashed lines on the plot) to smooth the data.

Table 1. Comparison of ${\it\alpha}$ values for experiments with Atwood number approximately 0.5. Here nD represents the refractive index at 589 nm. The refractive indices in this table are unmatched unless otherwise stated. The growth parameter ${\it\alpha}$ was extracted from mixing-layer width measurements using both the method of Dimonte & Schneider (Reference Dimonte and Schneider1996) and that of Cabot & Cook (Reference Cabot and Cook2006), where it was found that both methods yielded consistent results. Imaging for this study is performed by imaging refractive-index mismatch. Data from previous research are also compiled in the table (Youngs & Read Reference Youngs and Read1983; Kucherenko et al. Reference Kucherenko, Shibarshov, Chitaikin, Balabin and Pylaev1991; Youngs Reference Youngs1991; Dimonte & Schneider Reference Dimonte and Schneider2000).

5.2.3. Discussion and comparison of results

A compilation of measured ${\it\alpha}$ values obtained from the experiments described here for both the immiscible and miscible experiments along with those obtained in prior studies is shown in table 1. As described above, good agreement is observed in the present study between the forced and unforced immiscible experiments, with differences less than the respective error estimates indicating that, even though there may be increased mode coupling in the unforced case, it does not appear to strongly alter the late-time self-similar behaviour. The values from the miscible experiments do not show the same level of agreement between the forced and unforced cases. Nevertheless, the forced and unforced measurements are within the range of their respective error estimates. This discrepancy may be expected, owing to difficulties when imaging the miscible experiments. It should be noted that, because of their much slower growth, the miscible experiments are much more strongly affected by edge effects, resulting in vortical structures at the front and back walls of the tank caused by slight tank misalignments. These artifacts along with their associated effects on visualization are discussed in detail in Roberts (Reference Roberts2012, 3.1.3) and are indicated by the larger uncertainty estimates for this set of experiments.

When comparing miscible and immiscible forced experiments, it is observed that the ${\it\alpha}$ values associated with the miscible ones are significantly smaller than those found in the immiscible experiments. Furthermore, the differences between the miscible and immiscible values are significantly larger than their associated error estimates, indicating that miscibility does play a statistically significant role in the growth parameter. This effect can be explained by the fact that molecular mixing will result in a lowering of the local effective Atwood number, as discussed by Cook et al. (Reference Cook, Cabot and Miller2004). Cook et al. define the effective Atwood number as

(5.5) $$\begin{eqnarray}A_{e}\equiv \frac{{\it\rho}_{rms}}{\langle {\it\rho}\rangle |_{0}},\end{eqnarray}$$

which they found to approach $A_{e}=0.48A$ at late time in their miscible simulations. On the other hand, $A_{e}=A$ for immiscible fluids. Here $\langle {\it\rho}\rangle |_{0}$ represents the average density at the centre-plane ( $z=0$ ) and ${\it\rho}_{rms}$ is the centre-plane root mean square density deviation. Cook et al. argue that molecular mixing results in a decrease in the local buoyancy force experienced by regions of lighter and heavier fluids near the zero plane in the mixing layer and thus should result in a lowering of the RTI turbulent growth rate. Cook et al. also hypothesize that this mechanism is the reason why their miscible simulations yield lower ${\it\alpha}$ values than all previous simulations that modelled the fluids as immiscible. The results presented here thus support Cook et al.’s hypothesis that miscibility results in a significant lowering of the RTI growth constant ${\it\alpha}$ . Furthermore, the factor of two difference between our miscible and immiscible ${\it\alpha}$ measurements agrees well with the difference between Cook et al.’s result and those of previous immiscible simulations.

6. Summary and conclusions

In this study, a system of two stratified liquids that is accelerated downwards at a rate greater than gravity is used to study the Rayleigh–Taylor instability. Experiments were performed using a weight-and-pulley apparatus, which produces a net acceleration of approximately $1g$ , with fluid combinations having an Atwood number of $0.48$ . Owing to the large refractive index of the denser liquid for these large-Atwood-number experiments, the refractive index could not be matched. Thus, during an experiment the refractive-index mismatch is imaged. After the instability has progressed late enough in time, a mixing region develops. This mixing layer is assumed to be self-similar and turbulent. In this study, the effect of forcing and miscibility on the turbulent RTI was investigated. The initial conditions of these experiments were either forced to produce small-wavelength perturbations or left unforced (allowing small wavelengths, close in size to the fastest-growing wavelength, to grow). In addition, experiments were performed using miscible and immiscible liquid combinations. In all cases, we obtain a small-wavelength, finite-bandwidth initial perturbation consistent with the bubble-merger, mode-coupling case presented by Ramaprabhu et al. (Reference Ramaprabhu, Dimonte and Andrews2005).

The fact that the dominant scales appear to evolve in time proportionally with the mixing-layer width supports the assumption that the experiments are in the self-similar regime. Also, the Reynolds number was measured and compared to those obtained in earlier experimental studies, where it was verified that self-similarity was reached (from quantitative internal mixing region measurements). The Reynolds numbers achieved in the present experiments were found to be similar to those found in earlier studies, implying that the flow in our experiments is sufficiently turbulent to be self-similar. Using the assumption of self-similarity, ${\it\alpha}$ values were obtained in a consistent manner for all the experiments presented here. The growth parameter ${\it\alpha}$ was extracted from mixing-layer width measurements using both the method of Dimonte & Schneider (Reference Dimonte and Schneider1996) and that of Ristorcelli & Clark (Reference Ristorcelli and Clark2004) and Cabot & Cook (Reference Cabot and Cook2006), where it was found that both methods yielded consistent results. For the immiscible forced experiments, it was found that ${\it\alpha}_{s}=0.057\pm 0.014$ and ${\it\alpha}_{b}=0.044\pm 0.009$ , while for the immiscible unforced experiments ${\it\alpha}_{s}=0.059\pm 0.002$ and ${\it\alpha}_{b}=0.047\pm 0.007$ . The ${\it\alpha}$ value differences between the forced and unforced experiments are within their respective error estimates, thus indicating that the differences in forcing the initial perturbations had no measurable effect in the resulting ${\it\alpha}$ values. For the miscible forced experiments, it was found that ${\it\alpha}_{s}=0.017\pm 0.008$ and ${\it\alpha}_{b}=0.023\pm 0.007$ , while for the miscible unforced experiments, ${\it\alpha}_{s}=0.047\pm 0.023$ and ${\it\alpha}_{b}=0.030\pm 0.008$ . It is particularly notable that the ${\it\alpha}$ values measured for the miscible experiments are significantly smaller than those of the immiscible experiments and that, for three of the four comparisons made, this difference is statistically significant based on the calculated confidence intervals, thus indicating that miscibility plays a role in the late-time turbulent instability growth. It is important to note that the effect of miscibility to reduce the value of ${\it\alpha}$ has never been observed experimentally. However, it supports the hypothesis of Cook et al. (Reference Cook, Cabot and Miller2004) that molecular mixing results in the lowering of the buoyancy force experienced by light and heavy fluid regions near the centreline of the RTI mixing region. It is also significant that ${\it\alpha}$ values measured in the immiscible experiments of the present study are smaller than those measured in previous studies. This difference is most likely the result of the increased temporal resolution and the increased ensemble sizes used in the present study resulting in more accurate measurements of ${\it\alpha}$ at later times.

In this study we experimentally showed that imposing a small-wavelength initial perturbation on the interface does not significantly alter the value of ${\it\alpha}$ when compared to the case where a small-wavelength spectrum (from background noise perturbations) was allowed to develop due to viscous effects in the linear regime. This indicates that the form of a perturbation having a short enough wavelength and finite bandwidth does not affect the growth rate of the turbulent self-similar RTI and supports the conclusions put forward by Ramaprabhu et al. (Reference Ramaprabhu, Dimonte and Andrews2005). It was also found that miscibility does play a role in the turbulent RTI where the miscible experiments have a smaller growth rate than the immiscible ones.