Hostname: page-component-745bb68f8f-hvd4g Total loading time: 0 Render date: 2025-02-11T15:09:37.108Z Has data issue: false hasContentIssue false
  • English
  • Français

Non-realizability of the pure braid group as area-preserving homeomorphisms

Part of: Smooth dynamical systems: general theory Low-dimensional dynamical systems

Published online by Cambridge University Press:  11 June 2020

LEI CHEN Open the ORCID record for LEI CHEN [Opens in a new window]
LEI CHEN*
Affiliation:
Department of Mathematics, California Institute of Technology, Pasadena, CA91125, USA email chenlei@caltech.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 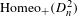$\operatorname{Homeo}_{+}(D_{n}^{2})$ be the group of orientation-preserving homeomorphisms of $D^{2}$ fixing the boundary pointwise and $n$ marked points as a set. The Nielsen realization problem for the braid group asks whether the natural projection 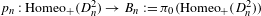$p_{n}:\operatorname{Homeo}_{+}(D_{n}^{2})\rightarrow B_{n}:=\unicode[STIX]{x1D70B}_{0}(\operatorname{Homeo}_{+}(D_{n}^{2}))$ has a section over subgroups of $B_{n}$. All of the previous methods use either torsion or Thurston stability, which do not apply to the pure braid group 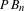$PB_{n}$, the subgroup of $B_{n}$ that fixes $n$ marked points pointwise. In this paper, we show that the pure braid group has no realization inside the area-preserving homeomorphisms using rotation numbers.

Keywords

group actionslow-dimensional dynamicstopological dynamics

MSC classification

Secondary: 37E30: Homeomorphisms and diffeomorphisms of planes and surfaces 37C25: Fixed points, periodic points, fixed-point index theory
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 41 , Issue 7 , July 2021 , pp. 1988 - 1999
Check for updates
Copyright
© The Author(s) 2020. Published by Cambridge University Press

References

Chen, L.. On the nonrealizability of braid groups by homeomorphisms. Geom. Topol. 23(7) (2019), 3735–3749.10.2140/gt.2019.23.3735CrossRefGoogle Scholar
Chen, L. and Markovic, V.. Non-realizability of the Torelli group as area-preserving homeomorphisms. J. Lond. Math. Soc., accepted. Preprint, 2019, arXiv:1904.09490.Google Scholar
Farb, B. and Margalit, D.. A Primer on Mapping Class Groups (Princeton Mathematical Series, 49) . Princeton University Press, Princeton, NJ, 2012.Google Scholar
Franks, J.. Rotation numbers and instability sets. Bull. Amer. Math. Soc. (N.S.) 40(3) (2003), 263–279.10.1090/S0273-0979-03-00983-2CrossRefGoogle Scholar
Franks, J. and Le Calvez, P.. Regions of instability for non-twist maps. Ergod. Th. & Dynam. Sys. 23(1) (2003), 111–141.10.1017/S0143385702000858CrossRefGoogle Scholar
Handel, M.. The rotation set of a homeomorphism of the annulus is closed. Comm. Math. Phys. 127(2) (1990), 339–349.10.1007/BF02096762CrossRefGoogle Scholar
Hubbard, J.. Teichmüller Theory and Applications to Geometry, Topology, and Dynamics. Vol. 1. Matrix Editions, Ithaca, NY, 2006, Teichmüller theory, with contributions by A. Douady, W. Dunbar, R. Roeder, S. Bonnot, D. Brown, A. Hatcher, C. Hruska and S. Mitra, with forewords by W. Thurston and C. Earle.Google Scholar
Mann, K. and Tshishiku, B.. Realization problems for diffeomorphism groups. Proc. Georgia International Topology Festival, accepted. Preprint, 2018, arXiv:1802.00490.Google Scholar
Markovic, V.. Realization of the mapping class group by homeomorphisms. Invent. Math. 168(3) (2007), 523–566.CrossRefGoogle Scholar
Matsumoto, S.. Prime end rotation numbers of invariant separating continua of annular homeomorphisms. Proc. Amer. Math. Soc. 140(3) (2012), 839–845.10.1090/S0002-9939-2011-11435-7CrossRefGoogle Scholar
Milnor, J.. Dynamics in One Complex Variable (Annals of Mathematics Studies, 160) , 3rd edn. Princeton University Press, Princeton, NJ, 2006.Google Scholar
Salter, N. and Tshishiku, B.. On the non-realizability of braid groups by diffeomorphisms. Bull. Lond. Math. Soc. 48(3) (2016), 457–471.10.1112/blms/bdw016CrossRefGoogle Scholar