Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ruelle, David
2009.
A review of linear response theory for general differentiable dynamical systems.
Nonlinearity,
Vol. 22,
Issue. 4,
p.
855.
Ruelle, David
2009.
Structure and f -Dependence of the A.C.I.M. for a Unimodal Map f of Misiurewicz Type.
Communications in Mathematical Physics,
Vol. 287,
Issue. 3,
p.
1039.
Vitolo, Renato
Holland, Mark P.
and
Ferro, Christopher A. T.
2009.
Robust extremes in chaotic deterministic systems.
Chaos: An Interdisciplinary Journal of Nonlinear Science,
Vol. 19,
Issue. 4,
Chekroun, Mickaël D.
Simonnet, Eric
and
Ghil, Michael
2011.
Stochastic climate dynamics: Random attractors and time-dependent invariant measures.
Physica D: Nonlinear Phenomena,
Vol. 240,
Issue. 21,
p.
1685.
Ruelle, David
2011.
Singularities of the susceptibility of a Sinai–Ruelle–Bowen measure in the presence of stable–unstable tangencies
.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 369,
Issue. 1935,
p.
482.
JIANG, MIAOHUA
2012.
Differentiating potential functions of SRB measures on hyperbolic attractors.
Ergodic Theory and Dynamical Systems,
Vol. 32,
Issue. 4,
p.
1350.
Ruelle, David
2012.
A Mechanical Model for Fourier’s Law of Heat Conduction.
Communications in Mathematical Physics,
Vol. 311,
Issue. 3,
p.
755.
Wang, Qiqi
Hu, Rui
and
Blonigan, Patrick
2014.
Least Squares Shadowing sensitivity analysis of chaotic limit cycle oscillations.
Journal of Computational Physics,
Vol. 267,
Issue. ,
p.
210.
Ainsworth, Gareth
2015.
The magnetic ray transform on Anosov surfaces.
Discrete & Continuous Dynamical Systems - A,
Vol. 35,
Issue. 5,
p.
1801.
Talnikar, Chaitanya
Wang, Qiqi
and
Laskowski, Gregory M.
2017.
Unsteady Adjoint of Pressure Loss for a Fundamental Transonic Turbine Vane.
Journal of Turbomachinery,
Vol. 139,
Issue. 3,
Antown, Fadi
Dragičević, Davor
and
Froyland, Gary
2018.
Optimal Linear Responses for Markov Chains and Stochastically Perturbed Dynamical Systems.
Journal of Statistical Physics,
Vol. 170,
Issue. 6,
p.
1051.
Ni, Angxiu
Wang, Qiqi
Fernández, Pablo
and
Talnikar, Chaitanya
2019.
Sensitivity analysis on chaotic dynamical systems by Finite Difference Non-Intrusive Least Squares Shadowing (FD-NILSS).
Journal of Computational Physics,
Vol. 394,
Issue. ,
p.
615.
Ni, Angxiu
2019.
Hyperbolicity, shadowing directions and sensitivity analysis of a turbulent three-dimensional flow.
Journal of Fluid Mechanics,
Vol. 863,
Issue. ,
p.
644.
Chandramoorthy, Nisha
Fernandez, Pablo
Talnikar, Chaitanya
and
Wang, Qiqi
2019.
Feasibility Analysis of Ensemble Sensitivity Computation in Turbulent Flows.
AIAA Journal,
Vol. 57,
Issue. 10,
p.
4514.
Chen, Xian
and
Jia, Chen
2020.
Mathematical foundation of nonequilibrium fluctuation–dissipation theorems for inhomogeneous diffusion processes with unbounded coefficients.
Stochastic Processes and their Applications,
Vol. 130,
Issue. 1,
p.
171.
Crimmins, Harry
and
Froyland, Gary
2020.
Fourier approximation of the statistical properties of Anosov maps on tori.
Nonlinearity,
Vol. 33,
Issue. 11,
p.
6244.
Antown, Fadi
Froyland, Gary
and
Junge, Oliver
2021.
Linear response for the dynamic Laplacian and finite-time coherent sets.
Nonlinearity,
Vol. 34,
Issue. 5,
p.
3337.
Ni, Angxiu
2021.
Approximating Linear Response by Nonintrusive Shadowing Algorithms.
SIAM Journal on Numerical Analysis,
Vol. 59,
Issue. 6,
p.
2843.
Chandramoorthy, Nisha
and
Wang, Qiqi
2022.
Efficient Computation of Linear Response of Chaotic Attractors with One-Dimensional Unstable Manifolds.
SIAM Journal on Applied Dynamical Systems,
Vol. 21,
Issue. 2,
p.
735.
Śliwiak, Adam A.
and
Wang, Qiqi
2023.
Approximating the linear response of physical chaos.
Nonlinear Dynamics,
Vol. 111,
Issue. 2,
p.
1835.


 vector field
vector field  on
on  , then
, then 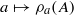 is
is 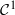 on (−ϵ,ϵ) and
on (−ϵ,ϵ) and