We study the back stable Schubert calculus of the infinite flag variety. Our main results are:

• – a formula for back stable (double) Schubert classes expressing them in terms of a symmetric function part and a finite part;

• – a novel definition of double and triple Stanley symmetric functions;

• – a proof of the positivity of double Edelman–Greene coefficients generalizing the results of Edelman–Greene and Lascoux–Schützenberger;

• – the definition of a new class of bumpless pipedreams, giving new formulae for double Schubert polynomials, back stable double Schubert polynomials, and a new form of the Edelman–Greene insertion algorithm;

• – the construction of the Peterson subalgebra of the infinite nilHecke algebra, extending work of Peterson in the affine case;

• – equivariant Pieri rules for the homology of the infinite Grassmannian;

• – homology divided difference operators that create the equivariant homology Schubert classes of the infinite Grassmannian.

We study the depth filtration on multiple zeta values, on the motivic Galois group of mixed Tate motives over $\mathbb {Z}$ and on the Grothendieck–Teichmüller group, and its relation to modular forms. Using period polynomials for cusp forms for $\mathrm {SL} _2(\mathbb {Z})$, we construct an explicit Lie algebra of solutions to the linearized double shuffle equations, which gives a conjectural description of all identities between multiple zeta values modulo $\zeta (2)$ and modulo lower depth. We formulate a single conjecture about the homology of this Lie algebra which implies conjectures due to Broadhurst and Kreimer, Racinet, Zagier, and Drinfeld on the structure of multiple zeta values and on the Grothendieck–Teichmüller Lie algebra.

We introduce irregular constructible sheaves, which are ${\mathbb {C}}$-constructible with coefficients in a finite version of the Novikov ring $\Lambda$ and special gradings. We show that the bounded derived category of cohomologically irregular constructible complexes is equivalent to the bounded derived category of holonomic ${\mathcal {D}}$-modules by a modification of D’Agnolo and Kashiwara's irregular Riemann–Hilbert correspondence. The bounded derived category of cohomologically irregular constructible complexes is equipped with the irregular perverse $t$-structure, which is a straightforward generalization of usual perverse $t$-structure, and we prove that its heart is equivalent to the abelian category of holonomic ${\mathcal {D}}$-modules. We also develop the algebraic version of the theory.

We revisit the paper [Automorphy lifting for residually reducible$l$-adic Galois representations, J. Amer. Math. Soc. 28 (2015), 785–870] by the third author. We prove new automorphy lifting theorems for residually reducible Galois representations of unitary type in which the residual representation is permitted to have an arbitrary number of irreducible constituents.

The commutative differential graded algebra $A_{\mathrm {PL}}(X)$ of polynomial forms on a simplicial set $X$ is a crucial tool in rational homotopy theory. In this note, we construct an integral version $A^{\mathcal {I}}(X)$ of $A_{\mathrm {PL}}(X)$. Our approach uses diagrams of chain complexes indexed by the category of finite sets and injections $\mathcal {I}$ to model $E_{\infty }$ differential graded algebras (dga) by strictly commutative objects, called commutative $\mathcal {I}$-dgas. We define a functor $A^{\mathcal {I}}$ from simplicial sets to commutative $\mathcal {I}$-dgas and show that it is a commutative lift of the usual cochain algebra functor. In particular, it gives rise to a new construction of the $E_{\infty }$ dga of cochains. The functor $A^{\mathcal {I}}$ shares many properties of $A_{\mathrm {PL}}$, and can be viewed as a generalization of $A_{\mathrm {PL}}$ that works over arbitrary commutative ground rings. Working over the integers, a theorem by Mandell implies that $A^{\mathcal {I}}(X)$ determines the homotopy type of $X$ when $X$ is a nilpotent space of finite type.

Let $G$ be a connected split reductive group over a finite field $\mathbb{F}_{q}$ and $X$ a smooth projective geometrically connected curve over $\mathbb{F}_{q}$. The $\ell$-adic cohomology of stacks of $G$-shtukas is a generalization of the space of automorphic forms with compact support over the function field of $X$. In this paper, we construct a constant term morphism on the cohomology of stacks of shtukas which is a generalization of the constant term morphism for automorphic forms. We also define the cuspidal cohomology which generalizes the space of cuspidal automorphic forms. Then we show that the cuspidal cohomology has finite dimension and that it is equal to the (rationally) Hecke-finite cohomology defined by V. Lafforgue.

We construct analogues of Rankin–Selberg integrals for Speh representations of the general linear group over a $p$-adic field. The integrals are in terms of the (extended) Shalika model and are expected to be the local counterparts of (suitably regularized) global integrals involving square-integrable automorphic forms and Eisenstein series on the general linear group over a global field. We relate the local integrals to the classical ones studied by Jacquet, Piatetski-Shapiro and Shalika. We also introduce a unitary structure for Speh representation on the Shalika model, as well as various other models including Zelevinsky’s degenerate Whittaker model.

We study compact complex three-dimensional manifolds with vanishing second Betti number. In particular, we show that a compact complex manifold homeomorphic to the six-dimensional sphere does carry any non-constant meromorphic function.

Building upon ideas of the second and third authors, we prove that at least $2^{(1-\unicode[STIX]{x1D700})(\log s)/(\text{log}\log s)}$ values of the Riemann zeta function at odd integers between 3 and $s$ are irrational, where $\unicode[STIX]{x1D700}$ is any positive real number and $s$ is large enough in terms of $\unicode[STIX]{x1D700}$. This lower bound is asymptotically larger than any power of $\log s$; it improves on the bound $(1-\unicode[STIX]{x1D700})(\log s)/(1+\log 2)$ that follows from the Ball–Rivoal theorem. The proof is based on construction of several linear forms in odd zeta values with related coefficients.

We exhibit invariants of smooth projective algebraic varieties with integer values, whose nonvanishing modulo $p$ prevents the existence of an action without fixed points of certain finite $p$-groups. The case of base fields of characteristic $p$ is included. Counterexamples are systematically provided to test the sharpness of our results.

In this paper we establish a general form of the mass transference principle for systems of linear forms conjectured in 2009. We also present a number of applications of this result to problems in Diophantine approximation. These include a general transference of Lebesgue measure Khintchine–Groshev type theorems to Hausdorff measure statements. The statements we obtain are applicable in both the homogeneous and inhomogeneous settings as well as allowing transference under any additional constraints on approximating integer points. In particular, we establish Hausdorff measure counterparts of some Khintchine–Groshev type theorems with primitivity constraints recently proved by Dani, Laurent and Nogueira.

Let $K$ be the field of fractions of a local Henselian discrete valuation ring ${\mathcal{O}}_{K}$ of characteristic zero with perfect residue field $k$. Assuming potential semi-stable reduction, we show that an unramified Galois action on the second $\ell$-adic cohomology group of a K3 surface over $K$ implies that the surface has good reduction after a finite and unramified extension. We give examples where this unramified extension is really needed. Moreover, we give applications to good reduction after tame extensions and Kuga–Satake Abelian varieties. On our way, we settle existence and termination of certain flops in mixed characteristic, and study group actions and their quotients on models of varieties.

Let $X\subset \mathbb{P}^{4}$ be a terminal factorial quartic 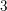$3$-fold. If 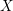$X$ is non-singular, 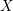$X$ is birationally rigid, i.e. the classical minimal model program on any terminal $\mathbb{Q}$-factorial projective variety 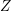$Z$ birational to $X$ always terminates with $X$. This no longer holds when 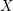$X$ is singular, but very few examples of non-rigid factorial quartics are known. In this article, we first bound the local analytic type of singularities that may occur on a terminal factorial quartic hypersurface $X\subset \mathbb{P}^{4}$. A singular point on such a hypersurface is of type $cA_{n}$ (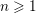$n\geqslant 1$), or of type $cD_{m}$ (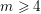$m\geqslant 4$) or of type 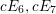$cE_{6},cE_{7}$ or 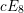$cE_{8}$. We first show that if 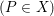$(P\in X)$ is of type 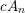$cA_{n}$, $n$ is at most 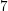$7$ and, if $(P\in X)$ is of type $cD_{m}$, 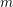$m$ is at most 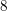$8$. We then construct examples of non-rigid factorial quartic hypersurfaces whose singular loci consist (a) of a single point of type 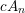$cA_{n}$ for 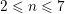$2\leqslant n\leqslant 7$, (b) of a single point of type $cD_{m}$ for 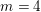$m=4$ or $5$ and (c) of a single point of type 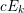$cE_{k}$ for $k=6,7$ or $8$.

We prove a Givental-style mirror theorem for toric Deligne–Mumford stacks ${\mathcal{X}}$. This determines the genus-zero Gromov–Witten invariants of ${\mathcal{X}}$ in terms of an explicit hypergeometric function, called the $I$-function, that takes values in the Chen–Ruan orbifold cohomology of ${\mathcal{X}}$.