The bipartite independence number of a graph 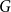 $G$, denoted as
$G$, denoted as 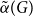 $\tilde \alpha (G)$, is the minimal number
$\tilde \alpha (G)$, is the minimal number 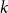 $k$ such that there exist positive integers
$k$ such that there exist positive integers  $a$ and
$a$ and 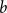 $b$ with
$b$ with 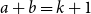 $a+b=k+1$ with the property that for any two disjoint sets
$a+b=k+1$ with the property that for any two disjoint sets 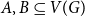 $A,B\subseteq V(G)$ with
$A,B\subseteq V(G)$ with  $|A|=a$ and
$|A|=a$ and 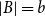 $|B|=b$, there is an edge between
$|B|=b$, there is an edge between 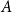 $A$ and
$A$ and 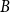 $B$. McDiarmid and Yolov showed that if
$B$. McDiarmid and Yolov showed that if 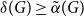 $\delta (G)\geq \tilde \alpha (G)$ then
$\delta (G)\geq \tilde \alpha (G)$ then 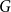 $G$ is Hamiltonian, extending the famous theorem of Dirac which states that if
$G$ is Hamiltonian, extending the famous theorem of Dirac which states that if 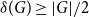 $\delta (G)\geq |G|/2$ then
$\delta (G)\geq |G|/2$ then  $G$ is Hamiltonian. In 1973, Bondy showed that, unless
$G$ is Hamiltonian. In 1973, Bondy showed that, unless 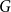 $G$ is a complete bipartite graph, Dirac’s Hamiltonicity condition also implies pancyclicity, i.e., existence of cycles of all the lengths from
$G$ is a complete bipartite graph, Dirac’s Hamiltonicity condition also implies pancyclicity, i.e., existence of cycles of all the lengths from  $3$ up to
$3$ up to  $n$. In this paper, we show that
$n$. In this paper, we show that  $\delta (G)\geq \tilde \alpha (G)$ implies that
$\delta (G)\geq \tilde \alpha (G)$ implies that 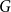 $G$ is pancyclic or that
$G$ is pancyclic or that 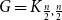 $G=K_{\frac{n}{2},\frac{n}{2}}$, thus extending the result of McDiarmid and Yolov, and generalizing the classic theorem of Bondy.
$G=K_{\frac{n}{2},\frac{n}{2}}$, thus extending the result of McDiarmid and Yolov, and generalizing the classic theorem of Bondy.
 ${\textit{d}}$-process
${\textit{d}}$-process