Hostname: page-component-745bb68f8f-kw2vx Total loading time: 0 Render date: 2025-02-06T22:29:18.230Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Diameters of Commuting Graphs Arising from Random Skew-Symmetric Matrices

Published online by Cambridge University Press:  04 February 2014

PETER HEGARTY  and
DMITRII ZHELEZOV
PETER HEGARTY
Affiliation:
Mathematical Sciences, Chalmers, 41296 Gothenburg, Sweden (e-mail: hegarty@chalmers.se, zhelezov@chalmers.se) Mathematical Sciences, University of Gothenburg, 41296 Gothenburg, Sweden
DMITRII ZHELEZOV
Affiliation:
Mathematical Sciences, Chalmers, 41296 Gothenburg, Sweden (e-mail: hegarty@chalmers.se, zhelezov@chalmers.se) Mathematical Sciences, University of Gothenburg, 41296 Gothenburg, Sweden
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We present a two-parameter family 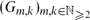$(G_{m,k})_{m, k \in \mathbb{N}_{\geq 2}}$, of finite, non-abelian random groups and propose that, for each fixed k, as m → ∞ the commuting graph of Gm,k is almost surely connected and of diameter k. We present heuristic arguments in favour of this conjecture, following the lines of classical arguments for the Erdős–Rényi random graph. As well as being of independent interest, our groups would, if our conjecture is true, provide a large family of counterexamples to the conjecture of Iranmanesh and Jafarzadeh that the commuting graph of a finite group, if connected, must have a bounded diameter. Simulations of our model yielded explicit examples of groups whose commuting graphs have all diameters from 2 up to 10.

Keywords

Primary 20P05
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 23 , Issue 3 , May 2014 , pp. 449 - 459
Copyright
Copyright © Cambridge University Press 2014 

References

[1]Alon, N. and Spencer, J. (2000) The Probabilistic Method, second edition, Wiley.CrossRefGoogle Scholar
[2]Bollobás, B. (1981) The diameter of random graphs. Trans. Amer. Math. Soc. 267 41–52.CrossRefGoogle Scholar
[3]Brauer, R. and Fowler, K. A. (1955) On groups of even order. Ann. of Math. (2) 62 565–583.CrossRefGoogle Scholar
[4]Giudici, M. and Parker, C. W. (2013) There is no upper bound for the diameter of the commuting graph of a finite group. J. Combin. Theory Ser. A 120 1600–1603.CrossRefGoogle Scholar
[5]Guidici, M. and Pope, A. (2013) On bounding the diameter of the commuting graph of a group. J. Group Theory (1) 17 131–149.Google Scholar
[6]Hegarty, P. and Zhelezov, D. Can commuting graphs of finite groups have arbitrarily large diameter? Preprint available at arXiv.org/abs/1204.5456.Google Scholar
[7]Iranmanesh, A. and Jafarzadeh, A. (2008) On the commuting graph associated with the symmetric and alternating groups. J. Algebra Appl. 7 129–146.CrossRefGoogle Scholar
[8]Klee, V. and Larman, D. (1981) Diameters of random graphs. Canad. J. Math. 33 618–640.CrossRefGoogle Scholar
[9]Morgan, G. L. and Parker, C. W. (2013) The diameter of the commuting graph of a finite group with trivial centre. J. Algebra 393 41–59.CrossRefGoogle Scholar
[10]Neumann, B. H. (1976) A problem of Paul Erdős on groups. J. Austral. Math. Soc. Ser. A 21 467–472.CrossRefGoogle Scholar
[11]Riordan, O. and Wormald, N. (2010) The diameter of sparse random graphs. Combin. Probab. Comput. 19 835–926.CrossRefGoogle Scholar
[12]Segev, Y. and Seitz, G. M. (2002) Anisotropic groups of type An and the commuting graph of finite simple groups. Pacific J. Math. 202 125–225.CrossRefGoogle Scholar