For linear nonuniform cellular automata (NUCA) which are local perturbations of linear CA over a group universe G and a finite-dimensional vector space alphabet V over an arbitrary field k, we investigate their Dedekind finiteness property, also known as the direct finiteness property, i.e., left or right invertibility implies invertibility. We say that the group G is $L^1$-surjunctive, resp. finitely $L^1$-surjunctive, if all such linear NUCA are automatically surjective whenever they are stably injective, resp. when in addition k is finite. In parallel, we introduce the ring $D^1(k[G])$ which is the Cartesian product $k[G] \times (k[G])[G]$ as an additive group but the multiplication is twisted in the second component. The ring $D^1(k[G])$ contains naturally the group ring $k[G]$ and we obtain a dynamical characterization of its stable finiteness for every field k in terms of the finite $L^1$-surjunctivity of the group G, which holds, for example, when G is residually finite or initially subamenable. Our results extend known results in the case of CA.

On post-critically finite self-similar sets, whose walk dimensions of diffusions are in general larger than 2, we find a sharp region where two classes of Besov spaces, the heat Besov spaces $B^{p,q}_\sigma (K)$ and the Lipschitz–Besov spaces $\Lambda ^{p,q}_\sigma (K)$, are identical. In particular, we provide concrete examples that $B^{p,q}_\sigma (K)=\Lambda ^{p,q}_\sigma (K)$ with $\sigma>1$. Our method is purely analytical, and does not involve heat kernel estimate.

We show that the first-order logical theory of the binary overlap-free words (and, more generally, the $\alpha $-free words for rational $\alpha $, $2 < \alpha \leq 7/3$), is decidable. As a consequence, many results previously obtained about this class through tedious case-based proofs can now be proved “automatically,” using a decision procedure, and new claims can be proved or disproved simply by restating them as logical formulas.

In this paper, a notion of non-microstate bi-free entropy with respect to completely positive maps is constructed thereby extending the notions of non-microstate bi-free entropy and free entropy with respect to a completely positive map. By extending the operator-valued bi-free structures to allow for more analytical arguments, a notion of conjugate variables is constructed using both moment and cumulant expressions. The notions of free Fisher information and entropy are then extended to this setting and used to show minima of the Fisher information and maxima of the non-microstate bi-free entropy at bi-R-diagonal elements.

Consider a minimal-free topological dynamical system $(X, \mathbb Z^d)$. It is shown that the radius of comparison of the crossed product C*-algebra $\mathrm {C}(X) \rtimes \mathbb Z^d$ is at most half the mean topological dimension of $(X, \mathbb Z^d)$. As a consequence, the C*-algebra $\mathrm {C}(X) \rtimes \mathbb Z^d$ is classified by the Elliott invariant if the mean dimension of $(X, \mathbb Z^d)$ is zero.

We use the cobordism distance on the smooth knot concordance group $\mathcal {C}$ to measure how close two knots are to being linearly dependent. Our measure, $\Delta (\mathcal {K}, \mathcal {J})$, is built by minimizing the cobordism distance between all pairs of knots, $\mathcal {K}'$ and $\mathcal {J}'$, in cyclic subgroups containing $\mathcal {K}$ and $\mathcal {J}$. When made precise, this leads to the definition of the projective space of the concordance group, ${\mathbb P}(\mathcal {C})$, upon which $\Delta $ defines an integer-valued metric. We explore basic properties of ${\mathbb P}(\mathcal {C})$ by using torus knots $T_{2,2k+1}$. Twist knots are used to demonstrate that the natural simplicial complex $\overline {({\mathbb P}(\mathcal {C}), \Delta )}$ associated with the metric space ${\mathbb P}(\mathcal {C})$ is infinite-dimensional.

We prove quantitative bounds for the inverse theorem for Gowers uniformity norms $\mathsf {U}^5$ and $\mathsf {U}^6$ in $\mathbb {F}_2^n$. The proof starts from an earlier partial result of Gowers and the author which reduces the inverse problem to a study of algebraic properties of certain multilinear forms. The bulk of the work in this paper is a study of the relationship between the natural actions of $\operatorname {Sym}_4$ and $\operatorname {Sym}_5$ on the space of multilinear forms and the partition rank, using an algebraic version of regularity method. Along the way, we give a positive answer to a conjecture of Tidor about approximately symmetric multilinear forms in five variables, which is known to be false in the case of four variables. Finally, we discuss the possible generalization of the argument for $\mathsf {U}^k$ norms.

Inspired by a remarkable work of Félix, Halperin, and Thomas on the asymptotic estimation of the ranks of rational homotopy groups, and more recent works of Wu and the authors on local hyperbolicity, we prove two asymptotic formulae for torsion rank of homotopy groups, one using ordinary homology and one using K-theory. We use these to obtain explicit quantitative asymptotic lower bounds on the torsion rank of the homotopy groups for many interesting spaces after suspension, including Moore spaces, Eilenberg–MacLane spaces, complex projective spaces, complex Grassmannians, Milnor hypersurfaces, and unitary groups.

In this article, we are concerned with the tempered fractional parabolic problem

$$ \begin{align*}\frac{\partial u}{\partial t}(x, t)-\left(\Delta+\lambda\right)^{\frac{\alpha}{2}} u(x, t)=f(u(x, t)), \end{align*} $$

where $-\left (\Delta +\lambda \right )^{\frac {\alpha }{2}}$ is a tempered fractional operator with $\alpha \in (0,2)$ and $\lambda $ is a sufficiently small positive constant. We first establish maximum principle principles for problems involving tempered fractional parabolic operators. And then, we develop the direct sliding methods for the tempered fractional parabolic problem, and discuss how they can be used to establish monotonicity results of solutions to the tempered fractional parabolic problem in various domains. We believe that our theory and methods can be conveniently applied to study parabolic problems involving other nonlocal operators.

Let $\mathcal {F}$ be a hereditary collection of finite subsets of 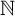$\mathbb {N}$. In this paper, we introduce and characterize $\mathcal {F}$-(almost) greedy bases. Given such a family 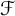$\mathcal {F}$, a basis 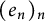$(e_n)_n$ for a Banach space X is called 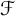$\mathcal {F}$-greedy if there is a constant 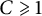$C\geqslant 1$ such that for each 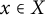$x\in X$, 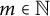$m \in \mathbb {N}$, and 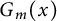$G_m(x)$, we have

$$ \begin{align*} \|x - G_m(x)\|\ \leqslant\ C \inf\left\{\left\|x-\sum_{n\in A}a_ne_n\right\|\,:\, |A|\leqslant m, A\in \mathcal{F}, (a_n)\subset \mathbb{K}\right\}. \end{align*} $$

$G_m(x)$

$\mathbb {K}$

$\mathcal {F}$

$\mathcal {F}$

$\mathcal {F}$

$\mathcal {F}$

$\mathcal {F}$

$\mathcal {F}$

Furthermore, we provide several examples of bases that are nontrivially 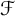$\mathcal {F}$-greedy. For a countable ordinal $\alpha $, we consider the case 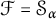$\mathcal {F}=\mathcal {S}_{\alpha }$, where $\mathcal {S}_{\alpha }$ is the Schreier family of order 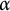$\alpha $. We show that for each $\alpha $, there is a basis that is $\mathcal {S}_{\alpha }$-greedy but is not 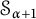$\mathcal {S}_{\alpha +1}$-greedy. In other words, we prove that none of the following implications can be reversed: for two countable ordinals 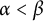$\alpha < \beta $,

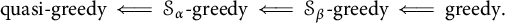$$ \begin{align*} \mbox{quasi-greedy}\ \Longleftarrow\ \mathcal{S}_{\alpha}\mbox{-greedy}\ \Longleftarrow\ \mathcal{S}_{\beta}\mbox{-greedy}\ \Longleftarrow\ \mbox{greedy}. \end{align*} $$

We show that the total variation mixing time is not quasi-isometry invariant, even for Cayley graphs. Namely, we construct a sequence of pairs of Cayley graphs with maps between them that twist the metric in a bounded way, while the ratio of the two mixing times goes to infinity. The Cayley graphs serving as an example have unbounded degrees. For non-transitive graphs, we construct bounded degree graphs for which the mixing time from the worst starting point for one graph is asymptotically smaller than the mixing time from the best starting point of the random walk on a network obtained by increasing some of the edge weights from 1 to $1+o(1)$.

We extend classical results of Perego and Rapagnetta on moduli spaces of sheaves of type OG10 to moduli spaces of Bridgeland semistable objects on the Kuznetsov component of a cubic fourfold. In particular, we determine the period of this class of varieties and use it to understand when they become birational to moduli spaces of sheaves on a K3 surface.