Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-02-11T05:44:38.306Z Has data issue: false hasContentIssue false
  • English
  • Français

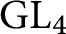A proof of the linear Arithmetic Fundamental Lemma for $ \operatorname {{\mathrm {GL}}}_4$

Part of: Forms and linear algebraic groups Arithmetic algebraic geometry Cycles and subschemes Algebraic combinatorics

Published online by Cambridge University Press:  23 November 2020

Qirui Li
Qirui Li*
Affiliation:
Department of Mathematics, University of Toronto, Toronto, Canada
*
e-mail: qiruili@math.utoronto.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 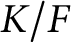$K/F$ be an unramified quadratic extension of a non-Archimedean local field. In a previous work [1], we proved a formula for the intersection number on Lubin–Tate spaces. The main result of this article is an algorithm for computation of this formula in certain special cases. As an application, we prove the linear Arithmetic Fundamental Lemma for $ \operatorname {{\mathrm {GL}}}_4$ with the unit element in the spherical Hecke Algebra.

Keywords

Double structuresorbital integralL-functionintegration

MSC classification

Primary: 14C17: Intersection theory, characteristic classes, intersection multiplicities
Secondary: 11G99: None of the above, but in this section 14C25: Algebraic cycles 05E15: Combinatorial aspects of groups and algebras 11E45: Analytic theory (Epstein zeta functions; relations with automorphic forms and functions)
Type
Article
Information
Canadian Journal of Mathematics , Volume 74 , Issue 2 , April 2022 , pp. 381 - 427
Check for updates
Copyright
© Canadian Mathematical Society 2020

References

Li, Q., An intersection number formula for CM cycles in Lubin–Tate towers. Preprint, 2018. http://arxiv.org/1803.07553 Google Scholar
Zhang, W., A conjectural linear Arithmetic Fundamental Lemma for Lubin–Tate space. Unpublished note.Google Scholar