No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 09 July 2019
The aim of this paper is to find a broad family of means defined on a subinterval of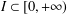 
$I\subset [0,+\infty )$ such that Equivalently, the averaging operator 
$$\begin{eqnarray}\mathop{\sum }_{n=1}^{\infty }\mathscr{M}(a_{1},\ldots ,a_{n})<+\infty \quad \text{for all }a\in \ell _{1}(I).\end{eqnarray}$$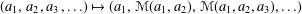
$(a_{1},\,a_{2},a_{3}\,,\ldots )\mapsto (a_{1},\,\mathscr{M}(a_{1},a_{2}),\,\mathscr{M}(a_{1},a_{2},a_{3}),\ldots )$ is a selfmapping of 
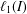$\ell _{1}(I)$. This property is closely related to the so-called Hardy inequality for means (which additionally requires boundedness of this operator). We prove that these two properties are equivalent in a broad family of so-called Gini means. Moreover, we show that this is not the case for quasi-arithmetic means, that is functions 
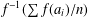$f^{-1}(\sum f(a_{i})/n)$, where 
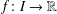$f:I\rightarrow \mathbb{R}$ is continuous and strictly monotone, 
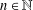$n\in \mathbb{N}$ and 
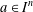$a\in I^{n}$. However, the weak Hardy property is localisable for this family.
You have
Access