In this paper, we consider a notion of nonmeasurablity with respect to Marczewski and Marczewski-like tree ideals 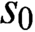$s_0$ , $m_0$ , $l_0$ , $cl_0$ , $h_0,$ and $ch_0$ . We show that there exists a subset of the Baire space $\omega ^\omega ,$ which is s-, l-, and m-nonmeasurable that forms a dominating m.e.d. family. We investigate a notion of 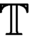${\mathbb {T}}$ -Bernstein sets—sets which intersect but do not contain any body of any tree from a given family of trees ${\mathbb {T}}$ . 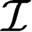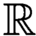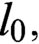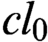We also obtain a result on ${\mathcal {I}}$ -Luzin sets, namely, we prove that if ${\mathfrak {c}}$ is a regular cardinal, then the algebraic sum (considered on the real line ${\mathbb {R}}$ ) of a generalized Luzin set and a generalized Sierpiński set belongs to $s_0, m_0$ , $l_0,$ and $cl_0$ .